Regional Ionospheric Modeling and Accuracy Assessment Using GNSS/LEO Satellites Observations
-
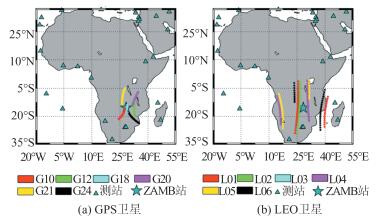
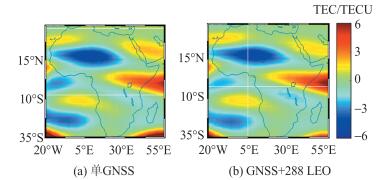
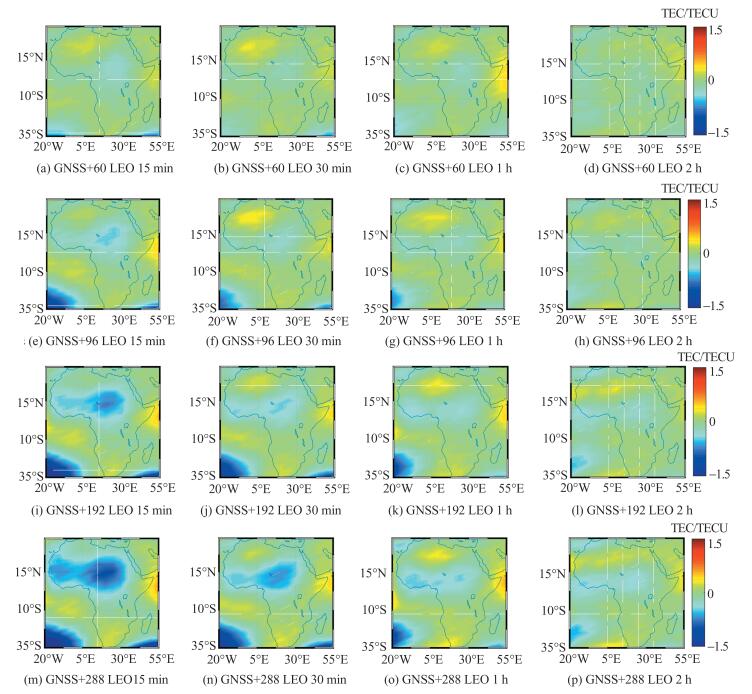
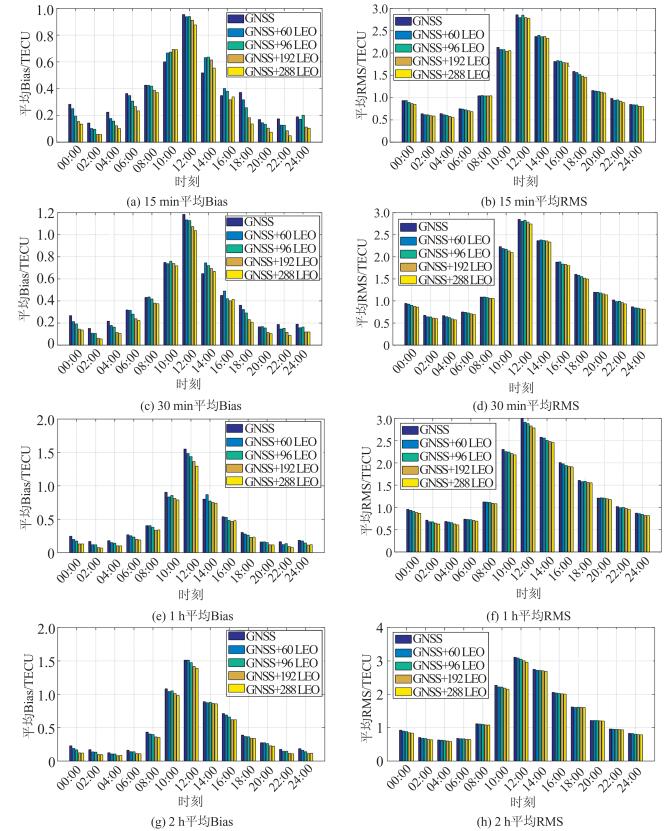
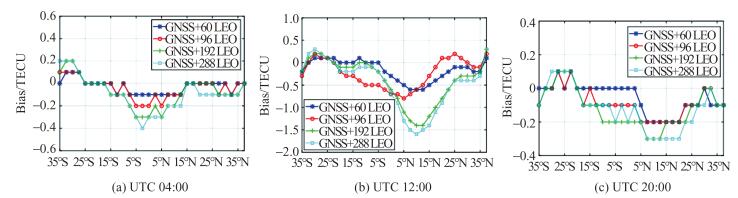
摘要: 高精度的电离层模型对于提高导航卫星系统的定位精度具有重要意义。低轨卫星的快速发展为建立高精度的电离层模型提供了新的契机。基于仿真数据模拟获得2017年1月1日—30日LEO（low earth orbit）和GNSS（global navigation satellite system）卫星观测数据，星座类型包括60、96、192和288颗卫星，以非洲区域为例，利用该数据研究GNSS和LEO卫星穿刺点的覆盖情况和联合建模精度。结果表明：加入LEO卫星后，穿刺点分布改善明显，能够大幅度提高穿刺点密度；单颗低轨卫星穿刺点的范围比GNSS卫星大，LEO卫星的高度角和方位角变化明显；随着低轨卫星数量的增加，融合建模的精度也随之提高；在12:00时东经30°不同纬度范围内，单GNSS建模和GNSS+288 LEO建模差值最大为-1.6 TECU（total electron content unit）；随着建模时长的增加，融合建模结果和单GNSS结果差值逐渐变小。
-
关键词:
- 联合GNSS/LEO卫星 /
- IRI模型 /
- 低轨卫星星座 /
- 电离层建模 /
- 数据仿真
Abstract: High-precision ionospheric model is of great significance for improving the positioning accuracy of navigation satellite system. With the rapid development of low earth orbit satellites, the establishment of a high-precision ionospheric model has provided new opportunities. Based on the simulation data, this paper obtains LEO (low earth orbit) and GNSS (global navigation satellite system) satellites observation data of January 1 to 30, 2017 by means of simulation. The constellation types include 60, 96, 192 and 288 satellites respectively. Based on these data and taking African region as an example, the coverage of GNSS and LEO satellites' ionospheric pierce points and the joint modeling accuracy are studied. The results show that, after LEO satellites are added, the distribution of ionospheric pierce points is significantly improved, which leads to a noticeable rise of their density. The range of ionospheric pierce points of single low-orbit satellite is larger than that of GNSS satellite, and the altitude angle and azimuth angle of LEO satellite change remarkably. With the increase of the number of low-orbit satellites, the accuracy of joint modeling also rises up. Within different latitudes of 30°E at UTC 12:00, the difference between GNSS-only and GNSS+288 LEO ionospheric modeling results is the largest, reaching -1.6 TECU. With the increase of modeling time, the difference between the joint modeling results and GNSS-only results gradually decreases. -
长航时高精度自主导航是发展水下自主航行器亟待解决的技术难题。惯性导航系统(inertial navigation system，INS)作为水下航行器的核心导航设备之一，因其存在随时间变化产生的累积误差，无法满足水下航行器长航时自主导航的高精度要求。地磁导航技术可以为水下航行器提供高隐蔽性的外部校正信息，修正由INS产生的累积误差[1-3]。地磁辅助惯性导航系统涉及到背景磁场构建、地磁导航匹配算法和地磁适配性分析等多项关键技术。适配性是指地磁图对匹配的适应性，即地磁场特征在相关匹配中能够表征地理位置的能力。通过对地磁图适配性的分析，挑选地磁特征显著、信息量丰富、适应性强的区域作为地磁适配区，能够有效提高地磁导航精度[4-7]。
为了解决多指标对单一决策的综合影响，目前已有诸多研究提出了分析方法，文献[8]提出了多属性决策评价地磁适配性的方法，该方法综合考虑地磁标准差、地磁粗糙度、地磁相关系数、地磁信息熵4个特征参数得到综合评价值，与匹配概率有较高的一致性；文献[9]提出了分形维数的评价方法，该方法从原理上分析了分形维数与适配性的关系，发现分形维数在适配性分析上具有很好的性能；文献[10]提出了基于粗糙集理论的地磁特征参数的选取方法，该方法能为适配区评价选取重要特征参数；文献[11]针对人为提取的特征不能客观描述深层次结构性特征的问题，提出了基于深度卷积神经网络的适配性分析方法，可以有效避免地磁特征人工提取的主观性；通过对地磁共生矩阵反演出的角二阶矩、对比度等统计参数的分析，文献[12]提出了基于地磁共生矩阵的地磁适配区选择算法，从多方向反映地磁变化特征以及地磁邻近区域的相关性；文献[13]提出了层次分析方法，定义了地磁场特征参数可信度，分析了特征参数的隐含信息，发现可信度比单一特征参数适配性评价效果更好。从定量角度可以将上述方法归纳为基于决策选优的适配性分析方法，排序和选优的思想是此类方法最显著的特征。通过排序来明确各候选匹配区适配性能的优劣，选优即选取适配性能好的区域作为适配区，然而这类方法存在人为设置阈值和判定准则难提取两个缺点。因此，为了使提取的准则更为客观，本文将模糊决策理论应用到地磁图适配性分析中。
模糊决策理论是运用模糊数学方法处理多目标决策问题，在分析过程中，这些多目标变量属于模糊因素[14]。本文基于地磁图适配性分析中存在多指标性和模糊性的特点，提出了基于模糊决策理论的地磁图适配性分析方法，对特征参数的模糊度指标进行加权分析，构建综合评价值来评定候选区适配性。针对地磁异常的无迹卡尔曼滤波(unscented Kalman filtering，UKF)算法，选取中国南海部分海域的全球地磁异常格网数据(earth magnetic anomaly grid 2，EMAG2)作为地磁异常基准图进行仿真实验，验证了定量分析结果的可靠性和该方法的可行性。
1 地磁图特征参数定义
本文将地磁场数据以格网的形式存储于计算机中，用离散点的形式表示地磁场在空间中的变化规律。设某地磁场区域的经纬度跨度为M×N网格(M为纬度跨度，N为经度跨度)，f(i, j)为网格点坐标(i, j)处的地磁异常值。描述地磁图适配性的特征参数多达十几种，根据地磁图特征参数与匹配概率的关联程度，本文选取地磁标准差、粗糙度、相关系数、坡度标准差和地磁信息熵作为评价候选匹配区适配性能的度量。上述5个地磁图特征参数的定义及其计算方法如下[15]。
1) 地磁标准差σ
地磁标准差反映了该区域地磁场离散程度和地磁场总体起伏情况。地磁标准差数值越大，表示该候选匹配区的地磁特征越明显，越适合做地磁匹配。其定义如下：
$$\sigma = \sqrt {\frac{1}{{MN - 1}}\mathop \sum \limits_{i = 1}^M \mathop \sum \limits_{j = 1}^N {{\left( {f\left( {i, j} \right) - \bar f} \right)}^2}} $$ (1) 式中，$\bar f = \frac{1}{{MN}}\mathop \sum \limits_{i = 1}^M \mathop \sum \limits_{j = 1}^N f\left({i, j} \right)$，表示候选匹配区内地磁场的均值。
2) 地磁粗糙度r
地磁粗糙度反映了候选匹配区地磁场的平均光滑程度和地磁场局部起伏状态。地磁粗糙度越大，表示该候选匹配区的地磁信息量越丰富，越有利于进行地磁匹配。其定义如下：
$$r = \frac{{{r_x} + {r_y}}}{2} $$ (2) $${r_x} = \sqrt {\frac{1}{{M\left( {N - 1} \right)}}\mathop \sum \limits_{i = 1}^M \mathop \sum \limits_{j = 1}^{N - 1} {{[f\left( {i, j} \right) - f\left( {i, j + 1} \right)]}^2}} $$ (3) $${r_y} = \sqrt {\frac{1}{{\left( {M - 1} \right)N}}\mathop \sum \limits_{i = 1}^{M - 1} \mathop \sum \limits_{j = 1}^N {{[f\left( {i, j} \right) - f\left( {i + 1, j} \right)]}^2}} $$ (4) 式中，rx表示该区域x方向粗糙度；ry表示该区域y方向粗糙度。
3) 相关系数ρ
地磁相关系数反映了地磁数据之间的独立性。地磁相关系数越小，表明该区域地磁信息越丰富，越有利于地磁匹配定位。定义如下：
$$\rho = \frac{{{\rho _x} + {\rho _y}}}{2} $$ (5) $${\rho _x} = \frac{1}{{M\left( {N - 1} \right){\sigma ^2}}}\mathop \sum \limits_{i = 1}^M \mathop \sum \limits_{j = 1}^{N - 1} \left[ {f\left( {i, j} \right) - \bar f} \right] \cdot \left[ {f\left( {i, j + 1} \right) - \bar f} \right] $$ (6) $${\rho _y} = \frac{1}{{\left( {M - 1} \right)N{\sigma ^2}}}\mathop \sum \limits_{i = 1}^{M - 1} \mathop \sum \limits_{j = 1}^N \left[ {f\left( {i, j} \right) - \bar f} \right] \cdot \left[ {f\left( {i + 1, j} \right) - \bar f} \right] $$ (7) 式中，ρx表示该区域x方向的相关系数；ρy表示该区域y方向的相关系数。
4) 坡度标准差S
地磁坡度S(i, j)为地磁场曲面上一点处的法线方向和垂直方向的夹角，可由地磁场在纬度方向、经度方向的变化率Sx(i, j)、Sy(i, j)确定，其定义如下：
$$\begin{array}{*{20}{c}} {{S_x}\left( {i, j} \right) = [f\left( {i + 1, j + 1} \right) + f\left( {i, j + 1} \right) + }\\ {f\left( {i - 1, j + 1} \right) - f\left( {i + 1, j - 1} \right) - }\\ {f\left( {i, j - 1} \right) - f\left( {i - 1, j - 1} \right)]/6} \end{array} $$ (8) $$\begin{array}{*{20}{c}} {{S_y}\left( {i, j} \right) = [f\left( {i + 1, j + 1} \right) + f\left( {i + 1, j} \right) + }\\ {f\left( {i + 1, j - 1} \right) - f\left( {i - 1, j + 1} \right) - }\\ {f\left( {i - 1, j} \right) - f\left( {i - 1, j - 1} \right)]/6} \end{array} $$ (9) $$S\left( {i, j} \right) = \sqrt {\left[ {{S_x}\left( {i, j} \right){]^2} + } \right[{S_y}\left( {i, j} \right){]^2}} $$ (10) 地磁坡度S(i, j)是针对候选匹配区中每一个格网点而言的，为了能够反映整个候选匹配区的地磁坡度情况，引入坡度标准差Sσ，其定义如下：
$${S_\sigma } = \sqrt {\frac{1}{{\left( {M - 2} \right)\left( {N - 2} \right) - 1}}\mathop \sum \limits_{i = 2}^{M - 1} \mathop \sum \limits_{j = 2}^{N - 1} {{(S\left( {i, j} \right) - \bar S)}^2}} $$ (11) $$\bar S = \frac{1}{{\left( {M - 2} \right)\left( {N - 2} \right)}}\mathop \sum \limits_{i = 2}^{M - 1} \mathop \sum \limits_{j = 2}^{N - 1} S\left( {i, j} \right) $$ (12) 5) 地磁信息熵H
地磁信息熵越小，代表地磁场特征越独特，该区域越有利于进行地磁匹配定位。其定义如下：
$$H = - \mathop \sum \limits_{i = 1}^M \mathop \sum \limits_{j = 1}^N p\left( {i, j} \right) \cdot {\rm{lo}}{{\rm{g}}_2}\;p\left( {i, j} \right) $$ (13) $$p\left( {i, j} \right) = \frac{{f\left( {i, j} \right)}}{{\mathop \sum \limits_{i = 1}^M \mathop \sum \limits_{j = 1}^N f\left( {i, j} \right)}} $$ (14) 当对数的底数取2时，熵的单位为bit。
2 基于模糊决策理论的地磁图适配性分析
为了综合考虑地磁标准差、粗糙度、相关系数、坡度标准差及地磁信息熵等5个特征参数对适配性分析的影响，首先构建指标矩阵，然后构建隶属度矩阵对这5种不同类属的特征参数进行模糊控制，最后引入权重向量进一步提高综合评价矩阵的合理性[16-17]。
1) 构建指标矩阵X
设有m块待选区域，有n个评价指标来描述，则有m×n阶指标矩阵，其定义如下：
$$\mathit{\boldsymbol{X}} = \left[ {\begin{array}{*{20}{c}} {{x_{11}}}&{{x_{12}}}& \cdots &{{x_{1n}}}\\ {{x_{21}}}&{{x_{22}}}& \cdots &{{x_{2n}}}\\ \vdots & \vdots &{}& \vdots \\ {{x_{m1}}}&{{x_{m2}}}& \cdots &{{x_{mn}}} \end{array}} \right] = {({x_{ij}})_{m \times n}} $$ (15) 式中，xij表示第i个待分析对象的第j个评价因素的指标特征量，$i = 1, 2 \cdots m, j = 1, 2 \cdots n$。
2) 指标归一化
隶属度rij'的计算如下：
$$r_{i j}^{\prime}=\left\{\begin{array}{l} x_{i j} / x_{\mathrm{max}}, \text { 越大越优型 } \\ x_{\min } / x_{i j}, \text { 越小越优型 } \end{array}\right. $$ (16) 式中，xmax表示xij的最大值；xmin表示xij的最小值。
地磁标准差、地磁粗糙度、地磁坡度属于越大越优型，相关系数及地磁信息熵则属于越小越优型。由于各属性的量纲和取值范围相差较大，需要将不同属性的量纲进行标准化处理，使标准化转换后的评价值rij均值为0，方差为1。计算如下：
$$r_{i j}=\frac{r_{i j}^{\prime}-m_{j}}{\sigma_{j}} $$ (17) $$m_{j}=\frac{1}{m} \sum\limits_{i=1}^{m} r_{i j}^{\prime} $$ (18) $$\sigma_{j}=\sqrt{\frac{1}{m-1} \sum\limits_{i=1}^{m}\left(r_{i j}^{\prime}-m_{j}\right)^{2}} $$ (19) 式中，mj为第j个属性的均值；σj表示第j个属性值的标准差。
3) 多目标模糊关联矩阵的构造
根据式(14)构建指标隶属度矩阵Rm×n，其定义如下：
$${\mathit{\boldsymbol{R}}_{m \times n}} = \left[ {\begin{array}{*{20}{c}} {{r_{11}}}&{{r_{12}}}& \cdots &{{r_{1n}}}\\ {{r_{21}}}&{{r_{22}}}& \cdots &{{r_{2n}}}\\ \vdots & \vdots &{}& \vdots \\ {{r_{m1}}}&{{r_{m2}}}& \cdots &{{r_{mn}}} \end{array}} \right] $$ (20) 式中，$i = 1, 2 \cdots m; j = 1, 2 \cdots n$。
4) 权重向量的确定
由于各指标对适配性的影响程度各不相同，为实现综合评价结果的合理性，必须对各指标进行加权处理，定义权向量w为：
$$\mathit{\boldsymbol{w}} = \left( {{w_1}, {w_2} \cdots {w_m}} \right) = \left( {\frac{{{s_1}}}{{\mathop \sum \limits_{i = 1}^m {s_i}}}, \frac{{{s_2}}}{{\mathop \sum \limits_{i = 1}^m {s_i}}} \cdots \frac{{{s_m}}}{{\mathop \sum \limits_{i = 1}^m {s_i}}}} \right){\rm{}} $$ (21) $${s_i} = {\rm{exp}}\left[ {\mu \mathop \sum \limits_{j = 1}^n \mathop \sum \limits_{k = 1}^n \left| {{r_{ij}} - {r_{ik}}} \right|/\left( {1 - \mu } \right) - 1} \right]{\rm{}} $$ (22) 式中，μ代表平衡系数(0 < μ < 1)。
5) 综合评判矩阵
将式(21)所得权重矩阵与式(20)所得隶属度矩阵组合构成综合评判矩阵V，其定义如下：
$$\mathit{\boldsymbol{V}} = \mathit{\boldsymbol{wR}} = \left( {{w_1}, {w_2} \cdots {w_m}} \right)\left[ {\begin{array}{*{20}{c}} {{r_{11}}}&{{r_{12}}}& \cdots &{{r_{1n}}}\\ {{r_{21}}}&{{r_{22}}}& \cdots &{{r_{2n}}}\\ \vdots & \vdots &{}& \vdots \\ {{r_{m1}}}&{{r_{m2}}}& \cdots &{r_{mn}^{}} \end{array}} \right] $$ (23) 3 数据计算及结果评定
本文选取分辨率为2″的全球地磁异常格网数据EMAG2，将范围为108°E~113°E、10°N~15°N的中国南海部分海域地磁异常格网数据作为实验的地磁异常基准图，如图 1所示，该地区的地磁图特征样式繁多，很难直观对各区域的适配性优劣作出直接判断。
将图 1所示区域等间隔划分为16个候选匹配区域，以便进行计算及结果分析，候选匹配区域1~16的地磁图特征参数统计量见表 1，表 1同时给出了相应地磁图特征参数的数值大小排序结果。
表 1 单一地磁图特征参数值及其排序Table 1. Rank Results of Single Geomagnetic Map Feature Parameter区域编号 标准差σ 粗糙度r 相关系数 坡度标准差S 信息熵H 数值/nT 排序 数值 排序 数值 排序 数值 排序 数值/bit 排序 1 4.144 9 9 0.933 9 12 0.992 1 5 1.342 2 10 10.112 7 5 2 3.520 3 11 1.530 7 3 0.977 2 14 1.856 7 6 10.014 2 13 3 4.374 3 8 1.771 3 2 0.994 7 3 2.304 1 2 9.823 9 16 4 3.708 0 10 1.131 8 8 0.991 6 6 1.432 7 8 10.113 7 4 5 4.409 5 7 0.960 3 10 0.987 8 8 1.094 6 13 10.180 2 1 6 2.698 5 14 0.866 9 13 0.987 3 9 1.274 9 11 10.024 0 12 7 6.987 7 2 1.049 4 9 0.996 0 1 1.481 2 7 10.000 0 14 8 4.459 7 5 0.845 5 14 0.994 4 4 0.969 7 14 10.150 5 2 9 2.649 9 15 2.067 4 1 0.960 9 15 2.829 0 1 10.041 4 10 10 2.865 5 12 1.286 5 5 0.984 2 11 1.861 3 4 10.059 7 8 11 2.753 5 13 0.392 0 16 0.985 5 10 0.602 6 16 10.092 6 6 12 4.416 4 6 1.247 4 6 0.978 6 13 1.857 8 5 10.031 4 11 13 2.552 3 16 0.935 5 11 0.958 7 16 1.242 5 12 9.943 1 15 14 7.359 4 1 1.339 9 4 0.995 2 2 1.908 8 3 10.062 0 7 15 4.815 8 4 0.742 0 15 0.990 8 7 0.896 8 15 10.041 8 9 16 5.330 5 3 1.158 5 7 0.983 6 12 1.414 7 9 10.117 2 3 由式(15)~(23)求解可得各适配区的综合评定结果，根据计算值大小对各区域适配性优劣进行排序，结果如表 2所示。
表 2 各候选匹配区综合评定值及其排序Table 2. Rank Results of Candidate Matching Regions区域编号 综合评定值 排序 1 2.033 5 10 2 2.774 0 6 3 3.431 6 2 4 2.165 1 8 5 1.680 1 13 6 1.927 8 11 7 2.291 1 7 8 1.499 8 14 9 4.170 7 1 10 2.775 5 4 11 0.954 0 16 12 2.775 1 5 13 1.869 3 12 14 2.872 6 3 15 1.394 1 15 16 2.144 4 9 由表 1可知，若分别以地磁粗糙度、地磁坡度作为评价依据，9号区域适配性最好；若以地磁信息熵作为评价依据，3号区域适配性最优。由此可知，利用单一特征参数作为评价依据所得结果并不一致。因此，单一地磁图特征参数作为候选匹配区适配性评价依据进行分析存在不合理性，而基于模糊决策理论能够综合主要特征参数进行加权分析，构建出综合评价值来评定候选匹配区的适配性。
为了验证基于模糊决策理论的地磁图适配性评估方法所得综合评定结果的正确性和可靠性，本文采用基于地磁异常的UKF滤波算法，选取16个候选匹配区当中的9号(排序1)、4号(排序8)和11号(排序16)3块区域进行仿真实验。在航迹仿真实验中，设置了航迹1和航迹2两条航迹进行验证分析。水下自主航行器(autonomous underwater vehicle，AUV)的运动状态如下：先进行匀加速运动，再匀速运动，然后转弯，最后变成匀速状态。惯导航迹仿真参数设置如表 3所示，区域9、4、11的仿真航迹结果分别如图 2、图 3、图 4所示。
表 3 仿真参数设置Table 3. Simulation Parameter Setting参数 参数值 惯性导航初始经度误差/n mile 2 惯性导航初始纬度误差/n mile 2 陀螺零偏/((°)·h-1) 0.01 加表零偏/(m·s-2) 1×10-5 AUV匀速运动速度/(n mile·h-1) 9.7 AUV偏航角/(°) 50 AUV航行时间/h 10.29 惯性导航初始状态方差/(°)2 0.001 地磁图噪声方差/(nT)2 10 地磁测量噪声方差/(nT)2 10 综合两条航迹，对9号、4号以及11号候选匹配区分别沿经度和纬度方向对基于UKF滤波方法的绝对匹配误差进行统计，结果如表 4所示。
表 4 绝对匹配误差统计值Table 4. Statistics of Absolute Matching Errors方向 编号 最大值/n mile 最小值/n mile 平均值/n mile 均方误差/n mile 纬向 9 1.869 6 0.003 1 0.935 7 0.332 0 4 4.493 2 0.003 2 2.332 3 2.186 0 11 18.897 5 0.028 6 10.584 4 6.002 7 经向 9 1.375 1 0.009 8 0.892 8 0.384 7 4 4.895 4 0.010 2 2.707 0 2.133 4 11 12.907 7 0.106 5 8.146 9 5.899 1 由图 2~4可知，9号区域较4号区域的匹配航迹更接近真实轨迹，11号区域则在匹配过程中出现误匹配现象。由表 4仿真实验结果可得，综合评价值更高的区域，纬向和经向绝对匹配误差也越小。实验结果表明，综合评价值高的区域其适配性能更好，可以选取相应区域作为适配区。
4 结语
本文提出了基于模糊决策理论的地磁图适配性分析方法，对图 1中的各个候选匹配区的适配性进行定量分析，得出综合评价值作为评价依据，并且进行航迹仿真实验验证了该方法的可靠性。实验结果表明，基于模糊决策的地磁图适配性分析结果与仿真实验结果具有较好的一致性，且综合评价值越高，匹配效果越好。且该方法解决了仅依据单一评价指标进行分析的不全面问题，得出综合评价值作为地磁图适配性优劣评判的定量分析依据，对航迹规划问题也具有一定指导意义。
致谢: 本研究的数值计算得到了武汉大学超级计算中心的支持和帮助，在此表示感谢。 -
![]()
图 1 6颗GPS卫星和6颗LEO卫星穿刺点分布情况
Figure 1. Distribution of Ionospheric Pierce Points of 6 GPS satellites and 6 LEO Satellites
![]()
图 2 GPS和LEO卫星的高度角和方位角对比
Figure 2. Comparison of Elevation Angles and Azimuth Angles Between GPS and LEO Satellites
![]()
图 3 GNSS、60颗LEO、96颗LEO、192颗LEO和288颗LEO的不同时长穿刺点分布情况
Figure 3. Distribution of IPPs at Different Durations of GNSS, 60, 96, 192 and 288 LEO Satellites
![]()
图 4 单GNSS和GNSS+288 LEO建模结果与IRI-GIM的差值
Figure 4. Differences of GNSS-only and GNSS+288 LEO Ionospheric Modeling Results with IRI-GIM
![]()
图 5 单GNSS与GNSS+60 LEO、GNSS+96 LEO、GNSS+192 LEO、GNSS+288 LEO不同时长建模结果的差值
Figure 5. Differences of Ionospheric Modeling Results at Different Durations of GNSS-Only, GNSS+60 LEO, GNSS+96 LEO, GNSS+192 LEO and GNSS+288 LEO
![]()
图 6 单GNSS、GNSS+60 LEO、GNSS+96 LEO、GNSS+192 LEO和GNSS+288 LEO不同时长建模结果的平均Bias和平均RMS值
Figure 6. Mean Biases and Mean RMSs of Ionospheric Modeling Results at Different Durations of GNSS-only, GNSS+60 LEO, GNSS+96 LEO, GNSS+192 LEO and GNSS+288 LEO
-
[1] Schaer S. Mapping and Predicting the Earths Ionosphere Using the Global Positioning System [D]. Bern, Switzerland: University of Bern, 1999
[2] 蔡昌盛, 高井祥, 李征航.利用GPS监测电离层总电子含量的季节性变化[J].武汉大学学报·信息科学版, 2006, 31(5): 451-453 http://ch.whu.edu.cn/article/id/2462 Cai Changsheng, Gao Jingxiang, Li Zhenghang. Monitoring Seasonal Variations of Ionospheric TEC Using GPS Measurement[J]. Geomatics and Information Science of Wuhan University, 2006, 31(5): 451-453 http://ch.whu.edu.cn/article/id/2462
[3] 霍星亮.基于GNSS的电离层形态监测与延迟模型研究[D].武汉: 中国科学院测量与地球物理研究所, 2008 Huo Xingliang. Research on Ionospheric Morphology Monitoring and Delay Model Based on GNSS[D]. Wuhan: Institute of Geodesy and Geophysics, Chinese Academy of Sciences, 2008
[4] 李征航, 陈锴, 刘万科, 等. GNSS电离层延迟模型的数学统一与方法扩展[J].武汉大学学报·信息科学版, 2007, 32(8): 699-703 http://ch.whu.edu.cn/article/id/1967 Li Zhenghang, Chen Kai, Liu Wanke, et al. Mathematical Unification and Method Expansion of GNSS Ionospheric Delay Model[J]. Geomatics and Information Science of Wuhan University, 2007, 32(8):699-703 http://ch.whu.edu.cn/article/id/1967
[5] 袁运斌.基于GPS的电离层监测及延迟改正理论与方法的研究[D].武汉: 中国科学院测量与地球物理研究所, 2002 Yuan Yunbin. Study on Theories and Methods of Correcting Ionospheric Delay and Monitoring Ionosphere Based on GPS[D]. Wuhan: Institute of Geodesy and Geophysics, Chinese Academy of Sciences, 2002
[6] 朱永兴, 谭述森, 任夏, 等. GNSS全球广播电离层模型精度分析[J].武汉大学学报·信息科学版, 2020, 45(5):768-775 doi: 10.13203/j.whugis20180439 Zhu Yongxing, Tan Shusen, Ren Xia, et al. Accuracy Analysis of GNSS Global Broadcast Ionospheric Model[J]. Geomatics and Information Science of Wuhan University, 2020, 45(5):768-775 doi: 10.13203/j.whugis20180439
[7] Feltens J, Dow J M, Martín-Mur T J, et al. Routine Production of Ionosphere TEC Maps at ESOC-First Results (IGS Presentation) [C]. The 1998 IGS AC Workshop, Darmstadt, Germany, 1998
[8] Mannucci A J, Wilson B D, Yuan D N, et al. A Global Mapping Technique for GPS-Derived Ionospheric Total Electron Content Measurements [J]. Radio Science, 1998, 33(31):565-582
[9] Hernández-Pajares M, Juan J M, Sanz J. New Approaches in Global Ionospheric Determination Using Ground GPS Data [J]. Journal of Atmospheric and Solar-Terrestrial Physics, 1999, 61(16):1 237-1 247 doi: 10.1016/S1364-6826(99)00054-1
[10] 章红平, 韩文慧, 黄玲, 等.地基GNSS全球电离层延迟建模[J].武汉大学学报·信息科学版, 2012, 37(10):1 186-1 189 http://ch.whu.edu.cn/article/id/355 Zhang Hongping, Han Wenhui, Huang Ling, et al. Modeling Global Ionospheric Delay with IGS Ground-Based GNSS Observations [J]. Geomatics and Information Science of Wuhan University, 2012, 37(10):1 186-1 189 http://ch.whu.edu.cn/article/id/355
[11] 陈鹏, 陈家君. GPS/GLONASS融合的全球电离层格网模型结果分析[J].大地测量与地球动力学, 2014, 34(5):70-74 https://www.cnki.com.cn/Article/CJFDTOTAL-DKXB201405015.htm Chen Peng, Chen Jiajun. Analysis of Global Ionospheric Grid Model Integrated GPS/GLONASS [J]. Journal of Geodesy and Geodynamics, 2014, 34(5):70-74 https://www.cnki.com.cn/Article/CJFDTOTAL-DKXB201405015.htm
[12] 党亚民, 王虎, 赵文娇, 等.融合BDS/GPS/GLONASS反演全球电离层特性研究[J].大地测量与地球动力学, 2015, 35(1):87-91 https://www.cnki.com.cn/Article/CJFDTOTAL-DKXB201501024.htm Dang Yamin, Wang Hu, Zhao Wenjiao, et al. Research of the Characteristics of Inversing Global Ionospheric with Fusing of BDS, GPS and GLONASS [J]. Journal of Geodesy and Geodynamics, 2015, 35(1):87-91 https://www.cnki.com.cn/Article/CJFDTOTAL-DKXB201501024.htm
[13] 张瑞.多模GNSS实时电离层精化建模及其应用研究[D].武汉: 武汉大学, 2013 Zhang Rui. Theory and Method on Multimode GNSS Real-Time Refinement Ionospheric Modeling and Its Application[D]. Wuhan: Wuhan University, 2013
[14] 熊波, 万卫星, 宁百齐, 等.基于北斗、GLONASS和GPS系统的中低纬电离层特性联合探测[J].地球物理学报, 2014, 57(11):3 586-3 599 https://www.cnki.com.cn/Article/CJFDTOTAL-DQWX201411013.htm Xiong Bo, Wan Weixing, Ning Baiqi, et al. Investigation of Mid- and Low-Latitude Ionosphere Based on BDS, GLONASS and GPS Observations[J]. Chinese Journal of Geophysics, 2014, 57(11):3 586-3 599 https://www.cnki.com.cn/Article/CJFDTOTAL-DQWX201411013.htm
[15] Ren X, Zhang X, Xie W, et al. Global Ionospheric Modelling Using Multi-GNSS: BeiDou, Galileo, GLONASS and GPS[J]. Scientific Reports, 2016, 6: 33 499 doi: 10.1038/srep33499
[16] 赵莹, 张小红. COSMIC掩星观测数据反演电离层电子密度廓线[J].武汉大学学报·信息科学版, 2010, 35(6): 644-648 http://ch.whu.edu.cn/article/id/980 Zhao Ying, Zhang Xiaohong. Inversion of Ionospheric Electron Density Profiles with COSMIC Occultation Data[J]. Geomatics and Information Science of Wuhan University, 2010, 35(6):644-648 http://ch.whu.edu.cn/article/id/980
[17] Alizadeh M M, Schuh H, Todorova S, et al. Global Ionosphere Maps of VTEC from GNSS, Satellite Altimetry, and Formosat-3/COSMIC Data[J]. Journal of Geodesy, 2011, 85(12): 975-987 doi: 10.1007/s00190-011-0449-z
[18] Chen P, Yao W, Zhu X. Combination of Ground- and Space-Based Data to Establish a Global Ionospheric Grid Model[J]. IEEE Transactions on Geoscience and Remote Sensing, 2015, 53(2): 1 073-1 081 doi: 10.1109/TGRS.2014.2333522
[19] de Selding P B. SpaceX to Build 4 000 Broadband Satellites in Seattle [OL]. http://spacenews.com/spacex-opening-seattle-plant-to-build-4000-broadband-satellites/, 2015
[20] de Selding P B. Virgin, Qualcomm Invest in OneWeb Satellite Internet Venture [OL]. http://spacenews.com/virgin-qualcomm-invest-in-global-satellite-internet-plan/, 2015
[21] Bilitza D, Altadill D, Truhlik V, et al. International Reference Ionosphere 2016: From Ionospheric Climate to Real-Time Weather Predictions[J]. Space Weather, 2017, 15(2): 418-429 doi: 10.1002/2016SW001593
下载:
计量
- 文章访问数: 1512
- HTML全文浏览量: 390
- PDF下载量: 171
