Application in Accuracy Assessment for DEM Interpolation Methods Based on a Variance-scale Law
-
摘要: 提出基于方差-尺度规律的新型插值方法评价体系,根据“在给定研究区域内,变量的统计方差随尺度增大而减小”的理论规律,提出三项预设的评价准则。以DEM插值应用为例,选取高精度曲面模型、样条插值、克里金插值、反距离权重插值4种方法进行对比评价。数值实验表明,方差-尺度递变趋势的评价准则值得存疑。两组DEM插值案例表明,地形的复杂特性、插值算子的光滑效应以及尺度的综合效应致使理论的渐变规律无法达到。引入采样方差值后,不同采样密度下仍可以将方差变化趋势及方差的整体大小作为精度评价准则,在对第二、第三条预设评价准则修缮后确立三项评价准则。综合所有案例可知,此评价体系原理简单、操作方便,为DEM插值方法评价提供了一套新的标准和框架,并且可针对不同采样密度条件提出完善的插值方法适用性建议。Abstract: A new accuracy assessment framework for interpolations, based on a variance-scale law, is proposed in this paper. Based findings in different research areas, it has been shown that the variance of a certain variable decreases with agrowing scale. According to this theoretical law, three presupposed assessment criteria have been proposed. Application cases in DEM interpolation were selected and four common methods, i.e., HASM, Spline, Kriging, and IDW were chosen for comparison in this new framework. The second assessment criterion, regarding changing trends, was found to be questionable in a numerical test. The results of two real-world DEM interpolation cases indicate that the theoretical changing trend in the variance-scale relation cannot be obtained in real terrain cases because of an complicated integrated effects, characteristic of the real-world terrain, and the smoothing effect of interpolators and interpolation scales. However, as the original sampling variance was introduced into the framework, the general changing trend of variances and the overall level of variances at most scales could still be used as accuracy assessment measures even under different sampling densities. After modifying the second and the third criterion, the final three criteria in this assessment framework were established. Both the numerical surface case and real-world DEM examples indicate that this assessment framework is simple in theory, convenient for usie, and is an objective and effective assessment method, widening the field of accuracy assessment in DEM interpolation. Moreover, based on the results of different sampling densities, this framework can also provide valuable application suggestions for choosing suitable interpolation methods in real-world cases.
-
Keywords:
- interpolation methods /
- DEM /
- accuracy assessment /
- variance /
- scale /
- HASM
-
以总体最小二乘作为误差变量(errors-in-variables,EIV)模型的严密估计方法在大地测量领域已经得到了深入的研究[1-6]。随着空间数据的种类越来越多样化,如何有效地确定相对权比来统一各类数据,从而自适应调整各类观测数据对模型参数估值的贡献,成为解决联合平差问题的关键。文献[7]给出了大地测量、地震和地质三类数据的联合反演模式和水准测量、GPS和重力三种数据联合反演断层参数的具体公式,介绍了求解优化问题所有全局最优解的区间算法;文献[8]通过大地测量数据与地震数据的联合反演,提出了联合反演中确定相对权比的方法;文献[9-13]采用分组平差(序贯平差)方法,将观测向量分为多组,降低了矩阵求逆的阶数,但分组平差过程中,未对各组数据附加相对权比;文献[14]提出了一种确定联合反演中相对权比的两步法,通过赫尔默特方差分量估计对不同种类数据进行归一化,再建立顾及相对权比的联合反演模型求解;文献[15]基于赫尔默特方差分量估计公式提出了联合反演的序贯算法;面对参数间存在约束条件的问题,文献[16]提出了附加约束条件的序贯平差;文献[17]研究了等式约束反演和联合反演的反演模型及目标函数的构建方法。但上述联合平差方法均基于最小二乘原理展开,未能顾及到模型系数矩阵的误差,且实际应用中,系数矩阵往往是由含有误差的观测元素组成的。文献[18-20]从方差分量估计的角度出发,分别给出了不同的总体最小二乘方差分量估计方法,直接求解不同类数据的单位权方差,然而,当数据种类增多时其方差分量往往存在不可估性问题。
基于此,本文在各类数据各自等精度的条件下,提出了一种将总体最小二权方法用于联合平差的新方法。
1 总体最小二乘联合平差方法
设有两种不同类的观测数据,则总体最小二乘联合平差问题建立的函数模型为:

(1) 式中,L1∈Rn1×1为第Ⅰ类观测向量;e1为第Ⅰ类观测向量的随机误差;L2∈Rn2×1为第Ⅱ类观测向量;e2为第Ⅱ类观测向量的随机误差;B1∈Rn1×m为第Ⅰ类观测方程列满秩系数矩阵;EB1为系数矩阵B1的误差;B2∈Rn2×m为第Ⅱ类观测方程列满秩系数矩阵;EB2为系数矩阵B2的误差;X∈Rm×1为m×1待估参数向量。
联合平差的随机模型为:

(2) 式中,eB1和eB2分别是将矩阵EB1和EB2按列拉直得到的列向量;σ012和σ022分别为第Ⅰ类和第Ⅱ类观测值及系数矩阵元素的验前单位权方差;Im、In1、In2分别为m阶、n1阶和n2阶单位阵;vec(·)表示矩阵拉直运算;ⓧ表示Kronecker积。
将式(1) 改造,可得:

(3) 式中,

(4) 根据式(3) ,由协因数传播定律容易得到e1和e2的协因数阵[6]:

(5) 
(6) 令e1的平差值为V1,e2的平差值为V2,X的平差值为${\hat{X}}$ ,则式(3) 变为:

(7) 则总体最小二乘联合平差准则为:

(8) 式中,



(9) 由式(9) ,根据求函数自由极值的方法,得:

(10) 将式(7) 代入式(10) 得:

(11) 即

(12) 令


则有法方程:

(13) 解法方程,得:

(14) 式(14) 通过多次迭代可以得到较准确的参数解。
同理,将两类观测数据扩展到k类观测数据的一般情况,可得到k类数据条件下,总体最小二乘联合平差的参数估值:

(15) 式中,


2 相对权比τ的确定方法
在实际应用中,由于各类型的数据之间具有不同的单位权方差,且联合平差前各类数据元素定权不准确,为使总体最小二乘联合平差法合理,应考虑不同类数据的相对权比,本节以两种方法为例说明。
2.1 验前单位权方差法[6, 8, 14]
由函数模型(1) 和随机模型(2) 可知,两类数据的验前单位权方差分别为σ012和σ022,则总体最小二乘联合平差中相对权比τ为:

(16) 由迭代解算得到参数估值,计算步骤为:

(17) 
(18) 
(19) 当

(20) 时,计算结束。式中‖·‖表示向量的2范数。
可知,当σ022=σ012≠0时,τ=0.5,此时为不考虑相对权比的联合平差问题,也即视第Ⅰ类数据和第Ⅱ类数据在联合平差中所占的权重等同。
2.2 判别函数最小化法[6, 14]
选定一个步长,让权比例因子τ遍历整个取值区间,将每个τ的取值代入式(17) ~(20) 中计算,可得到对应联合平差的参数估值${\hat{X}}$,由式(7) 得到相应的V1和V2,可得判别函数的估值为:

(21) 把式(21) 中的最小值对应的相对权比τ和此时相对权比下的参数估值作为最终结果。其他常用的函数将在§3中具体讨论。
3 算例与分析
3.1 线性方程组算例
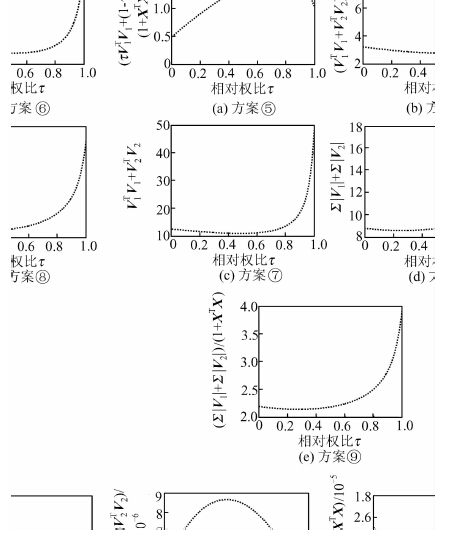
模拟一个线性参数估计问题,满足BtruetrueXtrue=Ltrue,其中Btrue、Ltrue为设计矩阵和观测值的真值,具体见表 1。模型参数的真值Xtrue=[111]T。现对前5组观测值向量和设计矩阵真值加入e1~N(0,σ2I)的噪声,其中σ012=0.5,作为第Ⅰ类平差数据;对后7组观测值向量和设计矩阵元素加入e2~N(0,σ022I)的噪声,其中σ022=0.1,作为第Ⅱ类平差数据;它们是分别采用① τ=1的总体最小二乘法; ② τ=0的总体最小二乘法; ③ τ=0.5的总体最小二乘联合平差法; ④ 验前单位权方差法,由(1-τ)/τ=σ012/σ022确定τ值; ⑤ =[τV1TV1+(1-τ)V2TV2]/(1+T)的判别函数最小化法; ⑥ =(V1TV1+V2TV2)/(1+T)的判别函数最小化法; ⑦ =V1TV1+V2TV2的判别函数最小化法; ⑧


 表 1 线性方程组观测向量和设计矩阵的真值Table 1. True Values of Observation Vectors and Design Matrice
表 1 线性方程组观测向量和设计矩阵的真值Table 1. True Values of Observation Vectors and Design Matrice数据类型 Btrue Ltrue 2.0 -5.2 3.1 -0.1 3.6 4.5 2.9 5.2 I -1.5 6.8 3.4 8.7 4.0 7.3 -9.1 2.2 6.71 -3.3 -4.5 -1.09 10.4 12.7 -5.8 17.3 -5.5 -7.6 -2.1 -15.2 8.2 4.5 -9.0 3.7 II -4.2 -2.3 8.6 2.1 -2.3 11.5 4.0 13.2 7.4 3.6 -4.2 6.8 9.1 -7.1 2.0 4.0 对这9种方案模拟100次。在方案①~④中,由于相对权比事先给定,计算该相对权比下对应各方案的参数估值和差值范数,最后对100次计算结果取平均值;在方案⑤~⑨的判别函数最小化法计算时,由于τ是按一定的步长(本文中步长取0.001) 遍历整个取值区间的(τ∈(0,1) ,因联合反演故τ不取0和1[15]),为保证对应某一相对权比下取值的一致性,每模拟一次数据,便计算每个τ对应的参数估值和差值范数,在完成100次运算后,取各相对权比下计算结果的均值,并把均值结果中判别函数值取最小时对应的相对权比及在此相对权比下计算结果的均值作为最终值,参数平差结果见表 2。本文还绘制了方案⑤~⑨的判别函数与相对权比之间的关系,如图 1所示。
表 2 参数平差结果Table 2. Results of Inversion Parameters方案 ${\hat{X}}$1 ${\hat{X}}$2 ${\hat{X}}$3 τ ‖Δ${\hat{X}}$‖ 真值 1.0000 1.0000 1.000 0 / / ① 1.037 0 1.023 4 1.044 3 1.000 0.380 7 ② 0.997 2 1.004 8 1.002 1 0.000 0.074 6 ③ 0.997 7 1.006 1 1.006 2 0.500 0.089 8 ④ 0.997 3 1.005 1 1.003 1 0.167 0.071 4 ⑤ 0.997 2 1.004 8 1.002 2 0.001 0.074 6 ⑥ 0.997 7 1.006 1 1.006 2 0.500 0.089 8 ⑦ 0.997 7 1.006 1 1.006 0 0.490 0.088 6 ⑧ 0.997 4 1.005 4 1.003 8 0.270 0.072 7 ⑨ 0.997 4 1.005 4 1.003 9 0.286 0.073 2 注:验前单位权方差法确定方案④的相对权比,(1-τ)/τ=0.5/0.1,即τ=0.167。‖Δ‖表示模型参数平差结果与真值差值的范数;τ为各方法计算的相对权比。 由表 2可知,由于数据量相对较少,数据精度相对较低,因此第I类数据的总体最小二乘平差结果最差(方案①);方案③对各类数据附加了相同的相对权比(τ=0.5) ,即视联合平差中各类数据的权重相等,因而结果相对较差;比较方案⑤与方案②,不难发现,若不加0<τ<1的限制,方案⑤与方案②等价,图 1(a)中散点图的最小值在最左端处取得,可见方案⑤的结果并没有起到权衡各类数据的作用;方案⑤与方案⑦也存在同样的问题,其判别函数求得的权比值为0.5和0.49,与实际值0.167相差较大,因而其解算结果较第II类数据的总体最小二乘平差结果要差(方案②),此时联合平差结果不如单一数据的平差结果;而方案⑧与方案⑨的结果则优于方案②,可见判别函数的选取对平差结果的影响很大。
3.2 坐标位移反演应变参数
在二维平面情况下,利用应变参数反演模型模拟生成反演应变参数的GPS坐标位移数据和边角网平差数据,其真值见表 3和表 4;坐标位移反演应变参数的公式为[21]:
序号 X/km Y/km u1/mm v1/mm 1 100.000 100.000 1.000 20.200 2 100.000 300.000 -1.000 18.600 3 100.000 500.000 -3.000 17.000 4 300.000 100.000 5.000 62.200 5 300.000 300.000 3.000 60.600 6 300.000 500.000 1.000 59.000 7 500.000 100.000 9.000 104.200 8 500.000 300.000 7.000 102.600 9 500.000 500.000 5.000 101.000 
(22) 式中,(xi,yi)为第i点近似坐标;ui为第i点纵坐标位移量;vi为第i点横坐标位移量;εx、εy分别为x、y轴方向上的线应变;γxy=2εxy为x、y方向上的剪应变;ω为转动惯量。
对GPS坐标数据及坐标位移真值加入e1~N(0,σ012I)的随机噪声,其中σ012=0.5 mm022;对边角网的坐标数据及位移真值加入e2~N(0,σ2I)的随机噪声,其中σ022=2.0 mm2,得到两类数据的模拟观测值。 采用表 2中的9种方案,模拟100次,按照§3.1中算例方法获得应变参数反演结果,见表 5。应变参数反演方案⑤~⑨的判别函数与相对权比之间的关系如图 2所示。
应变参数反演的算例中,方案⑥与方案⑦等价,且两方案所确定的相对权比与方案③相同,均为0.5,均未能合理反映每类数据在联合平差中的比重;而方案⑤确定的相对权比0.999(非常接近于1) ,其结果与方案①近似。可见在方案⑤下,联合平差退化为单一数据的总体最小二乘平差,这就意味着失去了联合平差的意义,显然不合理。
表 5 应变参数反演结果Table 5. Inversion Results of Model Parameters方案 ${\hat{\varepsilon }}$x/10-9 ${\hat{\varepsilon }}$ y/10-9 ${\hat{\varepsilon }}$ xy/10-9 ${\hat{\omega }}$/10-9 τ ‖Δ${\hat{X}}$‖ 真值 20.000 0 -8.000 0 100.000 0 110.000 0 / / 1 19.987 3 -7.808 4 99.954 9 109.932 7 1.000 1.691 5 2 20.270 4 -8.143 8 99.967 0 110.129 6 0.000 3.217 7 3 20.126 2 -7.972 4 99.961 8 110.039 4 0.500 1.845 7 4 20.042 6 -7.873 6 99.957 8 109.977 0 0.800 1.511 0 5 19.987 6 -7.808 8 99.954 9 109.932 9 0.999 1.689 9 6 20.126 2 -7.972 4 99.961 8 110.039 4 0.500 1.845 7 7 20.126 2 -7.972 4 99.961 8 110.039 4 0.500 1.845 7 8 20.092 4 -7.932 4 99.960 3 110.014 9 0.621 1.643 8 9 20.092 4 -7.932 4 99.960 3 110.014 9 0.621 1.6438 注:验前单位权方差法确定方案④的相对权比,(1-τ)/τ=0.5/2.0,即τ=0.8。 综合§3.1与§3.2中算例可知,两算例中验前单位权方差法(方案④)的差值范数最小,这与理论相符,这是因为给定的相对权比为实际权比;然而,在实际数据处理过程中,当验前随机模型不准确时,观测数据对参数解贡献的比重将不再合理,所以验前单位权方差法并不具备操作性;相比较而言,方案⑧与方案⑨的平差结果是判别函数最小化中最好的,从图 1(c)、1(d)和图 2(c)、2(d)中不难发现,其取得最小值的点相对接近各自的实际权比值,因此,在先验信息不准确时,采用以方案⑧、方案⑨为判别函数的判别函数最小化法可以取得更为有效的结果。
为了进一步探究加入的随机噪声对确定相对权比的影响,对§3.1和§3.2中算例模拟加入不同大小的随机噪声做模拟计算,结果见表 6、表 7。
表 6 §3.1中算例不同精度对确定相对权比的影响Table 6. Influence to theWeight Scaling Factor Determined by Adding Different Noise to Example in §3.1σ012 σ022 相对权比τ 方案⑤ 方案⑥ 方案⑦ 方案⑧ 方案⑨ 0.5 0.000 5 0.001 0.500 0.494 0.260 0.270 0.5 0.001 0.001 0.500 0.483 0.350 0.380 0.5 0.005 0.001 0.500 0.497 0.720 0.720 0.5 0.01 0.001 0.500 0.497 0.131 0.131 0.5 0.05 0.001 0.500 0.486 0.169 0.183 0.5 0.1 0.001 0.500 0.490 0.270 0.286 0.1 0.1 0.999 0.500 0.499 0.441 0.441 0.05 0.1 0.999 0.500 0.502 0.480 0.473 0.01 0.1 0.999 0.500 0.500 0.685 0.688 0.005 0.1 0.999 0.500 0.500 0.746 0.749 0.0005 0.1 0.999 0.500 0.500 0.869 0.873 σ012、σ022分别代表第I、II类数据的单位权方差。 表 7 §3.2中算例不同精度对确定相对权比的影响Table 7. Influence to the Weight Scaling Factor Determined by Adding Different Noise to Example in §3.2σ012 σ022 相对权比τ 方案⑤ 方案⑥ 方案⑦ 方案⑧ 方案⑨ 0.000 5 2.0 0.999 0.500 0.500 0.984 0.984 0.005 2.0 0.999 0.500 0.500 0.953 0.953 0.01 2.0 0.999 0.500 0.500 0.926 0.926 0.05 2.0 0.999 0.500 0.500 0.863 0.863 0.1 2.0 0.999 0.500 0.500 0.826 0.826 0.5 2.0 0.999 0.500 0.500 0.676 0.676 0.5 0.5 0.001 0.500 0.500 0.472 0.472 0.5 0.1 0.001 0.500 0.500 0.280 0.280 0.5 0.01 0.001 0.500 0.500 0.102 0.102 0.5 0.001 0.001 0.500 0.500 0.043 0.043 0.5 0.000 1 0.001 0.500 0.500 0.016 0.016 σ012、σ022分别代表GPS数据和边角网数据的单位权方差。 不难发现,对于方案⑤,随着各类数据加入的误差不同,其判别函数永远在两端处取到最小值。可见在不同的观测数据精度下,判别函数Φ=[τV1TV1+(1-τ)V2TV2]/(1+T)所确定的相对权比本身不能很好地拟合各类数据,因而其相对权比的取值与其他各方案的差别较大;而方案⑥、方案⑦的相对权比保持在0.5及附近,并没有起到权衡各类数据的作用;随着各类数据加入的误差不同,两算例中方案⑧与方案⑨所确定的相对权比相差不大,且比较靠近实际的相对权比值,结果更能反映实际情况,可知联合平差中权比例关系在求解模型参数中起着很重要的作用;但并不能以相对权比的大小来评价方案的好坏[22]。
4 结 语
本文将总体最小二乘方法应用于联合平差问题,推导了附有相对权比的总体最小二乘联合平差方法,并推广得到了多类数据情况下的一般平差计算公式。相对权比的确定是联合平差中求 解参数的关键,本文在两类数据各自等精度的前提下,分析了多种判别函数确定相对权比的方法,并对比了各自权比下的解算结果。然而本文在模拟算例中假设两类数据各自是等精度的,对于不等精度情况下的总体最小二乘联合平差还需要进一步研究,同时对于相对权比的确定方法也需要通过实际应用进一步验证和改进。
-
表 1 递进式抽样中的各项参数
Table 1 Parameters of Four Progressive Sampling Processes
抽样次数 样本数 抽样比例/% 抽样数 占总样本数比例/% 对应采样间隔/m 1 1 480 80 1 184 80 17 2 1 184 75 888 60 20 3 888 67 594 40 25 4 594 50 297 20 30 -
[1] 汤国安,刘学军,闾国年. 数字高程模型及地学分析的原理与方法[M]. 北京:科学出版社, 2005 Tang Guoan, Liu Xuejun, Lv Guonian. Theory and Methods of Digital Elevation Model and Geoanalysis[M]. Beijing:Science Press, 2005
[2] 黄健. 数字高程模型的质量检查[J]. 测绘通报, 2002, 2:55-56 http://www.cnki.com.cn/Article/CJFDTOTAL-CHTB200202021.htm Huang Jian. Checking on the Quality of DEM[J]. Bulletin of Surveying and Mapping, 2002,2:55-56 http://www.cnki.com.cn/Article/CJFDTOTAL-CHTB200202021.htm
[3] 王光霞, 崔凯, 戴军. 基于分形的DEM精度评估[J]. 测绘学院学报, 2005,22(2):107-109 http://www.cnki.com.cn/Article/CJFDTOTAL-JFJC200502011.htm Wang Guangxia, Cui Kai, Dai Jun. The Accuracy Evaluation of DEM Based on Fractal Analysis[J]. Journal of Institute of Surveying and Mapping,2005,22(2):107-109 http://www.cnki.com.cn/Article/CJFDTOTAL-JFJC200502011.htm
[4] 周兴华, 姚艺强, 赵吉先. DEM内插方法与精度评定[J]. 测绘科学, 2005, 30(5):86-88 http://www.cnki.com.cn/Article/CJFDTOTAL-CHKD200505030.htm Zhou Xinghua, Yao Yiqiang, Zhao Jixian. Research on Interpolation and Accuracy Assessment of DEM[J]. Science of Survey and Mapping,2005,30(5):86-88 http://www.cnki.com.cn/Article/CJFDTOTAL-CHKD200505030.htm
[5] 李志林, 朱庆. 数字高程模型[M]. 武汉:武汉大学出版社, 2000 Li Zhilin, Zhu Qing. Digital Elevation Model[M]. Wuhan:Wuhan University Press, 2000
[6] 胡鹏, 杨传勇, 吴艳兰, 等. 新数字高程模型:理论、方法、标准和应用[M].北京:测绘出版社, 2007 Hu Peng, Yang Chuanyong, Wu Yanlan. New DEM Theories, Methods, Standards and Application[M]. Beijing:Surveying and Mapping Press, 2007
[7] Wise S. Assessing the Quality for Hydrological Applications of Digital Elevation Models Derived from Contours[J]. Hydrological Processes, 2000, 14(11-12):1909-1929 doi: 10.1002/(ISSN)1099-1085
[8] Li Z L. Theoretical Models of the Accuracy of Digital Terrain Models:An Evaluation and Some Observations[J]. The Photogrammetric Record, 1993, 14(82):651-660 doi: 10.1111/phor.1993.14.issue-82
[9] Huang Y D. Evaluation of Information Loss in Digital Elevation Models with Digital Photogrammetric Systems[J]. The Photogrammetric Record, 2000, 16(95):781-791 doi: 10.1111/phor.2000.16.issue-95
[10] Aguilar F J, Aguilar M A, Aguera F, et al. The Accuracy of Grid Digital Elevation Models Linearly Constructed from Scattered Sample Data[J]. International Journal of Geographical Information Science, 2006, 20(2):169-192 doi: 10.1080/13658810500399670
[11] Shi W, Li Q, Zhu C. Estimating the Propagation Error of DEM from Higher-order Interpolation Algorithms[J]. International Journal of Remote Sensing, 2005, 26(14):3069-3084 doi: 10.1080/01431160500057905
[12] Hu P, Liu X H, Hu H. Accuracy Assessment of Digital Elevation Models Based on Approximation Theory[J]. Photogrammetric Engineering and Remote Sensing, 2009, 75(1):49-56 doi: 10.14358/PERS.75.1.49
[13] Journel A G, Huijbregts C J. Mining Geostatistics[M]. London:Academic Press, 1978
[14] Wiens J A. Spatial Scaling in Ecology[J]. Functional Ecology, 1989, 3(4):385-397 doi: 10.2307/2389612
[15] Marceau D J, Howarth P J, Gratton D J. Remote Sensing and the Measurement of Geographical Entities in a Forested Environment. 1. The Scale and Spatial Aggregation Problem[J]. Remote Sensing of Environment, 1994, 49(2):93-104 doi: 10.1016/0034-4257(94)90046-9
[16] Smagorinsky J. Global Atmospheric Modeling and the Numerical Simulation of Climate[M]. New York:John Wiley & Sons,1974:633-686
[17] Kirnbauer R, Bl schl G, Gutknecht D. Entering the Era of Distributed Snow Models[J]. Nordic Hydrology, 1994, 25(1):1-24 http://cn.bing.com/academic/profile?id=1996591456&encoded=0&v=paper_preview&mkt=zh-cn
[18] Bl schl G, Sivapalan M. Scale Issues in Hydrological Modelling-a Review[J]. Hydrological Processes, 1995, 9(3-4):251-290 doi: 10.1002/(ISSN)1099-1085
[19] Bl schl G. Statistical Upscaling and Downscaling in Hydrology[M]. London:John Wiley & Sons, 2006
[20] 贾旖旎, 汤国安, 刘学军. 高程内插方法对DEM所提取坡度、坡向精度的影响[J]. 地球信息科学学报, 2009, 11(1):36-42 http://www.cnki.com.cn/Article/CJFDTOTAL-DQXX200901009.htm Jia Yini, Tang Guoan, Liu Xuejun. The Impact of Elevation Interpolation on the Accuracy of Gradient and Aspect from DEMs[J].Journal of Geo-information Science,2009,11(1):36-42 http://www.cnki.com.cn/Article/CJFDTOTAL-DQXX200901009.htm
[21] 陈吉龙, 武伟, 刘洪斌. DEM内插算法对坡度坡向的影响[J]. 水土保持研究, 2008, 15(6):14-17 http://www.cnki.com.cn/Article/CJFDTOTAL-STBY200806004.htm Chen Jilong, Wu Wei, Liu Hongbin. Effect of DEM Interpolation on the Slope and Aspect[J].Research of Soil and Water Conservation,2008,15(6):14-17 http://www.cnki.com.cn/Article/CJFDTOTAL-STBY200806004.htm
[22] Myers D E. Statistical Models for Multiple-scaled Analysis[M] Boca Raton, FL:Lewis Publishers, 1997:273-293





 下载:
下载: