Hexagonal Grid Mean Free-Air Gravity Anomaly Data Construction and Its Statistical Advantage Analysis
-
摘要:
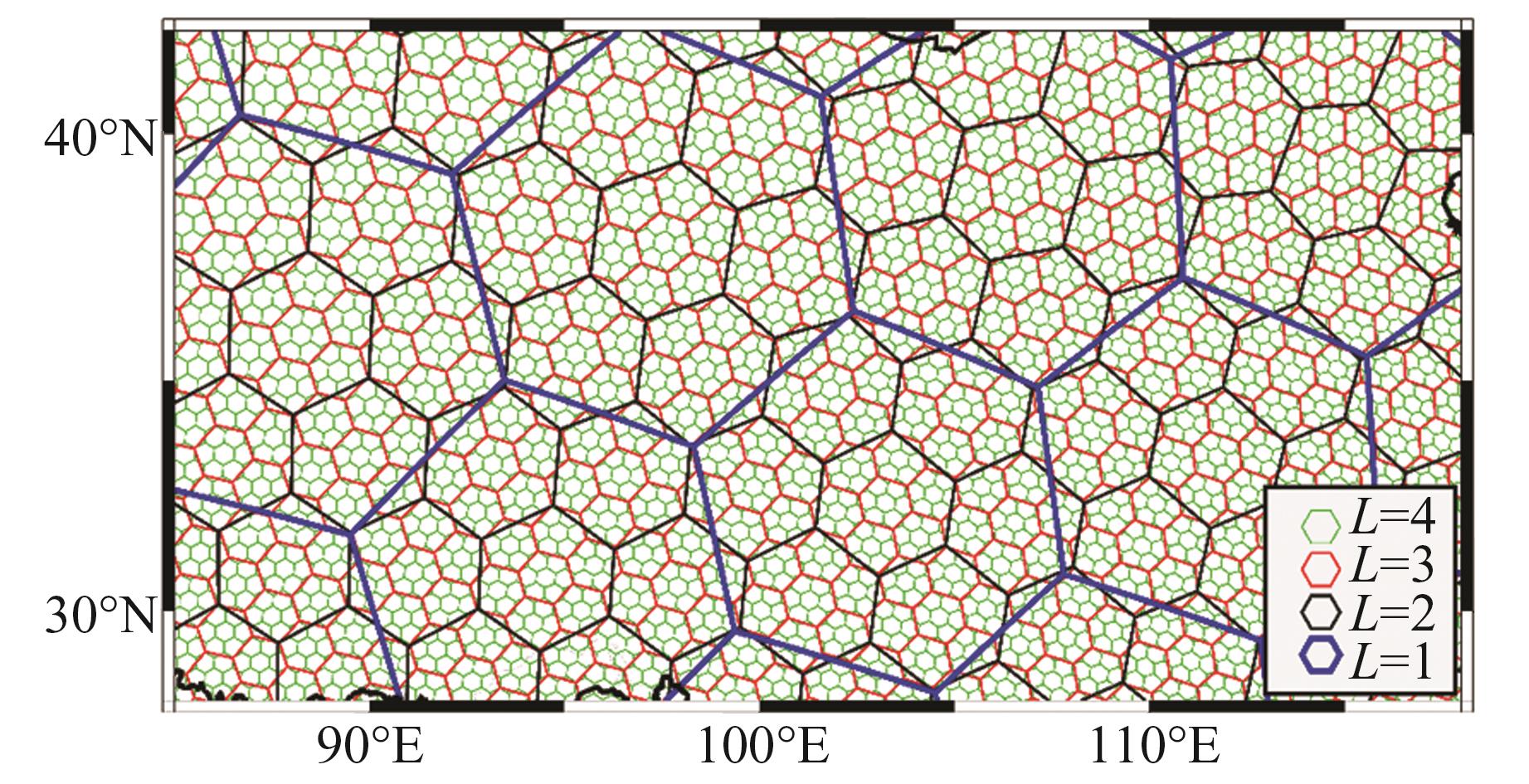
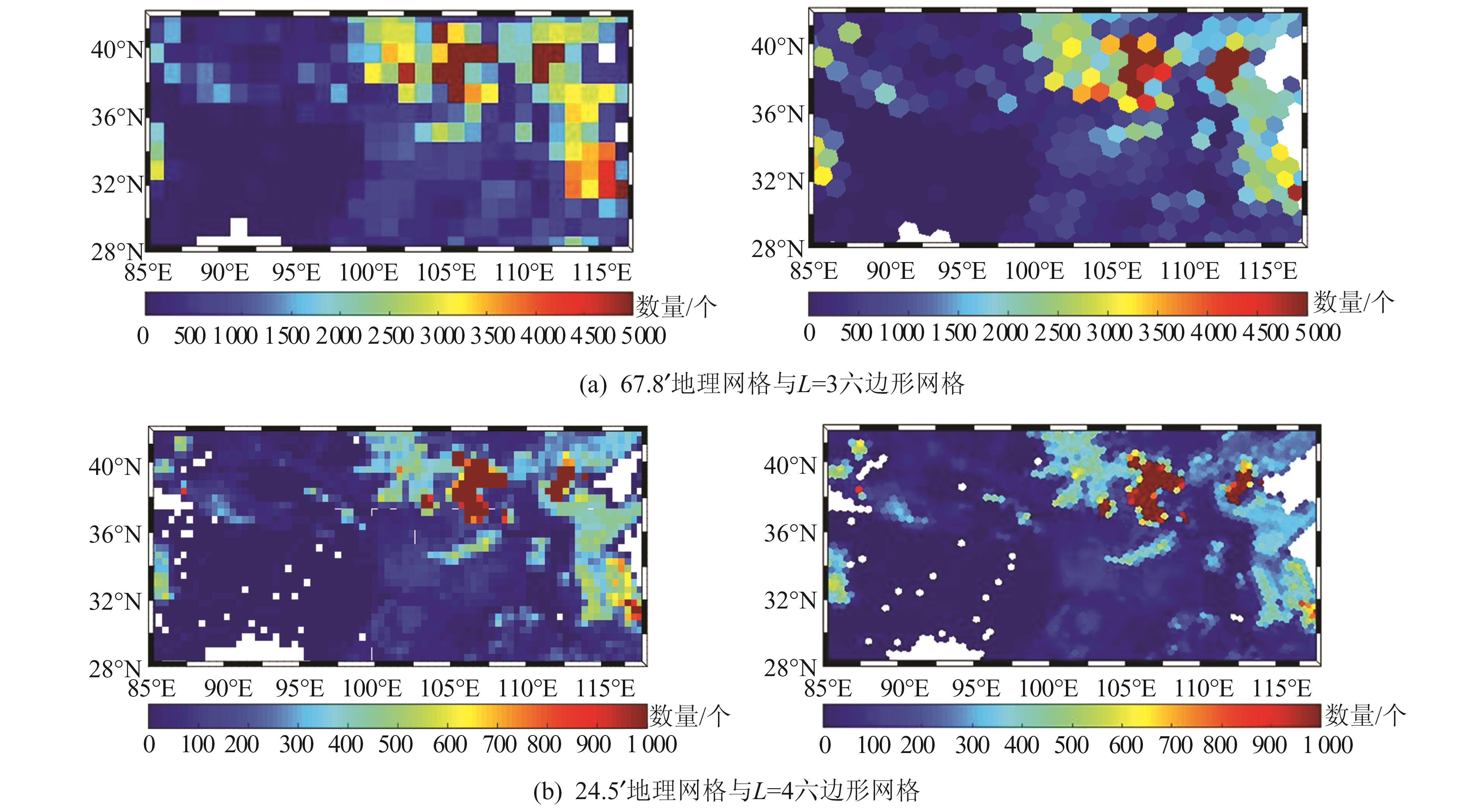
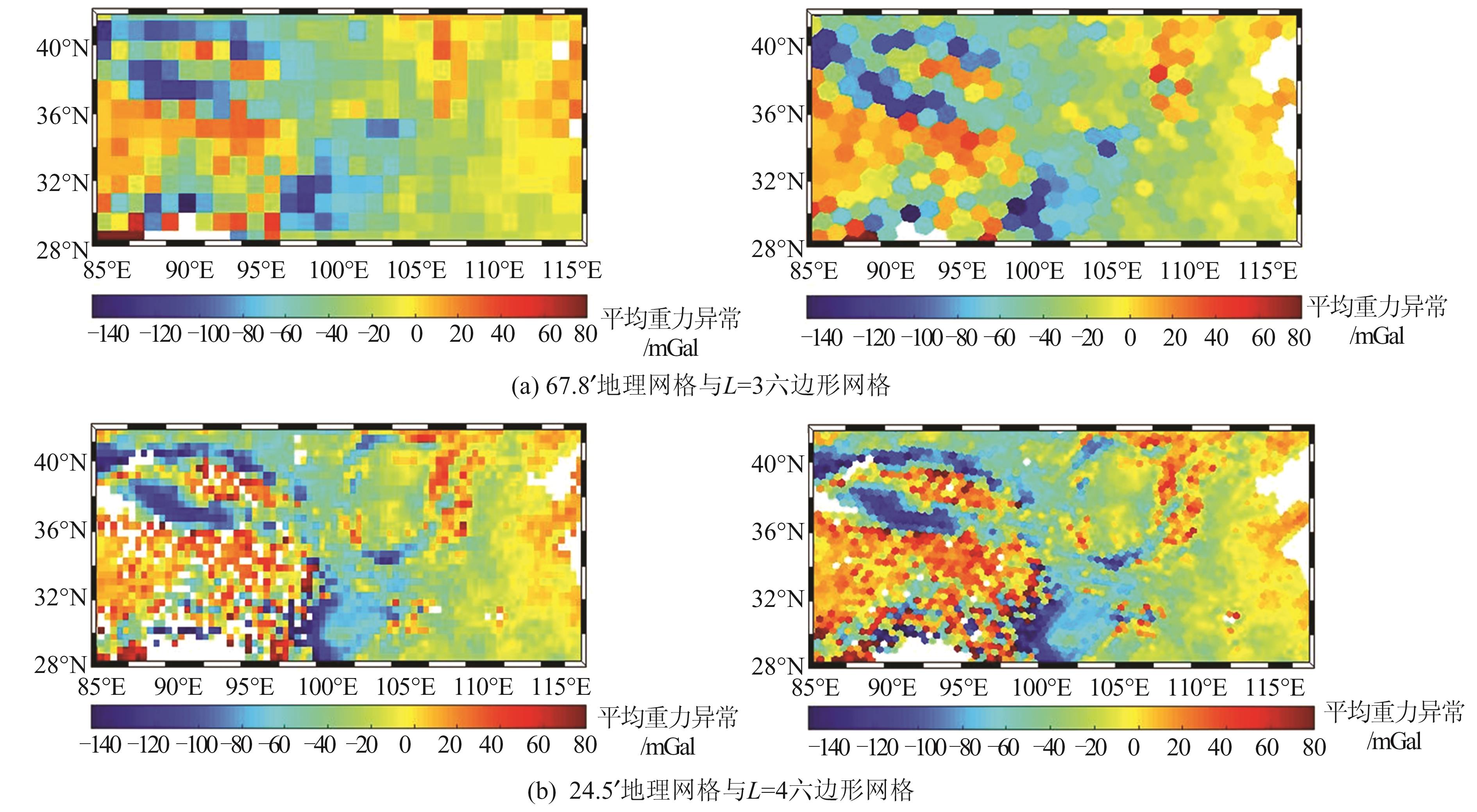
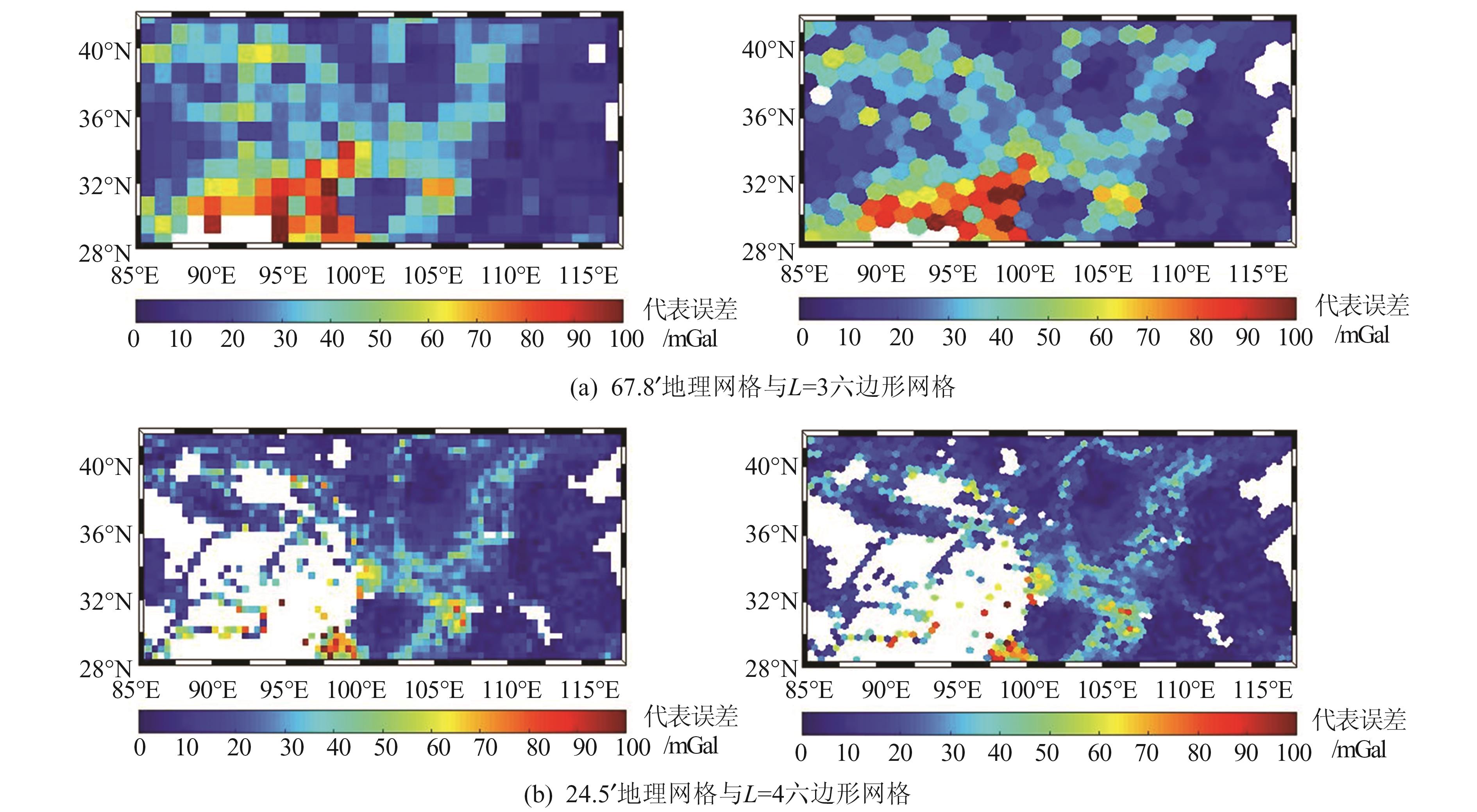
传统地理网格在重力场数据处理和解算中被广泛使用,但也带来诸多不便,例如网格面积不等、各向同性差等。针对地球重力场中地理网格的使用所带来的局限性,首次提出采用具有分层结构的六边形网格系统对重力测量数据分布区域进行划分,基于开源的H3离散球面网格系统构建中国大陆陆地重力测量数据区域3种分辨率的六边形网格,并利用中国81万余条实测重力资料,构建了上述3种分辨率下的六边形网格平均重力异常数值模型,以及平均大小与之对应的地理网格平均重力异常数值模型,最后统计对比了地理网格和六边形网格两种剖分方式下网格平均空间重力异常值的代表误差大小。结果表明,剖分层级L=3、4、5的六边形网格与面积近似相等的67.8′、24.5′、9.2′的四边形地理网格相比,网格内包含实测点的网格数量占总网格数量比更高,包含实测点的网格占比分别提高1.54%、1.44%和2.81%；平均网格重力异常代表误差分别减小0.398 mGal、0.259 mGal和0.188 mGal。综上所述,分层六边形网格系统因其近似等积和各项同性特征,在地球重力场数据统计和数据生产中具有应用优势。尽管层次六边形网格系统在地球重力场的球谐合成与分析、地形效应的快速计算、数值积分计算等方面也有良好的应用前景,但不可否认的是,它的使用和推广仍面临许多需要解决的难题,例如六边形网格的非等纬度分布引起的Legendre计算问题,以及如何使用快速傅里叶变换技术在该不规律分布下实现高效计算。
Abstract:ObjectivesThe widespread use of traditional geographic grids in gravity field data processing has brought many inconveniences, such as unequal grid areas and poor isotropic characteristics. In order to solve the above problems in the application of geographic grid in the earth gravity field, we propose for the first time to apply a hierarchical hexagonal grid system to gravity data gridding in the mainland of China.
MethodsFirst, an open-source discrete global grid system H3 is selected to generate hexagonal grid groups at three resolutions in the mainland of China. Then we use more than 810 000 in-situ gravity data to construct numerical models of average gravity anomaly on hexagonal grid with different resolutions and geographic grid with corresponding resolutions. Finally, we calculated and compared the commission errors of the grid average free-air gravity anomaly under the geographic grid and hexagonal grid.
ResultsThe results show that compared with 67.8′, 24.5′ and 9.2′ geographic grids, the hexagonal grids with grid partitioning levels L=3, 4, and 5 have approximately the same area. Hexagonal grids have more effective grids covering measured points than geographic grids. For example, the improvements of the percentages of effective grids in total in hexagonal grids under three resolution levels are 1.54%, 1.44% and 2.81%, respectively, compared to the geographic grids. Hexagonal grids have smaller commission error than geographic grids. For example, under the above three resolution levels, the mean commission errors of the hexagonal grid gravity anomalies are reduced by 0.398 mGal, 0.259 mGal and 0.188 mGal, respectively, compared to that of the geographic grid gravity anomalies.
ConclusionsThe layered hexagonal grid system has advantages in the application of earth gravity field data statistics and data production due to its quasi-homogeneous and quasi-isotropic horizontal resolution over the entire sphere. Although the layered hexagonal grid system also has good application prospects in spherical harmonic synthesis and analysis of the earth's gravity field, rapid calculation of terrain effects, numerical integration calculation, etc., it is undeniable that its use and promotion still faces many problems that need to be solved, such as the calculation problems caused by the non-equal latitude distribution of the hexagonal grids, and how to use fast fourier transformation technology to achieve efficient calculation under this uneven distribution.
-
http://ch.whu.edu.cn/cn/article/doi/10.13203/j.whugis20220569
-
![]()
图 3 中国部分区域六边形网格层次剖分结构示意图
Figure 3. Schematic Diagram of the Hierarchical Structure of Hexagonal Grid in Parts of China
![]()
图 4 网格内实测点数量统计情况
Figure 4. Distribution of Number of Grids Containing Measurement Points
![]()
图 6 网格平均重力异常的代表误差大小及分布
Figure 6. Magnitude and Distribution of Commission Errors of Grid-Averaged Gravity Anomalies
表 1 六边形网格的参数统计表
Table 1 Statistics of Hexagonal Grid Parameters
L NL 平均面积/km2 平均边长/km 0 122 4 250 546.847 700 0 1 107.712 591 000 1 842 607 220.978 242 9 418.676 005 500 2 5 882 86 745.854 034 7 158.244 655 800 3 41 162 12 392.264 862 1 59.810 857 940 4 288 122 1 770.323 551 7 22.606 379 400 5 2 016 842 252.903 364 5 8.544 408 276 6 14 117 882 36.129 052 1 3.229 482 772 
下载: 导出CSV
表 2 中国区域内两种网格剖分情况对比
Table 2 Comparison of Meshing of Two Shapes in China
六边形网格 四边形地理网格 L 网格数 分辨率/(′) 网格数 3 820 67.8 842 4 5 748 24.5 5 722 5 40 150 9.2 40 603
下载: 导出CSV
表 3 包含测量点的网格数量统计
Table 3 Statistics of Number of Grids Containing Measurement Points
六边形网格 四边形地理网格 L 网格数 有点网格数 有效网格占比/% 分辨率/(′) 网格数 有点网格数 有效网格占比/% 3 820 819 99.88 67.8 842 828 98.34 4 5 748 5 566 96.83 24.5 5 722 5 458 95.39 5 40 150 28 414 70.77 9.2 40 603 27 594 67.96
下载: 导出CSV
表 4 网格平均重力异常的代表误差均值统计情况
Table 4 Statistics of Commission Errors of Grid-Averaged Gravity Anomalies
六边形网格 四边形地理网格 L 网格数 代表误差/mGal 分辨率/(′) 统计网格数 代表误差/mGal 3 811 31.129 67.8 750 31.527 4 4 709 21.195 24.5 4 749 21.454 5 21 190 13.243 9.2 20 502 13.431
下载: 导出CSV
-
[1] 党亚民, 蒋涛, 陈俊勇. 全球高程基准研究进展[J]. 武汉大学学报（信息科学版）, 2022, 47（10）： 1576-1586. DANG Yamin, JIANG Tao, CHEN Junyong. Review on Research Progress of the Global Height Datum[J]. Geomatics and Information Science of Wuhan University, 2022, 47（10）： 1576-1586.
[2] SAHR K, WHITE D, KIMERLING A J. Geodesic Discrete Global Grid Systems[J]. Cartography and Geographic Information Science, 2003, 30（2）： 121-134.
[3] TOZER B, SANDWELL D T, SMITH W H F, et al. Global Bathymetry and Topography at 15 Arc Sec： SRTM15+[J]. Earth and Space Science, 2019, 6（10）： 1847-1864.
[4] 李新星. 确定地球重力场的六边形网格剖分方法研究[D]. 武汉： 武汉大学, 2021. LI Xinxing. Research on Hexagonal Grid Division Method for Determining the Earth's Gravity Field[D] Wuhan： Wuhan University, 2021.
[5] 黄谟涛, 邓凯亮, 欧阳永忠, 等. 海空重力测量及应用技术研究若干进展[J]. 武汉大学学报（信息科学版）, 2022, 47（10）： 1635-1650. HUANG Motao, DENG Kailiang, OUYANG Yongzhong, et al. Development and Study in Marine and Airborne Gravimetry and Its Application[J]. Geomatics and Information Science of Wuhan University, 2022, 47（10）： 1635-1650.
[6] LI X X, LI J C, TONG X C, et al. The Employment of Quasi-hexagonal Grids in Spherical Harmonic Analysis and Synthesis for the Earth’s Gravity Field[J]. Journal of Geodesy, 2022, 96（11）： 89.
[7] 童晓冲, 贲进. 空间信息剖分组织的全球离散格网理论与方法[M]. 北京： 测绘出版社, 2016. TONG Xiaochong, Jin BEN. The Principle and Method of Discrete Global Grid Systems for Geospatial Information Subdivision Organization[M]. Beijing： Sino Maps Press, 2016.
[8] LEE S, MORTARI D. Design of Constellations for Earth Observation with Intersatellite Links[J]. Journal of Guidance, Control, and Dynamics, 2016, 40（5）： 1263-1271.
[9] HSIA Y, BALE J B, GONEN S, et al. Design of a Hyperstable 60-Subunit Protein Dodecahedron. [J]. Nature, 2016, 535（7610）： 136-139.
[10] TEANBY N A. An Icosahedron-Based Method for even Binning of Globally Distributed Remote Sensing Data[J]. Computers & Geosciences, 2006, 32（9）： 1442-1450.
[11] DUTTON G H. A Hierarchical Coordinate System for Geoprocessing and Cartography： Working Through the Scales[M]. Berlin, Heidelberg： Springer, 1999.
[12] Dutton G. Encoding and Handling Geospatial Data with Hierarchical Triangular Meshes[C]//The 7th International Symposium on Spatial Data Handling, Amsterdam, Netherlands, 1996.
[13] DUTTON G. Improving Locational Specificity of Map Data—A Multi-resolution, Metadata-Driven Approach and Notation[J]. International Journal of Geographical Information Systems, 1996, 10（3）： 253-268.
[14] GOODCHILD M F, YANG S R. A Hierarchical Spatial Data Structure for Global Geographic Information Systems[J]. CVGIP： Graphical Models and Image Processing, 1992, 54（1）： 31-44.
[15] SUESS M, MATOS P, GUTIERREZ A, et al. Processing of SMOS Level 1c Data Onto a Discrete Global Grid[C]//IEEE International Geoscience and Remote Sensing Symposium, Anchorage AK, USA, 2004.
[16] KIDD R A, TROMMLER M, WAGNER W. The Development of a Processing Environment for Time-Series Analysis of Sea Winds Scatterometer Data[C]//IEEE International Geoscience and Remote Sensing Symposium, Toulouse, France, 2003.
[17] RANDALL D A, RINGLER T D, HEIKES R P, et al. Climate Modeling with Spherical Geodesic Grids[J]. Computing in Science & Engineering, 2002, 4（5）： 32-41.
[18] TSATCHA D, SAUX É, CLARAMUNT C. A Bidirectional Path-Finding Algorithm and Data Structure for Maritime Routing[J]. International Journal of Geographical Information Science, 2014, 28（7）： 1355-1377.
[19] BONDARUK B, ROBERTS S A, ROBERTSON C. Assessing the State of the Art in Discrete Global Grid Systems： OGC Criteria and Present Functiona-lity 1[J]. Geomatica, 2020, 74（1）： 9-30.
[20] 杨正辉, 魏子卿, 马健. 第二类连带勒让德函数及其一阶、二阶导数的递推计算方法[J]. 武汉大学学报（信息科学版）, 2020, 45（2）： 213-218. YANG Zhenghui, WEI Ziqing, MA Jian. Recursive Calculation Method for the Second Kind of Associated Legendre Functions and Its First and Second Derivatives[J]. Geomatics and Information Science of Wuhan University, 2020, 45（2）： 213-218.
[21] 崔家武, 周波阳, 罗志才, 等. 利用MPI并行算法实现球谐综合的效率分析[J]. 武汉大学学报（信息科学版）, 2019, 44（12）： 1802-1807. CUI Jiawu, ZHOU Boyang, LUO Zhicai, et al. Efficiency Analysis of Spherical Harmonic Synthesis Based on MPI Parallel Algorithm[J]. Geomatics and Information Science of Wuhan University, 2019, 44（12）： 1802-1807.
[22] 刘聪, 王正涛, 张华伟, 等. 利用残差地形模型空域法精化局部地球重力场[J]. 武汉大学学报（信息科学版）, 2022, 47（3）： 369-376. LIU Cong, WANG Zhengtao, ZHANG Huawei, et al. Refining Local Earth’s Gravity in Spatial Domain with Residual Terrain Modelling Technique[J]. Geomatics and Information Science of Wuhan University, 2022, 47（3）： 369-376.
[23] 李新星, 李建成, 刘晓刚, 等. 球谐旋转变换结合非全次Legendre方法的局部六边形网格重力场球谐综合[J]. 地球物理学报, 2021, 64（11）： 3933-3947. LI Xinxing, LI Jiancheng, LIU Xiaogang, et al. Spherical Harmonic Synthesis of Local Hexagonal Grid Point Gravity Anomalies with Non-Full-Order Legendre Method Combined with Spherical Harmonic Rotation Transformation[J]. Chinese Journal of Geophysics, 2021, 64（11）： 3933-3947.
[24] 黄谟涛, 管铮, 欧阳永忠. 海洋平均重力异常计算与精度估计[J]. 武汉大学学报（信息科学版）, 1995, 20（4）： 327-331. HUANG Motao, GUAN Zheng, OUYANG Yongzhong. Calculation and Accuracy Estimation of Marine Mean Free-Air Gravity Anomaly[J]. Geomatics and Information Science of Wuhan University, 1995, 20（4）： 327-331.
[25] 黄谟涛, 刘敏, 欧阳永忠, 等. 海洋重力场特征统计模型计算与分析[J]. 武汉大学学报（信息科学版）, 2019, 44（3）： 317-327. HUANG Motao, LIU Min, OUYANG Yongzhong, et al. Analysis and Calculation of the Statistical Models of Marine Gravity Field Character[J]. Geomatics and Information Science of Wuhan University, 2019, 44（3）： 317-327.
[26] 陆仲连. 地球重力场理论与方法[M]. 北京： 解放军出版社, 1996. LU Zhonglian. Theory and Method of Earth Gravity Field [M]. Beijing： The People's Liberation Army Press, 1996.
[27] 李姗姗, 吴晓平, 张传定, 等. 我国重力场新的统计特征参数的计算分析[J]. 地球物理学报, 2010, 53（5）： 1099-1108. LI Shanshan, WU Xiaoping, ZHANG Chuanding, et al. Calculation and Analysis of the New Statistical Character Parameters of Gravity Field in China[J]. Chinese Journal of Geophysics, 2010, 53（5）： 1099-1108.
[28] 国家质量监督检验检疫总局. 加密重力测量规范： GB/T 17944—2000[S]. 北京： 中国标准出版社, 2004. General Administration of Quality Supervision, Inspection and Quarantine of the People’s Republic of China. Specifications for the Dense Gravity Measurement： GB/T 17944—2000[S]. Beijing： Standards Press of China, 2004.
[29] 郑明阳, 贲进, 周建彬, 等. 局部区域多孔径六边形格网系统快速生成算法[J]. 武汉大学学报（信息科学版）, 2022, 47（9）： 1376-1382. ZHENG Mingyang, Jin BEN, ZHOU Jianbin, et al. Fast Generation Algorithm of Multi-aperture Hexagonal Grid Systems of Regional-Scale[J]. Geomatics and Information Science of Wuhan University, 2022, 47（9）： 1376-1382.
计量
- 文章访问数: 410
- HTML全文浏览量: 25
- PDF下载量: 32
