A Method for Solving Point Cloud Registration Models Using Dual Quaternion Descriptions of Point‑Planar Feature Constraints
-
摘要:
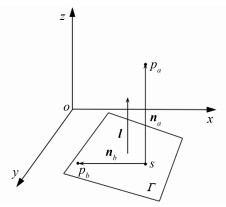
点云数据的高精度配准是保证空间物体表面三维数据完整性的关键,针对相邻测站点云数据存在位置、姿态和尺度差异的问题,提出一种在点面特征约束下利用对偶四元素描述的点云配准模型求解方法。首先,利用对偶四元素表示空间相似变换的旋转矩阵和平移向量,在此基础上顾及尺度因子,依据点在平面内和点在平面外分别所构建的向量与平面的法向量之间存在垂直和平行的空间拓扑关系,并以此作为空间相似变换的约束条件,基于最小二乘准则构建平差模型;然后,引入Levenberg-Marquardt法解算平差模型,以避免平差处理中可能由初始值的不恰当性,或由雅克比矩阵所构建的实对称矩阵接近奇异时而导致迭代不收敛;最后,通过两组实验与现有方法进行对比分析,实验结果表明,所提方法能有效实现点云配准。因此,点面特征约束下顾及尺度因子且利用对偶四元素实现空间相似变换的方法具有较强的实用价值。
Abstract:ObjectivesThe high-precision registration of point cloud data is the key to ensure the integrity of 3D data on the surface of spatial objects. To address the problem that there are differences in position, attitude and scale of cloud data from neighboring stations, a method is proposed to solve the registration model of point cloud described by the dual quaternion under the constraints of point-planar feature.
MethodsFirst, the rotation matrix and translation vector of the spatially similar transformation are represented by the dual quaternion, based on which the scale factor is taken into account and the vertical and parallel spatial topological relationships exist between the vectors constructed by the points in the plane and the points out of the plane respectively and the normal vectors of the plane, and this is used as the constraint of the spatially similar transformation to construct the parity model based on the least squares criterion. Then the Levenberg-Marquardt method is introduced to solve the level-difference model to avoid the possible non-convergence of the iterations in the level-difference treatment due to the inappropriateness of the initial values or due to the fact that the real symmetric matrix constructed by the Jacobi matrix is close to singularity.
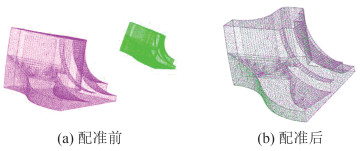
ResultTwo sets of experiments are compared and analyzed with the existing methods, and the experimental results show that the proposed method can effectively achieve point cloud registration.
ConclusionsTherefore, the method that takes into account the scale factor under the point-planar feature constraint and uses the dual quaternion to realize the spatial similarity transformation has a strong practical value.
-
Keywords:
- measurement /
- point cloud registration /
- dual quaternion /
- point and surface features /
- scale factor
-
随着社会的进步和技术的发展,人们对于位置服务的需求日益增加。特别是自动驾驶、无人机和物联网等技术的日益进步,越来越多的人关注如何在复杂环境下实现精密导航定位。然而,任何单一的技术都不能够在所有环境下随时提供高精度、高可用性、高连续性和高可靠性的定位结果[1]。因此,融合多种定位手段成为了导航定位领域研究的热点[2-3],全球卫星导航定位系统(global navigation satellite system,GNSS)、惯性导航系统(inertial navigation system,INS)和视觉的组合是其中一种具有潜力的组合方式。
GNSS具有全球、全天候、实时的导航定位授时能力,被广泛应用于交通等领域[4]。Wübbena等[5]于2005年提出PPP-RTK(precise point positioning real time kinematic)的概念,PPP-RTK急需解决的问题是模糊度的快速固定[6]。文献[7-9]分别提出了未校正相位延迟(uncalibrated phase bias,UPD)、整数钟和解耦钟的固定方法,文献[10-11]基于实测数据对这3种方法进行了比较分析。近年来,PPP-RTK技术逐渐从无电离层组合向非差非组合方向发展。非差非组合通过约束电离层和对流层参数,在避免了无电离层组合将观测噪声放大了近3倍的缺点的同时,能进一步加速PPP的收敛[12]。对非差非组合而言,通常认为大气增强和模糊度固定PPP技术即为PPP-RTK技术。PPP-RTK避免了RTK覆盖范围受限和PPP收敛时间长的缺陷,在车载导航等领域具有很广泛的应用前景。鉴于此,一些地区的官方机构已经推出了PPP-RTK服务,例如日本的准天顶星系统(quasi-zenith satellite system,QZSS)于2018年开始提供厘米级精度的服务[13]。
尽管PPP-RTK具有众多优势,但是仍免不了受卫星信号被遮挡的影响,意味着在复杂环境下,PPP-RTK不能够提供高精度的定位服务。当信号受建筑物遮挡影响很严重时,可用卫星数少于4颗,此时PPP-RTK将不能提供定位结果。INS能在没有外部输入的情况下提供导航信息,但是误差随时间发散较快。INS在GNSS信号被遮挡时辅助提供定位结果,GNSS约束INS的误差发散,两者能形成良好的互补。Rabbou等[14]和Gao等[15]研究了PPP/INS紧组合,Gu等[16]对大气增强PPP/INS紧组合进行了深入分析,但PPP-RTK/INS相关的研究相对较少,仍有待进一步深入研究。
PPP-RTK与INS进行组合在GNSS信号较长时间中断时,由于INS航位推算误差的发散,依旧产生较大的定位误差,因此需要进一步引入其他辅助的传感器来限制INS的误漂移误差。视觉里程计(visual odometry,VO)的误差发散较慢,可以对INS的漂移误差进行约束。但单目视觉里程计缺少尺度信息,因此可以通过INS和VO组合的方式,通过INS来恢复单目VO的尺度信息,即为视觉惯性里程计(visual inertial odometry,VIO)。VIO的融合方式主要分基于扩展卡尔曼滤波[17-19]和非线性优化[20-21],前者结构简单,但线性化误差较大,后者精度更高,但对算力要求很高,计算负担大。多状态约束卡尔曼滤波(multistate constraint Kalman filter,MSCKF)[17]是一种基于扩展卡尔曼滤波的VIO,能在大尺度环境下提供良好的状态估计。MSCKF将多帧影像对同一个特征点的观测转化为相机状态之间的约束,计算复杂度与特征点数仅成线性关系[22]。
GNSS、INS和视觉的组合方式能更好地形成互补,GNSS为INS和视觉提供绝对位置信息,INS和视觉在GNSS信号被干扰时提供比较精确的航位推算信息。Kim等[23]等使用六自由度的同步定位与测图(simultaneous localization and mapping,SLAM)辅助GNSS/INS在GNSS拒止位置环境下提供可靠的导航解,Won等[24]提出了GNSS、INS和视觉的选择性组合方法,并且证明选择性组合方法要明显优于非组合的方式。以上这些组合中仅仅用到了GNSS的伪距定位,相位的潜力未能得到充分挖掘。Liu[25]提出了GNSS/INS/立体视觉/地图匹配系统的紧组合模型;Li等[26]实现了RTK、INS和单目相机的紧组合;Li等[27]实现了城市复杂环境下PPP和立体视觉惯性导航系统(stereo visual INS,SVINS)的半紧组合,并进行了测试分析;方文涛[28]实现了大气增强PPP/微机电系统(micro-electro-mechanical system,MEMS)惯导/视觉里程计融合定位研究。PPP和RTK与其他定位及技术的组合研究均有了一定的进展,但目前对于PPP-RTK的研究集中于改善PPP-RTK本身的性能,与其他定位技术组合的研究较少。PPP-RTK、INS和视觉的组合有望实现在城市环境下高精度连续定位,但其实际性能表现仍有待进一步研究。
对此,本文提出多系统非差非组合PPP-RTK与VIO的半紧组合模型,并且评估多系统PPP-RTK/VIO半紧组合在城市复杂环境下的定位表现,探究多系统PPP-RTK/VIO半紧组合在城市车载导航应用场景下的潜力。
1 PPP-RTK/VIO组合模型
PPP-RTK/VIO组合模型是由非差非组合PPP-RTK和单目VIO组成,基于非差非组合PPP-RTK和单目VIO模型推导,可得到组合模型。
1.1 PPP-RTK模型
非差非组合GNSS PPP观测模型通常表示如下[29-30]:
式中,
、 分别为伪距和相位观测值; 为卫星至接收机的几何距离; 为接收机钟差误差; 分别为天顶对流层误差和电离层误差; 、 分别为对应的投影函数; 分别为接收机端和卫星端硬件延迟误差; 、 分别为浮点模糊度和波长; 分别为伪距和相位观测噪声;角标 分别表示对应的频率、卫星号、接收机 系统,本文中主要涉及全球定位系统(global positioning system,GPS)和北斗卫星导航系统(BeiDou navigation satellite system,BDS),分别简记为G和C。 对卫星端硬件延迟进行改正以及使用对流层的先验模型后,对式(1)进行线性化,则有:
式中,
、 分别为伪距和相位的验前残差(observed-minus-computed,OMC); 为卫地距在接收机坐标系下的方向余弦向量; 为接收机位置误差向量; 为天顶对流层湿延迟残差。对于电离层延迟的参数化,采用Shi等[31]和Zhao等[29]提出的DESIGN-5(deterministic plus stochastic ionosphere model for GNSS)模型,对电离层误差建模如下: 式中,
为描述电离层趋势项变化的系数,用于描述单站上空电离层延迟的空间分布,反映了电离层延迟的结构特性;随机参数 用于表示电离层在时域上的随机特性;dL、dB为电离层穿刺点(ionospheric pierce point,IPP)与测站近似坐标的经纬度差值; 为电离层误差虚拟观测值,可以通过先验模型或者高精度电离层产品获取; 是其对应的观测噪声。由此可将待估状态量 表示为: 式中
为接收机对应GPS和BDS的钟差; 是两个频率上的浮点模糊度; 和 是DESIGN模型的参数。 对于PPP-RTK中模糊度整数解,浮点模糊度
进一步建模为: 式中,n代表整数模糊度;
、 分别代表接收机和卫星端UPD(uncalibrated phase delays)。由式(2)得到浮点模糊度之后,通过服务端提供的UPD产品来恢复模糊度的整数特性。进一步采用LAMBDA(least-squares ambiguity decorrelation adjustment)[32]方法来搜索最优的模糊度固定值。将模糊度固定值作为约束条件,得到模糊度固定的PPP定位结果[33-34]。 1.2 INS模型
选用e系(地心地固系)作为机械编排的参考系,惯性系统的误差状态建模为:
式中,
表示地心地固坐标系下INS中心的位置改正向量; 表示地心地固坐标系下INS中心的速度改正向量; 表示姿态误差; 、 分别表示加速度计和陀螺仪的零偏误差; 、 分别表示加速度计和陀螺仪的比例因子误差。对于上述的位置、速度、姿态误差,姿态误差模型采用 角模型,其连续型动态模型通常表达如下[35]: $$\begin{array}{l} \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;{\left( {{\rm{\delta }}\dot x_{{\rm{INS}}}^e{\rm{\delta }}\dot v_{{\rm{INS}}}^e\dot \phi } \right)^{\rm{T}}} = \\ \left( {\begin{array}{*{20}{c}} {{\rm{\delta }}v_{{\rm{INS}}}^e}\\ { - {\rm{2}}\omega _{ie}^e \times {\rm{\delta }}v_{{\rm{INS}}}^e + R_b^e{f^b} \times \phi + R_b^e{\rm{\delta }}{f^b} + {\rm{\delta }}{g^e}}\\ { - \omega _{ie}^e \times \phi - R_b^e\delta \omega _{ib}^b} \end{array}} \right) \end{array}$$ 式中,
为地球自转的角速度矢量; 为载体坐标系转换到地心地固系的方向余弦矩阵; 为经误差补偿后加速度计输出的三维比力矢量; 和 是惯导的器件误差,考虑利用惯导零偏误差和比例因子误差来建模惯性器件误差方程: 式中
、 分别是陀螺仪和加速度计的量测噪声。而对陀螺仪和加速度计零偏、比例因子误差建模为一阶高斯马尔可夫过程: 式中,
、 分别为加速度计和陀螺仪零偏误差、比例因子误差一阶高斯马尔可夫过程的驱动噪声; 、 为对应的相关时间。 1.3 VIO模型
基于INS误差状态量,构建视觉惯导误差状态量。对第i时刻的相机状态而言,建立其误差状态为
,因此对第k时刻的基于MSCKF的VIO误差状态量建模如下: 式中,
、 分别为i时刻(i = j,j+1,j+2… k)相机的位置误差向量和失准角向量,当有新时刻的视觉数据引入时,就对上述状态进行状态增广。 基于不在当前帧所观测到的静止特征点,可以构建多状态约束滤波的量测模型。通过数学变换实现将前n个时刻的相机状态与相应的静止特征点之间的约束关系转换为前n个时刻相机状态之间的约束关系。假定k+1时刻静止特征点
不被观测到,但在 被观测到的任意i时刻均有: 式中,
为 点在第i时刻相机坐标系下的位置向量; 和 分别表示第i时刻相机相对于e系的方向余弦矩阵和位置向量; 为 点在e系下的位置向量,可通过多张影像三角测量获得。 构建相机量测误差状态量如下:
式中,
为特征点 重投影得到的像素坐标估计值; 为特征点 在第i时刻的像素观测值; 为对应的观测噪声。针对量测方程的系数阵,采用链式法则进行求解可得: $$\begin{array}{l} {z_{{P_k},i}} = {\rm{\delta }}{\left( {{u_{{P_k},i}},{v_{{P_k},i}}} \right)^{\rm{T}}} = \frac{{\partial {{\left( {{u_{{P_k},i}},{v_{{P_k},i}}} \right)}^{\rm{T}}}}}{{\partial p_{{P_k},i}^{{c_i}}}}{\rm{\delta }}p_{{P_k},i}^{{c_i}} = \\ \;\;\;\;\;\;\;\;\;\;{H_{x,i}}{x_{i,c}} + {H_{f,i}}{\rm{\delta }}p_{{P_k}}^e + {\varepsilon _{{P_k},i}} \end{array}$$ 式中,
为相机焦距; 是特征点在相机坐标系下的位置;×表示由向量构建反对称矩阵。 由于
不是待估状态量,并且对 的量均为已知,这些量可由惯性测量单元(inertial measurement unit,IMU)机械编排获得,因此可以针对 求解其左零空间矩阵A,使得: 对式(13)两边同时乘以
矩阵,可得: 式中,
; 。 1.4 PPP-RTK/VIO半紧组合模型
在实际硬件平台上,惯导的位置
与GNSS的天线相位中心位置 不重合,两者之间的空间关系表示为: 式中,
为杆臂信息,描述的是GNSS天线相位中心到IMU中心的向量在IMU坐标系下的投影。对式(16)进行微分可得到: 将PPP-RTK获得的位置信息记为
,VIO递推得到的位置记为 ,则可得到PPP-RTK/VIO的半紧组合观测方程: 1.5 模型结构
PPP-RTK/VIO半紧组合算法结构如图 1所示,GNSS接收到观测数据后,结合大气增强数据进行PPP解算,得到浮点解后,加入UPD产品进行模糊度固定得到PPP-RTK定位结果。IMU进行机械编排推算下一时刻的位置,当接收到相机数据后,进行特征点提取和跟踪,相机和IMU数据同步则进行相机状态增广,并进行融合获得定位结果,当VIO与GNSS数据同步后,加入PPP-RTK定位结果到VIO滤波器中,得到PPP-RTK/VIO半紧组合输出的导航信息。
2 实验与结果分析
为了评估多系统PPP-RTK/VIO半紧组合的定位精度和可靠性,开发了FUSING(Fusing in GNSS)软件平台。目前,该平台实现了多系统轨道钟差估计,电离层、对流层建模、多频多系统偏差解算以及多源融合定位数据处理等[36]。本文在FUSING平台的基础上,进一步实现了多系统PPP-RTK/VIO半紧组合数据处理,并利用车载实验进行了验证。
2.1 实验说明
测试数据所采用的车载平台如图 2所示。MEMS级惯导采集的数据适用于测试评估组合系统的表现,导航级惯导适用于计算参考解。两种IMU采样频率都是200 Hz,设备参数如表 1所示。灰度相机Basler acA640-90gm以659×494的分辨率、10 Hz的频率采集图像数据。UBLOX-M8T用于时间同步,产生PPS(pulse per second)信号触发相机曝光。GNSS数据由Trimble Net R9接收机以1 Hz的频率采集。IMU中心和GNSS天线相位中心的杆臂补偿在实验前手动测量,IMU和相机的外参由联合标定工具Kalibr( https://github.com/ethz-asl/kalibr/)标定。
表 1 惯导设备参数Table 1. Performance Specification of the IMU Sensors惯导设备 随机游走 零偏稳定性 速度/( -1 -1) 角度/( -1) 陀螺/( -1) 加速度计/mGal MEMS级惯导 0.03 0.17 8 200 导航级惯导 0.003 0.03 0.027 15 测试数据为2020-01-02于武汉大学校内采集,采集数据时车速大约为5 m/s。图 3所示为测试轨迹和真实场景,可以看到测试环境中遮挡物较多,道路狭窄,两侧道路上都有较多的树木,还有一些建筑物,易对GNSS信号造成干扰。
如图 4所示为可用卫星数和截止角为10°时的位置精度强弱度(position dilution of precision,PDOP)值,GPS和GPS+BDS的平均可用卫星数分别为5.21颗和10.72颗。从图 4可以看出可用卫星数波动较大,说明GNSS信号受干扰比较严重;GPS和GPS+BDS的PDOP值分别为20.06和4.45,可见,卫星可用数下降时,PDOP值显著增大。
考虑到解算大气增强和UPD产品需要基站数据,收集了武汉周边的基站数据,图 5所示为用于解算大气增强和UPD产品的基站分布图,一共有7个基站,站间平均距离为40 km。图 5中红色轨迹为车载测试的轨迹。GNSS数据处理策略如表 2所示,定位结果以RMS(root mean square)的方式评估,用RTK/INS双向平滑松组合作为参考解。参考解采用商业软件GINS计算的结果,其标称精度可达到平面2 cm,高程3 cm。
表 2 GNSS数据处理策略Table 2. GNSS Data Processing Strategy2.2 结果分析
所有解算的结果均以1 Hz的频率输出。G、C分别代表GPS系统和北斗系统,LC代表松组合。从图 6(a)可以看出,G-PPP-RTK明显优于G-PPP的定位效果,加入北斗系统后,定位误差序列的波动减小,定位精度更高,但是仍然是跳跃很明显,定位不够稳定。结合图 4卫星可用数可以看出,单GPS有很多历元可用卫星数小于4颗,此时无法解算出定位结果,定位误差序列图中被GC-PPP-RTK的定位误差序列掩盖而难以直观看出。但从东向周内秒355 250 s处(图 6(a)中黑框所示)仍旧可以明显看出G-PPP定位中断,而北斗的引入改善了定位连续性。加入惯导后(图 6(b)),GC-PPP-RTK因受信号干扰而定位精度不稳定的情况得到明显改善,水平和高程方向均很明显。相比于加入惯导,直接引入VIO,对GC-PPP-RTK的精度提升效果更加明显,北、东、地3个方向的定位误差序列都趋于比较稳定的状态,由此可以看出,GC-PPP-RTK/VIO的组合方式明显优于其余4种定位解算策略。
为了进一步验证结论,表 3中列出了各种解算策略的统计精度。其中可用性定义为输出定位结果的历元数与该时段内的总历元数的比值,以此来评估定位的连续性。从表 3可以看出,北斗系统的引入大大提高了定位的连续性。定位精度方面,由于定位连续性显著提高,GC-PPP-RTK在信号被干扰的情况下输出定位结果的历元数增加,导致误差较大的定位结果占比增加,因此高程方向上的统计结果要比G-PPP和G-PPP-RTK差。为了体现不同组合方式的定位效果,表 4进一步以GC-PPP-RTK为参考,给出了GC-PPP-RTK/INS松组合和GC-PPP-RTK/VIO半紧组合对定位精度的提升效果。统计结果显示,引入惯导、VIO后,水平和高程相对于GC-PPP-RTK,均带来了20%~50%的精度提升,GC-PPP-RTK/VIO的定位效果最好,定位精度水平达到0.58 m,高程达到1.12 m,基本满足车道级的定位需求。
表 3 PPP和PPP-RTK的定位误差(RMS)统计结果Table 3. RMS of the Position Difference of PPP and PPP-RTK解算策略 水平/m 精度提升/% 高程/m 精度提升/% 可用性/% G-PPP 0.97 2.21 82.2 G-PPP-RTK 0.91 6.2 1.46 33.9 82.2 GC-PPP-RTK 0.85 12.4 2.29 -3.6 99.4 表 4 不同组合方式的定位误差(RMS)统计结果Table 4. RMS of the Position Difference of Different Integration Models解算策略 水平/m 精度提升/% 高程/m 精度提升/% 可用性/% GC-PPP-RTK 0.85 2.29 99.4 GC-PPP-RTK/INS LC 0.62 27.1 1.64 28.4 100 GC-PPP-RTK/VIO 0.58 31.8 1.12 51.1 100 考虑到在车载导航中速度和姿态也是重要的导航信息,本文也对组合系统的测速和姿态估计能力进行了评估。以GC-PPP-RTK/INS LC作为参考解,从图 7可以看出,GC-PPP-RTK/VIO的测速精度明显更好,精度更高,也更加稳定。由于GC-PPP-RTK高程方向定位误差较大且波动非常明显,一定程度上导致GC-PPP-RTK/INS LC高程方向的测速精度较低且波动很大,明显低于平面测速精度。GC-PPP-RTK/VIO组合方式对高程方向的定位约束较好,定位精度较高,高程方向的测速精度也明显提高。从表 5可以看出,GC-PPP-RTK/VIO在北向、东向和地向的测速精度分别达到0.04 m/s、0.04 m/s和0.02 m/s,相比于GC-PPP-RTK/INS LC,在地向的速度估计提升了83.3%,效果非常显著,北向和东向也分别提升了33.3%和20.0%。
表 5 不同解算策略的测速误差(RMS)统计结果Table 5. RMS of the Velocity Estimation Difference of Different Solutions解算策略 北向/(m·s-1) 东向/(m·s-1) 地向/(m·s-1) GC-PPP-RTK/INS LC 0.06 0.05 0.12 GC-PPP-RTK/VIO 0.04 0.04 0.02 精度提升/% 33.3 20.0 83.3 由于车载导航中,横滚角和俯仰角都是小角度且比较稳定,所以两种解算策略对横滚角和俯仰角的估计精度没有明显差别,这从图 8的误差序列和表 6的统计结果都可以看出来。而对于航向角,两种解算策略的估计精度则差别明显,GC-PPP-RTK/VIO对航向角的估计精度明显优于GC-PPP-RTK/INS LC,提升达到73.0%。
表 6 不同解算策略的姿态估计误差(RMS)统计结果Table 6. RMS of the Pose Estimation Difference of Different Solutions解算策略 横滚角/(°) 俯仰角/(°) 航向角/(°) GC-PPP-RTK/INS LC 0.10 0.06 0.63 GC-PPP-RTK/VIO 0.10 0.06 0.17 精度提升/% 0 0 73.0 3 结语
测试结果表明,为了改善城市环境下车载导航的定位定姿和测速表现,本文提出了多系统PPP-RTK/VIO半紧组合算法模型,并利用城市环境车载数据进行了测试,测试环境选用武汉大学校园,为典型的GNSS信号受干扰比较严重的场景。另外收集了武汉周边的基站数据用于解算大气增强和UPD产品。
城市复杂环境下,PPP-RTK定位精度相比于PPP有明显改善,但定位精度仍然有待提高。PPP-RTK与VIO组合能带来明显的精度提高,相比于GC-PPP-RTK,平面和高程定位精度分别提升了31.8%和51.1%,达到了平面0.58 m、高程1.12 m的定位精度水平,基本满足车道级定位的需求。在测速和姿态估计方面,GC-PPP-RTK/VIO明显优于GC-PPP-RTK/INS LC,测速精度北向、东向和地向分别达到了0.04 m/s、0.04 m/s和0.02 m/s,横滚角、俯仰角和航向角的估计精度分别达到了0.10°、0.06°和0.17°。
本文实现了多系统PPP-RTK/VIO半紧组合算法,PPP-RTK/VIO紧组合算法模型仍有待进一步研究。PPP-RTK/VIO紧组合算法在使用一个滤波器的情况下,如何维持滤波器的稳定性,VIO的加入能否给PPP-RTK的模糊度固定带来明显的改善效果,以及其定位定姿和测速潜力等,都有待进一步研究和挖掘。
-
表 1 理论配准参数与解算出的配准参数
Table 1 Theoretical Values and Calculated Registration Parameters
统计项 旋转矩阵R 平移向量T/m 尺度因子λ 理论值 0.969 8 -0.141 3 0.198 6 -3.477 4 2.000 0 0.171 0 0.975 1 -0.141 3 -10.821 8 -0.173 6 0.171 0 0.969 8 1.067 1 解算值 0.969 5 -0.141 5 0.198 2 -3.477 8 2.000 0 0.170 6 0.975 4 -0.141 0 -10.822 1 -0.173 2 0.171 2 0.969 6 1.066 9 表 2 同名特征平面法向量和平面距离的残差及其RMSE
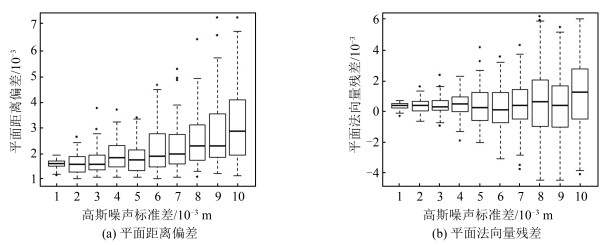
Table 2 Residuals of Normal and Planar Distances of Same Name Feature Plane and Their RMSE
$ \mathrm{\Delta } $nx/10-3 $ \mathrm{\Delta } $ny/10-3 $ \mathrm{\Delta } $nz/10-3 m$ {}_{\mathrm{\Delta }n} $/10-3 $ \mathrm{\Delta }d $/mm m$ {}_{\mathrm{\Delta }d} $/mm -0.001 -0.007 0.002 0.251 0.014 5 0.015 2 0.000 0.019 -0.028 0.017 2 -0.038 0.049 0.382 0.014 9 0.044 0.310 -0.058 0.015 2 0.000 -0.002 0.001 0.014 3 表 3 不同方法解算的配准参数
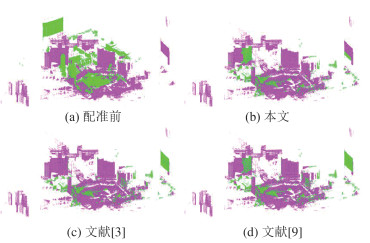
Table 3 Registration Parameters Calculated by Different Methods
方法 旋转矩阵R 平移向量T/m 尺度因子λ 本文 -0.208 7 0.978 3 0.000 1 10.789 8 1.000 4 -0.978 2 -0.208 7 0.000 0 -50.956 2 0.000 0 0.000 0 1.000 0 -0.409 3 文献[3] -0.209 4 0.978 4 0.000 1 10.786 7 0.999 5 -0.977 3 -0.208 2 0.000 0 -50.959 2 0.000 0 0.000 0 1.000 0 -0.408 4 文献[9] -0.209 1 0.977 7 0.000 0 10.788 4 0.999 9 -0.977 9 -0.207 8 0.000 0 -50.953 5 0.000 0 0.000 1 1.000 0 -0.405 9 表 4 不同方法配准后同名特征平面法向量和平面距离的残差及其RMSE
Table 4 Residuals of Normal and Planar Distances of Same Name Feature Plane and Their RMSE After Registration by Different Methods
方法 $ \mathrm{\Delta } $nx $ \mathrm{\Delta } $ny $ \mathrm{\Delta } $nz m$ {}_{\mathrm{\Delta }n} $ $ \mathrm{\Delta }d $/mm m$ {}_{\mathrm{\Delta }d} $/mm 本文 -0.001 5 0.004 2 -0.001 1 0.024 8 0.071 3 0.069 8 -0.005 0 -0.002 1 0.004 4 0.059 8 0.046 6 0.011 5 0.007 6 0.055 0 0.001 0 0.000 9 -0.005 0 0.065 2 -0.000 8 0.000 3 0.000 0 0.059 8 文献[3] -0.001 6 0.004 3 -0.001 2 0.025 5 0.088 4 0.082 2 -0.005 3 -0.002 6 0.004 8 0.077 4 0.047 7 0.012 4 0.008 3 0.069 8 0.001 7 0.000 4 -0.005 0 0.068 3 -0.000 9 0.000 5 0.000 0 0.060 9 文献[9] -0.001 7 0.004 5 -0.001 8 0.025 0 0.051 5 0.070 0 -0.005 5 -0.002 3 0.005 1 0.076 6 0.045 6 0.014 9 0.007 9 0.056 5 0.001 2 0.001 9 -0.005 8 0.062 4 -0.000 8 0.000 3 0.000 0 0.063 1 -
[1] 盛庆红, 陈姝文, 柳建锋, 等. 基于Plücker直线的LiDAR点云配准法[J]. 测绘学报, 2016, 45(1): 58-64. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB201601010.htm Sheng Qinghong, Chen Shuwen, Liu Jianfeng, et al. LiDAR Point Cloud Registration Based on Plücker Line[J]. Acta Geodaetica et Cartographica Sinica, 2016, 45(1): 58-64. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB201601010.htm
[2] 闫利, 刘华, 陈长军, 等. 无地面控制点的车载激光扫描系统外标定方法[J]. 武汉大学学报(信息科学版), 2015, 40(08): 1018-1022. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201508005.htm Yan Li, Liu Hua, Chen Changjun, et al. A Calibration Method of Mobile Laser System Without Control Points[J]. Geomatics and Information Science of Wuhan University, 2015, 40(8): 1018-1022. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201508005.htm
[3] 王永波, 汪云甲, 韩新哲, 等. 点线特征约束下基于单位四元数描述的LiDAR点云配准算法[J]. 中国矿业大学学报, 2018, 47(3): 671-677. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGKD201803022.htm Wang Yongbo, Wang Yunjia, Han Xinzhe, et al. A Unit Quaternion Based, Point-Linear Feature Constrained Registration Approach for Terrestrial LiDAR Point Clouds[J]. Journal of China University of Mining & Technology, 2018, 47(3): 671-677. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGKD201803022.htm
[4] 王畅, 舒勤, 杨赟秀, 等. 利用结构特征的点云快速配准算法[J]. 光学学报, 2018, 38(9): 175-182. https://www.cnki.com.cn/Article/CJFDTOTAL-GXXB201809023.htm Wang Chang, Shu Qin, Yang Yunxiu, et al. Quick Registration Algorithm of Point Clouds Using Structure Feature[J]. Acta Optica Sinica, 2018, 38(9): 175-182. https://www.cnki.com.cn/Article/CJFDTOTAL-GXXB201809023.htm
[5] Nakamura T, Wakita S. Robust Global Scan Matching Method Using Congruence Transformation Invariant Feature Descriptors and a Geometric Constraint Between Keypoints[C]// IEEE International Conference on Systems, Man, and Cybernetics, San Diego, USA, 2014.
[6] Jensfelt P, Kristensen S. Active Global Localization for a Mobile Robot Using Multiple Hypothesis Tracking[J]. IEEE Transactions on Robotics and Automation, 2001, 17(5): 748-760. doi: 10.1109/70.964673
[7] Guo Y L, Bennamoun M, Sohel F, et al. A Comprehensive Performance Evaluation of 3D Local Feature Descriptors[J]. International Journal of Computer Vision, 2016, 116(1): 66-89. doi: 10.1007/s11263-015-0824-y
[8] Ge X M. Automatic Markerless Registration of Point Clouds with Semantic-Keypoint-Based 4-Points Congruent Sets[J]. ISPRS Journal of Photogrammetry and Remote Sensing, 2017, 130: 344-357. doi: 10.1016/j.isprsjprs.2017.06.011
[9] 柴双武, 杨晓琴. 基于对偶四元数构建的直线基元点云拼接方法[J]. 光学学报, 2019, 39(12): 416-424. https://www.cnki.com.cn/Article/CJFDTOTAL-GXXB201912048.htm Chai Shuangwu, Yang Xiaoqin. Line Primitive Point Cloud Registration Method Based on Dual Quaternion[J]. Acta Optica Sinica, 2019, 39(12): 416-424. https://www.cnki.com.cn/Article/CJFDTOTAL-GXXB201912048.htm
[10] Li J, Zhong R, Hu Q, et al. Feature-Based Laser Scan Matching and Its Application for Indoor Mapping[J]. Sensors, 2016, 16(8): 1265. doi: 10.3390/s16081265
[11] Núñez P, Vázquez M N, Toro J, et al. Natural Landmark Extraction for Mobile Robot Navigation Based on an Adaptive Curvature Estimation[J]. Robotics and Autonomous Systems, 2008, 56(3): 247-264. doi: 10.1016/j.robot.2007.07.005
[12] Yang B S, Dong Z, Liang F X, et al. Automatic Registration of Large-Scale Urban Scene Point Clouds Based on Semantic Feature Points[J]. ISPRS Journal of Photogrammetry and Remote Sensing, 2016, 113: 43-58. doi: 10.1016/j.isprsjprs.2015.12.005
[13] Zhang J, Singh S. Low-Drift and Real-Time LiDAR Odometry and Mapping[J]. Autonomous Robots, 2017, 41(2): 401-416. doi: 10.1007/s10514-016-9548-2
[14] 郑德华, 岳东杰, 岳建平. 基于几何特征约束的建筑物点云配准算法[J]. 测绘学报, 2008, 37(4): 464-468. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB200804013.htm Zheng Dehua, Yue Dongjie, Yue Jianping. Geometric Feature Constraint Based Algorithm for Building Scanning Point Cloud Registration[J]. Acta Geodaetica et Cartographica Sinica, 2008, 37(4): 464-468. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB200804013.htm
[15] Zhang D, Huang T, Li G, et al. Robust Algorithm for Registration of Building Point Clouds Using Planar Patches[J]. ISPRS Journal of Photogrammetry and Remote Sensing, 2012, 138(1): 31-37.
[16] Wang H, Wang X. 3D Registration Based on Planar Feature Segmentation and Plane Fit[J]. Applied Mechanics and Materials, 2012, 233: 274-277.
[17] 张良, 马洪超, 高广, 等. 点、线相似不变性的城区航空影像与机载激光雷达点云自动配准[J]. 测绘学报, 2014, 43(4): 372-379. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB201404009.htm Zhang Liang, Ma Hongchao, Gao Guang, et al. Automatic Registration of Urban Aerial Images with Airborne LiDAR Points Based on Line-Point Similarity Invariants[J]. Acta Geodaetica et Cartographica Sinica, 2014, 43(4): 372-379. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB201404009.htm
[18] Yuan X, Zhao C, Tang Z, et al. LiDAR Scan-Matching for Mobile Robot Localization from 3D Point Clouds[J]. Information Technology Journal, 2010, 9(1): 27-33.
[19] 王永波, 杨化超, 刘燕华, 等. 线状特征约束下基于四元数描述的LiDAR点云配准方法[J]. 武汉大学学报(信息科学版), 2013, 38(9): 1057-1062. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201309012.htm Wang Yongbo, Yang Huachao, Liu Yanhua, et al. Linear-Feature-Constrained Registration of LiDAR Point Cloud via Quaternion[J]. Geomatics and Information Science of Wuhan University, 2013, 38(9): 1057-1062. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201309012.htm
[20] 龚辉, 姜挺, 江刚武, 等. 利用单位对偶四元数进行航空影像区域网平差解算[J]. 武汉大学学报(信息科学版), 2012, 37(2): 154-159. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201202008.htm Gong Hui, Jiang Ting, Jiang Gangwu, et al. Bundle Block Adjustment of Aerial Imagery Based on Unit Dual Quaternion[J]. Geomatics and Information Science of Wuhan University, 2012, 37(2): 154-159. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201202008.htm
[21] 姬亭, 盛庆红, 王惠南, 等. 对偶四元数单片空间后方交会算法[J]. 中国图象图形学报, 2012, 17(4): 494-503. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGTB201204010.htm Ji Ting, Sheng Qinghong, Wang Huinan, et al. Dual Quaternion of Space Resection with Single-Image[J]. Journal of Image and Graphics, 2012, 17(4): 494-503. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGTB201204010.htm
[22] 王永波, 汪云甲, 佘雯雯, 等. 直线特征约束下利用Plücker坐标描述的LiDAR点云无初值配准方法[J]. 武汉大学学报(信息科学版), 2018, 43(9): 1376-1384. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201809014.htm Wang Yongbo, Wang Yunjia, She Wenwen, et al. A Linear Features-Constrained, Plücker Coordinates-Based, Closed-Form Registration Approach to Terrestrial LiDAR Point Clouds[J]. Geomatics and Information Science of Wuhan University, 2018, 43(9): 1376-1384. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201809014.htm
[23] 盛庆红, 柳建锋, 虞梦昕, 等. 对偶四元数近景影像空中三角测量法[J]. 测绘学报, 2015, 44(5): 503-509. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB201505007.htm Sheng Qinghong, Liu Jianfeng, Yu Mengxin, et al. Aerial Triangulation Close-Range Images with Dual Quaternion[J]. Acta Geodaetica et Cartographica Sinica, 2015, 44(5): 503-509. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB201505007.htm
[24] Ueda K, Yamashita N. On a Global Complexity Bound of the Levenberg-Marquardt Method[J]. Journal of Optimization Theory and Applications, 2010, 147(3): 443-453.
[25] Chen L, Ma Y. Shamanskii-Like Levenberg-Marquardt Method with a New Line Search for Systems of Nonlinear Equations[J]. Journal of Systems Science and Complexity, 2020, 33(5): 1694-1707.
[26] Dong Z, Yang B, Liu Y, et al. A Novel Binary Shape Context for 3D Local Surface Description[J]. ISPRS Journal of Photogrammetry and Remote Sensing, 2017, 130: 431-452.
[27] Dong Z, Yang B, Liang F, et al. Hierarchical Registration of Unordered TLS Point Clouds Based on Binary Shape Context Descriptor[J]. ISPRS Journal of Photogrammetry and Remote Sensing, 2018, 144: 61-79.
[28] Dong Z, Liang F, Yang B, et al. Registration of Large-Scale Terrestrial Laser Scanner Point Clouds: A Review and Benchmark[J]. ISPRS Journal of Photogrammetry and Remote Sensing, 2020, 163: 327-342.
-
期刊类型引用(6)
1. 王晗,陈怡霖,季钰姣,杜若琳. 基于多模态唇部状态识别的语音导航抗干扰系统. 江苏大学学报(自然科学版). 2025(01): 82-90 .  百度学术
百度学术
2. 何进辛,高井祥,潘诚,王永辉. 移动窗口多普勒平滑伪距增强的GNSS/IMU/单目视觉紧组合定位. 测绘通报. 2025(01): 101-106 .  百度学术
百度学术
3. 王博,武瑞宏,许双安,叶茂,邓川,张卫龙. 低成本亚米级PPP/INS组合导航定位系统研究. 铁道勘察. 2023(02): 8-13+43 .  百度学术
百度学术
4. 祝浩祈,王甫红,张万威,栾梦杰,程雨欣. 一种城市环境下GNSS/MEMS IMU车载实时精密定位方法. 武汉大学学报(信息科学版). 2023(07): 1232-1240 .  百度学术
百度学术
5. 朱东琴,王红茹,岳敬轩. 基于EM估计的多模型车载组合导航算法. 仪器仪表学报. 2022(07): 239-246 .  百度学术
百度学术
6. 李楠,向文豪. 城市环境中无人机作战导航定位研究现状综述. 无人系统技术. 2022(04): 75-87 .  百度学术
百度学术
其他类型引用(9)






 下载:
下载: