圆走航模式下海底控制点对称差分定位模型及分析

马越原; 曾安敏; 许扬胤

doi:10.13203/j.whugis20210087

圆走航模式下海底控制点对称差分定位模型及分析

马越原^{1, 2,},
曾安敏^{3, 4},
许扬胤^{3, 4}

1.
北京跟踪与通信技术研究所,北京,100094
2.
智慧地球实验室,北京,100094
3.
地理信息工程国家重点实验室,陕西西安,710054
4.
西安测绘研究所,陕西西安,710054

基金项目:

国家自然科学基金 41931076

国家自然科学基金 41874016

国家重点研发计划 2020YFB0505801

详细信息

作者简介:
马越原,博士,主要研究方向为海洋大地测量。mayueyuanieu@163.com

计量
- 文章访问数: 108
- HTML全文浏览量: 27
- PDF下载量: 24
出版历程
- 收稿日期: 2022-06-21
- 网络出版日期: 2024-07-16
- 刊出日期: 2024-07-04

Symmetric Difference Positioning Model and Analysis of Sailing Circle Mode of Seafloor Control Points

MA Yueyuan^{1, 2,},
ZENG Anmin^{3, 4},
XU Yangyin^{3, 4}

1.
Beijing Institute of Tracking and Telecommunication Technology, Beijing100094, China
2.
Key Laboratory of Smart Earth, Beijing100094, China
3.
State Key Laboratory of Geo-Information Engineering, Xi'an710054, China
4.
Xi'an Research Institute of Surveying and Mapping, Xi'an710054, China

摘要

摘要:
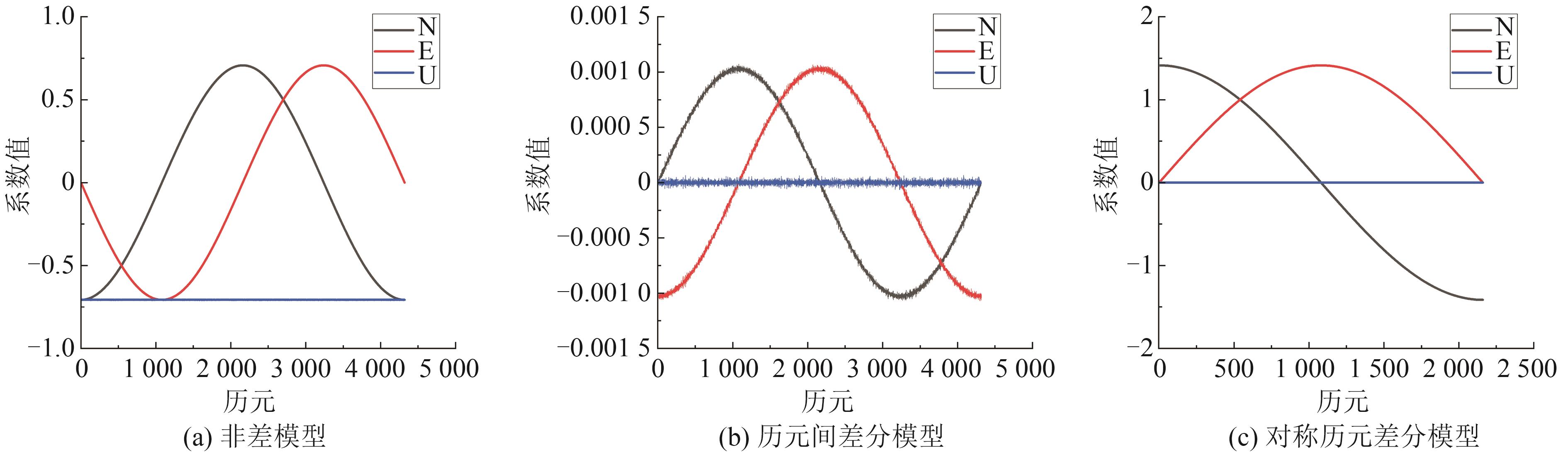
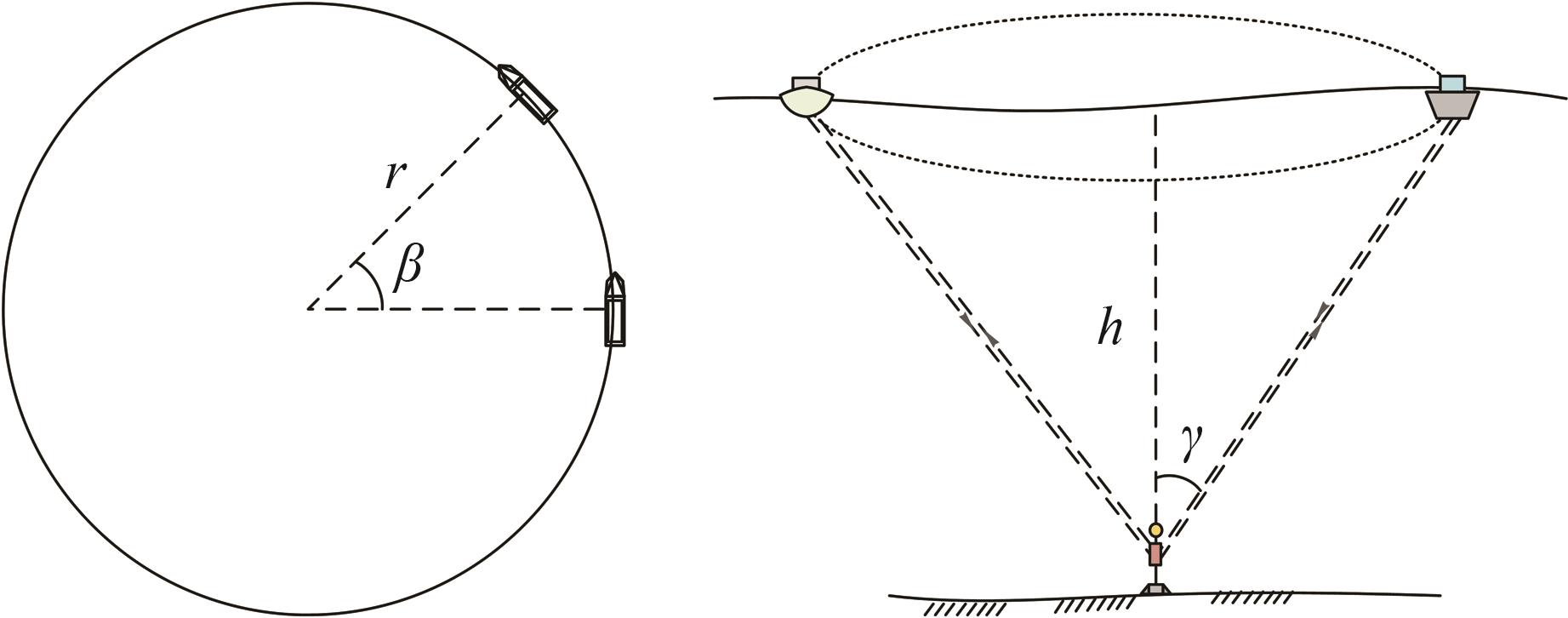
削弱声学定位过程中系统误差对海底控制点位坐标精度的影响是建设高精度海底控制网过程中亟待解决的关键问题。首先,分析了非差和历元间差分两种定位模型；其次,由于声速在海水中的变化受长周期误差和短周期误差的影响,声速变化引起的系统误差同样可认为具有周期性变化的特征,因此,构建了对称差分定位函数模型。模拟仿真和实测数据分析结果表明,当观测时长为系统误差变化周期的偶数倍时,对称差分定位模型可以有效削弱系统误差对定位结果的影响,但是当观测时长不足系统误差变化周期的偶数倍时,该模型将不再适用。
- 声学定位 /
- 海底控制点 /
- 系统误差 /
- 圆走航 /
- 对称差分 /
- 定位模型
Abstract:
Objectives
The critical problem to be solved in the construction of a high-precision seafloor control network is to reduce the influence of systematic errors on the coordinate accuracy of seafloor control points during acoustic positioning.
Methods
First, we analyze two positioning models: Non-difference and difference between epochs. Second, due to the change of sound velocity in seawater is affected by the long period term error and the short period term error, the system error caused by the shift in sound velocity in the measurement also has the characteristics of periodic change.
Results and Conclusions
Based on this premise, a symmetric epoch differential positioning model was constructed. The results of simulation analysis and measured data analysis show that when the observation duration is an even multiple of the system error variation period, the symmetric differential positioning model can effectively reduce the influence of the system error on the positioning results. Still, when the observation duration is not an even multiple of the system error variation period, the model is no longer applicable.
- acoustic positioning /
- seafloor control points /
- systematic error /
- sailing circle mode /
- symmetric difference /
- positioning model
http://ch.whu.edu.cn/cn/article/doi/10.13203/j.whugis20210087

HTML全文

http://ch.whu.edu.cn/cn/article/doi/10.13203/j.whugis20210087

图 1 圆走航示意图

Figure 1. Sailing Circle Diagram

下载: 全尺寸图片幻灯片

图 2 各类系统误差

Figure 2. All Kinds of System Errors

下载: 全尺寸图片幻灯片

图 3 总的系统误差

Figure 3. Total System Errors

下载: 全尺寸图片幻灯片

图 4 设计矩阵

Figure 4. Design Matrix

下载: 全尺寸图片幻灯片

图 5 不同模型计算的残差值（仿真实验1）

Figure 5. Residuals Calculated by Different Models （Simulated Experiment 1）

下载: 全尺寸图片幻灯片

图 6 不同模型计算的残差值（仿真实验2）

Figure 6. Residuals Calculated by Different Models （Simulated Experiment 2）

下载: 全尺寸图片幻灯片

图 7 测量轨迹

Figure 7. Survey Ship Trajectory

下载: 全尺寸图片幻灯片

图 8 设计矩阵(实测算例)

Figure 8. Design Matrix （True Example）

下载: 全尺寸图片幻灯片

图 9 不同模型计算的残差值(实测算例)

Figure 9. Residuals Calculated by Different Models （True Example）

下载: 全尺寸图片幻灯片

表 1 不同定位模型计算的矩阵 $N$ 、 $N^{- 1}$ （仿真实验1）

Table 1 $N$ , $N^{- 1}$ Calculated by Different Positioning Models (Simulated Experiment 1)

定位模型	$N$			$N^{- 1}$
非差	1 080			0.001
	0.000	1 080		0.000	0.001
	0.000	0.000	2 160	0.000	0.000	0.001
历元间差分	1 080			0.001
	－0.000	1 080		0.000	0.001
	－0.000	0.001	0.000	0.491	－1.225	1.337×10⁶
对称历元差分	1 080			0.001
	－0.001	1 080		0.000	0.001
	0.000	0.001	0.000	－0.533	1.831	2.647×10⁶

下载: 导出CSV

表 2 不同定位模型的残差统计（仿真实验1）/m

Table 2 Residual Statistics by Different Positioning Models (Simulated Experiment 1)/m

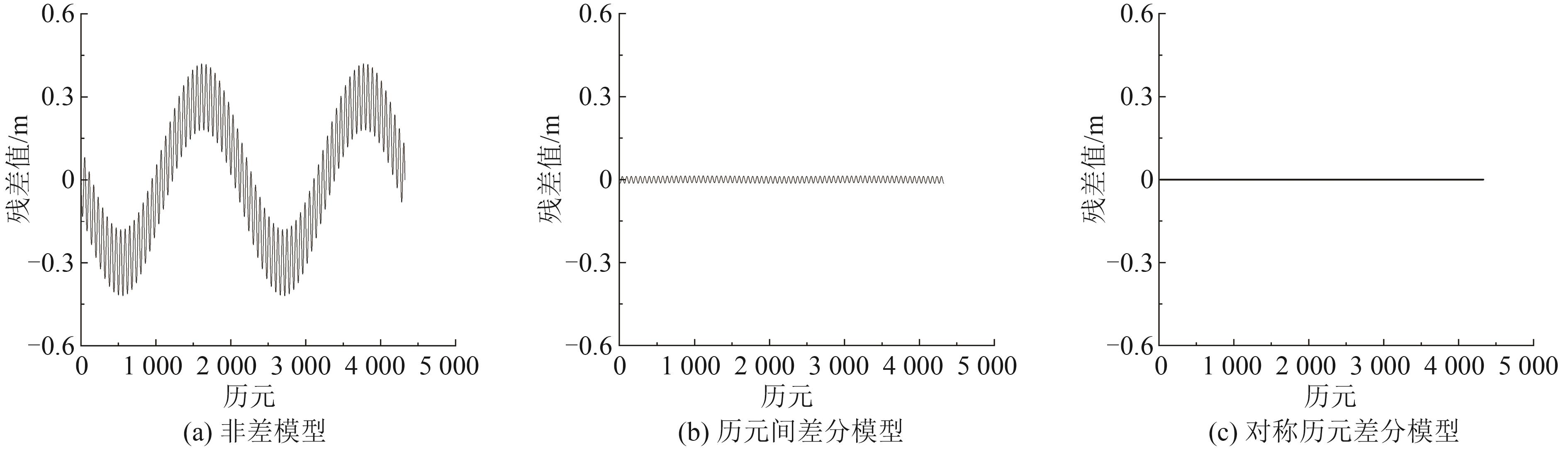
定位模型	最大值	最小值	RMS
非差	0.420	－0.420	0.229
历元间差分	0.015	－0.015	0.009
对称历元差分	－0.000	0.000	0.000

下载: 导出CSV

表 3 不同定位模型的定位精度统计（仿真实验1）/m

Table 3 Statistics of Positioning Accuracy of Different Positioning Models (Simulated Experiment 1)/m

定位模型	$m_{0}$	$m_{e}$	$m_{n}$	$m_{u}$
非差	0.229	0.007	0.007	0.005
历元间差分	0.229	0.007	0.007	262.4
对称历元差分	0.000	0.000	0.000	0.000

下载: 导出CSV

表 4 不同定位模型计算的矩阵 $N$ 、 $N^{- 1}$ （仿真实验2）

Table 4 $N$ , $N^{- 1}$ Calculated by Different Positioning Models （Simulated Experiment 2）

定位模型	$N$			$N^{- 1}$
非差	180.0			0.006
	0.000	180.0		0.000	0.006
	0.000	0.000	360.0	0.000	0.000	0.003
历元间差分	180.0			0.006
	0.000	180.0		0.000	0.006
	－0.000	0.000	0.000	10.68	－2.588	8.107×10⁶
对称历元差分	180.0			0.006
	0.000	180.0		0.000	0.006
	0.000	0.000	0.000	－14.60	－3.690	1.729×10⁷

下载: 导出CSV

表 5 不同定位模型的残差统计(仿真实验2)/m

Table 5 Residual Statistics by Different Positioning Models (Simulated Experiment 2)/m

定位模型	最大值	最小值	RMS
非差	0.212	－0.196	0.094
历元间差分	0.013	－0.013	0.009
对称历元差分	0.044	－0.152	0.057

下载: 导出CSV

表 6 不同定位模型的定位精度统计(仿真实验2)/m

Table 6 Statistics of Positioning Accuracy of Different Positioning Models (Simulated Experiment 2)/m

定位模型	$m_{0}$	$m_{e}$	$m_{n}$	$m_{u}$
非差	0.094	0.007	0.007	0.007
历元间差分	0.094	0.007	0.007	226.7
对称历元差分	0.041	0.003	0.003	169.2

下载: 导出CSV

表 7 不同定位模型计算的矩阵 $N$ 、 $N^{- 1}$ （实测算例）

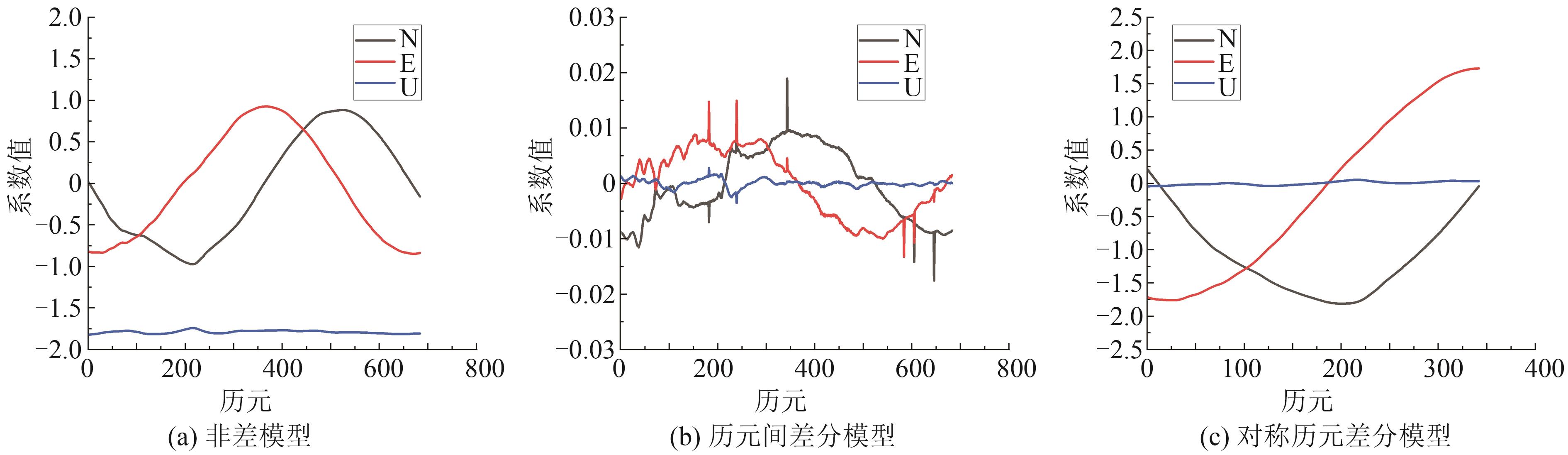
Table 7 $N$ , $N^{- 1}$ by Different Positioning Models （True Example）

定位模型	$N$			$N^{- 1}$
非差	272.6			0.004
	21.03	270.4		0.000	0.004
	78.53	40.49	2 193	0.000	0.000	0.000
历元间差分	268.9			0.004
	19.67	269.0		－0.001	0.006
	－0.663	4.445	0.203	0.029	－0.133	7.941
对称历元差分	266.3			0.004
	19.75	267.3		－0.002	0.009
	－0.703	4.332	0.124	0.078	－0.332	20.11

下载: 导出CSV

表 8 不同定位模型的残差统计（实测算例）/m

Table 8 Residual Statistical by Different Positioning Models （True Example）/m

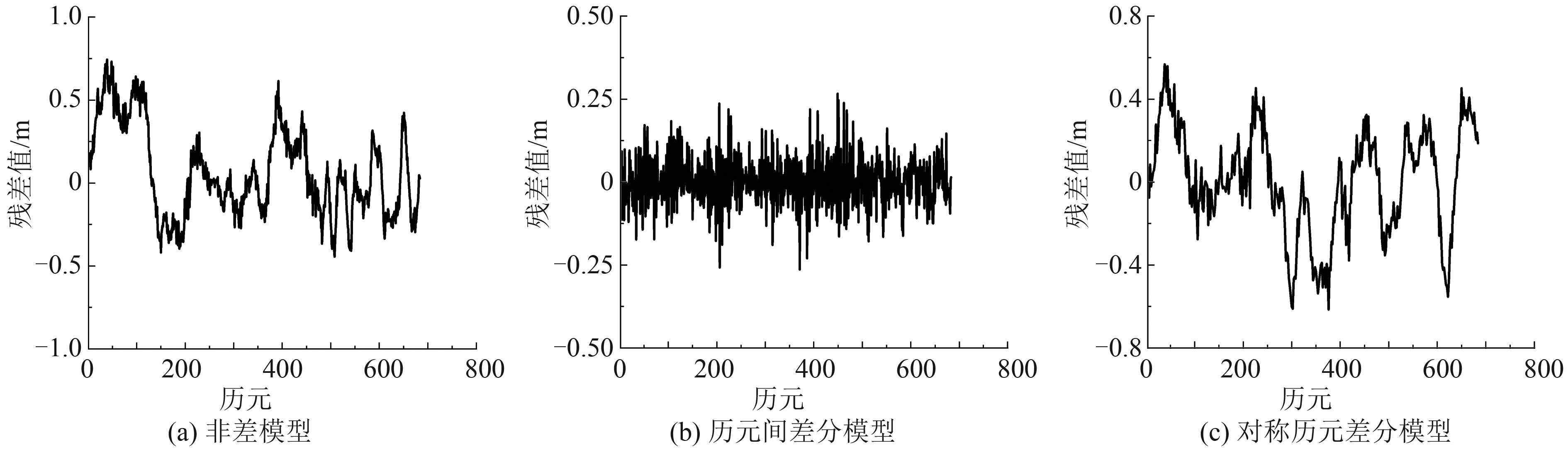
定位模型	最大值	最小值	RMS
非差	0.645	－0.540	0.264
历元间差分	0.274	－0.264	0.075
对称历元差分	0.538	－0.561	0.249

下载: 导出CSV

表 9 不同定位模型的定位精度统计（实测算例）/m

Table 9 Statistical of Positioning Accuracy of Different Positioning Models （True Example）/m

定位模型	$m_{0}$	$m_{e}$	$m_{n}$	$m_{u}$
非差	0.264	0.016	0.016	0.006
历元间差分	0.251	0.016	0.019	0.706
对称历元差分	0.177	0.011	0.017	0.792

下载: 导出CSV

参考文献(33)

[1]	宁津生, 王华, 程鹏飞, 等. 2000国家大地坐标系框架体系建设及其进展[J]. 武汉大学学报（信息科学版）, 2015, 40（5）： 569-573. Ning Jinsheng, Wang Hua, Cheng Pengfei, et al. System Construction and Its Progress of China Geodetic Coordinate System 2000[J]. Geomatics and Information Science of Wuhan University, 2015, 40（5）： 569-573.
[2]	杨元喜. 2000中国大地坐标系[J]. 科学通报, 2009, 54（16）： 2271-2276. Yang Yuanxi. 2000 China Geodetic Coordinate System[J]. Chinese Science Bulletin, 2009, 54（16）： 2271-2276.
[3]	吴富梅, 刘光明, 魏子卿. 利用局域欧拉矢量法建立CGCS2000速度场模型[J]. 武汉大学学报（信息科学版）, 2012, 37（4）： 432-435. Wu Fumei, Liu Guangming, Wei Ziqing. Velocity Field Model of CGCS2000 Based on Euler Vector of Local Area[J]. Geomatics and Information Science of Wuhan University, 2012, 37（4）： 432-435.
[4]	Yang Y, Qin X. Resilient Observation Models for Seafloor Geodetic Positioning [J]. Journal of Geodesy, 2021, 95（7）： 1-13.
[5]	刘经南, 赵建虎, 马金叶. 通导遥一体化深远海PNT基准及服务网络构想[J]. 武汉大学学报（信息科学版）, 2022, 47（10）： 1523-1534. Liu Jingnan,Zhao Jianhu,Ma Jinye.Concept of Constructing the Underwater PNT Network with the Abilities of Communication,Navigation and Remote Sensing in the Deep Sea[J].Geomatics and Information Scien‑ce of Wuhan University, 2022, 47（10）： 1523-1534.
[6]	杨元喜, 刘焱雄, 孙大军, 等. 海底大地基准网建设及其关键技术[J]. 中国科学：地球科学, 2020, 50（7）： 936-945. Yang Yuanxi, Liu Yanxiong, Sun Dajun, et al.Seafloor Geodetic Network Establishment and Key Technologies[J]. Scientia Sinica （Terrae）, 2020, 50（7）： 936-945.
[7]	刘经南,陈冠旭, 赵建虎,等. 海洋时空基准网的进展与趋势[J]. 武汉大学学报（信息科学版）, 2019, 44（1）： 17-37. Liu Jingnan, Chen Guanxu, Zhao Jianhu, et al. Development and Trends of Marine Space-Time Frame Network [J]. Geomatics and Information Science of Wuhan University, 2019, 44（1）： 17-37.
[8]	李林阳, 吕志平, 崔阳. 海底大地测量控制网研究进展综述[J]. 测绘通报, 2018 （1）： 8-13. Li Linyang, Zhiping Lü, Cui Yang. Summary of the Research Progress of Seafloor Geodetic Control Network[J]. Bulletin of Surveying and Mapping, 2018（1）： 8-13.
[9]	Yamada T, Ando M, Tadokoro K, et al. Error Evaluation in Acoustic Positioning of a Single Transponder for Seafloor Crustal Deformation Measurements[J]. Earth Planets Space, 2002, 54： 871-881.
[10]	Osada Y, Fujimoto H, Miura S, et al. Estimation and Correction for the Effect of Sound Velocity Variation on GPS/Acoustic Seafloor Positioning： An Experiment off Hawaii Island[J]. Earth Planets & Space, 2003, 55（10）： 17-20.
[11]	Chen Guanxu, Liu Yang, Liu Yanxiong, et al. Adjustiment of Transceiver Lever Arm Offset and Sound Speed Bias for GNSS-Acoustic Positioning[J]. Remote Sensing, 2019, 31： 693-707.
[12]	马越原,杨元喜, 曾安敏,等. 顾及偏移参数先验信息的海底控制点贝叶斯估计模型[J]. 武汉大学学报信息科学版, 2023, 48（9）： 1465-1472. Ma Yueyuan, Yang Yuanxi, Zeng Anmin, et al. Bayesian Estimation Model of Seafloor Control Points Based on Prior Information of Offset Parameters[J].Geomatics and Information Science of Wuhan University, 2023, 48（9）： 1465-1472.
[13]	Mcintyre M C. Design and Testing of a seafloor Geodetic System[D]. San Diegom, CA： University of California, 1989.
[14]	马越原,杨元喜,曾安敏.GNSS-A直线测量模式及航迹组合优化分析[J].地球物理学报,2022,65（10）：3797-3808. Ma Yueyuan, Yang Yuanxi, Zeng Anmin. GNSS-A Straight-Line Survey Pattern and Trajectory Combination Optimization Analysis[J]. Chinese Journal of Geophysics2022, 65 （10）： 3797–3808.
[15]	Fujita M, Ishikawa T, Mochizuki M, et al. GPS/Acoustic Seafloor Geodetic Observation： Method of Data Analysis and its Application [J]. Earth Planets Space, 2006, 58： 265–275.
[16]	吴永亭. LBL精密定位理论方法研究及软件系统研制[D]. 武汉：武汉大学,2013. Wu Yongting. Study on Theory and Method of Precise LBL Positioning and Development of Positioning Software System [D]. Wuhan： Wuhan University, 2013.
[17]	薛树强, 杨元喜. 广义反距离加权空间推估法[J]. 武汉大学学报（信息科学版）, 2013, 38（12）： 1435-1439. Xue Shuqiang, Yang Yuanxi. Generalized Inverse Distance Weighting Methodfor Spatial Interpolation[J]. Geomatics and Information Science of Wuhan University, 2013, 38（12）： 1435-1439.
[18]	Zhao J, Zhou Y, Zhang H, et al. A New Method for Absolute Datum Transfer in Seafloor Control Network Measurement [J]. Journal of Marine Science and Technology, 2016, 21, 216-226.
[19]	Chen Guanxu, Liu Yang, Liu Yanxiong,et al. Improving GNSS-Acoustic Positioning by Optimizing the Ship's Track Lines and Observation Combinations[J]. Journal of Geodesy, 2021, 94（6）： 61.
[20]	孙文舟, 曾安敏, 赵翔. 顾及参考深度误差的海底控制点迭代算法[J]. 武汉大学学报（信息科学版）, 2024, 49（2）： 324-330. Sun Wenzhou, Zeng Anmin, Zhao Xiang. Iterative Algorithm of Seafloor Control Points Positioning Taking Reference Depth Error into Account[J]. Geomatics and Information Science of Wuhan University, 2024, 49（2）： 324-330.
[21]	Xu P L, Ando M, Tadokoro K．Precise Three-Dimensional Seafloor Geodetic Deformation Measurements Using Difference Techniques [J]．Earth, Planets and Space, 2005, 57（9）： 795-808.
[22]	Wang J, Xu T, Nie W, et al. The Construction of Sound Speed Field Based on Back Propagation Neural Network in the Global Ocean[J]. Marine Geodesy, 2020, 43（6）： 1-14.
[23]	王燕, 林旺生, 梁国龙,等. 声线弯曲对同步水声定位系统影响分析及修正[J], 声学技术, 2009, 28（5）： 123-124. Wang Yan, Lin Wangsheng, Liang Guolong, et al. The Influence and Revision of Ray Bending in Synchronous Underwater Acoustic Positioning System[J]. Technical Acoustics, 2009, 28（5）： 123-124.
[24]	李圣雪,王振杰,聂志喜,等.一种适用于深海长基线定位的自适应分层声线跟踪法[J]. 海洋通报, 2015, 34（5）： 491-498. Li Shengxue, Wang Zhenjie, Nie Zhixi, et al. A Self-adapting Division Ray-Tracing Method in the Long Baseline Acoustic Positioning [J].Marine Scien‑ce Bulletin, 2015, 34（5）： 491-498.
[25]	王振杰, 李圣雪, 聂志喜,等. 水声定位中一种大入射角声线跟踪方法[J]. 武汉大学学报（信息科学版）, 2016, 41（10）： 1404-1408 Wang Zhenjie, Li Shengxue, Nie Zhixi, et al. A Large Incidence Angle Ray-Tracing Method for Underwater Acoustic Positioning[J]. Geomatics and Information Science of Wuhan University, 2016, 41（10）： 1404-1408.
[26]	阳凡林, 辛明真, 刘经南, 等. 超短基线多信标约束的深海动态定位方法探讨[J]. 武汉大学学报（信息科学版）, 2023, 48（11）： 1876-1883. Yang Fanlin, Xin Mingzhen, Liu Jingnan,et al. Discussion of Deep-Sea Dynamic Positioning Methods with Ultra-short Baseline Multi-beacon Constraint[J]. Geomatics and Information Science of Wuhan University, 2023, 48（11）： 1876-1883.
[27]	Yang F L, Lu Xiu S, Li Jia B, et al．Precise Positioning of Underwater Static Objects Without Sound Speed Profile [J]．Marine Geodesy, 2011, 34（2）： 138-151.
[28]	孙文舟, 殷晓冬, 曾安敏, 等. 海底控制点定位初始入射角迭代计算方法的比较研究[J]. 武汉大学学报（信息科学版）, 2020, 45（10）： 1588-1593. Sun Wenzhou, Yin Xiaodong, Zeng Anmin, et al. Calculating the Starting Incidence Angle by Iterative Method for Positioning Seafloor Control Points[J]. Geomatics and Information Science of Wuhan University, 2020, 45（10）： 1588-1593.
[29]	Spiess F N, Chadwell C D, Hildebrand J A, et al. Precise GPS/Acoustic Positioning of Seafloor Referen‑ce Points for Tectonic Studies[J].Physics of the Earth & Planetary Interiors,1998,108（2）： 101-112.
[30]	陶本藻.相关平差与分组平差[J].测绘通报,1978（4）：10-14. Tao Benzao. Correlation Adjustment and Grouping Adjustment[J]. Bulletin of Surveying and Mapping, 1978（4）： 10-14.
[31]	Ma Y Y, Yang Y X, Li J L, et al. Model with Systematic Error Difference Constraint for Absolute Positioning of Seafloor Geodetic Stations[J].Ocean Engineering, 2024, 297： 117093.
[32]	Valérie B, Bouin M N, Stéphane C, et al. Absolute Seafloor Vertical Positioning Using Combined Pressure Gauge and Kinematic GPS Data[J]. Journal of Geodesy, 2010, 84（1）：65-77.
[33]	赵建虎,邹亚靖,吴永亭,等.深度约束的海底控制网点坐标确定方法[J].哈尔滨工业大学学报,2016,48（10）：137-141. Zhao Jianhu, Zou Yajing, Wu Yongting, et al. Determination of Underwater Control Point Coordinate Based on Constraint of Water Depth[J]. Journal of Harbin Institute of Technology, 2016,48（10）： 137-141.

施引文献(68)

期刊类型引用(32)

1.	冷琴，曹铭. 基于DMSP/OLS与NPP/VIIRS夜间灯光数据的江西省城市扩展研究. 江西科学. 2024(01): 41-45 . 百度学术
2.	马小龙，柳思聪，郑守住. 基于多源遥感数据的鬼城现象分析方法. 北京测绘. 2024(08): 1087-1092 . 百度学术
3.	饶俊，丁海萍，许朋朋，李敏. 基于珞珈一号的上海市建成区提取研究. 江西科学. 2023(02): 261-265 . 百度学术
4.	唐小辉，蔡中祥，刘宏建，樊新刚. 基于NPP-VIIRS夜间灯光数据的产业结构估测——以河南省为例. 河南大学学报(自然科学版). 2023(03): 305-313 . 百度学术
5.	谢志伟，单佳强，孙立双，彭博，刘永睿，黄超. 基于多源遥感图像和复杂网络的城市主城区识别方法. 测绘与空间地理信息. 2023(08): 1-5 . 百度学术
6.	李鹏，黎楚安，杨继梅，李振洪，王厚杰. 典型海岸带城市扩张的多源遥感监测与预测——以青岛市为例. 测绘通报. 2022(01): 105-109 . 百度学术
7.	雷依凡，路春燕，苏颖，黄雨菲. 基于多源夜间灯光数据的城市活力与城市扩张耦合关系研究——以海峡西岸城市群为例. 人文地理. 2022(02): 119-131 . 百度学术
8.	卢世俊. 乌鲁木齐城市空间扩展特征及驱动机制. 武汉大学学报(信息科学版). 2022(07): 1025-1034 . 百度学术
9.	伍亿真，施开放，余柏蒗，李川龙. 利用NPP-VIIRS夜间灯光遥感数据分析城市蔓延对雾霾污染的影响. 武汉大学学报(信息科学版). 2021(05): 777-789 . 百度学术
10.	厉飞，闫庆武，邹雅婧，刘保丽. 利用夜间灯光POI的城市建成区提取精度研究——以珞珈一号01星和NPP/VIIRS夜间灯光影像为例. 武汉大学学报(信息科学版). 2021(06): 825-835 . 百度学术
11.	丛康林，董超，薄鑫宇，韩佳坤. 基于夜光遥感的山东省城市时空格局演化分析. 测绘地理信息. 2021(05): 78-82 . 百度学术
12.	王方民，骆畅，杨朝现，刘勇. 基于兴趣点密度与城市扩张曲线的城市建成区边界识别. 西南大学学报(自然科学版). 2021(12): 115-126 . 百度学术
13.	李欣，葛冠英. 国土空间规划城市体检评估中城区范围划定的济南实践. 北京测绘. 2021(12): 1578-1582 . 百度学术
14.	张周. 基于DMSP/LANDSAT数据的成渝城市群空间演化特征分析研究. 重庆建筑. 2020(01): 26-31 . 百度学术
15.	罗婧，黄铁兰，吴桂华，朱腾. 基于夜光遥感的粤港澳大湾区城市空间格局变化分析. 智能城市. 2020(01): 1-3 . 百度学术
16.	刘效江，王浩，宁晓刚，余凡，王成港，郝铭辉. 引入路网和建筑物信息的DMSP/OLS数据去饱和方法. 武汉大学学报(信息科学版). 2020(03): 374-383 . 百度学术
17.	郑渊茂，何原荣，王晓荣，高元衡. 夜光遥感数据应用述评与展望. 遥感信息. 2020(03): 1-14 . 百度学术
18.	闫庆武，厉飞，李玲. 基于2种夜间灯光影像亮度修正指数的城市建成区提取研究. 地球信息科学学报. 2020(08): 1714-1724 . 百度学术
19.	孙立双，韩耀辉，谢志伟，李如仁. 采用夜光遥感数据提取城市建成区的邻域极值法. 武汉大学学报(信息科学版). 2020(10): 1619-1625 . 百度学术
20.	何建华，高文凯. UBII:一种协同经济、社会与人类活动的城市边界识别指数. 测绘与空间地理信息. 2019(02): 8-11+15 . 百度学术
21.	白贺庭，马明国，阎然，刘康甯，隽楚涵. 基于夜间灯光数据的重庆市城市扩张研究. 遥感技术与应用. 2019(01): 216-224 . 百度学术
22.	郑洪晗，桂志鹏，栗法，吴华意，武旭芳，张济勇，韩文军. 夜间灯光数据和兴趣点数据结合的建成区提取方法. 地理与地理信息科学. 2019(02): 25-32 . 百度学术
23.	李勇. 山东沿海城市带空间格局及演变特征研究. 山东师范大学学报(自然科学版). 2019(02): 228-235 . 百度学术
24.	王晓茹，唐志光，王建，邓刚，王欣，魏俊锋. 亚洲高山区融雪末期雪线高度空间差异的影响因素分析. 冰川冻土. 2019(05): 1173-1182 . 百度学术
25.	柯文聪，陶超，马进龙，刘莹，邹峥嵘. 基于Landsat与DMSP-OLS的非监督城区提取方法研究. 测绘与空间地理信息. 2018(07): 183-186 . 百度学术
26.	林晓娟，房世峰，徐亚莉，邹宝裕，罗明良. 基于道路交叉点邻域扩张曲线的城市边界识别——以成都、西安、武汉、南京和长沙为例. 地理科学进展. 2018(06): 781-789 . 百度学术
27.	宁晓刚，王浩，张翰超，刘娅菲，庞博，郝铭辉. 2000—2016年中国地级以上城市高精度城区边界遥感提取及时空扩展分析. 武汉大学学报(信息科学版). 2018(12): 1916-1926 . 百度学术
28.	刘建良，赵国忱，倪愿. 基于DMSP/OLS夜间灯光数据进行提取的实证研究——以福州为例. 测绘与空间地理信息. 2017(09): 175-178+181 . 百度学术
29.	杨任飞，罗红霞，周盛，程玉丝，陈婧祎，向海燕，雷茜. 夜间灯光数据驱动的成渝城市群空间形成过程重建及分析. 地球信息科学学报. 2017(05): 653-661 . 百度学术
30.	李治，杨晓梅，孟樊，陈曦，杨丰硕. 城市建成区多源遥感协同提取方法研究. 地球信息科学学报. 2017(11): 1522-1529 . 百度学术
31.	张锋. 夜光遥感数据应用研究综述. 建设科技. 2017(14): 50-52 . 百度学术
32.	赵航，倪杰. 基于夜晚灯光数据的城市建成城区提取与格局分析. 科学家. 2016(12): 5+15 . 百度学术