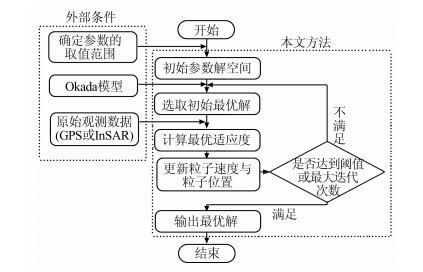
Particle Swarm Optimization Algorithm with Dynamic Inertia Factors for Inversion of Fault Parameters
-
摘要: 采用大地测量观测数据进行地震断层参数反演是大地测量反演的研究热点，也是研究地震发生机制的重点。针对目前在断层参数反演中所用粒子群算法反演精度较低的问题, 分析了地震断层参数反演的非线性特点和基本粒子群算法的特征。考虑到基本粒子群算法在处理高度非线性问题时易陷入局部最优解，且求解过程中局部最优解与全局最优解之间会相互影响，通过分段调整影响粒子速度的惯性因子和影响全局最优解与局部最优解的加速因子，得到了一种适用于地震断层参数反演的分段动态调整参数的粒子群算法，应用于模拟地震与拉奎拉真实地震的断层参数反演。模拟地震实验结果表明，所提算法具有稳定性，且求得的断层倾角、滑动角较多峰值粒子群算法更接近真值。在2009年拉奎拉地震实例中，所提算法求得的断层参数正演后所得形变量与地表观测值的均方根误差为5.2 mm，优于多峰值粒子群算法的6.7 mm。以上结果表明，所提算法获得的断层模型更符合真实的断裂条件，具有一定的实际应用价值。Abstract:Objectives Inversion of seismic fault parameters using geodetic observation data is a hotspot in geodetic inversion, and it is also the focus of studying the mechanism of earthquake occurrence. Aiming at the low accuracy of particle swarm optimization (PSO) currently used in fault parameter inversion, this paper analyzes the nonlinear characteristics of seismic fault parameter inversion and the characteristics of basic PSO. Basic PSO is easy to fall into a local optimal solution in highly nonlinear problems, and the local optimal solution and the global optimal solution may affect each other during the PSO solution process. This paper proposed a new particle swarm algorithm for inversion of fault parameters to solve the local optimization.Methods In this paper, we adopted the strategy of segmentally and dynamically adjusting the parameters, including the inertia factor that affects the particle velocity and the acceleration factors that affect the local and global optimal solutions.Results The proposed algorithm was applied to inverse the fault parameters for the simulation earthquake and the L?Aquila earthquake. The results of the simulation earthquake show that the proposed algorithm is stable, and the inclination and sliding angle obtained by the proposed algorithm are closer to the true value. The results of the L?Aquila earthquake show that the root mean squared error (RMSE) of surface observations and deformation variables obtained by the proposed algorithm is 5.2 mm, which is better than 6.7 mm obtained by multi-peak particle swarm optimization (MPSO).Conclusions The experiment results show that the fault model obtained by the proposed algorithm is more consistent with the true fracture condition, and the proposed algorithm has practical application value.
-
目前，北斗导航卫星系统(BDS)已实现局域覆盖，随着系统建设的不断完善和应用的不断拓展，与之相关的各类数据处理软件的开发成为重要的研究内容。因此，自主开发北斗高精度数据处理软件，成为发展高精度位置服务的迫切任务[1-8]。因北斗导航卫星系统与GPS在星座构造、坐标框架、时间系统、信号频率等方面具有明显差异[9-15]，现有的高精度GPS数据处理软件无法直接处理北斗数据。本文针对北斗高精度数据处理的系统设计、数据流、功能模块及高精度算法实现等进行了研究，研制开发了一套高精度北斗基线解算软件BGO(BeiDou Navigation Satellite System/Global Positioning System Office)，并将其用于高速铁路高精度控制测量建网。通过与商业软件TGO(Trimble Geomatics Office)和TBC(Trimble Business Center)，及高精度科研软件Bernese进行对比测试、性能分析，验证了该软件的正确性和有效性。
1 系统的设计与模块算法的实现
1.1 系统设计与数据流分析
北斗和GPS基线解算软件主要包含北斗基线处理、GPS基线处理及联合基线处理3大模块。各模块间相互独立，但使用相同的数据结构，且数据流基本一致。数据处理流程如图 1所示。
基线解算之前，需选择有效双频观测数据，具体包含低高度角卫星剔除、观测值粗差剔除、星历未获取观测数据剔除等。剔除质量较差的观测数据可通过可视化的方式实现。通过双频数据组合有效消除电离层延迟影响，伪距消电离组合能算出测站精确至10 m内的概略位置，从而形成网络拓扑图，便于用户查看站点的平面分布。基线解算时，北斗与GPS独立系统数据处理算法相同；联合处理需选择统一的坐标和时间框架，随着多余观测数的增加，还需设置合理的模糊度固定限值。基线解算后，进行网平差，应剔除不合格基线，直至平差结果满足要求。
1.2 高精度基线解算算法实现
高精度基线解算利用双差观测量建立误差方程，北斗双差观测量构造如式(1)：
$$ \mathit{\Delta} \nabla L^{{C_m}{C_n}}_{{S_i}{S_j}} = \left( {L^{{C_n}}_{{S_j}} - L^{{C_n}}_{{S_i}}} \right) - \left( {L^{{C_m}}_{{S_j}} - L^{{C_m}}_{{S_i}}} \right) $$ (1) 式中，Δ▽L表示双差观测量；Si和Sj表示任意站点；Cm和Cn表示任意北斗卫星。
依据式(1)构建的双差观测量，建立误差方程，如式(2)：
$$ \left[ \begin{array}{l} \mathit{\Delta} \nabla \boldsymbol{\varPhi} \\ \mathit{\Delta} \nabla \boldsymbol{P} \end{array} \right] = \boldsymbol{BX} + \boldsymbol{A}\mathit{\Delta} \nabla \boldsymbol{N} + \boldsymbol{V} $$ (2) 式中，Δ▽Φ和Δ▽P分别表示卫星载波相位和伪距双差观测量；X表示基线向量；Δ▽N表示双差整周模糊度；B和A为系数阵；V为残差向量。
利用式(2)构建的误差方程，解算基线向量和双差整周模糊度浮点解。利用LAMBAD方法[16, 17]固定双差整周模糊度后去除。再利用载波相位观测值获取高精度基线向量结果。基线解算过程中，主要利用抗差估计的切比雪夫多项式拟合法[18]及MW-GF组合法[19]探测与修复周跳。
对北斗和GPS双系统基线解算，只需将各系统的双差观测量误差方程叠加后平差计算，即可实现双系统联合基线解算。但需注意，星间差分需选择同一系统卫星，否则会引入系统间信号硬件延迟[20]，影响双差整周模糊度的固定。另外，北斗和GPS在时间框架、坐标框架等存在一定差异，双系统联合解算需保证框架的统一。
北斗和GPS时间转换公式如式(3)：
$$ {t_C} = {t_G}-14\;{\rm{s}} $$ (3) 式中，tC和tG分别表示北斗时和GPS时，两者均为原子时，起算原点不同[13]。
北斗和GPS坐标转换公式如式(4)：
$$ \begin{array}{c} \left[ {\begin{array}{*{20}{c}} {{X_C}}\\ {{Y_C}}\\ {{Z_C}} \end{array}} \right] = \left[ {\begin{array}{*{20}{c}} {{X_G}}\\ {{Y_G}}\\ {{Z_G}} \end{array}} \right] + \left[ {\begin{array}{*{20}{c}} {{T_X}}\\ {{T_Y}}\\ {{T_Z}} \end{array}} \right] + \\ \left[ {\begin{array}{*{20}{c}} D&{ - {R_Z}}&{{R_Y}}\\ {{R_Z}}&D&{ - {R_X}}\\ { - {R_Y}}&{{R_X}}&D \end{array}} \right]\left[ {\begin{array}{*{20}{c}} {{X_G}}\\ {{Y_G}}\\ {{Z_G}} \end{array}} \right] \end{array} $$ (4) 式中，北斗坐标(XC，YC，ZC)与GPS坐标(XG，YG，ZG)可通过七参数TX、TY、TZ、D、RX、RY、RZ进行转换。北斗CGCS2000坐标系采用ITRF97框架2000历元的坐标和速度场，当前GPS WGS84坐标和ITRF08基本一致。因此，可利用ITRF97框架2000历元与ITRF08间转换的七参数(ITRF网站公布)实现北斗与GPS坐标框架的统一[11, 12]。
2 BGO数据处理实例与性能测试
2.1 高速铁路CPI控制网基线解算
处理高速铁路CPI控制网时，通过读取观测文件和星历文件，单点定位生成控制网的基线网络拓扑图，如图 2所示。基线解算前，设置相关参数包括卫星截止高度角、误差限差参数、框架、对流层模型、电离层模型、模糊度Ratio值、同步最小观测历元数等。设置完成后，可选择北斗、GPS、联合3种模式进行基线解算。基线解算完成后，软件界面中将显示解算的基线分量及其精度，并可显示残差向量检核基线解算效果。
2.2 BGO、TGO、Bernese软件处理GPS基线结果比较
为了测试BGO解算GPS基线的正确性，将其与TGO和Bernese软件处理结果进行了比较，得到57条GPS基线(基线最长6 667 m，最短446 m)的比较结果，如图 3所示。
![]()
图 3 BGO、TGO、Bernese软件处理GPS基线分量比较Figure 3. Comparing GPS Baseline Components from BGO, TGO and Bernese Software图 3(a)、3(b)分别表示BGO软件与TGO、Bernese软件处理GPS基线分量的差值ΔX、ΔY、ΔZ。图 3(a)中，BGO和TGO有52条基线在X、Y、Z方向的分量差值均在2 cm内，有48条基线各分量差值在mm级。TGO解算少量基线验后方差分量超限，与BGO基线分量差值较大。图 3(b)中，BGO和Bernese有55条基线在X、Y、Z方向的分量差值均在2 cm内，有49条基线各分量差值在mm级。
图 4(a)~4(c)分别表示BGO、TGO、Bernese软件处理GPS基线的内符合精度σX、σY、σZ(BGO、TGO、Bernese软件基线解算精度分别精确至0.1 mm、1 mm和0.1 mm)。整体上，约90%的基线3个软件的解算精度相当。
![]()
图 4 BGO、TGO、Bernese的GPS基线内符合精度比较Figure 4. Comparing GPS Baseline Precision from BGO, TGO and Bernese Software2.3 BGO、TBC软件处理北斗与GPS联合基线结果
为了测试BGO解算北斗与GPS联合基线的性能，本文选用美国Trimble的商业软件TBC与之进行比较。同上57条基线，每条基线观测数据均包含北斗与GPS观测数据。图 5展示了BGO和TBC处理北斗与GPS联合基线分量的差值ΔX、ΔY、ΔZ。图 5可见，98%的基线分量差值分布在mm级，表明BGO软件处理联合基线能达到与TBC软件相当的水平。另外，两者内符合精度绝大部分均在mm级，故图 5中未加以比较。
![]()
图 5 BGO与TBC软件处理北斗与GPS联合基线分量比较Figure 5. Comparing BDS and GPS Combined Baseline Components from BGO and TBC Software由此可知，BGO软件处理GPS基线、北斗与GPS联合基线的内外符合精度能达到TGO、Bernese、TBC相当的水平。因此，以BGO软件处理GPS、北斗与GPS联合基线结果为参考值，分析该软件处理北斗基线结果的正确性和可靠性，如图 6和图 7所示。图 6比较了北斗与GPS、联合基线分量的差值，图 7比较了北斗、GPS、联合基线解算的内符合精度。
![]()
图 6 BGO软件处理北斗与GPS、联合基线分量比较Figure 6. Comparing BDS, GPS and BDS/GPS Combined Baseline Components from BGO Software![]()
图 7 北斗、GPS、联合基线解的内符合精度统计Figure 7. The Statistics of Precision of BDS, GPS and BDS/GPS Combined Baseline Solutions图 6(a)表示BGO软件处理北斗与GPS基线分量的差值ΔX、ΔY、ΔZ，其中有43条基线在X、Y、Z方向上的分量差值Δx、Δy、Δz在2 cm内，有31条基线在X、Y、Z方向上的分量差值在mm级。图 6(b)表示BGO软件处理北斗与联合基线分量的差值，其中有54条基线在X、Y、Z方向上的分量差值在2 cm内，有38条基线在X、Y、Z方向上的分量差值在mm级(图 6中第6条基线北斗为浮点解，各分量差值结果较大，图中置为0)。
图 7中，93%的联合基线在X、Y、Z方向上的分量精度分别优于0.5 mm、1 mm、0.5 mm；约90%的北斗基线和95%的GPS基线在X、Y、Z方向上的分量精度分别优于1 mm、2 mm、1 mm。由北斗、GPS、联合基线3者精度比较可知，在北斗试运行阶段，GPS基线内符合精度略优于北斗，北斗与GPS联合系统基线内符合精度明显高于独立系统。
2.4 BGO基线网平差及其精度分析
BGO具备网平差功能，根据网平差后的基线分量改正数、相对中误差、点位精度等判断基线解算结果的可靠性。对上述解算的北斗、GPS、联合基线分别进行无约束网平差。
北斗、GPS、联合基线无约束网平差的平差改正数δX、δY、δZ绝大部分在±1 cm内，如图 8(a)~8(c)所示。最弱边相对中误差优于5.5 ppm(规范限值)，具体见表 1。据图 8、表 1及《高速铁路工程测量规范》[21]可知，BGO能合理稳定地解算北斗、GPS及联合基线，解算结果中的基线向量改正数、最弱边相对中误差、最弱点点位精度均满足CPI控制测量要求，各系统解算均能精确获得24个CPI控制点坐标。
![]()
图 8 GPS、北斗、联合无约束网平差基线向量改正数Figure 8. Baseline Vector Corrections from GPS, BDS and BDS/GPS Combined Unconstrained Adjustment表 1 GPS、北斗、联合无约束平差结果统计Table 1. The Statistics of GPS, BDS and BDS/GPS Combined Unconstrained Adjustment Results解算模式 独立基线 多余观测数 控制点个数 最弱边相对中误差/ppm 最弱点点位精度/mm GPS 55 66 24 3.6 23.6 北斗 51 57 24 3.1 26.9 联合 57 72 24 3.7 17.9 3 结语
本文系统地研究了北斗与GPS联合基线解算的算法，自主开发了北斗高精度基线解算软件BGO。通过实测高铁CPI控制网的数据处理测试表明：软件能进行高精度地处理北斗与GPS数据, 以及北斗与GPS联合数据处理；GPS基线解算性能与天宝TGO软件相当，能达到与Bernese软件一致的精度；北斗与GPS基线处理能达到与TBC相当的水平。BGO最大的优势在于能对北斗和GPS进行联合解算，从而提高北斗或GPS单系统的基线解算合格率和精度。经高速铁路CPI控制网实例测试，证明该软件处理基线结果可用于高精度北斗和GPS测量控制网的数据处理。
致谢: 本文所用的MPSO算法为冯万鹏博士、李振洪教授的原始代码，在此表示感谢。 -
![]()
图 2 两种算法的点位偏差与点位偏差的差值
Figure 2. Point Deviations of Two Methods and Differences in Deviations
表 1 基本PSO实验结果
Table 1 Experiment Results of Basic PSO
参数 真值 反演(1) 反演(2) 反演(3) … 反演(50) 50次均值 顶深/km 0.42 0.36 0.42 0.43 … 0.43 0.42 底深/m 14.28 14.53 14.28 14.26 … 14.26 14.31 走向角/(°) 73.51 74.26 73.51 73.52 … 73.53 73.53 倾角/(°) 60.00 60.15 60.00 59.99 … 59.97 60.04 长度/km 54.58 54.48 54.58 54.52 … 54.22 54.55 滑动角/(°) 97.32 97.39 97.32 97.31 … 96.90 97.17 滑动量/m 1.53 1.51 1.53 1.54 … 1.55 1.53 
下载: 导出CSV
表 2 本文算法实验结果
Table 2 Experiment Results of the Proposed Algorithm
参数 真值 反演(1) 反演(2) 反演(3) … 反演(50) 50次均值 顶深/km 0.42 0.42 0.42 0.42 … 0.42 0.42 底深/m 14.28 14.28 14.28 14.28 … 14.27 14.28 走向角/(°) 73.51 74.52 73.50 73.51 … 73.51 73.51 倾角/(°) 60.00 60.00 60.00 60.00 … 60.00 60.00 长度/km 54.58 54.48 54.58 54.56 … 54.58 54.58 滑动角/(°) 97.32 97.32 97.31 97.30 … 97.34 97.31 滑动量/m 1.53 1.53 1.53 1.53 … 1.53 1.53
下载: 导出CSV
表 3 模拟地震实验的参数真值、搜索区间与反演结果
Table 3 Parameter Values, Search Intervals and Inversion Results of the Simulation Earthquake Experiment
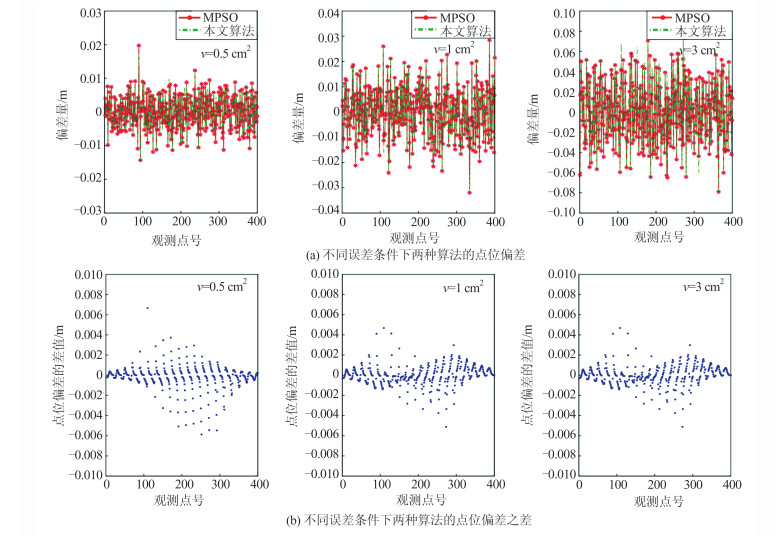
参数 预设真值 搜索区间 反演结果 上区间 下区间 MPSO0.5 本文算法0.5 MPSO1 本文算法1 MPSO3 本文算法3 顶深/km 0.00 0.00 10.00 0.00 0.00 0.10 0.15 0.20 0.23 底深/km 14.70 10.00 30.00 14.72 14.68 14.48 14.54 14.65 14.32 走向角/(°) 70 0 360 70.08 69.96 70.22 70.12 70.63 70.51 倾角/(°) 60 0 90 59.92 60.05 58.75 59.97 56.26 59.39 长度/km 50 30 80 49.89 49.88 50.11 50.25 50.30 50.10 滑动角/(°) 80 -180 180 79.82 80.05 79.58 79.86 81.19 80.97 滑动量/m 1.42 0.00 5.00 1.42 1.42 1.41 1.42 1.47 1.48 X坐标/km 0.00 -5.00 5.00 0.02 0.01 0.13 0.04 0.13 0.53 Y坐标/km 0.00 -5.00 5.00 0.01 -0.01 -0.10 0.03 -0.76 0.35 注: 表中反演结果中的上标0.5、1、3分别代表方差为0.5 cm2、1 cm2、3 cm2
下载: 导出CSV
表 4 拉奎拉地震的搜索区间与反演结果
Table 4 Search Intervals and Inversion Results for the L?Aquila Earthquake
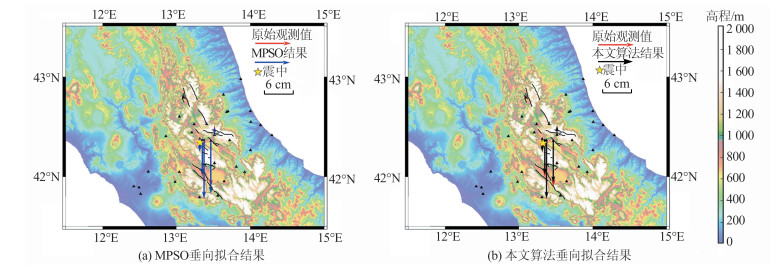
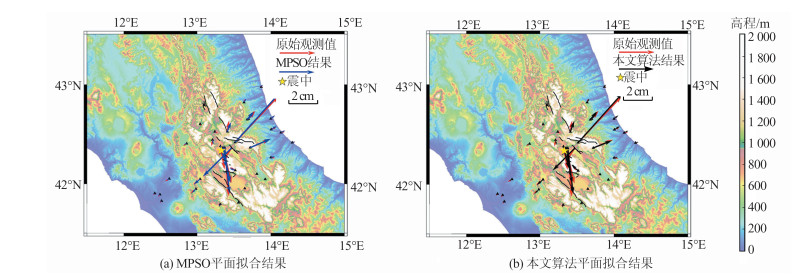
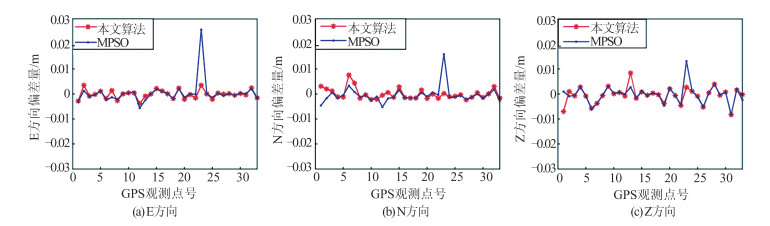
参数 搜索区间 反演结果 上区间 下区间 MPSO 本文算法 Anzidei等[30] 温扬茂等[28] Wang等[29] Atzori等[31] 顶深/km 0.00 5.00 0.02 0.28 — 2.94 2 1.9 底深/km 5.00 20.00 12.38 15.47 — 11.69 10 12.2 走向角/(°) 120.00 160.00 138.66 133.85 140 143.9 144 133 倾角/(°) 30.00 70.00 49.37 50.65 55.3 51.5 46.8 47 长度/km 0.00 30.00 15.46 12.50 13 13.36 13 12.2 滑动角/(°) -140.00 -80.00 -92.33 -98.22 -98 -106.3 -105.1 -103 滑动量/m 0.00 1.50 0.48 0.53 0.49 0.6 0.50 0.56 矩震级/Mw — — 6.34 6.31 6.3 6.32 6.3 6.3
下载: 导出CSV
-
[1] 张永志. 位错理论及其在大地变形研究中的应用[M]. 西安: 西安交通大学出版社, 2011 Zhang Yongzhi. Dislocation Theory and Its Application in the Study of Earth Deformation[M]. Xi?an: Xi?an Jiaotong University Press, 2011
[2] Steketee J A. On Volterra's Dislocation in a Semi-Infinite Elastic Medium[J]. Canadian Journal of Physics, 1958, 36(2): 192-205 doi: 10.1139/p58-024
[3] Okada Y. Surface Deformation to Shear and Tensile Faults in a Halfspace[J]. Bulletin of the Seismological Society of America, 1985, 75(4): 1 135-1 154 http://www.researchgate.net/publication/308358507_surface_deformation_due_to_shear_and_tensile_faults_in_a_halfspace
[4] Okada Y. Inernal Deformation due to Shear and Tensile Fault in a Half Space[J]. Bulletin of the Seismological Society of America, 1992, 92(2): 1 018-1 040 http://ci.nii.ac.jp/naid/10010574975
[5] 冯万鹏, 李振洪. InSAR资料约束下震源参数的PSO混合算法反演策略[J]. 地球物理学进展, 2010, 25(4): 1 189-1 196 https://www.cnki.com.cn/Article/CJFDTOTAL-DQWJ201004008.htm Feng Wanpeng, Li Zhenhong. A Novel Hybrid PSO/Simplex Algorithm for Determining Earthquake Source Parameters Using InSAR Data[J]. Progress in Geophysics, 2010, 25(4): 1 189-1 196 https://www.cnki.com.cn/Article/CJFDTOTAL-DQWJ201004008.htm
[6] Wright T J, Lu Z, Wicks C. Source Model for the Mw 6.7, 23 October 2002, Nenana Mountain Earthquake (Alaska) from InSAR[J]. Geophysical Research Letters, 2003, 30(18): 381-398 doi: 10.1029/2003GL018014
[7] 许才军, 温扬茂. 基于InSAR数据的西藏玛尼Ms 7.9级地震的地壳不均匀性研究[J]. 武汉大学学报·信息科学版, 2008, 33(8): 846-849 http://ch.whu.edu.cn/article/id/1666 Xu Caijun, Wen Yangmao. Nonhomogeneity of the Crust from Ms 7.9 Manyi (Tibet) Earthquake with InSAR Observation[J]. Geomatics and Information Science of Wunan University, 2008, 33(8): 846-849 http://ch.whu.edu.cn/article/id/1666
[8] Jonsson S. Fault Slip Distribution of the 1999 Mw 7.1 Hector Mine, California, Earthquake, Estimated from Satellite Radar and GPS Measurements[J]. Bulletin of the Seismological Society of America, 2002, 92(4): 1 377-1 389 doi: 10.1785/0120000922
[9] Pedersen R, Jonsson S, Árnadóttir T, et al. Fault Slip Distribution of 2 June 2000 Mw 6.5 Earthquakes in South Iceland Estimated from Joint Inversion of InSAR and GPS Measurements[J]. Earth and Planetary Science Letters, 2003, 213(3-4): 487-502 doi: 10.1016/S0012-821X(03)00302-9
[10] Walters R J, Elliott J R, D?Agostino N, et al. The 2009 L?Aquila Earthquake (Central Italy): A Source Mechanism and Implications for Seismic Hazard[J]. Geophysical Research Letters, 2009, 36: L17312 doi: 10.1029/2009GL039337
[11] 易远元, 王家映. 地球物理资料非线性反演方法讲座(十)——粒子群反演方法[J]. 工程地球物理学报, 2009, 6(4): 385-389 https://www.cnki.com.cn/Article/CJFDTOTAL-GCDQ200904000.htm Yi Yuanyuan, Wang Jiaying. Lecture on Non-Linear Inverse Methods in Geophysical Data(10) Particle Swarm Optimization Inversion Method[J]. Chinese Journal of Engineering Geophysics, 2009, 6(4): 385-389 https://www.cnki.com.cn/Article/CJFDTOTAL-GCDQ200904000.htm
[12] Eberhart R, Kennedy J. A New Optimizer Using Particle Swarm Theory[C]// Proceedings of the Sixth International Symposium on Micro Machine and Human Science, Nagoya, Japan, 1995
[13] He S, Prempain E, Wu Q H. An Improved Particle Swarm Optimizer for Mechanical Design Optimization Problems[J]. Engineering Optimization, 2004, 36(5): 585-605 doi: 10.1080/03052150410001704854
[14] Sha D Y, Hsu C Y. A New Particle Swarm Optimization for the Open Shop Scheduling Problem[J]. Computers and Operations Research, 2008, 35(10): 3 243-3 261 doi: 10.1016/j.cor.2007.02.019
[15] Gong M, Cai Q, Chen X, et al. Complex Network Clustering by Multiobjective Discrete Particle Swarm Optimization Based on Decomposition[J]. IEEE Transactions on Evolutionary Computation, 2014, 18(1): 82-97 doi: 10.1109/TEVC.2013.2260862
[16] Chamisi P, Couceiro M S, Martins F M L, et al. Multilevel Image Segmentation Based on Fractional-Order Darwinian Particle Swarm Optimization[J]. IEEE Transactions on Geoscience & Remote Sensing, 2014, 52(5): 2 382-2 394 http://ieeexplore.ieee.org/document/6524014/citations
[17] 冯万鹏, 李振洪, 李春来. 利用InSAR确定2009年4月6日Mw 6.3拉奎拉(Italy)地震最优震源模型[J]. 地球物理学进展, 2010, 25(5): 1 550-1 559 https://www.cnki.com.cn/Article/CJFDTOTAL-DQWJ201005006.htm Feng Wanpeng, Li Zhenhong, Li Chunlai. Optimal Source Parameters of the 6 April 2009 Mw 6.3 L?Aquila, Italy Earthquake from InSAR Observations[J]. Progress in Geophysics, 2010, 25(5): 1 550- 1 559 https://www.cnki.com.cn/Article/CJFDTOTAL-DQWJ201005006.htm
[18] Shi Y, Eberhart R. A Modified Particle Swarm Optimizer[C]. 1998 IEEE International Conference on Evolutionary Computation, Anchorage, AK, USA, 1999
[19] 崔红梅, 朱庆保. 微粒群算法的参数选择及收敛性分析[J]. 计算机工程与应用, 2007, 43(23): 89-91 doi: 10.3321/j.issn:1002-8331.2007.23.028 Cui Hongmei, Zhu Qingbao. Convergence Analysis and Parameter Selection in Particle Swarm Optimization[J]. Computer Engineering and Applications, 2007, 43(23): 89-91 doi: 10.3321/j.issn:1002-8331.2007.23.028
[20] 姜建国, 田旻, 王向前, 等. 采用扰动加速因子的自适应粒子群优化算法[J]. 西安电子科技大学学报(自然科学版), 2012, 39(4): 74-80 doi: 10.3969/j.issn.1001-2400.2012.04.014 Jiang Jianguo, Tian Min, Wang Xiangqian, et al. Adaptive Particle Swarm Optimization via Distur-bing Acceleration Coefficents[J]. Journal of Xidian University (Natural Science), 2012, 39(4): 74-80 doi: 10.3969/j.issn.1001-2400.2012.04.014
[21] Fan H Y. A Modification to Particle Swarm Optimization Algorithm[J]. Engineering Computations, 2002, 19(8): 970-989 doi: 10.1108/02644400210450378
[22] Ratnaweera A, Halgamuge S K, Watson H C. Self-Organizing Hierarchical Particle Swarm Optimizer with Time-Varying Acceleration Coefficients[J]. IEEE Transactions on Evolutionary Computation, 2004, 8(3): 240-255 doi: 10.1109/TEVC.2004.826071
[23] 王晓英, 邢志栋, 黄瑞平. 改进的粒子群优化算法[J]. 计算机应用与软件, 2008, 25(5): 85-86 doi: 10.3969/j.issn.1000-386X.2008.05.034 Wang Xiaoying, Xing Zhidong, Huang Ruiping. An Improved Particle Swarm Optimization Algorithm[J]. Computer Applications and Software, 2008, 25(5): 85-86 doi: 10.3969/j.issn.1000-386X.2008.05.034
[24] Clerc M. The Swarm and the Queen: Towards a Deterministic and Adaptive Particle Swarm Optimization[C]//Proceedings of the 1999 Congress on Evolutionary Computation-CEC99, Washington D C, USA, 1999
[25] 赵英文. 总体最小二乘精度评定方法研究[D]. 抚州: 东华理工大学, 2017 Zhao Yingwen. Research on Overall Least Squares Accuracy Assessment Method[D]. Fuzhou: East China University of Technology, 2017
[26] Boncio P, Pizzi A, Brozzetti F, et al. Coseismic Ground Deformation of the 6 April 2009 L?Aquila Earthquake (Central Italy, Mw 6.3)[J]. Geophysical Research Letters, 2010, 37(6): L17307
[27] Cheloni D, D?Agostino N, D?Anastasio E, et al. Coseismic and Initial Pos-Tseismic Slip of the 2009 Mw 6.3 L?Aquila Earthquake, Italy, from GPS Measurements[J]. Geophysical Journal International, 2010, 181(3): 1 539-1 546 http://adsabs.harvard.edu/abs/2009AGUFM.U13C..03C
[28] 温扬茂, 何平, 许才军, 等. 联合Envisat和ALOS卫星影像确定L?Aquila地震震源机制[J]. 地球物理学报, 2012, 55(1): 53-65 doi: 10.6038/j.issn.0001-5733.2012.01.006 Wen Yangmao, He Ping, Xu Caijun, et al. Source Parameters of the 2009 L?Aquila Earthquake, Italy from Envisat and ALOS Satellite SAR Images[J]. Chinese Journal of Geophysics, 2012, 55(1): 53-65 doi: 10.6038/j.issn.0001-5733.2012.01.006
[29] Wang L Y, Gao H, Feng G C, et al. Source Para-meters and Triggering Links of the Earthquake Sequence in Central Italy from 2009 to 2016 Analyzed with GPS and InSAR Data[J]. Tectonophysics, 2018, 744: 285-295 doi: 10.1016/j.tecto.2018.07.013
[30] Anzidei M, Boschi E, Cannelli V, et al. Coseismic Deformation of the Destructive April 6, 2009 L?Aquila Earthquake (Central Italy) from GPS Data[J]. Geophysical Research Letters, 2009, 36(17): L17307 doi: 10.1029/2009GL039145
[31] Atzori S, Hunstad I, Chini M, et al. Finite Fault Inversion of DInSAR Coseismic Displacement of the 2009 L?Aquila Earthquake (Central Italy)[J]. Geophysical Research Letters, 2009, 36(15): L15305 doi: 10.1029/2009GL039293/abstract
[32] Wells D L, Coppersmith K J. New Empirical Relationships Among Magnitude, Rupture Length, Rupture Area, and Surface Displacement[J]. Bulletin of the Seismological Society of America, 1994, 84(4): 974-1 002 http://gji.oxfordjournals.org/cgi/ijlink?linkType=ABST&journalCode=ssabull&resid=84/4/974
[33] Hanks T C, Kanamori H. A Moment Magnitude Scale[J]. Journal of Geophysical Research: Solid Earth, 1979, 84(B5): 2 348-2 350 doi: 10.1029/JB084iB05p02348
-
期刊类型引用(8)
1. 张莹，任战利，兰华平，祁凯，邢光远，夏岩. 关中盆地新近系蓝田-灞河组热储层物性及渗流特征研究. 地质通报. 2024(05): 712-725 . 
百度学术
2. 吴陈冰洁，罗璐，高楠安，汪新伟，崔梓贤. 关中盆地西安凹陷新近系砂岩热储特征研究. 现代地质. 2024(06): 1571-1584 . 百度学术
3. 张欢，陈应涛，陶威，陈涛，余文鑫，艾卉卉. 不同拉伸方式和速度下的伸展构造砂箱物理模拟实验研究. 西北地质. 2023(02): 327-336 . 百度学术
4. 颜复康，田镇，杨志强，杨兵，梁沛. 厄瓜多尔俯冲区震间闭锁与粘弹性变形研究. 大地测量与地球动力学. 2023(10): 1080-1085 . 百度学术
5. 张莹，任战利，邢光远，祁凯，夏岩. 渭河盆地新近系热储层特征. 地质通报. 2023(11): 1993-2005 . 百度学术
6. 徐斌，张艳. 地下水化学类型分区的GIS空间分析模型. 武汉大学学报(信息科学版). 2019(06): 866-874 . 百度学术
7. 闫俊义，吕睿，赵涛，王莹，白若冰，古云鹤. 关中盆地地壳应力场特征分析. 山西地震. 2019(03): 39-41 . 百度学术
8. 白相东，关成尧，张艳，袁四化，刘晓燕. 渭河盆地断层系统运动学体制分解与探讨. 防灾科技学院学报. 2018(03): 8-16 . 百度学术
其他类型引用(10)
计量
- 文章访问数: 1038
- HTML全文浏览量: 302
- PDF下载量: 92
- 被引次数: 18
