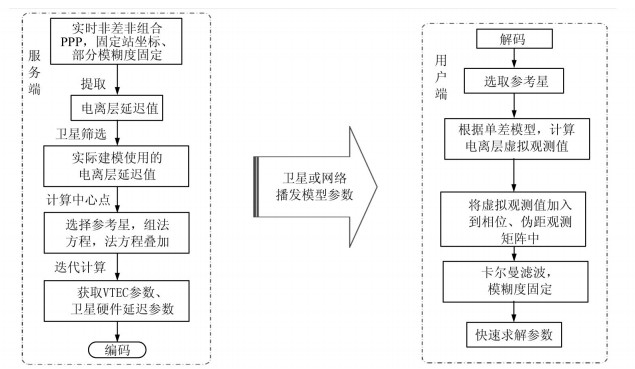
Wide-Area Between-Satellite Single-Difference VTEC Ionospheric Model and Its Assessment for Undifferenced and Uncombined PPP
-
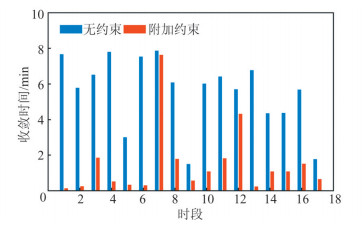
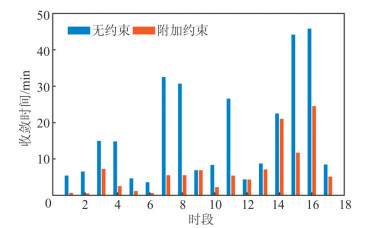
摘要: 高精度电离层修正是非差非组合精密单点定位（precise point positioning, PPP）加速收敛的重要前提。首先基于参考站网台站观测数据, 以非差非组合精密单点定位提取的电离层延迟作为建模数据源，提出一种基于多项式模型的估计天顶电离层延迟参数以及卫星硬件延迟的单差电离层模型。然后开发了服务端和用户端相应软件系统，服务端提取电离层延迟和进行单差建模，并将模型参数播发给用户端作为电离层约束进行非差非组合精密单点定位。最后在欧洲地区通过PPP提取电离层进行拟合实验，结果表明，广域地区GPS和俄罗斯GLONASS（global navigation satellite system）单系统电离层模型内外符合精度分别为1 TECu（total electron content unit）和3 TECu。采用电离层约束的非差非组合动态精密单点定位，统计136个1 h时段的定位结果，发现在附加电离层约束PPP实验中，78个时段（57.35%）收敛时间在5 min内，97个时段（71.32%）在10 min内，122个时段（89.7%）在15 min内，132个时段（97.06%）在25 min内；在无约束PPP实验中，上述收敛时间内结果分别为15个（11.03%）、64个（47.06%）、91个（66.91%）、110个（80.88%）。Abstract:Objectives High-precision ionospheric correction is an important prerequisite for undifferenced and uncombined precise point positioning (PPP) with fast convergence capability. Different from the traditional use of pseudoranges to extract ionospheric information, the ionospheric accuracy obtained by PPP with fixed ambiguity is higher.Methods A wide-area vertical total electron content(VTEC) ionospheric model is developed based on a reference network, where the VTEC of each station is extracted from the undifferenced and uncombined PPP approach. The proposed VTEC model is expressed in the between-satellite single-differenced polynomial function of the sites' latitude and solar hour angle. A data processing procedure and software system are developed for both processing server and user PPP client. In the server part, the VTEC ionospheric delay of the whole network is estimated, the VTEC polynomial function is established and the model parameters are broadcasted to the PPP clients. In the user PPP client, real-time positioning using the broadcasted wide-area VTEC parameters are performed.Results Experiments using the GNSS network in the European region are performed, and results show that the internal RMSE(root mean squared error)and external RMSE of the derived VTEC model is around 3 TECus and 1 TECu for the GLONASS(global navigation satellite system) and GPS, respectively. User kinematic PPP test results using the new VTEC ionospheric model show that, 78 of the whole 136 (57.35%) kinematic undifferenced and uncombined PPP hourly samples converge in 5 minutes, where for 97 samples (71.32%) converge in 10 minutes, 122 samples(89.7%)converge in 15 minutes and 132 samples(97.06%)converge without 25 minutes. In the unconstrained PPP experiment, the results in the above convergence time are 15 (11.03%), 64 (47.06%), 91 (66.91%), and 110 (80.88%), respectively.Conclusions Experiments show that single-difference VTEC ionospheric model has a huge improvement in the accuracy of extracting the ionosphere and shortening the real-time PPP convergence time in a wide-area.
-
目前，北斗导航卫星系统(BDS)已实现局域覆盖，随着系统建设的不断完善和应用的不断拓展，与之相关的各类数据处理软件的开发成为重要的研究内容。因此，自主开发北斗高精度数据处理软件，成为发展高精度位置服务的迫切任务[1-8]。因北斗导航卫星系统与GPS在星座构造、坐标框架、时间系统、信号频率等方面具有明显差异[9-15]，现有的高精度GPS数据处理软件无法直接处理北斗数据。本文针对北斗高精度数据处理的系统设计、数据流、功能模块及高精度算法实现等进行了研究，研制开发了一套高精度北斗基线解算软件BGO(BeiDou Navigation Satellite System/Global Positioning System Office)，并将其用于高速铁路高精度控制测量建网。通过与商业软件TGO(Trimble Geomatics Office)和TBC(Trimble Business Center)，及高精度科研软件Bernese进行对比测试、性能分析，验证了该软件的正确性和有效性。
1 系统的设计与模块算法的实现
1.1 系统设计与数据流分析
北斗和GPS基线解算软件主要包含北斗基线处理、GPS基线处理及联合基线处理3大模块。各模块间相互独立，但使用相同的数据结构，且数据流基本一致。数据处理流程如图 1所示。
基线解算之前，需选择有效双频观测数据，具体包含低高度角卫星剔除、观测值粗差剔除、星历未获取观测数据剔除等。剔除质量较差的观测数据可通过可视化的方式实现。通过双频数据组合有效消除电离层延迟影响，伪距消电离组合能算出测站精确至10 m内的概略位置，从而形成网络拓扑图，便于用户查看站点的平面分布。基线解算时，北斗与GPS独立系统数据处理算法相同；联合处理需选择统一的坐标和时间框架，随着多余观测数的增加，还需设置合理的模糊度固定限值。基线解算后，进行网平差，应剔除不合格基线，直至平差结果满足要求。
1.2 高精度基线解算算法实现
高精度基线解算利用双差观测量建立误差方程，北斗双差观测量构造如式(1)：
$$ \mathit{\Delta} \nabla L^{{C_m}{C_n}}_{{S_i}{S_j}} = \left( {L^{{C_n}}_{{S_j}} - L^{{C_n}}_{{S_i}}} \right) - \left( {L^{{C_m}}_{{S_j}} - L^{{C_m}}_{{S_i}}} \right) $$ (1) 式中，Δ▽L表示双差观测量；Si和Sj表示任意站点；Cm和Cn表示任意北斗卫星。
依据式(1)构建的双差观测量，建立误差方程，如式(2)：
$$ \left[ \begin{array}{l} \mathit{\Delta} \nabla \boldsymbol{\varPhi} \\ \mathit{\Delta} \nabla \boldsymbol{P} \end{array} \right] = \boldsymbol{BX} + \boldsymbol{A}\mathit{\Delta} \nabla \boldsymbol{N} + \boldsymbol{V} $$ (2) 式中，Δ▽Φ和Δ▽P分别表示卫星载波相位和伪距双差观测量；X表示基线向量；Δ▽N表示双差整周模糊度；B和A为系数阵；V为残差向量。
利用式(2)构建的误差方程，解算基线向量和双差整周模糊度浮点解。利用LAMBAD方法[16, 17]固定双差整周模糊度后去除。再利用载波相位观测值获取高精度基线向量结果。基线解算过程中，主要利用抗差估计的切比雪夫多项式拟合法[18]及MW-GF组合法[19]探测与修复周跳。
对北斗和GPS双系统基线解算，只需将各系统的双差观测量误差方程叠加后平差计算，即可实现双系统联合基线解算。但需注意，星间差分需选择同一系统卫星，否则会引入系统间信号硬件延迟[20]，影响双差整周模糊度的固定。另外，北斗和GPS在时间框架、坐标框架等存在一定差异，双系统联合解算需保证框架的统一。
北斗和GPS时间转换公式如式(3)：
$$ {t_C} = {t_G}-14\;{\rm{s}} $$ (3) 式中，tC和tG分别表示北斗时和GPS时，两者均为原子时，起算原点不同[13]。
北斗和GPS坐标转换公式如式(4)：
$$ \begin{array}{c} \left[ {\begin{array}{*{20}{c}} {{X_C}}\\ {{Y_C}}\\ {{Z_C}} \end{array}} \right] = \left[ {\begin{array}{*{20}{c}} {{X_G}}\\ {{Y_G}}\\ {{Z_G}} \end{array}} \right] + \left[ {\begin{array}{*{20}{c}} {{T_X}}\\ {{T_Y}}\\ {{T_Z}} \end{array}} \right] + \\ \left[ {\begin{array}{*{20}{c}} D&{ - {R_Z}}&{{R_Y}}\\ {{R_Z}}&D&{ - {R_X}}\\ { - {R_Y}}&{{R_X}}&D \end{array}} \right]\left[ {\begin{array}{*{20}{c}} {{X_G}}\\ {{Y_G}}\\ {{Z_G}} \end{array}} \right] \end{array} $$ (4) 式中，北斗坐标(XC，YC，ZC)与GPS坐标(XG，YG，ZG)可通过七参数TX、TY、TZ、D、RX、RY、RZ进行转换。北斗CGCS2000坐标系采用ITRF97框架2000历元的坐标和速度场，当前GPS WGS84坐标和ITRF08基本一致。因此，可利用ITRF97框架2000历元与ITRF08间转换的七参数(ITRF网站公布)实现北斗与GPS坐标框架的统一[11, 12]。
2 BGO数据处理实例与性能测试
2.1 高速铁路CPI控制网基线解算
处理高速铁路CPI控制网时，通过读取观测文件和星历文件，单点定位生成控制网的基线网络拓扑图，如图 2所示。基线解算前，设置相关参数包括卫星截止高度角、误差限差参数、框架、对流层模型、电离层模型、模糊度Ratio值、同步最小观测历元数等。设置完成后，可选择北斗、GPS、联合3种模式进行基线解算。基线解算完成后，软件界面中将显示解算的基线分量及其精度，并可显示残差向量检核基线解算效果。
2.2 BGO、TGO、Bernese软件处理GPS基线结果比较
为了测试BGO解算GPS基线的正确性，将其与TGO和Bernese软件处理结果进行了比较，得到57条GPS基线(基线最长6 667 m，最短446 m)的比较结果，如图 3所示。
![]()
图 3 BGO、TGO、Bernese软件处理GPS基线分量比较Figure 3. Comparing GPS Baseline Components from BGO, TGO and Bernese Software图 3(a)、3(b)分别表示BGO软件与TGO、Bernese软件处理GPS基线分量的差值ΔX、ΔY、ΔZ。图 3(a)中，BGO和TGO有52条基线在X、Y、Z方向的分量差值均在2 cm内，有48条基线各分量差值在mm级。TGO解算少量基线验后方差分量超限，与BGO基线分量差值较大。图 3(b)中，BGO和Bernese有55条基线在X、Y、Z方向的分量差值均在2 cm内，有49条基线各分量差值在mm级。
图 4(a)~4(c)分别表示BGO、TGO、Bernese软件处理GPS基线的内符合精度σX、σY、σZ(BGO、TGO、Bernese软件基线解算精度分别精确至0.1 mm、1 mm和0.1 mm)。整体上，约90%的基线3个软件的解算精度相当。
![]()
图 4 BGO、TGO、Bernese的GPS基线内符合精度比较Figure 4. Comparing GPS Baseline Precision from BGO, TGO and Bernese Software2.3 BGO、TBC软件处理北斗与GPS联合基线结果
为了测试BGO解算北斗与GPS联合基线的性能，本文选用美国Trimble的商业软件TBC与之进行比较。同上57条基线，每条基线观测数据均包含北斗与GPS观测数据。图 5展示了BGO和TBC处理北斗与GPS联合基线分量的差值ΔX、ΔY、ΔZ。图 5可见，98%的基线分量差值分布在mm级，表明BGO软件处理联合基线能达到与TBC软件相当的水平。另外，两者内符合精度绝大部分均在mm级，故图 5中未加以比较。
![]()
图 5 BGO与TBC软件处理北斗与GPS联合基线分量比较Figure 5. Comparing BDS and GPS Combined Baseline Components from BGO and TBC Software由此可知，BGO软件处理GPS基线、北斗与GPS联合基线的内外符合精度能达到TGO、Bernese、TBC相当的水平。因此，以BGO软件处理GPS、北斗与GPS联合基线结果为参考值，分析该软件处理北斗基线结果的正确性和可靠性，如图 6和图 7所示。图 6比较了北斗与GPS、联合基线分量的差值，图 7比较了北斗、GPS、联合基线解算的内符合精度。
![]()
图 6 BGO软件处理北斗与GPS、联合基线分量比较Figure 6. Comparing BDS, GPS and BDS/GPS Combined Baseline Components from BGO Software![]()
图 7 北斗、GPS、联合基线解的内符合精度统计Figure 7. The Statistics of Precision of BDS, GPS and BDS/GPS Combined Baseline Solutions图 6(a)表示BGO软件处理北斗与GPS基线分量的差值ΔX、ΔY、ΔZ，其中有43条基线在X、Y、Z方向上的分量差值Δx、Δy、Δz在2 cm内，有31条基线在X、Y、Z方向上的分量差值在mm级。图 6(b)表示BGO软件处理北斗与联合基线分量的差值，其中有54条基线在X、Y、Z方向上的分量差值在2 cm内，有38条基线在X、Y、Z方向上的分量差值在mm级(图 6中第6条基线北斗为浮点解，各分量差值结果较大，图中置为0)。
图 7中，93%的联合基线在X、Y、Z方向上的分量精度分别优于0.5 mm、1 mm、0.5 mm；约90%的北斗基线和95%的GPS基线在X、Y、Z方向上的分量精度分别优于1 mm、2 mm、1 mm。由北斗、GPS、联合基线3者精度比较可知，在北斗试运行阶段，GPS基线内符合精度略优于北斗，北斗与GPS联合系统基线内符合精度明显高于独立系统。
2.4 BGO基线网平差及其精度分析
BGO具备网平差功能，根据网平差后的基线分量改正数、相对中误差、点位精度等判断基线解算结果的可靠性。对上述解算的北斗、GPS、联合基线分别进行无约束网平差。
北斗、GPS、联合基线无约束网平差的平差改正数δX、δY、δZ绝大部分在±1 cm内，如图 8(a)~8(c)所示。最弱边相对中误差优于5.5 ppm(规范限值)，具体见表 1。据图 8、表 1及《高速铁路工程测量规范》[21]可知，BGO能合理稳定地解算北斗、GPS及联合基线，解算结果中的基线向量改正数、最弱边相对中误差、最弱点点位精度均满足CPI控制测量要求，各系统解算均能精确获得24个CPI控制点坐标。
![]()
图 8 GPS、北斗、联合无约束网平差基线向量改正数Figure 8. Baseline Vector Corrections from GPS, BDS and BDS/GPS Combined Unconstrained Adjustment表 1 GPS、北斗、联合无约束平差结果统计Table 1. The Statistics of GPS, BDS and BDS/GPS Combined Unconstrained Adjustment Results解算模式 独立基线 多余观测数 控制点个数 最弱边相对中误差/ppm 最弱点点位精度/mm GPS 55 66 24 3.6 23.6 北斗 51 57 24 3.1 26.9 联合 57 72 24 3.7 17.9 3 结语
本文系统地研究了北斗与GPS联合基线解算的算法，自主开发了北斗高精度基线解算软件BGO。通过实测高铁CPI控制网的数据处理测试表明：软件能进行高精度地处理北斗与GPS数据, 以及北斗与GPS联合数据处理；GPS基线解算性能与天宝TGO软件相当，能达到与Bernese软件一致的精度；北斗与GPS基线处理能达到与TBC相当的水平。BGO最大的优势在于能对北斗和GPS进行联合解算，从而提高北斗或GPS单系统的基线解算合格率和精度。经高速铁路CPI控制网实例测试，证明该软件处理基线结果可用于高精度北斗和GPS测量控制网的数据处理。
-
![]()
图 3 广域星间单差电离层模型内符合RMSE
Figure 3. Internal RMSE of Wide-Area Between-Satellite Single-Difference VTEC Ionospheric Model
![]()
图 4 广域星间单差电离层模型外符合RMSE
Figure 4. External RMSE of Wide-Area Between-Satellite Single-Differenced VTEC Ionospheric Model
![]()
图 5 GRAZ站两个时段附加电离层约束与无电离层约束获取的动态坐标与真值差值序列
Figure 5. Comparison of Difference Time Series of Kinematic Coordinates with and Without Ionosphere Constraint of Station GRAZ
![]()
图 6 GRAZ测站附加电离层约束与无电离层约束动态PPP的收敛时间
Figure 6. Comparison of Convergence Time with and Without Ionosphere Constraint of Station GRAZ
![]()
图 7 HUGE测站电离层约束与无电离层约束动态PPP的收敛时间
Figure 7. Comparison of Convergence Time with and Without Ionosphere Constraint of Station HUGE
表 1 收敛时间统计表
Table 1 Statistics of Convergence Time
收敛时间指标 附加电离层约束 无约束 5 min内收敛 78（57.35%） 15（11.03%） 10 min内收敛 97（71.32%） 64（47.06%） 15 min内收敛 122（89.7%） 91（66.91%) 25 min内收敛 132（97.06%） 110（80.88%) 25 min以上收敛 4（2.94%） 26（19.12%） 
下载: 导出CSV
表 2 收敛时间提升百分比统计表
Table 2 Statistics of Convergence TimeImprovement Effect
收敛效果指标 个数 占总量（136个）百分比/% 有收敛提升 124 91.18 提升20%以上 113 83.09 提升50%以上 95 69.85
下载: 导出CSV
-
[1] 张小红, 李星星, 郭斐, 等. GPS单频精密单点定位软件实现与精度分析[J]. 武汉大学学报·信息科学版, 2008, 33(8): 783-787 http://ch.whu.edu.cn/article/id/1678 Zhang Xiaohong, Li Xingxing, Guo Fei, et al. Realization and Precision Analysis of Single-Frequency Precise Point Positioning Software[J]. Geomatics and Information Science of Wuhan University, 2008, 33(8): 783-787 http://ch.whu.edu.cn/article/id/1678
[2] 房成贺, 陈俊平, 兰孝奇, 等. 联合BDS/GPS的北斗广域差分实时电离层延迟格网改正方法研究[J]. 大地测量与地球动力学, 2019, 39(2): 169-177 https://www.cnki.com.cn/Article/CJFDTOTAL-DKXB201902013.htm Fang Chenghe, Chen Junping, Lan Xiaoqi, et al. Research on BeiDou Wide Area Real-Time Differential Ionospheric Delay Grid Correction Based on BDS/GPS Observations[J]. Journal of Geodesy and Geodynamics, 2019, 39(2): 169-177 https://www.cnki.com.cn/Article/CJFDTOTAL-DKXB201902013.htm
[3] Li Xingxing, Ge Maorong, Zhang Hongping, et al. A Method for Improving Uncalibrated Phase Delay Estimation and Ambiguity-Fixing in Real-Time Precise Point Positioning[J]. Journal of Geodesy, 2013, 87 (5): 405-416 doi: 10.1007/s00190-013-0611-x
[4] 刘长建. GNSS电离层建模方法与质量控制研究[D]. 河南: 信息工程大学, 2011 Liu Changjian. Study on Modeling Method and Model Quality Control of Ionosphere Based on GNSS[D].Henan: Information Engineering University, 2011
[5] 袁运斌. 基于GPS的电离层监测及延迟改正理论与方法的研究[D]. 武汉: 中国科学院研究生院测量与地球物理研究所, 2002 Yuan Yunbin. Study on Theories and Methods of Correcting Ionospheric Delay and Monitoring Ionosphere Based on GPS[D].Wuhan: Institute of Geodesy and Geophysics, Chinese Academy of Sciences, 2002
[6] Schaer S. Mapping and Predicting the Earth's Ionosphere Using the Global Positioning System[D]. Switzerland: University of Bern, 1999
[7] Feltrns J, Schaer S. IGS Products for the Ionosphere[J]. Proceeding of the IGS Ac Workshop, 1998: 225-232 http://citeseerx.ist.psu.edu/viewdoc/summary?cid=25102061
[8] Feltens J. Development of a New Three-Dimensional Mathematical Ionosphere Model at European Space Agency/European Space Operations Centre[J]. Space Weather the International Journal of Researh & Applications, 2007, 5(5): 177-180 doi: 10.1029/2006SW000294/full
[9] Ghoddousi-Fard R, Héroux P, Danskin D, et al. Developing a GPS TEC Mapping Service over Canada[J]. Space Weather-The International Journal of Research & Applications, 2011, 9(6): 1-10 doi: 10.1029/2010SW000621
[10] Zhang H, Xu P, Han W, et al. Eliminating Negative VTEC in Global Ionosphere Maps Using Inequality-Constrained Least Squares[J]. Advances in Space Research, 2013, 51(6): 988-1 000 doi: 10.1016/j.asr.2012.06.026
[11] Hernández-Pajares M, Roma-Dollase D, Krankowski A, et al. Methodology and Consistency of Slant and Vertical Assessments for Ionosphere Electron Model[J]. Journal of Geodesy, 2017(2): 1-10 doi: 10.1007%2Fs00190-017-1032-z
[12] 章红平, 韩文慧, 黄玲, 等. 地基GNSS全球电离层延迟建模[J]. 武汉大学学报·信息科学版, 2012, 37(10): 1 186-1 189 http://ch.whu.edu.cn/article/id/355 Zhang Hongping, Han Wenhui, Huang Ling, et al. Modeling Global Ionospheric Delay with IGS Ground-based GNSS Obervations[J]. Geomatics and Information Science of Wuhan University, 2012, 37(10): 1 186-1 189 http://ch.whu.edu.cn/article/id/355
[13] Tang W M, Jin L, Xu K. Proformance Analysis of Ionsphere Moitoring with BeiDou CORS Observational Data[J]. Journal of Navigation, 2014, 67(3): 511-522 doi: 10.1017/S0373463313000854
[14] 张瑞. 多模GNSS实时电离层精化建模及其应用研究[D]. 武汉: 武汉大学, 2013 Zhang Rui. Theory and Method on Multimode GNSS Real-Time Refinement Ionospheric Modeling and Its Application[D]. Wuhan: Wuhan University, 2013
[15] 熊波, 万卫星, 宁百齐, 等. 基于北斗、GLONASS和GPS系统的中低纬度电离层特性联合探测[J]. 地球物理学报, 2014, 57(11): 3 586-3 599 doi: 10.6038/cjg20141112 Xiong Bo, Wan Weixing, Ning Baiqi, et al. Investigation of Mid-Latitude and Low-Latitude Ionosphere Based on BDS, GLONASS and GPS Observations[J]. Chinese Journal of Geophysics, 2014, 57(11): 3 586-3 599 doi: 10.6038/cjg20141112
[16] 张宝成, 欧吉坤, 李子申, 等. 利用精密单点定位求解电离层延迟[J]. 地球物理学报, 2011, 54(4): 950-957 doi: 10.3969/j.issn.0001-5733.2011.04.009 Zhang Baocheng, Ou Jikun, Li Zishen, et al. Determination of Ionospheric Observabels with Precise Point Positioning[J]. Chinese Journal of Geophysics, 2011, 54(4): 950-957 doi: 10.3969/j.issn.0001-5733.2011.04.009
[17] 李玮, 程鹏飞, 秘金钟. 利用非组合精密单点定位提取区域电离层延迟及其精度评定[J]. 武汉大学学报·信息科学版, 2011, 36(10): 1 200-1 203 http://ch.whu.edu.cn/article/id/695 Li Wei, Cheng Pengfei, Bi Jinzhong. Regional Ionosphere Delays'Calibration and Accuracy Assessment Based on Uncombined Precise Point Positioning[J]. Geomatics and Information Science of Wuhan University, 2011, 36(10): 1 200-1 203 http://ch.whu.edu.cn/article/id/695
[18] Rovira-Garcia A, Juan J M, Sanz J, et al. A Worldwide Ionosphere Model for Fast Precise Point Positioning[J]. IEEE Transactions on Geoscience & Remote Sensing, 2015, 53(8): 4 596-4 604 http://ieeexplore.ieee.org/document/7053952/references
[19] 房成贺. GNSS硬件延迟解算及实时电离层延迟改正方法研究[D]. 南京: 河海大学, 2017 Fang Chenghe. Research on GNSS Differential Code Bias and Real-time Ionospheric Delay Correction Calculation[D]. Nanjing: Hohai University, 2017
[20] 章红平, 高周正, 牛小骥, 等. GPS非差非组合精密单点定位算法研究[J]. 武汉大学学报·信息科学版, 2013, 38(12): 1 396-1 399 http://ch.whu.edu.cn/article/id/2833 Zhang Hongping, Gao Zhouzheng, Niu Xiaoji, et al. Research on GPS Precise Point Positioning with Un-differential and Un-combined Observations[J]. Geomatics and Information Science of Wuhan University, 2013, 38(12): 1 396-1 399 http://ch.whu.edu.cn/article/id/2833
[21] Zhou Feng, Dong Danan, Li Pan, et al. Influence of Stochastic Modeling for Intersystem Biases on MultiGNSS Undiferenced and Uncombined Precise Point Positioning[J]. GPS Solutions, 2019, 23(3): 59-65 doi: 10.1007/s10291-019-0852-0
[22] 肖玉钢, 王峥, 陈华, 等. 法方程叠加的多模GNSS双差精密算法[J]. 测绘通报, 2018(10): 88-92 https://www.cnki.com.cn/Article/CJFDTOTAL-CHTB201810020.htm Xiao Yugang, Wang Zheng, Chen Hua, et al. A Precise Multi-GNSS Data Processing Algorithm with Double Difference Observations Based on the Normal Equation Stacking Technique[J]. Bulletin of Surveying and Mapping, 2018(10): 88-92 https://www.cnki.com.cn/Article/CJFDTOTAL-CHTB201810020.htm
[23] 张辉, 郝金明, 刘伟平, 等. 估计接收机差分码偏差的GPS/BDS非组合精密单点定位模型[J]. 武汉大学学报·信息科学版, 2019, 44(4): 495-500, 592 doi: 10.13203/j.whugis20170119 Zhang Hui, Hao Jinming, Liu Weiping, et al. GPS/BDS Precise Point Positioning Model with Receiver DCB Parameters for Raw Observations[J]. Geomatics and Information Science of Wuhan University, 2019, 44(4): 495-500, 592 doi: 10.13203/j.whugis20170119
[24] 付伟, 姚顽强, 陈鹏, 等. 基于多项式的区域电离层建模精度分析与验证[J]. 北京测绘, 2018, 32(10): 1 219-1 225 https://www.cnki.com.cn/Article/CJFDTOTAL-BJCH201810024.htm Fu Wei, Yao Wanqiang, Chen Peng, et al. Polynomial Based Accuracy and Validation of Regional Ionospheric Modeling[J]. Beijing Surveying and Mapping, 2018, 32(10): 1 219-1 225 https://www.cnki.com.cn/Article/CJFDTOTAL-BJCH201810024.htm
[25] Zhou Feng, Dong Danan, Li Weiwei, et al. GAMP: An Open-Source Software of Multi-GNSS Precise Point Positioning Using Undifferenced and Uncombined Observations[J]. GPS Solutions, 2018, 22 (2): 1-10 http://smartsearch.nstl.gov.cn/paper_detail.html?id=136b66966a20eff09edb091a70187768
[26] 周承松, 彭月, 张小红, 等. 精密单点定位收敛时间的确定方法研究[J]. 导航定位学报, 2016, 4(1): 80-87 https://www.cnki.com.cn/Article/CJFDTOTAL-CHWZ201601016.htm Zhou Chengsong, Peng Yue, Zhang Xiaohong, et al. A Study of Method in Determining the Precise Point Positioning Convergence Time[J]. Journal of Navigation and Positioning, 2016, 4(1): 80-87 https://www.cnki.com.cn/Article/CJFDTOTAL-CHWZ201601016.htm
-
期刊类型引用(6)
1. 柯文清 ，陈业滨 ，赵志刚 ，韩德志 ，郭仁忠 . 基于文献计量的新世纪地图可视化研究演变和热点分析. 地理与地理信息科学. 2025(01): 15-23 . 
百度学术
2. 于峰一泽，汤国安，陆鼎阳，林晓芬，胡光辉，沈婕，吴明光. 语言学视角下的地图演化. 地理学报. 2024(01): 171-186 . 百度学术
3. 韩德志，郭仁忠，陈业滨，赵志刚，柯文清. 基于可视化维度理论的泛地图知识推荐方法. 地球信息科学学报. 2024(01): 110-120 . 百度学术
4. 刘强，刘金花，王磊斌，陈鑫，赵志斌，赵晓艳，李英奎. 使用虚拟现实技术提高冰川地貌表达维度的研究. 冰川冻土. 2024(03): 1087-1098 . 百度学术
5. 邓志钢，郭仁忠，陈业滨，马丁，赵志刚，朱维. 面向轨迹可视化的泛地图表达维度关联方法及应用. 测绘通报. 2024(11): 56-60+96 . 百度学术
6. 柯婷，杨品福，任福，李连营，杨晨. 内河航行参考图地图符号形式化表达. 地理空间信息. 2024(12): 102-105 . 百度学术
其他类型引用(4)
计量
- 文章访问数:
- HTML全文浏览量:
- PDF下载量:
- 被引次数: 10
