Temporal and Spatial Analysis of COSMIC Occultation Inversion of Wet Temperature Profile Quality
-
摘要: 为研究COSMIC（constellation observing system for meteorology，ionosphere and climate）掩星反演湿温廓线的质量，需对数据误差特性进行量化研究。首先采用线性插值的方法，以时间窗3 h、水平距离300 km为匹配准则，对0.2~30 km各高度层温度的平均偏差和标准差进行统计分析，研究随海拔高、季节和纬度带变化的温度平均偏差特性。然后采用2016年的全球探空数据集分析全球区域的COSMIC湿温廓线质量，以及北温带COSMIC湿温廓线质量随季节变化的特点，探究不同纬度带地区COSMIC掩星湿温廓线质量随纬度变化的特点。结果表明，全球范围内温度平均偏差为-0.16 K, 掩星数据和探空站资料精度相当；季节变化的统计量F=0.999 6 > 0.05，该因素对COSMIC湿温廓线质量影响不显著；纬度带变化的统计量F=0.024 4 < 0.05，该因素对COSMIC湿温廓线质量有显著影响，尤其是热带地区受水汽影响较大，温度平均偏差处于峰值，偏差高于0.25 K，南温带地区次之。Abstract:Objectives In order to study the wet temperature profile quality of COSMIC (constellation observing system for meteorology, ionosphere and climate) occultation inversion, statistical analysis of data error characteristics is needed.Methods Firstly, the linear interpolation method is used to analyze the average deviation and standard deviation of each layer temperature of 0.2-30 km with time window 3 h and horizontal distance 300 km as matching criteria. The temperature average deviation characteristics treat as a function of altitude, season and latitude are studied. Then, through the use of 2016 annual IGRA (integrated global radiosonde archive) sounding data, the COSMIC wet temperature profile quality in the global region is analyzed, and the characteristics of the COSMIC wet temperature profile quality in the north temperate zone are sorted out. The COSMIC occultation wet temperature profile in different latitude zones is explored. The characteristics of quality are treat as a function of latitude.Results The results show that the average temperature deviation in the global range is -0.16 K, the deviation is lower than 0.2 K, and the COSMIC occultation wet temperature profile quality is similar to the sounding accuracy.The seasonal variation statistic F=0.999 6 > 0.05, this factor has no significant effect on the COSMIC wet temperature profile quality. The statistic of the latitude band change F=0.024 4 < 0.05, which has a significant effect on the COSMIC wet temperature profile quality. In particular, the tropical region is greatly affected by water vapor, the average temperature deviation is at the peak, the deviation is higher than 0.25 K, and the southern temperate zone is second.Conclusions This paper carries out experimental analysis and reveals the error characteristics of GNSS occultation technology detection data, including two different dimensions of time and space, which will promote research in the fields of air weather monitoring and climate change.
-
Keywords:
- COSMIC occultation /
- sounding data /
- wet temperature profile /
- significance test
-
目前，北斗导航卫星系统(BDS)已实现局域覆盖，随着系统建设的不断完善和应用的不断拓展，与之相关的各类数据处理软件的开发成为重要的研究内容。因此，自主开发北斗高精度数据处理软件，成为发展高精度位置服务的迫切任务[1-8]。因北斗导航卫星系统与GPS在星座构造、坐标框架、时间系统、信号频率等方面具有明显差异[9-15]，现有的高精度GPS数据处理软件无法直接处理北斗数据。本文针对北斗高精度数据处理的系统设计、数据流、功能模块及高精度算法实现等进行了研究，研制开发了一套高精度北斗基线解算软件BGO(BeiDou Navigation Satellite System/Global Positioning System Office)，并将其用于高速铁路高精度控制测量建网。通过与商业软件TGO(Trimble Geomatics Office)和TBC(Trimble Business Center)，及高精度科研软件Bernese进行对比测试、性能分析，验证了该软件的正确性和有效性。
1 系统的设计与模块算法的实现
1.1 系统设计与数据流分析
北斗和GPS基线解算软件主要包含北斗基线处理、GPS基线处理及联合基线处理3大模块。各模块间相互独立，但使用相同的数据结构，且数据流基本一致。数据处理流程如图 1所示。
基线解算之前，需选择有效双频观测数据，具体包含低高度角卫星剔除、观测值粗差剔除、星历未获取观测数据剔除等。剔除质量较差的观测数据可通过可视化的方式实现。通过双频数据组合有效消除电离层延迟影响，伪距消电离组合能算出测站精确至10 m内的概略位置，从而形成网络拓扑图，便于用户查看站点的平面分布。基线解算时，北斗与GPS独立系统数据处理算法相同；联合处理需选择统一的坐标和时间框架，随着多余观测数的增加，还需设置合理的模糊度固定限值。基线解算后，进行网平差，应剔除不合格基线，直至平差结果满足要求。
1.2 高精度基线解算算法实现
高精度基线解算利用双差观测量建立误差方程，北斗双差观测量构造如式(1)：
$$ \mathit{\Delta} \nabla L^{{C_m}{C_n}}_{{S_i}{S_j}} = \left( {L^{{C_n}}_{{S_j}} - L^{{C_n}}_{{S_i}}} \right) - \left( {L^{{C_m}}_{{S_j}} - L^{{C_m}}_{{S_i}}} \right) $$ (1) 式中，Δ▽L表示双差观测量；Si和Sj表示任意站点；Cm和Cn表示任意北斗卫星。
依据式(1)构建的双差观测量，建立误差方程，如式(2)：
$$ \left[ \begin{array}{l} \mathit{\Delta} \nabla \boldsymbol{\varPhi} \\ \mathit{\Delta} \nabla \boldsymbol{P} \end{array} \right] = \boldsymbol{BX} + \boldsymbol{A}\mathit{\Delta} \nabla \boldsymbol{N} + \boldsymbol{V} $$ (2) 式中，Δ▽Φ和Δ▽P分别表示卫星载波相位和伪距双差观测量；X表示基线向量；Δ▽N表示双差整周模糊度；B和A为系数阵；V为残差向量。
利用式(2)构建的误差方程，解算基线向量和双差整周模糊度浮点解。利用LAMBAD方法[16, 17]固定双差整周模糊度后去除。再利用载波相位观测值获取高精度基线向量结果。基线解算过程中，主要利用抗差估计的切比雪夫多项式拟合法[18]及MW-GF组合法[19]探测与修复周跳。
对北斗和GPS双系统基线解算，只需将各系统的双差观测量误差方程叠加后平差计算，即可实现双系统联合基线解算。但需注意，星间差分需选择同一系统卫星，否则会引入系统间信号硬件延迟[20]，影响双差整周模糊度的固定。另外，北斗和GPS在时间框架、坐标框架等存在一定差异，双系统联合解算需保证框架的统一。
北斗和GPS时间转换公式如式(3)：
$$ {t_C} = {t_G}-14\;{\rm{s}} $$ (3) 式中，tC和tG分别表示北斗时和GPS时，两者均为原子时，起算原点不同[13]。
北斗和GPS坐标转换公式如式(4)：
$$ \begin{array}{c} \left[ {\begin{array}{*{20}{c}} {{X_C}}\\ {{Y_C}}\\ {{Z_C}} \end{array}} \right] = \left[ {\begin{array}{*{20}{c}} {{X_G}}\\ {{Y_G}}\\ {{Z_G}} \end{array}} \right] + \left[ {\begin{array}{*{20}{c}} {{T_X}}\\ {{T_Y}}\\ {{T_Z}} \end{array}} \right] + \\ \left[ {\begin{array}{*{20}{c}} D&{ - {R_Z}}&{{R_Y}}\\ {{R_Z}}&D&{ - {R_X}}\\ { - {R_Y}}&{{R_X}}&D \end{array}} \right]\left[ {\begin{array}{*{20}{c}} {{X_G}}\\ {{Y_G}}\\ {{Z_G}} \end{array}} \right] \end{array} $$ (4) 式中，北斗坐标(XC，YC，ZC)与GPS坐标(XG，YG，ZG)可通过七参数TX、TY、TZ、D、RX、RY、RZ进行转换。北斗CGCS2000坐标系采用ITRF97框架2000历元的坐标和速度场，当前GPS WGS84坐标和ITRF08基本一致。因此，可利用ITRF97框架2000历元与ITRF08间转换的七参数(ITRF网站公布)实现北斗与GPS坐标框架的统一[11, 12]。
2 BGO数据处理实例与性能测试
2.1 高速铁路CPI控制网基线解算
处理高速铁路CPI控制网时，通过读取观测文件和星历文件，单点定位生成控制网的基线网络拓扑图，如图 2所示。基线解算前，设置相关参数包括卫星截止高度角、误差限差参数、框架、对流层模型、电离层模型、模糊度Ratio值、同步最小观测历元数等。设置完成后，可选择北斗、GPS、联合3种模式进行基线解算。基线解算完成后，软件界面中将显示解算的基线分量及其精度，并可显示残差向量检核基线解算效果。
2.2 BGO、TGO、Bernese软件处理GPS基线结果比较
为了测试BGO解算GPS基线的正确性，将其与TGO和Bernese软件处理结果进行了比较，得到57条GPS基线(基线最长6 667 m，最短446 m)的比较结果，如图 3所示。
![]()
图 3 BGO、TGO、Bernese软件处理GPS基线分量比较Figure 3. Comparing GPS Baseline Components from BGO, TGO and Bernese Software图 3(a)、3(b)分别表示BGO软件与TGO、Bernese软件处理GPS基线分量的差值ΔX、ΔY、ΔZ。图 3(a)中，BGO和TGO有52条基线在X、Y、Z方向的分量差值均在2 cm内，有48条基线各分量差值在mm级。TGO解算少量基线验后方差分量超限，与BGO基线分量差值较大。图 3(b)中，BGO和Bernese有55条基线在X、Y、Z方向的分量差值均在2 cm内，有49条基线各分量差值在mm级。
图 4(a)~4(c)分别表示BGO、TGO、Bernese软件处理GPS基线的内符合精度σX、σY、σZ(BGO、TGO、Bernese软件基线解算精度分别精确至0.1 mm、1 mm和0.1 mm)。整体上，约90%的基线3个软件的解算精度相当。
![]()
图 4 BGO、TGO、Bernese的GPS基线内符合精度比较Figure 4. Comparing GPS Baseline Precision from BGO, TGO and Bernese Software2.3 BGO、TBC软件处理北斗与GPS联合基线结果
为了测试BGO解算北斗与GPS联合基线的性能，本文选用美国Trimble的商业软件TBC与之进行比较。同上57条基线，每条基线观测数据均包含北斗与GPS观测数据。图 5展示了BGO和TBC处理北斗与GPS联合基线分量的差值ΔX、ΔY、ΔZ。图 5可见，98%的基线分量差值分布在mm级，表明BGO软件处理联合基线能达到与TBC软件相当的水平。另外，两者内符合精度绝大部分均在mm级，故图 5中未加以比较。
![]()
图 5 BGO与TBC软件处理北斗与GPS联合基线分量比较Figure 5. Comparing BDS and GPS Combined Baseline Components from BGO and TBC Software由此可知，BGO软件处理GPS基线、北斗与GPS联合基线的内外符合精度能达到TGO、Bernese、TBC相当的水平。因此，以BGO软件处理GPS、北斗与GPS联合基线结果为参考值，分析该软件处理北斗基线结果的正确性和可靠性，如图 6和图 7所示。图 6比较了北斗与GPS、联合基线分量的差值，图 7比较了北斗、GPS、联合基线解算的内符合精度。
![]()
图 6 BGO软件处理北斗与GPS、联合基线分量比较Figure 6. Comparing BDS, GPS and BDS/GPS Combined Baseline Components from BGO Software![]()
图 7 北斗、GPS、联合基线解的内符合精度统计Figure 7. The Statistics of Precision of BDS, GPS and BDS/GPS Combined Baseline Solutions图 6(a)表示BGO软件处理北斗与GPS基线分量的差值ΔX、ΔY、ΔZ，其中有43条基线在X、Y、Z方向上的分量差值Δx、Δy、Δz在2 cm内，有31条基线在X、Y、Z方向上的分量差值在mm级。图 6(b)表示BGO软件处理北斗与联合基线分量的差值，其中有54条基线在X、Y、Z方向上的分量差值在2 cm内，有38条基线在X、Y、Z方向上的分量差值在mm级(图 6中第6条基线北斗为浮点解，各分量差值结果较大，图中置为0)。
图 7中，93%的联合基线在X、Y、Z方向上的分量精度分别优于0.5 mm、1 mm、0.5 mm；约90%的北斗基线和95%的GPS基线在X、Y、Z方向上的分量精度分别优于1 mm、2 mm、1 mm。由北斗、GPS、联合基线3者精度比较可知，在北斗试运行阶段，GPS基线内符合精度略优于北斗，北斗与GPS联合系统基线内符合精度明显高于独立系统。
2.4 BGO基线网平差及其精度分析
BGO具备网平差功能，根据网平差后的基线分量改正数、相对中误差、点位精度等判断基线解算结果的可靠性。对上述解算的北斗、GPS、联合基线分别进行无约束网平差。
北斗、GPS、联合基线无约束网平差的平差改正数δX、δY、δZ绝大部分在±1 cm内，如图 8(a)~8(c)所示。最弱边相对中误差优于5.5 ppm(规范限值)，具体见表 1。据图 8、表 1及《高速铁路工程测量规范》[21]可知，BGO能合理稳定地解算北斗、GPS及联合基线，解算结果中的基线向量改正数、最弱边相对中误差、最弱点点位精度均满足CPI控制测量要求，各系统解算均能精确获得24个CPI控制点坐标。
![]()
图 8 GPS、北斗、联合无约束网平差基线向量改正数Figure 8. Baseline Vector Corrections from GPS, BDS and BDS/GPS Combined Unconstrained Adjustment表 1 GPS、北斗、联合无约束平差结果统计Table 1. The Statistics of GPS, BDS and BDS/GPS Combined Unconstrained Adjustment Results解算模式 独立基线 多余观测数 控制点个数 最弱边相对中误差/ppm 最弱点点位精度/mm GPS 55 66 24 3.6 23.6 北斗 51 57 24 3.1 26.9 联合 57 72 24 3.7 17.9 3 结语
本文系统地研究了北斗与GPS联合基线解算的算法，自主开发了北斗高精度基线解算软件BGO。通过实测高铁CPI控制网的数据处理测试表明：软件能进行高精度地处理北斗与GPS数据, 以及北斗与GPS联合数据处理；GPS基线解算性能与天宝TGO软件相当，能达到与Bernese软件一致的精度；北斗与GPS基线处理能达到与TBC相当的水平。BGO最大的优势在于能对北斗和GPS进行联合解算，从而提高北斗或GPS单系统的基线解算合格率和精度。经高速铁路CPI控制网实例测试，证明该软件处理基线结果可用于高精度北斗和GPS测量控制网的数据处理。
致谢: 感谢CDAAC提供掩星资料和NOAA提供无线电探空数据。 -
![]()
图 1 COSMIC掩星数据和北温带湿温廓线与探空站数据不同季节的温度偏差变化曲线图
Figure 1. COSMIC Occultation Data and Curves of Temperature Deviations of Wet Temperature Profile and Sounding Station Data in Different Seasons
![]()
图 2 不同季节温度偏差的均值及置信区间箱线图
Figure 2. Box Plots of Mean Values and Confidence Intervals of Temperature Deviations for Different Seasons
![]()
图 3 COSMIC掩星数据和不同纬度带地区探空站资料的温度偏差变化曲线
Figure 3. COSMIC Occultation Data and Curves of Temperature Deviation of the Sounding Station Data in Different Latitude Zones
![]()
图 4 不同统计区域均值及置信区间箱线图
Figure 4. Box Plots of Mean Values and Confidence Intervals in Different Statistical Areas
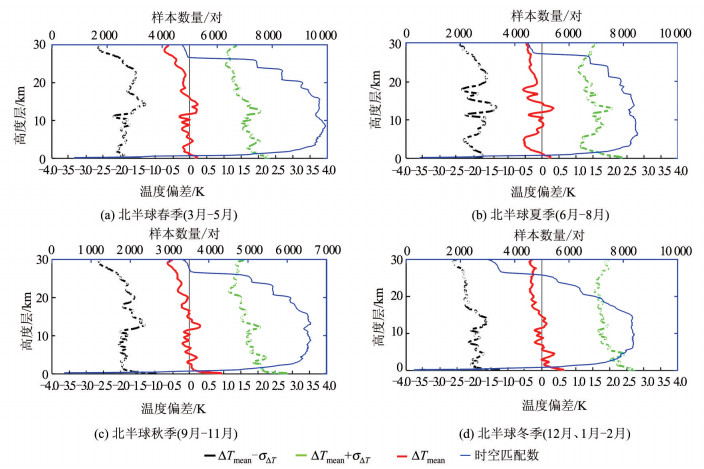
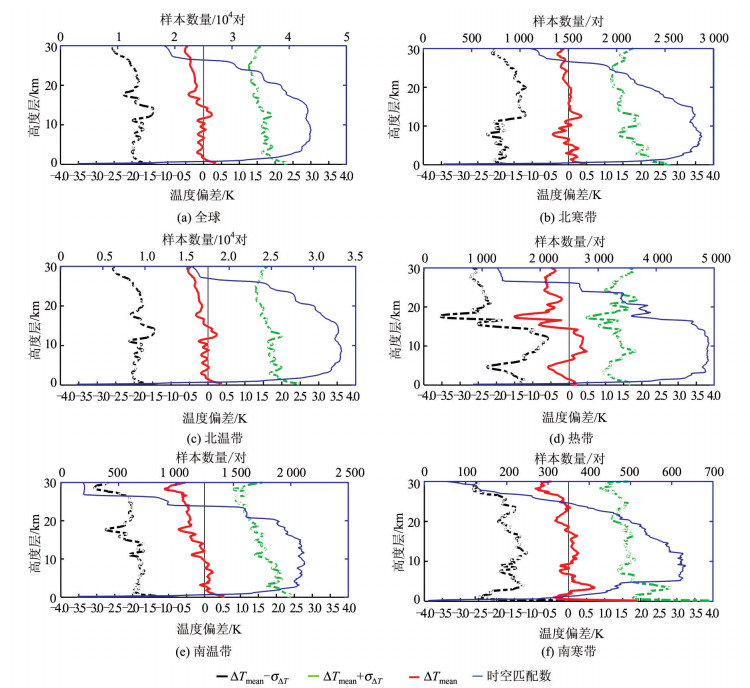
表 1 COSMIC资料统计区域分布
Table 1 Statistical Distribution of COSMIC Data
区域 纬度范围 经度范围 全球 90°S~90°N 180°W~180°E 北寒带 66.5°N~90°N 180°W~180°E 北温带 23.5°N~66.5°N 180°W~180°E 热带 23.5°S~23.5°N 180°W~180°E 南温带 23.5°S~66.5°S 180°W~180°E 南寒带 66.5°S~90°S 180°W~180°E 
下载: 导出CSV
表 2 全球范围探空站资料各个纬度带COSMIC掩星资料时空匹配样本对数
Table 2 Number of Space⁃Time Matching Samples Based on COSMIC Occultation Data at Different Latitudes of Global Sounding Station Data
时期 全球区域 北寒带 北温带 热带 南温带 南寒带 3月—5月 13 110 984 10 206 1 221 513 186 6月—8月 11 628 732 8 712 1 437 582 165 9月—11月 9 093 627 6 828 1 002 528 108 12月、
1月—2月11 433 603 8 814 1 290 549 177 全年 45 264 2 946 34 560 4 950 2 172 636
下载: 导出CSV
表 3 不同月份、不同地区温度偏差统计/K
Table 3 Statistics of Temperature Deviation of Different Regions in Different Months/K
时期 统计参数 全球区域 北寒带 北温带 热带 南温带 南寒带 3月—5月 平均偏差
标准差-0.168 6 -0.074 3 -0.161 4 -0.311 8 -0.258 6 0.048 7 1.689 1 1.591 8 1.695 1 1.614 9 1.756 1 1.429 6 6月—8月 平均偏差
标准差-0.223 4 -0.069 2 -0.235 3 -0.297 2 -0.290 5 0.202 2 1.684 4 1.601 6 1.682 8 1.602 5 1.891 3 1.901 9 9月—11月 平均偏差 -0.123 3 -0.121 2 -0.112 9 -0.317 9 -0.181 5 -0.354 4 标准差 1.740 0 1.562 5 1.760 4 1.516 0 1.832 6 1.957 4 12月、1月—2月 平均偏差
标准差-0.121 1 -0.005 6 -0.102 6 -0.219 7 -0.326 9 -0.162 3 1.918 7 1.932 5 1.968 3 1.627 7 1.711 2 1.445 1 全年 平均偏差
标准差-0.162 8 -0.023 5 -0.157 5 -0.285 0 -0.264 2 -0.051 6 1.757 2 1.628 4 1.778 1 1.599 4 1.805 2 1.673 9
下载: 导出CSV
表 4 季节变化因素方差分析表
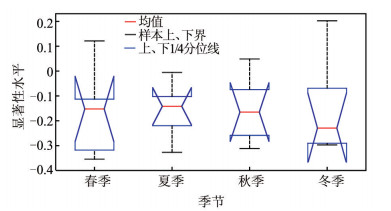
Table 4 Variance Analysis of Seasonal Factor
方差来源 平方和 自由度 均方差 F值 置信度 组间 0.000 28 3 0.000 1 0.01 0.999 6 组内 0.473 91 20 0.023 7 总和 0.474 19 23
下载: 导出CSV
表 5 纬度带因素方差分析表
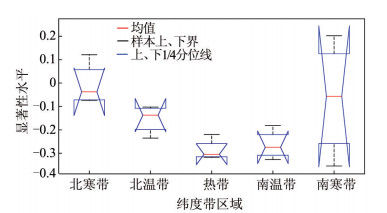
Table 5 Variance Analysis of Latitude Factor
方差来源 平方和 自由度 均方差 F值 置信度 组间 0.236 16 4 0.059 0 3.83 0.024 4 组内 0.231 05 15 0.015 0 总和 0.467 21 19
下载: 导出CSV
-
[1] Steiner A K, Kirchengast G, Ladreiter H P. Inversion, Error Analysis, and Validation of GPS/MET Occultation Data[J]. Annales Geophysicae, 1998, 17(1): 122-138 doi: 10.1007/s00585-999-0122-5
[2] Businger S, Chiswell S R, Bevis M, et al. The Promise of GPS in Atmospheric Monitoring[J]. Bulletin of the American Meteorological Society, 2011, 77(1): 5-18 http://www.nrcresearchpress.com/servlet/linkout?suffix=rg1/ref1&dbid=16&doi=10.1139%2FE09-010&key=10.1175%2F1520-0477(1996)0772.0.CO%3B2
[3] Kursinski E R, Hajj G A, Schofield J T, et al. Observing Earth's Atmosphere with Radio Occultation Measurements Using the Global Positioning System[J]. Journal of Geophysical Research: Atmospheres, 1997, 102(D19): 23 429-23 465 doi: 10.1029/97JD01569
[4] 徐晓华, 刘树纶, 罗佳. 利用COSMIC掩星折射指数分析全球大气边界层顶结构变化[J]. 武汉大学学报·信息科学版, 2018, 43(1): 94-100 doi: 10.13203/j.whugis20160183 Xu Xiaohua, Liu Shulun, Luo Jia. Analysis of Global Atmospheric Boundary Layer Top Structure Changes Using COSMIC Occultation Refraction Index[J]. Geomatics and Information Science of Wuhan University, 2018, 43(1): 94-100 doi: 10.13203/j.whugis20160183
[5] Scherllinpirscher B, Steiner A K, Kirchengast G, et al. The Power of Vertical Geolocation of Atmospheric Profiles from GNSS Radio Occultation[J]. Journal of Geophysical Research Atmospheres, 2017, 122(3): 1 595-1 616 doi: 10.1002/2016JD025902
[6] Ware R, Rocken C, Solheim F, et al. GPS Sounding of the Atmosphere from Low Earth Orbit: Preliminary Results[J]. Bulletin of the American Meteorological Society, 1996, 77(1): 19-40 doi: 10.1175/1520-0477(1996)077<0019:GSOTAF>2.0.CO;2
[7] 乐新安, 郭英华, 曾桢, 等. 近地空间环境的GNSS无线电掩星探测技术[J]. 地球物理学报, 2016, 59(4): 1 161-1 188 https://www.cnki.com.cn/Article/CJFDTOTAL-DQWX201604001.htm Le Xin'an, Guo Yinghua, Zeng Zhen, et al. GNSS Radio Occultation Detection Technology in Near-Earth Space Environment[J]. Chinese Journal of Geophysics, 2016, 59(4): 1 161-1 188 https://www.cnki.com.cn/Article/CJFDTOTAL-DQWX201604001.htm
[8] Hajj G A, Ao C O, Iijima B A, et al. CHAMP and SAC-C Atmospheric Occultation Results and Intercomparisons[J]. Journal of Geophysical Research Atmospheres, 2004, 109(D6): 6 109 doi: 10.1029/2003JD003909/pdf
[9] Ma Z, Kuo Y H, Ralph F M, et al. Assimilation of GPS Radio Occultation Data for an Intense Atmospheric River with the NCEP Regional GSI System[J]. Journal of Changchun University of Science & Technology, 2010, 139(7): 188-197 http://www.ingentaconnect.com/content/mnp/estu/2010/00000025/00000004/art00005
[10] Rocken C, Anthes R, Exner M, et al. Analysis and Validation of GPS/MET Data in the Neutral Atmosphere[J]. Journal of Geophysical Research Atmospheres, 1997, 1 022(D25): 29 849-29 866 http://ci.nii.ac.jp/naid/10013126790/
[11] Schmidt T, Heise S, Wickert J, et al. GPS Radio Occultation with CHAMP and SAC-C: Global Monitoring of Thermal Tropopause Parameters[J]. Atmospheric Chemistry and Physics, 2005, 5(6): 1 473-1 488 doi: 10.5194/acp-5-1473-2005
[12] 王洪, 曹云昌, 肖稳安. COSMIC掩星数据与L波段探空数据的对比分析[J]. 气象, 2010, 36(9): 14-20 https://www.cnki.com.cn/Article/CJFDTOTAL-QXXX201009005.htm Wang Hong, Cao Yunchang, Xiao Wen'an. Comparison Between the COSMIC and L Band Radiosonde Data[J]. Meteorology, 2010, 36(9): 14-20 https://www.cnki.com.cn/Article/CJFDTOTAL-QXXX201009005.htm
[13] 王伯睿, 刘晓阳, 王久珂. COSMIC掩星反演数据质量分析[J]. 北京大学学报(自然科学版), 2013, 49(2): 241-251 https://www.cnki.com.cn/Article/CJFDTOTAL-BJDZ201302012.htm Wang Borui, Liu Xiaoyang, Wang Jiuke. COSMIC Occultation Inversion Data Quality Analysis[J]. Journal of Peking University(Natural Sciences), 2013, 49(2): 241-251 https://www.cnki.com.cn/Article/CJFDTOTAL-BJDZ201302012.htm
[14] Fu E, Zhang K, Marion K, et al. Assessing COSMIC GPS Radio Occultation Derived Atmospheric Parameters Using Australian Radiosonde Network Data[J]. Procedia Earth and Planetary Science, 2009, 1(1): 1 054-1 059 doi: 10.1016/j.proeps.2009.09.162
[15] Sun B, Reale A, Seidel D J, et al. Comparing Radiosonde and COSMIC Atmospheric Profile Data to Quantify Differences Among Radiosonde Types and the Effects of Imperfect Collocation on Comparison Statistics[J]. Journal of Geophysical Research Atmospheres, 2010, 115: D23104 doi: 10.1029/2010JD014457
[16] Kishore P, Namboothiri S P, Jiang J H, et al. Global Temperature Estimates in the Troposphere and Stratosphere: A Validation Study of COSMIC/FORMOSAT-3 Measurements[J]. Atmospheric Chemistry and Physics Discussions, 2008, 8(3): 8 327-8 355 http://onlinelibrary.wiley.com/resolve/reference/ADS?id=2008ACPD....8.8327K
[17] He W, Ho S, Chen H, et al. Assessment of Radiosonde Temperature Measurements in the Upper Troposphere and Lower Stratosphere Using COSMIC Radio Occultation Data[J]. Geophysical Research Letters, 2009, 36(17): 1 397-1 413 doi: 10.1029/2009GL038712/full
[18] 苟小平, 符养, 郭粤宁, 等. COSMIC计划及掩星资料误差分析[J]. 气象科学, 2009, 29(3): 348-354 doi: 10.3969/j.issn.1009-0827.2009.03.011 Gou Xiaoping, Fu Yang, Guo Yuening, et al. COSMIC Mission and Error Analysis of GPS Radio Occultation Data[J]. Scientia Meteorologica Sinica, 2009, 29(3): 348-354 doi: 10.3969/j.issn.1009-0827.2009.03.011
[19] 徐晓华, 李娟, 罗佳, 等. COSMIC掩星电子密度廓线的质量检核[J]. 国防科技大学学报, 2018, 40(5): 54-59 https://www.cnki.com.cn/Article/CJFDTOTAL-GFKJ201805009.htm Xu Xiaohua, Li Juan, Luo Jia, et al. Quality Check of the Ionospheric Density Profiles from COSMIC Radio Occultation Observations[J]. Journal of National University of Defense Technology, 2018, 40(5): 54-59 https://www.cnki.com.cn/Article/CJFDTOTAL-GFKJ201805009.htm
[20] 廖蜜, 张鹏, 毕研盟, 等. 风云三号气象卫星掩星大气产品精度的初步检验[J]. 气象学报, 2015, 73(6): 1 131-1 140 https://www.cnki.com.cn/Article/CJFDTOTAL-QXXB201506011.htm Liao Mi, Zhang Peng, Bi Yanmeng, et al. A Preliminary Estimation of the Radio Occultation Products Accuracy from the Fengyun-3C Meteorological Satellite[J]. Acta Meteor Sinica, 2015, 73(6): 1 131-1 140 https://www.cnki.com.cn/Article/CJFDTOTAL-QXXB201506011.htm
[21] Zou X, Zeng Z.A Quality Control Procedure for GPS Radio Occultation Data[J]. Journal of Geophysical Research Atmospheres, 2006, 111(D2): D02112 doi: 10.1029/2005JD005846/full
[22] 徐晓华, 汪海洪. 不同季节GPS掩星廓线精度的比较研究[J]. 武汉大学学报·信息科学版, 2010, 35(6): 639-643 http://ch.whu.edu.cn/article/id/979 Xu Xiaohua, Wang Haihong. Comparative Study on the Accuracy of GPS Occultation Profiles in Different Seasons[J]. Geomatics and Information Science of Wuhan University, 2010, 35(6): 639-643 http://ch.whu.edu.cn/article/id/979
-
期刊类型引用(8)
1. 张莹，任战利，兰华平，祁凯，邢光远，夏岩. 关中盆地新近系蓝田-灞河组热储层物性及渗流特征研究. 地质通报. 2024(05): 712-725 . 
百度学术
2. 吴陈冰洁，罗璐，高楠安，汪新伟，崔梓贤. 关中盆地西安凹陷新近系砂岩热储特征研究. 现代地质. 2024(06): 1571-1584 . 百度学术
3. 张欢，陈应涛，陶威，陈涛，余文鑫，艾卉卉. 不同拉伸方式和速度下的伸展构造砂箱物理模拟实验研究. 西北地质. 2023(02): 327-336 . 百度学术
4. 颜复康，田镇，杨志强，杨兵，梁沛. 厄瓜多尔俯冲区震间闭锁与粘弹性变形研究. 大地测量与地球动力学. 2023(10): 1080-1085 . 百度学术
5. 张莹，任战利，邢光远，祁凯，夏岩. 渭河盆地新近系热储层特征. 地质通报. 2023(11): 1993-2005 . 百度学术
6. 徐斌，张艳. 地下水化学类型分区的GIS空间分析模型. 武汉大学学报(信息科学版). 2019(06): 866-874 . 百度学术
7. 闫俊义，吕睿，赵涛，王莹，白若冰，古云鹤. 关中盆地地壳应力场特征分析. 山西地震. 2019(03): 39-41 . 百度学术
8. 白相东，关成尧，张艳，袁四化，刘晓燕. 渭河盆地断层系统运动学体制分解与探讨. 防灾科技学院学报. 2018(03): 8-16 . 百度学术
其他类型引用(10)
计量
- 文章访问数:
- HTML全文浏览量:
- PDF下载量:
- 被引次数: 18
