A Multi-Parameter Regularization Method in Downward Continuation for Airborne Gravity Data
-
摘要: 为了克服航空重力向下延拓解算的病态性影响，介绍了一种多参数正则化方法，以均方误差最小为目标函数，设计了选取正则化参数的迭代算法，并比较了基于L曲线法、广义交叉核实(generalized cross-validation，GCV)方法选取正则化参数的Tikhonov正则化方法，同时给出了均方误差意义下多参数正则化解优于最小二乘估计的条件。基于EGM2008地球重力场模型进行了仿真试验，计算结果表明，多参数正则化方法能够保证向下延拓结果的可靠性和稳定性，并优于现有的Tikhonov正则化方法，验证了多参数方法在航空重力向下延拓中的可行性。
-
关键词:
- 航空重力 /
- 向下延拓 /
- Tikhonov正则化 /
- 多参数正则化
Abstract: To overcome the ill-posed problem of downward continuation for airborne gravity data, the paper proposes an alternative multi-parameter regularization method. The algorithm for selecting the regularization is proposed based on the minimization of mean squares error (MSE).In addition, the comparative analysis of the L-curve and generalized eross-validation(GCV) method of selecting the Tikhonov regularization parameter is conducted. Under of MSE, the condition that the multi-parameter regularization method is superior to the least-squares is given. By making using of the EGM2008 earth gravity model, the simulation experiment is performed. The numerical results show that the multi-parameter regularization method can guarantee the reliability and stability of downward continuation. And the multi-parameter regularization method shows the better performance than the general Tikhonov regularization method so that the feasibility of multiparameter regularization method in downward continuation for airborne gravity data is demonstrated.-
Keywords:
- airborne gravity /
- downward continuation /
- Tikhonov regularization /
- multi-parameter
-
-
![]()
图 2 3 mGal误差条件下航空重力向下延拓值与地面参考重力值的差值绝对值(区域1)
Figure 2. Absolute Difference of Down‐Continue Results and Reference Value with 3 mGal Random Error (First Area)
![]()
图 3 5 mGal误差条件下航空重力向下延拓值与地面参考重力值的差值绝对值(区域1)
Figure 3. Absolute Difference of Down‐Continue Results and Reference Value with 5 mGal Random Error (First Area)
![]()
图 4 3 mGal误差条件下航空重力向下延拓值与地面参考重力值的差值绝对值(区域2)
Figure 4. Absolute Difference of Down‐Continue Results and Reference Value with 3 mGal Random Error (Second Area)
![]()
图 5 5 mGal误差条件下航空重力向下延拓值与地面参考重力值的差值绝对值(区域2)
Figure 5. Absolute Difference of Down‐Continue Results and Reference Value with 5 mGal Random Error (Second Area)
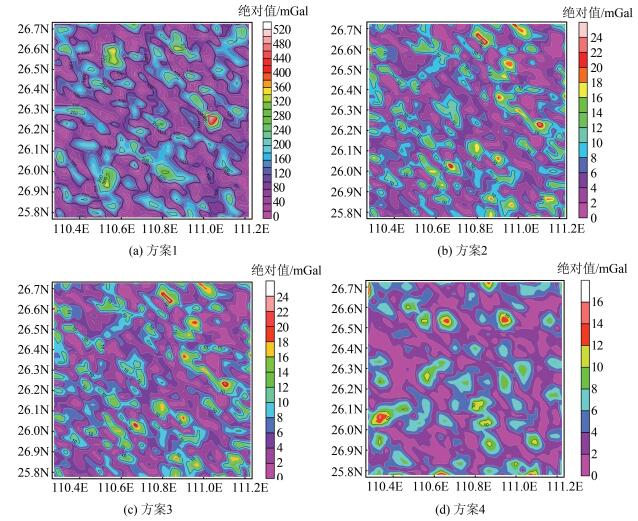
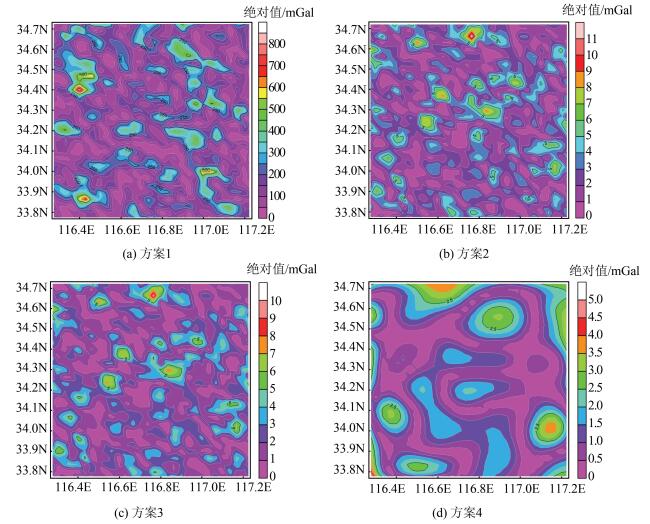
表 1 各种方案大地水准面重力异常与参考值之间距离绝对值的统计结果(区域1) /mGal
Table 1 Statistical Results of Computing Geoid Gravity Gravity Anomaly and Reference Value for Each Scheme(First Area) /mGal
误差 方案 最大值 最小值 平均值 RMS 3 1 520.3 0.2 109.2 134.7 2 29.1 0.0 6.7 8.5 3 27.8 0.0 6.4 8.1 4 18.3 0.0 4.2 5.3 5 1 804.3 0.8 194.5 245.9 2 43.8 0.0 8.2 10.4 3 36.0 0.0 6.9 8.7 4 23.0 0.0 5.3 6.8 
下载: 导出CSV
表 2 各种方案大地水准面重力异常与参考值之间距离绝对值的统计结果(区域2)/mGal
Table 2 Statistical Results of Computing Geoid Gravity Gravity Anomaly and Reference Value for Each Scheme(Second Area) /mGal
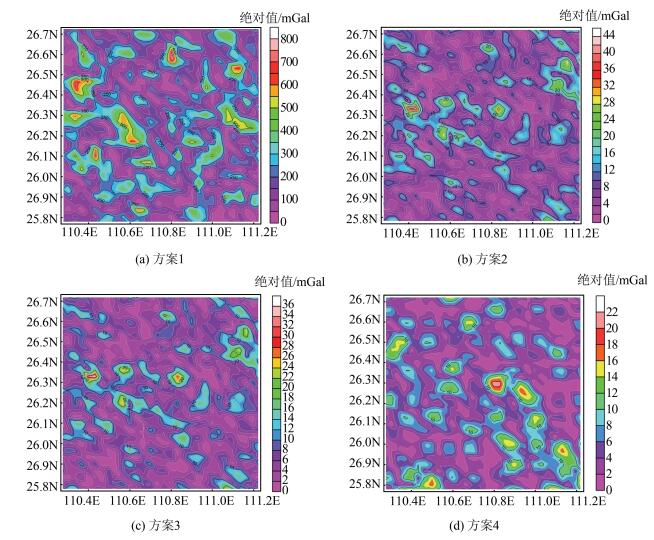
误差 方案 最大值 最小值 平均值 RMS 3 1 866.8 0.5 164.6 209.9 2 11.9 0.0 2.5 3.1 3 9.9 0.0 2.2 2.7 4 5.6 0.0 1.2 1.6 5 1 1 027.1 0.7 259.0 327.0 2 16.1 0.0 3.3 4.2 3 11.7 0.0 2.7 3.3 4 3.3 0.0 1.9 2.4
下载: 导出CSV
-
[1] 孙中苗.航空重力测量理论、方法及应用研究[D].郑州: 信息工程大学, 2004 http://cdmd.cnki.com.cn/Article/CDMD-90008-2005030005.htm Sun Zhongmiao. Theory, Methods and Applications of Airborne Gravimetry[D]. Zhengzhou: Information Engineering University, 2004 http://cdmd.cnki.com.cn/Article/CDMD-90008-2005030005.htm
[2] Kern M. An Analysis of the Combination and Downward Continuation of Satellite, Airborne and Terrestrial Gravity Data[D]. Calgary: University of Calgary, 2003
[3] Novak P, Heck B. Downward Continuation and Geoid Determination Based on Band-limited Airborne Gravity Data[J]. Journal of Geodesy, 2002, 76(5):269-278 doi: 10.1007/s00190-002-0252-y
[4] 王兴涛, 夏哲仁, 石磬, 等.航空重力测量数据向延拓方法的比较[J].地球物理学报, 2004, 47(6):1 017-1 021 http://www.cnki.com.cn/Article/CJFDTotal-DQWX200406012.htm Wang Xintao, Xia Zheren, Shi Pan, et al. A Comparison of Different Downward Continuation Method for Airborne Gravity Data[J]. Chinese Journal of Geophysics, 2004, 47(6):1 017-1 021 http://www.cnki.com.cn/Article/CJFDTotal-DQWX200406012.htm
[5] 陈欣, 翟国君, 暴景阳, 等.航空重力向下延拓的最小二乘配置Tikhonov正则化法[J].武汉大学学报·信息科学版, 2018, 43(4):578-585 doi: 10.13203/j.whugis20150728 Chen Xin, Zhai Guojun, Bao Jingyang, et al. Least Squares Collocation‐Tikhonov Regularization Method for the Downward Continuation of Airborne Gravity Data[J]. Geomatics and Information Science of Wu-han University, 2018, 43(4):578-585 doi: 10.13203/j.whugis20150728
[6] 郝燕玲, 成怡, 孙枫, 等. Tikhono正则化向下延拓算法仿真实验研究[J].仪器仪表学报, 2008, 29(8):2 190-2 194 http://www.cnki.com.cn/Article/CJFDTotal-YQXB200803030.htm Hao Yanling, Cheng Yi, Sun Feng, et al. Simulation Research on Tikhonov Regularization Algorithm Downward Continuation[J]. Chinese Journal of Scientific Instrument, 2008, 29(8):2 190-2 194 http://www.cnki.com.cn/Article/CJFDTotal-YQXB200803030.htm
[7] 邓凯亮, 暴景阳, 黄谟涛, 等.航空重力数据向下延拓的Tikhonov正则化法仿真研究[J].武汉大学学报·信息科学版, 2010, 35(12):1 414-1 417 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=whchkjdxxb201012007 Deng Kailiang, Bao Jingyang, Huang Motao, et al. Simulation of Tikhonov Regularization Algorithmin Downward Continuation of Airborne Gravity Data[J]. Geomatics and Information Science of Wuhan University, 2010, 35(12):1 414-1 417 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=whchkjdxxb201012007
[8] 顾勇为, 归庆明.航空重力测量数据向下延拓基于信噪比的正则化的研究[J].测绘学报, 2010, 39(5):458-464 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=chxb201005004 Gu Yongwei, Gui Qingming. Study of Regularization Based on Signal ‐ to ‐ Noise Index in Airborne Gravity Downward to the Earth Surface[J].Acta Geodaetica et Cartographica Sinica, 2010, 39(5): 458-464 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=chxb201005004
[9] 顾勇为, 归庆明, 韩松辉, 等.航空重力向下延拓分组修正的正则化解法[J].武汉大学学报·信息科学版, 2013, 38(6):720-724 http://ch.whu.edu.cn/article/id/2675 Gu Yongwei, Gui Qingming, Han Songhui, et al. Regularization by Grouping Correction in Downward‐Continuation of Airborne Gravity[J]. Geomatics and Information Science of Wuhan University, 2013, 38(6):720-724 http://ch.whu.edu.cn/article/id/2675
[10] 邓凯亮, 黄谟涛, 暴景阳, 等.向下延拓航空重力数据的Tikhonov双参数正则化法[J].测绘学报, 2011, 40(6):690-696 http://www.cnki.com.cn/Article/CJFDTotal-CHXB201106005.htm Deng Kailiang, Huang Motao, Bao Jingyang, et al.Tikhonov Two-parameter Regularization Algorithm in Downward Continuation of Airborne Gravity Data[J]. Acta Geodaetica et Cartographica Sinica, 2011, 40(6):690-696 http://www.cnki.com.cn/Article/CJFDTotal-CHXB201106005.htm
[11] 蒋涛, 李建成, 王正涛, 等.航空重力向下延拓病态问题的求解[J].测绘学报, 2011, 40(6):684-689 http://www.cnki.com.cn/Article/CJFDTotal-CHXB201106004.htm Jiang Tao, Li Jiancheng, Wang Zhengtao, et al. Solution of Ill-posed Problem in Downward Continuation of Airborne Gravity[J]. Acta Geodaetica et Cartographica Sinica, 2011, 40(6):684-689 http://www.cnki.com.cn/Article/CJFDTotal-CHXB201106004.htm
[12] Lu S, Pereverzev V S. Multi-parameter Regularization and Its Numerical Realization [J]. NumerMath, 2011, 118:1-31 http://d.old.wanfangdata.com.cn/NSTLQK/NSTL_QKJJ0228163020/
[13] Koch K. Parameter Estimation and Hypothesis Test in Linear Model [M]. Germany:Springer, 1998
[14] Hansen P C. Rank-Deficient and Discrete Ill-posed Problems[M]. Philadelphia:SIAM, 1998
[15] Antman S S, Marsden J E, Sirovich L. Statistical and Computational Inverse Problems[M]. Germany:Springer, 2005
[16] Hansen P C. Analysis of Discrete Ill-posed Problems by Means of the L-curve[J]. SIAM Rev, 1992, 34:561-580 doi: 10.1137/1034115
[17] Golub G H, Heath M. Wahba G. Generalized CrossValidation as a Method for Choosing a Good Ridge Parameter [J]. Technometrics, 1979, 21:215-223 doi: 10.1080/00401706.1979.10489751
[18] 王松桂.线性模型的理论及其应用[M].合肥:安徽教育出版社, 1987 Wang Songgui. Theory of Linear Model and Its Applications[M]. Hefei:Anhui Education Press, 1987
-
期刊类型引用(3)
1. 周永章，陈川，张旗，王功文，肖凡，沈文杰，卞静，王亚，杨威，焦守涛，刘艳鹏，韩枫. 地质大数据分析的若干工具与应用. 大地构造与成矿学. 2020(02): 173-182 . 
百度学术
2. 王叶晨梓，杜震洪，张丰，刘仁义. 面向分片地图的多分辨率格点数据统一存取方法. 浙江大学学报(理学版). 2017(05): 584-590 . 百度学术
3. 朱建章，石强，陈凤娥，史晓丹，董泽民，秦前清. 遥感大数据研究现状与发展趋势. 中国图象图形学报. 2016(11): 1425-1439 . 百度学术
其他类型引用(6)
计量
- 文章访问数:
- HTML全文浏览量:
- PDF下载量:
- 被引次数: 9
