Modeling Azimuthal Steel-Track Deformation to Determine the Reference Point of Wheel-Track VLBI Antenna
-
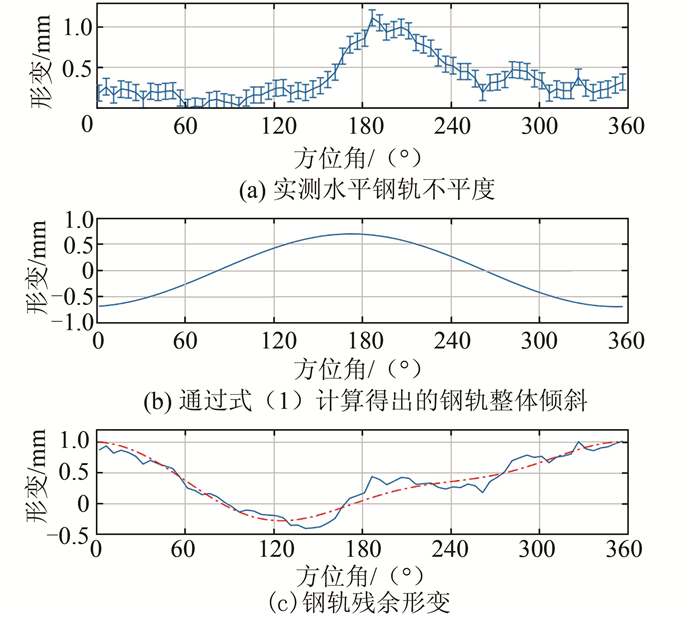
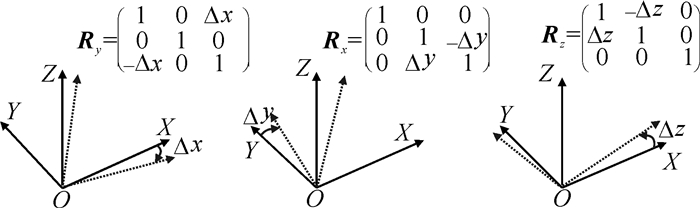
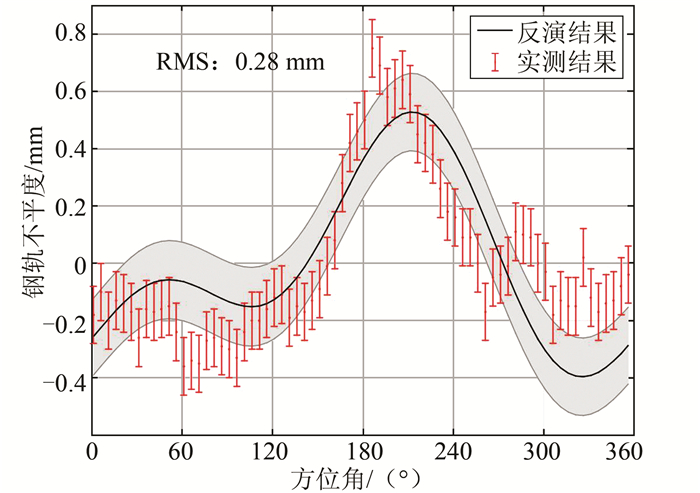
摘要: 为在本地测量中更精确地测定VLBI（very long baseline interferometry）天线参考点（reference point，RP）点位，分析了水平钢轨残余形变对四轮座架式天线的影响，首次提出并推导了用以改正归心解算靶标点（target point，TP）坐标的水平钢轨残余形变模型，结合实测靶标坐标与钢轨水准数据，解释了TP坐标残差中的系统性效应，精化了TP理论坐标，显著提高了参考点测定精度。结果表明，采用水平钢轨残余形变改正模型与估算模型可分别使靶标坐标归心拟后残差中误差提高50%和65%，归心精度均可提高约30%。此外，本钢轨残余形变估算模型还可有效用于该类天线钢轨形变的监测。Abstract: To determine a more precise reference point (RP) coordinate of Very Long Baseline Interfero-metry (VLBI) antenna in a local surveying, the effects of azimuthal track deformations in four-wheels and pedestal-based radio telescopes are analyzed, and an azimuthal steel-track residual deformation correction model is firstly proposed and derived to correct the coordinates of target points (TPs), which are used to determine the RP position. Using the real measurements of TPs and the leveling data of the steel track, systematic effects in residual of TP coordinates are explained and theoretical TP coordinates are corrected so that the corresponding RP precision is significantly improved. The results show that applying azimuthal steel-track residual deformation correction and estimation models can improve the standard error of post-fit TP residuals by 50% and 65%, respectively. The RP precision can improve about 30%. Moreover, the proposed steel-track residual deformation estimation model can be well applied to monitor the azimuth steel-track deformation of four-wheels and pedestal-based antenna.
-
Keywords:
- reference point /
- VLBI /
- local surrey /
- steel-track deformation correction /
- wheel-track mount
-
-
![]()
图 4 利用3类模型拟合7个靶标所得TP拟后残差
Figure 4. Post-Residuals to Observations of 7 Targets Fitted by 3 Different IMs
![]()
图 5 未加入钢轨残余形变改正的靶标分量残差(点)和钢轨残余形变模型所计算的改正值(线)
Figure 5. Component Residuals (Dots) Without Steel-Track Residual Deformation Correction and Corrections (Lines) Calculated by Steel-Track Residual Deformation
![]()
图 7 钢轨不平度的反演与实测结果对比图
Figure 7. Comparison Between Inversion and Real Steel-Track Unevenness
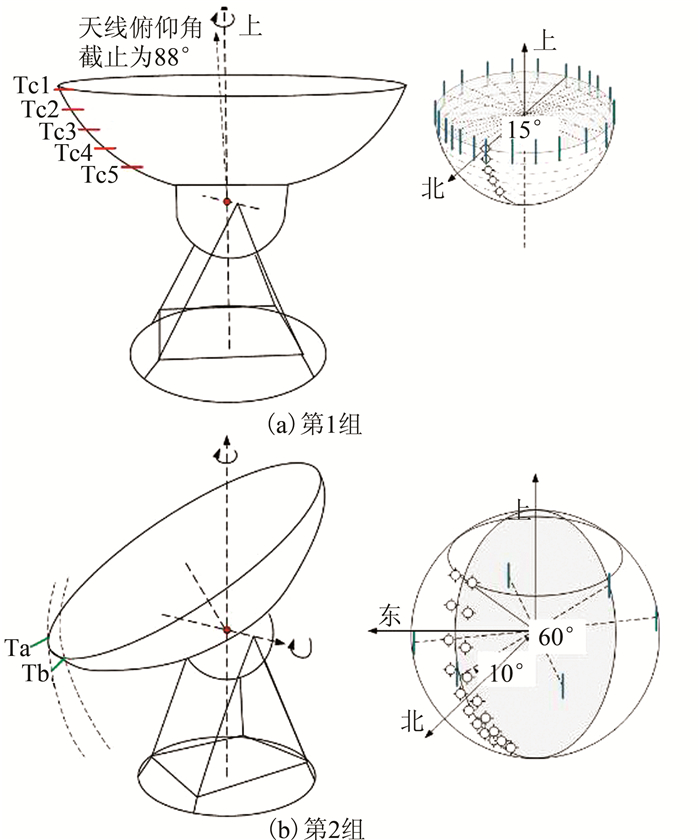
表 1 观测靶标信息统计
Table 1 Statistics of Observed Targets
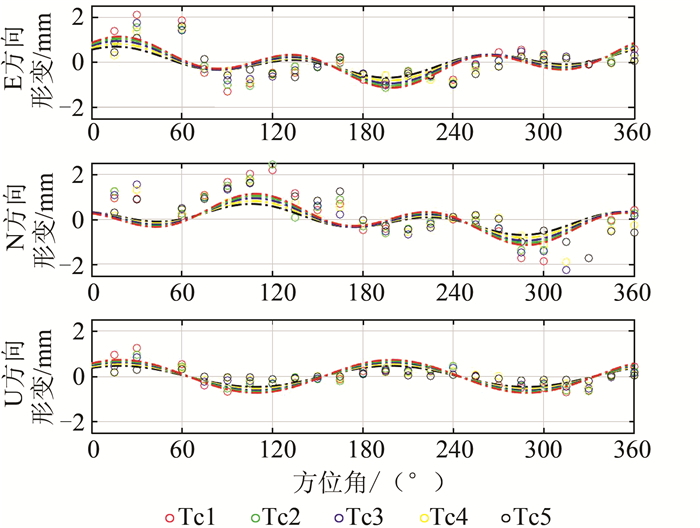
项目 第1组 第2组 靶标名称 Tc1~Tc5 Ta、Tb 靶标数目 5 2 方位定向/(°) 0, 15, 30…360 10, 70, 130…310 方位步进/(°) 15 60 俯仰定向/(°) 88 10, 20, 30…80, 88 俯仰步进/(°) - 10或8 TP观测量数目 125 108 TP分量(1σ/mm) 1.0, 1.0, 1.7 0.3, 0.3, 0.2 
下载: 导出CSV
表 2 不同归心与改正算法所解算参考点点位坐标(1 σ精度)以及模型拟合优度
Table 2 RP Coordinates (1 σ Precision) and Goodness of Fit Based on the Different IMs and Corrections
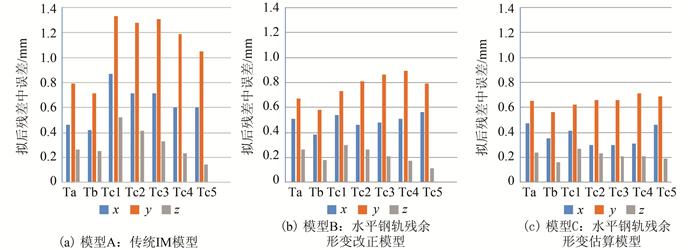
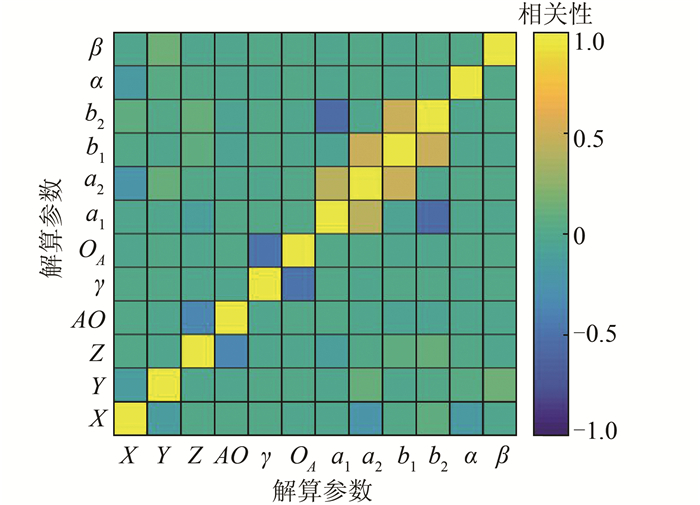
模型 Δx/mm Δy/mm Δz/mm $\frac{{{\chi ^2}}}{{{\mathop{\rm dof}\nolimits} }} $ A -0.09(0.09) -0.11(0.11) 0.07(0.18) 6.9 B -0.23(0.07) 0.11(0.08) -0.08(0.15) 4.4 C 0(0.07) 0(0.07) 0(0.13) 3.5 注：表中所给值为各模型所解算参考点坐标与(63 365.95, -12 396.01, 8 695.44)mm的差值
下载: 导出CSV
-
[1] Seitz M, Angermann D, Bloßfeld M, et al. The 2008 DGFI Realization of the ITRS:DTRF2008[J]. Journal of Geodesy, 2012, 86(12):1097-1123 doi: 10.1007/s00190-012-0567-2
[2] Altamimi Z, Rebischung P, Métivier L, et al. ITRF2014:A New Release of the International Terrestrial Reference Frame Modeling Nonlinear Station Motions[J]. Journal of Geophysical Research Solid Earth, 2016, 121(8):6109-6131 doi: 10.1002/2016JB013098
[3] Bachmann S, Messerschmitt L, Thaller D. IVS Contribution to ITRF2014[M]. Berlin, Heidelberg:Springer, 2015
[4] Zhang Z, Liu X. A VLBI Baseline Post-Adjustment Approach for Station Velocity Estimation in Eurasian Continent[J]. Advances in Space Research, 2014, 54(8):1563-1570 doi: 10.1016/j.asr.2014.06.032
[5] Sarti P, Sillard P, Vittuari L. Surveying Co-Located Space-Geodetic Instruments for ITRF Computation[J]. Journal of Geodesy, 2004, 78(3):210-222 doi: 10.1007/s00190-004-0387-0
[6] Dawson J, Sarti P, Johnston G M, et al. Indirect Approach to Invariant Point Determination for SLR and VLBI Systems:An Assessment[J]. Journal of Geodesy, 2007, 81(6-8):433-441 doi: 10.1007/s00190-006-0125-x
[7] 李金岭, 乔书波, 刘鹂, 等. 2008年佘山25m射电天线归心测量[J].武汉大学学报·信息科学版, 2010, 35(12):1387-1391 http://www.cnki.com.cn/Article/CJFDTotal-WHCH201012002.htm Li Jinling, Qiao Shubo, Liu Li, et al. Site Survey at Sheshan 25 m Radio Telescope in 2008[J]. Geomatic and Information Science of Wuhan University, 2010, 35(12):1387-1391 http://www.cnki.com.cn/Article/CJFDTotal-WHCH201012002.htm
[8] 李金岭, 张津维.利用GPS测量监测VLBI天线参考点的仿真分析[J].武汉大学学报·信息科学版, 2013, 38(12):1387-1391 http://ch.whu.edu.cn/CN/abstract/abstract2816.shtml Li Jinling, Zhang Jinwei. Simulation Analysis of Monitoring of VLBI Antennas Reference Point Via GPS Observations[J]. Geomatic and Information Science of Wuhan University, 2013, 38(12):1387-1391 http://ch.whu.edu.cn/CN/abstract/abstract2816.shtml
[9] Gong X, Shen Y, Wang J, et al. Surveying Colocated GNSS, VLBI, and SLR Stations in China[J]. Journal of Surveying Engineering, 2014, 140(1):28-34 doi: 10.1061/(ASCE)SU.1943-5428.0000118
[10] Leinen S, Becker M, Dow J, et al. Geodetic Determination of Radio Telescope Antenna Reference Point and Rotation Axis Parameters[J]. Journal of Surveying Engineering, 2007, 133(133):41-51 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=38965ab49c052750ec06857d7db35e52
[11] L sler M. New Mathematical Model for Reference Point Determination of an Azimuth-Elevation Type Radio Telescope[J]. Journal of Surveying Engineering, 2009, 135(4):131-135 doi: 10.1061/(ASCE)SU.1943-5428.0000010
[12] Mcginness H, Gale G, Levy R. Estimated Displacements for the VLBI Reference Point of the DSS 1326-m Antenna[C]. The Deep Space Network, Pasadena, California, 1979 http://adsabs.harvard.edu/abs/1979dsn..nasa...36M
[13] Condon J. GBT Pointing Equations[OL]. http://library.nrao.edu/public/memos/gbt/legacy/GBT Memo 75.pdf, 1992
[14] Gawrtonski W, Baher F, Gama E. Track-Level-Compensation Look-Up Table Improves Antenna Pointing Precision[J]. SPIE, 2002, 6273:1-9 http://d.old.wanfangdata.com.cn/NSTLHY/NSTL_HYCC026959358/
[15] 李永江, 艾力玉苏甫, 张正禄, 等.天线轨道变形精密测量与指向偏差模型研究[J].武汉大学学报·信息科学版, 2013, 38(2):176-180 http://ch.whu.edu.cn/CN/abstract/abstract6099.shtml Li Yongjiang, Aili Yu, Zhang Zhenglu, et al. Antenna Track Deformation Precise Measurement and Pointing Error Model[J]. Geomatic and Information Science of Wuhan University, 2013, 38(2):176-180 http://ch.whu.edu.cn/CN/abstract/abstract6099.shtml
[16] Sarti P, Abbondanza C, Vittuari L. Gravity Dependent Signal Path Variation in a Large VLBI Teles-cope Modelled with a Combination of Surveying Methods[J]. Journal of Geodesy, 2009, 83(11):1115-1126 doi: 10.1007/s00190-009-0331-4
[17] Nothnagel A. Conventions on Thermal Expansion Modelling of Radio Telescopes for Geodetic and Astrometric VLBI[J]. Journal of Geodesy, 2009, 83(8):787-792 doi: 10.1007/s00190-008-0284-z
[18] 张阿丽, 熊福文, 朱文耀, 等.新疆天文台25 mVLBI、GPS归心测量[J].大地测量与地球动力学, 2015, 35(4):680-683 http://www.cnki.com.cn/Article/CJFDTotal-DKXB201504032.htm Zhang Ali, Xiong Fuwen, Zhu Wenyao, et al. Co-location Survey at Xinjiang Astronomical Observatory 25 m VLBI and GPS Station[J]. Journal of Geodesy and Geodynamics, 2015, 35(4):680-683 http://www.cnki.com.cn/Article/CJFDTotal-DKXB201504032.htm
[19] 张阿丽, 熊福文, 朱文耀.南山并置站本地连接测量GPS控制网数据精度分析[J].测绘通报, 2013(12):4-7 http://d.old.wanfangdata.com.cn/Periodical/chtb201312002 Zhang Ali, Xiong Fuwen, Zhu Wenyao. The Accuracy and Data Analysis of GPS Control Network at Nanshan Colocation Survey[J]. Bulletin of Surveying and Mapping, 2013(12):4-7 http://d.old.wanfangdata.com.cn/Periodical/chtb201312002
[20] 张阿丽, 熊福文, 朱文耀.新疆天文台本地连接测量的GPS资料归算[J].大地测量与地球动力学, 2013, 33(5):129-132 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=dkxbydz201305029 Zhang Ali, Xiong Fuwen, Zhu Wenyao. Analysis on GPS Observations in Colocation Survey at Xinjiang Astronomical Observatory[J]. Journal of Geodesy and Geodynamics, 2013, 33(5):129-132 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=dkxbydz201305029
[21] Koch K R. Robust Estimations for the Nonlinear Gauss Helmert Model by the Expectation Maximization Algorithm[J]. Journal of Geodesy, 2014, 88:263-271 doi: 10.1007/s00190-013-0681-9
-
期刊类型引用(14)
1. 刘学习，朱守庆，陈国，张克非，郑南山，刘婧璇. 基于全球统一坐标框架的GNSS精密轨道与钟差产品一致性分析. 测绘学报. 2025(03): 432-447 . 
百度学术
2. 冯晓亮，陈欢，李厚芝. 不同观测环境中的多模GNSS数据质量自动化检测方法. 测绘工程. 2024(06): 56-61 . 百度学术
3. 刘嘉伟，孙保琪，韩蕊，张喆，王侃，袁海波，杨旭海. GNSS多系统RTK授时性能分析. 导航定位与授时. 2023(03): 49-58 . 百度学术
4. 王浩浩，郝明，庄文泉. GNSS实时卫星钟差估计在地震监测中的应用. 导航定位与授时. 2023(03): 108-116 . 百度学术
5. 周长江，余海锋，王林伟，雷云平，岳彩亚. 无频间钟偏差改正的BDS-2三频非组合PPP随机模型优化. 测绘通报. 2023(12): 164-168 . 百度学术
6. 潘丽静，刘翔，夏川茹，王雷雷. GNSS精密卫星钟差实时估计与分析. 城市勘测. 2021(06): 73-76 . 百度学术
7. 郭磊，王甫红，桑吉章，张万威. 一种新的利用历元间位置变化量约束的GNSS导航算法. 武汉大学学报(信息科学版). 2020(01): 21-27 . 百度学术
8. 陶钧，张柔. GPS/BeiDou/Galileo/GLONASS实时精密卫星钟差估计. 测绘地理信息. 2020(03): 102-106 . 百度学术
9. 黄观文，王浩浩，谢威，曹钰. GNSS实时卫星钟差估计技术进展. 导航定位与授时. 2020(05): 1-9 . 百度学术
10. 张浩，赵兴旺，陈佩文，谢毅. GPS/BDS卫星钟差融合解算模型及精度分析. 合肥工业大学学报(自然科学版). 2020(09): 1192-1196 . 百度学术
11. 叶珍，李浩军. GNSS卫星钟差估计与结果分析. 导航定位与授时. 2019(03): 88-94 . 百度学术
12. 盛剑锋，张彩红，谭凯. 一种全球导航卫星系统钟差估计优化方案的量化研究. 科学技术与工程. 2019(14): 14-21 . 百度学术
13. 王尔申，赵珩，曲萍萍，庞涛，孙军. 基于拉格朗日插值法的卫星导航空间信号精度评估算法. 沈阳航空航天大学学报. 2019(04): 43-48 . 百度学术
14. 李云，崔文刚. 精密单点定位技术发展及应用. 科学技术与工程. 2019(27): 1-11 . 百度学术
其他类型引用(10)
计量
- 文章访问数: 1725
- HTML全文浏览量: 221
- PDF下载量: 109
- 被引次数: 24
