A Robust Normal Estimation Method for Terrestrial Laser Scanning Point Cloud Based on Minimum Covariance Determinant
-
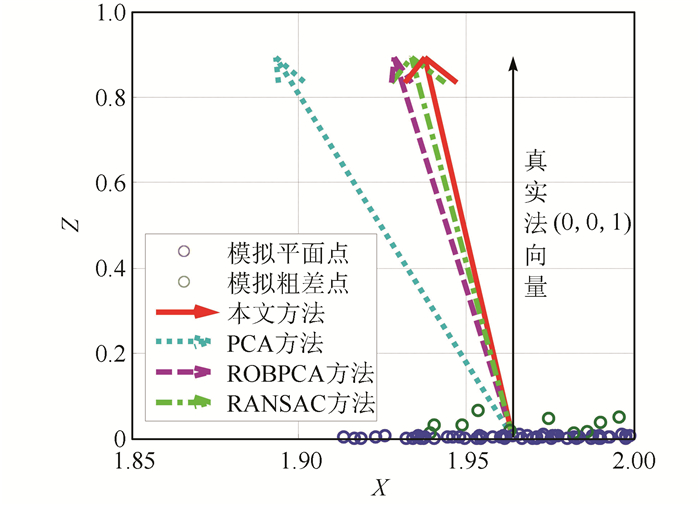
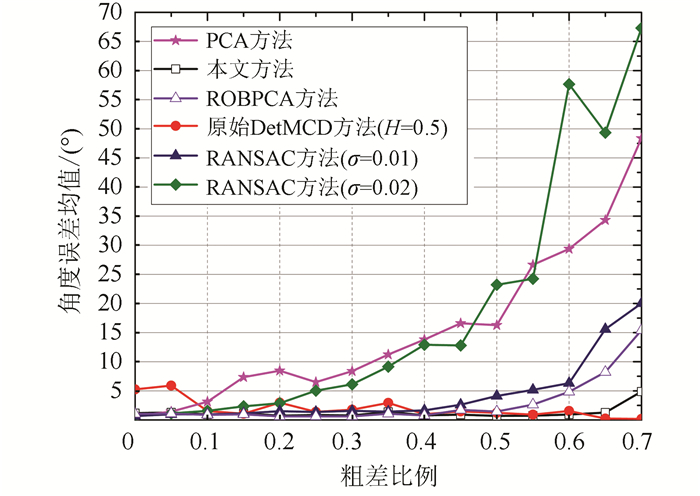
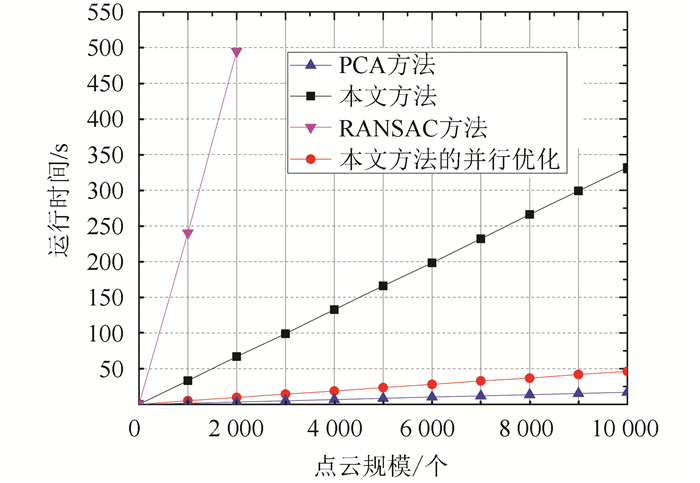
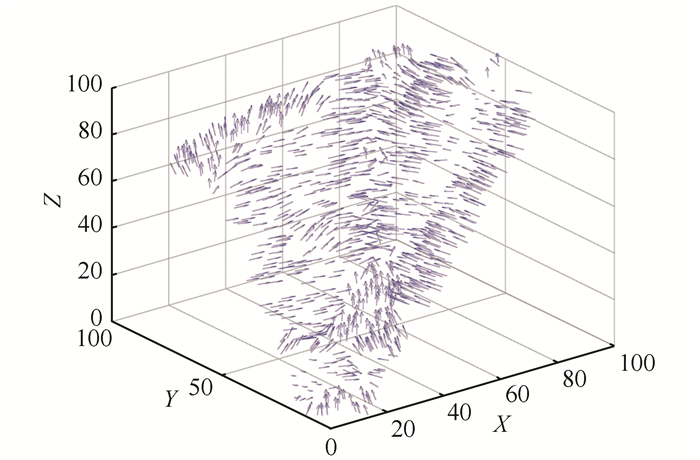
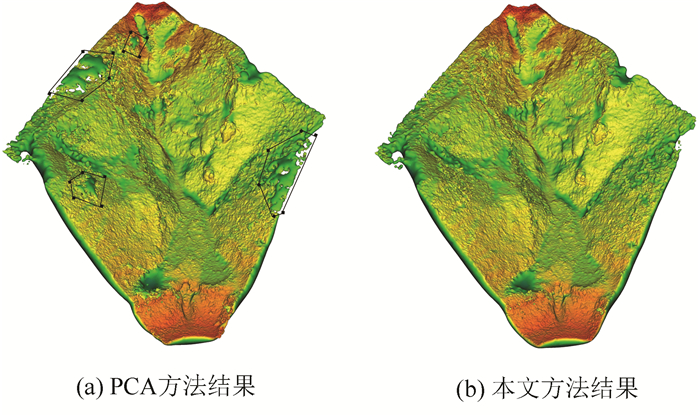
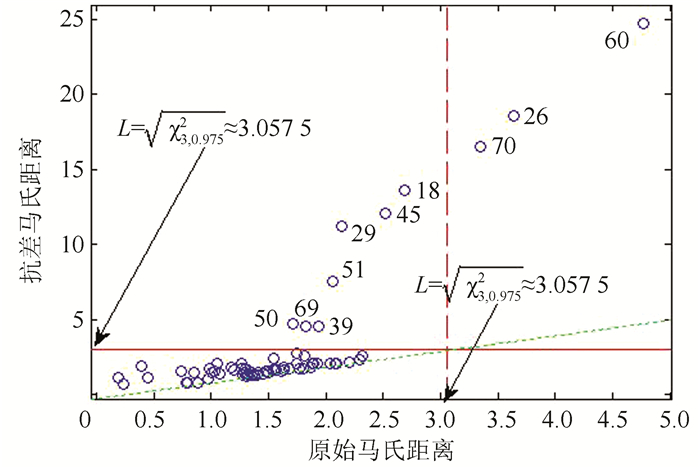
摘要: 针对地面激光扫描点云中的粗差与不均匀采样对法向量计算的影响，基于最小广义方差估计与局部平面拟合原理提出了一种抗差法向量求解方法。首先通过快速近似最近邻居搜索算法得到最近k邻居点集，然后由确定型最小广义方差估计方法和多元马氏距离得到邻居点集协方差矩阵的抗差估计，最后根据主成分分析法（principal component analysis，PCA）计算得到抗差法向量。通过构造的模拟地面激光扫描（terrestrial laser scanning，TLS）点云数据将提出的方法分别与基于PCA、鲁棒PCA和随机抽样一致的法向量求解方法进行实验比较。结果表明，所提方法的抗差性能优异，且并行优化改进后可以满足大规模TLS点云的计算需求。将该方法应用于实际野外地形TLS点云数据，由求解的抗差法向量重建的泊松表面更符合实际地形，表明了该方法在实际应用中的有效性。
-
关键词:
- 点云 /
- 地面激光扫描 /
- 法向量求解 /
- 主成分分析 /
- 确定型最小广义方差估计
Abstract: A robust normal estimation method based on local plane fitting and minimum covariance determinant (MCD) is proposed for terrestrial laser scanning (TLS) point cloud with gross errors and non-uniform sampling. Firstly, fast library for approximate nearest neighbors algorithm is performed to retrieve k nearest neighbor point set. Then, robust estimation of its covariance is calculated by DetMCD (deterministic MCD) and multivariate Mahalanobis distance. Finally, robust estimation of normal vector is calculated through principal component analysis (PCA) method. Compared with PCA, robust PCA and random sample consensus based normal estimation method, on simulated TLS point cloud, experimental results show that the proposed method can get more accurate normal estimation under the influences of gross errors. And its parallel improvement can meet the requirement of efficiency for large scale TLS point cloud processing. Further experiment on real TLS data from natural terrain shows that the proposed method helps to better Poisson surface reconstruction and prove its effectiveness in practical application. -
-
![]()
图 1 邻居点的马氏距离与抗差马氏距离对粗差的识别
Figure 1. Gross Error Identification Based on Original Mahalanobis Distance and Robust Mahalanobis Distance of Each Neighbor Point
![]()
图 2 不同方法计算出的模拟TLS点云中一组邻居点的法向量(粗差点比例为30%)
Figure 2. Normals of a Neighbor Point Set in Simulated TLS Point Cloud by Different Methods (with 30% Gross Error)
![]()
图 3 不同粗差比例对不同方法角度计算误差均值的影响
Figure 3. Impact of Different Gross Error Rates on Mean of Normal Angle Errors Calculated by Different Methods
![]()
图 4 不同方法不同点云规模的时间效率比较
Figure 4. Comparison of Running Time with Different Point Cloud Scales by Different Methods
![]()
图 5 本文方法得到的真实TLS点云的抗差法向量
Figure 5. Robust Normal Estimation of Real TLS Point Cloud by the Proposed Method
-
[1] 李国俊, 李宗春, 孙元超, 等.利用Delaunay细分进行噪声点云曲面重建[J].武汉大学学报·信息科学版, 2017, 42(1):123-129 http://ch.whu.edu.cn/CN/abstract/abstract5645.shtml Li Guojun, Li Zongchun, Sun Yuanchao, et al. Using Delaunay Refinement to Reconstruct Surface from Noisy Point Clouds[J]. Geomatics and Information Science of Wuhan University, 2017, 42(1): 123-129 http://ch.whu.edu.cn/CN/abstract/abstract5645.shtml
[2] 谭凯, 程效军, 张吉星. TLS强度数据的入射角及距离效应改正方法[J].武汉大学学报·信息科学版, 2017, 42(2):223-228 http://ch.whu.edu.cn/CN/abstract/abstract5665.shtml Tan Kai, Cheng Xiaojun, Zhang Jixing. Correction for Incidence Angle and Distance Effects on TLS Intensity Data[J]. Geomatics and Information Science of Wuhan University, 2017, 42(2): 223-228 http://ch.whu.edu.cn/CN/abstract/abstract5665.shtml
[3] Hoppe H, DeRose T, Duchamp T, et al. Surface Reconstruction from Unorganized Points[C]. The 19th Annual Conference on Computer Graphics and Interactive Techniques, New York, USA, 1992 http://dl.acm.org/citation.cfm?id=134011
[4] 李宝, 程志全, 党岗, 等.三维点云法向量估计综述[J].计算机工程与应用, 2010, 46(23): 1-7 doi: 10.3778/j.issn.1002-8331.2010.23.001 Li Bao, Cheng Zhiquan, Dang Gang, et al. Survey on Normal Estimation for 3D Point Clouds[J]. Computer Engineering and Applications, 2010, 46(23): 1-7 doi: 10.3778/j.issn.1002-8331.2010.23.001
[5] Yang M, Lee E. Segmentation of Measured Point Data Using a Parametric Quadric Surface Approximation[J]. Computer-Aided Design, 1999, 31(7): 449-457 doi: 10.1016/S0010-4485(99)00042-1
[6] Alexa M, Behr J, Cohen-Or D, et al. Point Set Surfaces[C]. The Conference on Visualization'01, San Diego, California, USA, 2001
[7] Pauly M, Keiser R, Kobbelt L P, et al. Shape Modeling with Point-Sampled Geometry[J]. ACM Transactions on Graphics, 2003, 22(3): 641-650 doi: 10.1145/882262
[8] Fleishman S, Cohenor D, Silva C T. Robust Moving Least-Squares Fitting with Sharp Features[J]. ACM Transactions on Graphics, 2005, 24(3): 544-552 doi: 10.1145/1073204
[9] Castillo E, Liang J, Zhao H K. Point Cloud Segmentation and Denoising via Constrained Nonlinear Least Squares Normal Estimates[M]// Breuß M, Bruckstein A, Maragos P. Innovations for Shape Analysis. Berlin, Heidelberg: Springer, 2013
[10] Schnabel R, Wahl R, Klein R. Efficient RANSAC for Point-Cloud Shape Detection[J]. Computer Graphics Forum, 2007, 26(2): 214-226 doi: 10.1111/cgf.2007.26.issue-2
[11] Yoon M, Lee Y, Lee S, et al. Surface and Normal Ensembles for Surface Reconstruction[J]. Compu-ter-Aided Design, 2007, 39(5): 408-420 doi: 10.1016/j.cad.2007.02.008
[12] Li B, Schnabel R, Klein R, et al. Robust Normal Estimation for Point Clouds with Sharp Features[J]. Computers & Graphics, 2010, 34(2): 94-106 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=JJ0215979225
[13] Hubert M, Rousseeuw P J, Verdonck T. A Deterministic Algorithm for Robust Location and Scatter[J]. Journal of Computational & Graphical Statistics, 2012, 21(3): 618-637 doi: 10.1080/10618600.2012.672100
[14] Muja M, Lowe D G. Scalable Nearest Neighbor Algorithms for High Dimensional Data[J]. IEEE Transactions on Pattern Analysis & Machine Intelligence, 2014, 36(11): 2 227-2 240 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=CC0213214818
[15] Mitra N J, Nguyen A, Guibas L. Estimating Surface Normals in Noisy Point Cloud Data[J]. International Journal of Computational Geometry & Applications, 2004, 14(4-5): 261-276 http://www.ams.org/mathscinet-getitem?mr=2087824
[16] Rousseeuw P J. Multivariate Estimation with High Breakdown Point[J]. Mathematical Statistics and Applications, 1985, B: 283-297 http://d.old.wanfangdata.com.cn/OAPaper/oai_arXiv.org_1004.4883
[17] Nurunnabi A, Belton D, West G. Diagnostic-Robust Statistical Analysis for Local Surface Fitting in 3D Point Cloud Data[C]. The 22nd Congress of International Society for Photogrammetry and Remote Sensing, Melbourne, Australia, 2012
[18] McLachlan J. Discriminant Analysis and Statistical Pattern Recognition[J]. Technometrics, 1993, 88(422): 695-697 http://d.old.wanfangdata.com.cn/Periodical/rjxb200504005
[19] 王醒策, 蔡建平, 武仲科, 等.局部表面拟合的点云模型法向估计及重定向算法[J].计算机辅助设计与图形学学报, 2015, 27(4): 614-620 http://d.old.wanfangdata.com.cn/Periodical/jsjfzsjytxxxb201504007 Wang Xingce, Cai Jianping, Wu Zhongke, et al. Normal Estimation and Normal Orientation for Point Cloud Model Based on Improved Local Surface Fitting[J]. Journal of Computer-Aided Design & Computer Graphics, 2015, 27(4): 614-620 http://d.old.wanfangdata.com.cn/Periodical/jsjfzsjytxxxb201504007
[20] Hubert M, Rousseeuw P J. ROBPCA: A New Approach to Robust Principal Component Analysis[J]. Technometrics, 2005, 47(1): 64-79 doi: 10.1198/004017004000000563
[21] Fischler M A, Bolles R C. Random Sample Consensus: A Paradigm for Model Fitting with Applications to Image Analysis and Automated Cartography[J]. Communications of the ACM, 1981, 24(6): 381-395 doi: 10.1145/358669.358692
-
期刊类型引用(3)
1. 周永章，陈川，张旗，王功文，肖凡，沈文杰，卞静，王亚，杨威，焦守涛，刘艳鹏，韩枫. 地质大数据分析的若干工具与应用. 大地构造与成矿学. 2020(02): 173-182 . 
百度学术
2. 王叶晨梓，杜震洪，张丰，刘仁义. 面向分片地图的多分辨率格点数据统一存取方法. 浙江大学学报(理学版). 2017(05): 584-590 . 百度学术
3. 朱建章，石强，陈凤娥，史晓丹，董泽民，秦前清. 遥感大数据研究现状与发展趋势. 中国图象图形学报. 2016(11): 1425-1439 . 百度学术
其他类型引用(6)
下载:
计量
- 文章访问数: 1615
- HTML全文浏览量: 205
- PDF下载量: 234
- 被引次数: 9
