Test and Equivalent Verification of Gravity Correction Models for Platform Tilt in Sea-Borne and Air-Borne Gravimetry
-
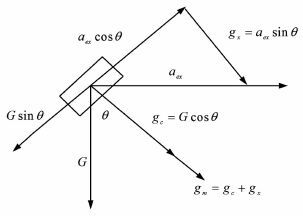
摘要: 关于重力测量稳定平台倾斜改正模型选用问题,国内外学者和机构至今未取得完全一致的意见。从理论上证明了当前国际上推荐使用的三种重力平台倾斜改正模型的等价性,估算了平台倾斜重力改正的量值大小,并采用航空重力测量实测数据,对三种改正模型进行了数值验证和对比分析,得出了比较明确的结论,为实际作业选用合适的数据处理流程和改正模型提供了理论依据。Abstract: Currently there is no consensus among scholars on the appropriate choice of gravity correction models for platform tilt in sea-borne and airborne gravimetry. In this paper, the equivalency of the three existing correction models recommended for usage are analysed theoretically and platform tilt effect is empirically evaluated. A set of airborne data from practical survey lines is used to examine and compare the numerical value of platform tilt effect of the three existing correction models. The results clearly and definitely prove our efforts, and this offers useful theoretical support for the choice of data processing steps and correction models in application of airborne gravimetry.
-
-
![]()
图 2 重力改正随水平加速度大小变化情况
Figure 2. Variation of Gravity Correction Changes with Horizontal Acceleration
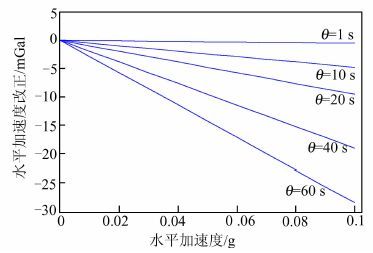
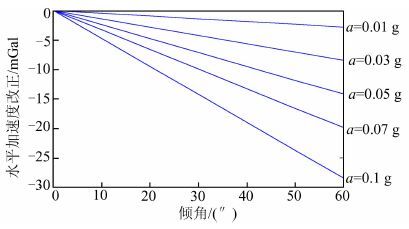
表 1 不同倾角条件下的改正数计算值对比/(10-5m\5s-2)
Table 1 Calculation Results from Different Models with Different Tilt Angles /(10-5m\5s-2)
θx、θy 30″ 1′ 10′ 30′ 1° 2° 3° 5° 模型一 -1.85 -3.65 -29.05 -37.40 74.45 745.74 2 013.1 6 328.9 模型二 -1.85 -3.65 -29.05 -37.40 74.46 745.97 2 014.5 6 342.8 模型三 -1.85 -3.65 -29.05 -37.40 74.43 745.11 2 009.6 6 301.0 
下载: 导出CSV
表 2 模型一分步与一步滤波计算效果对比/(10-5m\5s-2)
Table 2 Calculation Results from Model 1 Using Step by Step Filtering/(10-5m\5s-2)
预滤波 长度/s 加速度预滤波 改正数总滤波 滤波长度/s 加速度不做预滤波 改正数一步滤波 均值 均方根 均值 均方根 均值 均方根 均值 均方根 0 -0.96 6.68 -0.97 1.43 0 -0.96 6.68 -0.96 6.68 20 -0.69 5.14 -0.70 1.24 20 -0.96 6.68 -0.96 5.03 60 -0.42 3.81 -0.43 0.95 60 -0.96 6.68 -0.96 3.33 100 -0.26 2.39 -0.26 0.66 100 -0.96 6.68 -0.96 2.35 140 -0.17 1.31 -0.17 0.45 140 -0.96 6.68 -0.96 1.89 180 -0.12 0.69 -0.12 0.30 180 -0.96 6.68 -0.96 1.65 220 -0.10 0.38 -0.10 0.21 220 -0.96 6.68 -0.97 1.49 240 -0.09 0.30 -0.09 0.18 240 -0.96 6.68 -0.97 1.43
下载: 导出CSV
表 3 模型二分步滤波计算效果对比/(10-5m\5s-2)
Table 3 Calculation Results from Model 2 Using Step by Step Filtering/(10-5m\5s-2)
预滤波长度/s 斜倾角预滤波 改正数总滤波 加速度预滤波 改正数总滤波 均值 均方根 均值 均方根 均值 均方根 均值 均方根 0 -0.96 6.68 -0.97 1.43 -0.96 6.68 -0.97 1.43 20 -0.77 6.21 -0.77 1.37 -0.55 5.47 -0.56 1.16 60 -0.70 5.66 -0.70 1.30 -0.14 4.48 -0.16 0.79 100 -0.63 4.99 -0.63 1.28 0.29 3.33 0.28 0.51 140 -0.53 4.30 -0.53 1.19 0.66 2.41 0.65 0.69 180 -0.42 3.67 -0.42 1.03 0.94 2.08 0.93 1.03 220 -0.33 3.12 -0.33 0.85 1.13 2.17 1.13 1.31 240 -0.29 2.88 -0.29 0.77 1.20 2.25 1.20 1.42
下载: 导出CSV
表 4 模型三分步滤波计算效果对比/(10-5m\5s-2)
Table 4 Calculation Results from Model 3 Using Step by Step Filtering/(10-5m\5s-2)
预滤波长度/s 斜倾角预滤波 改正数总滤波 加速度预滤波 改正数总滤波 均值 均方根 均值 均方根 均值 均方根 均值 均方根 0 -0.96 6.68 -0.97 1.43 -0.96 6.68 -0.97 1.43 20 -0.71 6.10 -0.72 1.27 -0.92 5.54 -0.93 1.40 60 -0.38 5.44 -0.38 1.06 -0.91 4.71 -0.92 1.31 100 -0.12 4.70 -0.12 0.95 -1.01 3.70 -1.02 1.35 140 0.01 4.03 0.01 0.90 -1.16 2.97 -1.16 1.51 180 0.05 3.45 0.05 0.82 -1.30 2.69 -1.30 1.68 220 0.04 2.95 0.04 0.71 -1.41 2.67 -1.41 1.80 240 0.04 2.72 0.04 0.66 -1.46 2.69 -1.46 1.85
下载: 导出CSV
-
[1] 黄谟涛,翟国君,管铮,等.海洋重力场测定及其应用[M].北京:测绘出版社,2005 Huang Motao, Zhai Guojun, Guan Zheng, et al. The Determination and Application of Marine Gravity Field[M]. Beijing:Surveying and Mapping Press, 2005
[2] 孙中苗.航空重力测量理论、方法及应用研究[D].郑州:信息工程大学,2004 Sun Zhongmiao. Theory, Methods and Applications of Airborne Gravimetry[D]. Zhengzhou:Information Engineering University, 2004
[3] Schwarz K P, Li Y C. An Introduction to AirborneGravimetry and Its Boundary Value Problems[R]. Lecture Notes, IAG International Summer School, Como Italy, 1996
[4] Alberts B. Regional Gravity Field Modeling Using Airborne Gravimetry Data[C]. Netherlands Geodetic Commission, Delft, 2009
[5] Valliant H D.The LaCoste and Romberg Air/Sea Gravity Meter:An Overview[M].2nd ed. Florida:CRC Press, 1991
[6] Sokolov A. High Accuracy Airborne Gravity Measurements Methods and Equipment[C]. 18th IFAC World Congress, Milano, 2011
[7] Olson D. GT-1A and GT-2A Airborne Gravimeters:Improvements in Design, Operation, and Processing from 2003 to 2010[C]. Airborne Gravity Workshop, Canberra, 2010
[8] Ferguson S, Hammada Y. Experiences with AIRGrav:Results from a New Airborne Gravimeter[C]. IAG International Symposium on Gravity, Geoid and Geodynamics, Canada, 2000
[9] 奚碚华,于浩,周贤高.海洋重力测量误差补偿技术[J]. 中国惯性技术学报,2011,19(1):1-5 Xi Beihua, Yu Hao, Zhou Xiangao. Compensation of Ocean Gravity Measurement Errors[J]. Journal of Chinese Inertial Technology, 2011, 19(1):1-5
[10] Swain C J.Short Note:Horizontal Acceleration Corrections in Airborne Gravimetry[J]. Geophysics, 1996, 61(1):273-276
[11] 欧阳永忠,陆秀平,黄谟涛,等.L&R海空重力仪测量误差综合补偿方法[J].武汉大学学报·信息科学版,2011,36(5):625-629 Ouyang Yongzhong, Lu Xiuping, Huang Motao, et al. An Integrated Method for Compensating the Systematic Errors of Marine and Airborne Measurements from L&R Gravimeter[J]. Geomatics and Information Science of Wuhan University, 2011, 36(5):625-629
[12] 黄谟涛,欧阳永忠,翟国君,等.海面与航空重力测量重复测线精度评估公式注记[J].武汉大学学报·信息科学版,2013,38(10):1175-1177 Huang Motao, Ouyang Yongzhong, Zhai Guojun, et al. Comment on the Formulas of Accuracy Evaluation for Multi-line Overlapping Measurements in Ship-Borne and Airborne Gravity Survey[J]. Geomatics and Information Science of Wuhan University, 2013, 38(10):1175-1177
[13] 石磐,孙中苗,肖云.航空重力测量中水平加速度改正的计算与频谱分析[J]. 武汉大学学报·信息科学版,2001,26(6):549-554 Shi Pan, Sun Zhongmiao, Xiao Yun. Calculation and Spectra Analysis of Horizontal Acceleration Corrections for Airborne Gravimetry[J]. Geomatics and Information Science of Wuhan University, 2001, 26(6):549-554
[14] Peters M F,Brozena J M. Methods to Improve Existing Shipboard Gravimeters for Airborne Gravimetry[C]. IAG Symposium on Airborne Gravity Field Determination, Colorado, 1995
[15] Olesen A V. Improved Airborne Scalar Gravimetry for Regional Gravity Field Mapping and Geoid Determination[D].Copenhagen:University of Copenhagen,2002
[16] 李宏生,赵立业,周百令,等.水下重力辅助导航实时水平加速度改正方法[J].中国惯性技术学报,2009,17(2):159-164 Li Hongsheng, Zhao Liye, Zhou Bailing, et al. Real-Time Horizontal Acceleration Correction Method in Underwater Gravimetry for Gravity-Aided Navigation Systems[J]. Journal of Chinese Inertial Technology, 2009, 17(2):159-164
[17] 孙中苗,夏哲仁,李迎春,等. L&R航空重力仪的水平加速度改正[J].测绘科学技术学报,2007,24(4):259-262 Sun Zhongmiao, Xia Zheren, Li Yingchun, et al. Horizontal Acceleration Correction for the L&R Airborne Gravimeter[J]. Journal of Zhengzhou Institute of Surveying and Mapping, 2007, 24(4):259-262
[18] GJB 6561-2008.航空重力测量作业规范[S].北京:总装备部军标出版发行部,2008 GJB 6561-2008. Rules for Operations of Airborne Gravimetry[S]. Beijing:Military Standard Press of the Headquarters of General Equipment, 2008
[19] 孙中苗,翟振和,肖云,等.航空重力测量的系统误差补偿[J].地球物理学报,2013,56(1):47-52 Sun Zhongmiao, Zhai Zhenhe, Xiao Yun, et al. Systematic Error Compensation for Airborne Gravimetry[J]. Chinese Journal of Geophysics, 2013, 56(1):47-52
[20] 田颜锋,李姗姗,肖凡. 航空重力测量水平加速度改正的小波预处理[J].大地测量与地球动力学,2012,32(2):115-119 Tian Yanfeng, Li Shanshan, Xiao Fan. Wavelet Pre-processing for Horizontal Acceleration of Aerial Gravity Measurement[J]. Journal of Geodesy and Geodynamics, 2012, 32(2):115-119
[21] Lacoste L. Measurement of Gravity at Sea and in the Air[J]. Reviews of Geophysics, 1967, 5(4):477-526
[22] Li Xiaopeng. An Exact Formula for the Tilt Correction in Scalar Airborne Gravimetry[J]. Journal of Applied Geodesy, 2011, 5:81-85
[23] Neumeyer J, Schafer U, Kremer J. Derivation of Gravity Anomalies from Airborne Gravimeter and IMU Recoding-Validation with Regional Analytic Models Using Ground and Satellite Gravity Data[J]. Journal of Geodynamics, 2009, 47:191-200
[24] Hwang C, Hsiao Y S, Shih H C, et al. Geodetic and Geophysical Results from a Taiwan Airborne Gravity Survey:Data Reduction and Accuracy Assessment[J]. Journal of Geophysical Research, 2007, DOI: 10.1029/2005JB004220
[25] 欧阳永忠,邓凯亮,陆秀平,等.多型航空重力仪同机测试及其数据分析[J].海洋测绘,2013,33(4):6-11 Ouyang Yongzhong, Deng Kailiang, Lu Xiuping, et al. Test of Multi-type Gravimeters and Analysis[J]. Hydrographic Surveying and Charting, 2013, 33(4):6-11
[26] 陈怀琛.数字信号处理教程[M].北京:电子工业出版社,2013 Chen Huaichen. A Course for Digital Signal Processing[M]. Beijing:Publishing House of Electronics Industry, 2013
计量
- 文章访问数: 1676
- HTML全文浏览量: 217
- PDF下载量: 364
