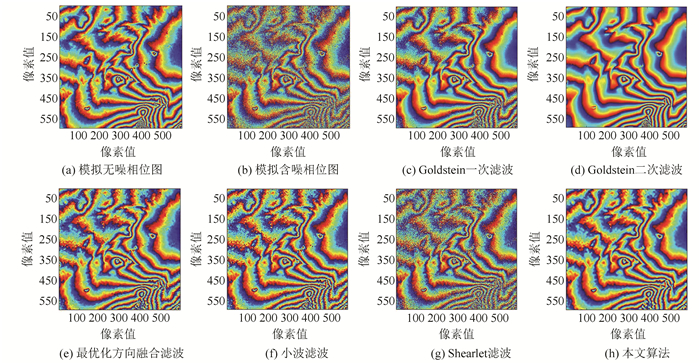
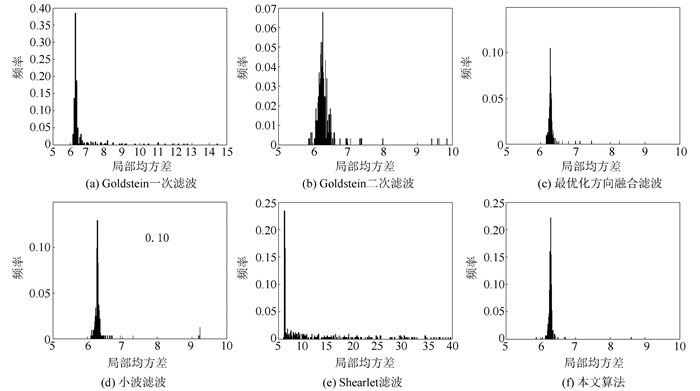
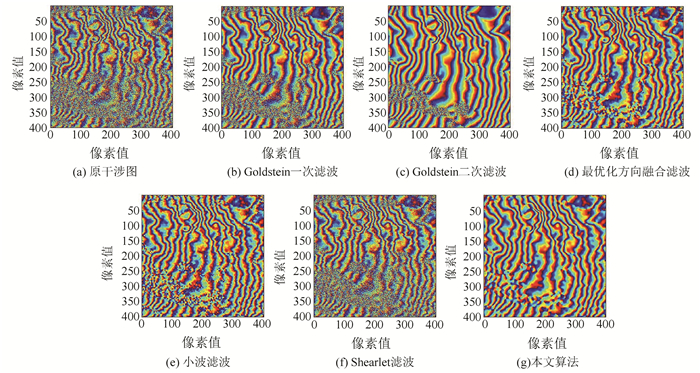
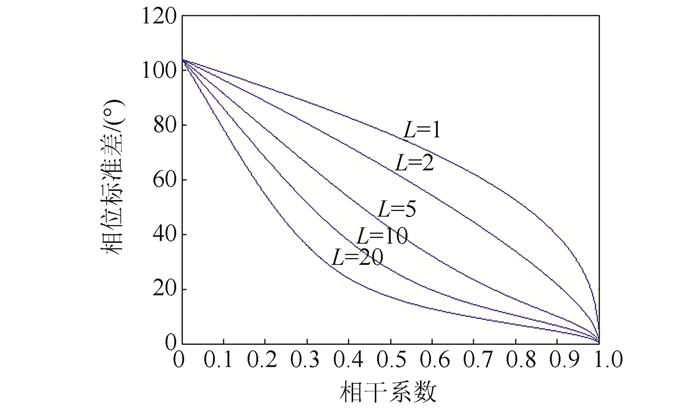
-
摘要: 干涉图小波阈值法滤波未考虑干涉相位统计特性, 导致在低相干区域得到的滤波效果不能令人满意。针对这一问题, 提出了一种剪切波变换与干涉相位标准差相结合的相位滤波算法。该算法将干涉相位统计特性与剪切波阈值滤波结合起来, 利用相位标准差改正滤波阈值以提高滤波效果。此外, 为了评价干涉图的滤波效果并为实测数据选择合适的滤波方法提供参考, 将模拟干涉图解缠结果的局部均方差分布作为滤波质量评价指标。将此算法与Goldstein滤波、小波滤波、最优方向融合滤波和剪切波软阈值滤波进行比较, 结果表明所提算法能更有效地削弱干涉图噪声, 同时保留干涉图的细节信息, 避免了低相干地区弱滤波问题。Abstract: In interferogram filtering, traditional threshold filtering algorithm based on wavelet transform does not consider the statistical properties of SAR's interference phase, and the filtering effect obtained in the low coherence regions is not satisfactory.This paper presents a kind of phase noise filtering algorithm combining the shearlet transform and standard deviation of phase.The algorithm uses the phase standard deviation to correct the filter threshold and improve the filtering effect.In addition, in order to evaluate the filtering effect and to select the appropriate filtering method for the measured data, a local mean square error distribution of the simulated interferogram is proposed as the filtering quality evaluation index.Compared with Goldstein filtering, wavelet filtering, optimal direction fusion filtering and shearlet soft threshold filtering, the results show that the proposed method can not only weaken the noise of interferogram, but also keep the details and avoid the weak filtering in low coherence regions.
-
Keywords:
- interferogram /
- shearlet /
- InSAR /
- filter
-
-
![]()
图 1 相位标准差与相干系数及视数的关系
Figure 1. Phase Standard Deviation Versus Coherence and Look Number
![]()
图 2 不同方法的模拟干涉图滤波结果比较
Figure 2. Comparison of Filtering Results of Simulated Interferogram by Different Methods
![]()
图 3 不同方法的模拟干涉图滤波结果评价直方图
Figure 3. Evaluation Histogram of Filtering Results of Simulated Interferogram by Different Methods
![]()
图 4 意大利Etna火山地区不同方法的干涉图滤波结果
Figure 4. Filtering Results of Interferogram over Etna Volcano in Italy by Different Methods
表 1 不同方法的模拟干涉图滤波结果定量比较
Table 1 Quantative Comparison of Filtering Results of Simulated Interferogram by Different Methods
滤波方法 正残差点数 负残差点数 RMS SPD PSD 无噪相位图 0 0 - 1.137 1×105 1.217 5×105 含噪相位图 16 070 16 074 3.347 3 4.919 6×105 6.731 3×105 Goldstein一次滤波 2 867 2 870 1.371 5 2.588 6×105 3.389 5×105 Goldstein二次滤波 90 90 1.283 8 0.729 6×105 0.780 0×105 最优化方向融合滤波 341 343 1.146 8 1.279 3×105 1.454 6×105 小波滤波 358 356 1.219 6 1.305 6×105 1.366 2×105 Shearlet滤波 12 901 12 908 1.734 4 4.380 7×105 5.930 2×105 本文算法 54 59 1.070 8 0.864 6×105 0.866 9×105 
下载: 导出CSV
表 2 不同方法的实测干涉图滤波结果定量比较
Table 2 Quantative Comparison of Filtering Results of Measured Interferogram by Different Methods
滤波方法 正残差点数 负残差点数 残差减少率/% SPD PSD 原干涉图 8 376 8 365 - 2.377 0×105 3.267 8×105 Goldstein一次滤波 4 640 4 638 44.58 1.655 5×105 2.181 5×105 Goldstein二次滤波 1 689 1 686 79.84 1.044 7×105 1.234 2×105 最优化方向融合滤波 341 342 95.92 8.839 6×104 9.592 7×104 小波滤波 505 505 93.96 9.563 5×104 1.016 1×105 Shearlet滤波 7 284 7 273 13.05 2.165 3×105 2.932 5×105 本文算法 143 147 98.27 7.674 1×104 7.859 8×104
下载: 导出CSV
-
[1] Song R, Guo H, Liu G, et al.Improved Goldstein SAR Interferogram Filter Based on Adaptive-Neighborhood Technique[J]. IEEE Geoscience & Remote Sensing Letters, 2014, 12(1):140-144 http://adsabs.harvard.edu/abs/2015IGRSL..12..140S
[2] Wang Y, Huang H, Dong Z, et al.Modified Patch-Based Locally Optimal Wiener Method for Interfero-metric SAR Phase Filtering[J].ISPRS Journal of Photogrammetry & Remote Sensing, 2016, 114(4):10-23 http://adsabs.harvard.edu/abs/2016JPRS..114...10W
[3] Lin X, Li F, Meng D, et al.Nonlocal SAR Interferometric Phase Filtering Through Higher Order Singular Value Decomposition[J].IEEE Geoscience & Remote Sensing Letters, 2015, 12(4):806-810 http://adsabs.harvard.edu/abs/2015IGRSL..12..806L
[4] Suo Z, Li M, Zhang Q, et al.InSAR Phase Noise Filter in Frequency Domain[J].IEEE Transactions on Geoscience & Remote Sensing, 2016, 54(2):1185-1195 doi: 10.1049/cp.2015.1050
[5] 靳国旺, 韩晓丁, 贾博, 等.InSAR干涉图的矢量分离式小波滤波[J].武汉大学学报·信息科学版, 2008, 33(2):132-135 http://ch.whu.edu.cn/CN/abstract/abstract1526.shtml Jin Guowang, Han Xiaoding, Jia Bo, et al.Filtering for InSAR Interferograms by Vector Decomposing and Wavelet Transformation[J].Geomatics and Information Science of Wuhan University, 2008, 33(2):132-135 http://ch.whu.edu.cn/CN/abstract/abstract1526.shtml
[6] 何儒云, 王耀南.一种基于小波变换的InSAR干涉图滤波方法[J].测绘学报, 2006, 35(2):128-132 http://www.cnki.com.cn/Article/CJFDTOTAL-CHXB200502003.htm He Ruyun, Wang Yaonan.InSAR Interferogram Filtering Based on Wavelet Transform[J].Acta Geodaetica et Cartographica Sinica, 2006, 35(2):128-132 http://www.cnki.com.cn/Article/CJFDTOTAL-CHXB200502003.htm
[7] Abdallah W B, Abdelfattah R.Two-Dimensional Wavelet Algorithm for Interferometric Synthetic Aperture Radar Phase Filtering Enhancement[J].Journal of Applied Remote Sensing, 2015, 9(1):1-17
[8] 范洪冬, 邓喀中, 庞蕾, 等.结合边缘信息的DT-CWT干涉图滤波算法[J].武汉大学学报·信息科学版, 2012, 37(7):810-813 http://ch.whu.edu.cn/CN/abstract/abstract257.shtml Fan Hongdong, Deng Kazhong, Pang Lei, et al.Interferogram Filtering Algorithm by Considering Edge Information in DT-CWT Domain[J].Geoma-tics and Information Science of Wuhan University, 2012, 37(7):810-813 http://ch.whu.edu.cn/CN/abstract/abstract257.shtml
[9] 高国荣, 许录平, 冯冬竹.利用非抽样Shearlet域GSM模型进行图像去噪[J].武汉大学学报·信息科学版, 2013, 38(7):778-782 http://ch.whu.edu.cn/CN/abstract/abstract2689.shtml Gao Guorong, Xu Luping, Feng Dongzhu.Image Denoising Based on the NSST Domain GSM Model[J].Geomatics and Information Science of Wuhan University, 2013, 38(7):778-782 http://ch.whu.edu.cn/CN/abstract/abstract2689.shtml
[10] Kutyniok G, Lim W Q.Compactly Supported Shearlets are Optimally Sparse[J].Journal of Approximation Theory, 2010, 163(11):1564-1589 http://arxiv.org/abs/1009.4359?context=math.FA
[11] Gupta D, Anand R S, Tyagi B.Speckle Filtering of Ultrasound Images Using a Modified Non-linear Diffusion Model in Non-subsampled Shearlet Domain[J].IEEE Transactions on Image Processing, 2015, 9(2):107-117 doi: 10.1049/iet-ipr.2014.0330
[12] Wang Q J, Zhang X H, Zhu J J, et al.Designing and Implementation of Variance-Dependent Goldstein Radar Interferogram Filtering[J].Journal of Central South University, 2014, 21(8):3295-3301 doi: 10.1007/s11771-014-2302-z
[13] Chen Y F, Xu H P.Comparisons of Speckle Noise Filtering Methods on Interferometric Synthetic Aperture Radar Images[J].Journal of Computers, 2014, 9(4):908-915 doi: 10.1080/02757259409532206
[14] 尹宏杰, 李志伟, 丁晓利, 等.InSAR干涉图最优化方向融合滤波[J].遥感学报, 2009, 13(6):1099-1113 doi: 10.11834/jrs.20090609 Yin Hongjie, Li Zhiwei, Ding Xiaoli, et al.Optimal Integration-Based Adaptive Direction Filter for InSAR Interferogram[J].Journal of Remote Sen-sing, 2009, 13(6):1099-1113 doi: 10.11834/jrs.20090609
[15] Patel V M, Easley G R, Chellappa R. Multiscale Directional Filtering of Noisy InSAR Phase Images[C]. The SPIE Conference on Independent Component Analyses, Wavelets, Neural Networks, Biosystems, and Nanoengineering, Orlando, Florida, USA, 2010
[16] Donoho D L.De-noising by Soft-Thresholding[J].IEEE Transactions on Information Theory, 2002, 41(3):613-627 http://citeseerx.ist.psu.edu/viewdoc/summary?doi=10.1.1.161.9704&rank=7
[17] Martinez C L, Canovas X F.SAR Interferometric Phase Noise Reduction Using Wavelet Transform[J].Electronics Letters, 2001, 37(10):649-651 doi: 10.1049/el:20010438
[18] Franceschetti G, Lanari R.Synthetic Aperture Radar Processing[M].2nd ed.Florida:CRC Press Inc, 2016
[19] Li Z W, Ding X L, Huang C, et al.Improved Fil-tering Parameter Determination for the Goldstein Radar Interferogram Filter[J].ISPRS Journal of Photogrammetry & Remote Sensing, 2008, 63(6):621-634 http://adsabs.harvard.edu/abs/2008JPRS...63..621L
计量
- 文章访问数:
- HTML全文浏览量:
- PDF下载量:
