多频多模GNSS高精度定位关键技术与挑战

李博峰; 苗维凯; 陈广鄂

doi:10.13203/j.whugis20230309

多频多模GNSS高精度定位关键技术与挑战

1.
同济大学测绘与地理信息学院, 上海, 200092

基金项目:

国家自然科学基金 42225401

上海市科委科技创新行动计划 21511103902

上海市科委科技创新行动计划 22511103003

上海市产业协同创新（科技）项目 XTCX-KJ-2023-35

上海市产业协同创新（科技）项目 XTCX-KJ-2022-09

详细信息

作者简介:
李博峰，博士，教授，研究方向为测量数据处理理论、GNSS精密定轨定位、多传感器融合综合PNT。bofeng_li@tongji.edu.cn

中图分类号: P228
计量
- 文章访问数: 853
- HTML全文浏览量: 1138
- PDF下载量: 357
出版历程
- 收稿日期: 2023-08-22
- 网络出版日期: 2023-10-09
- 刊出日期: 2023-11-04

Key Technologies and Challenges of Multi-frequency and Multi-GNSS High-Precision Positioning

College of Surveying and Geoinformatics, Tongji University, Shanghai 200092, China

摘要

摘要:
高精度全球导航卫星系统（global navigation satellite system，GNSS）定位技术拓展了位置服务行业的纵深发展，在智慧城市、智能交通和无人驾驶等领域得到了广泛应用，是当前卫星导航领域的热点。梳理了GNSS定位技术的现状和发展脉络，阐述多频多模GNSS高精度定位所涉及的整数模糊度固定、函数模型和随机模型融合等共性基础理论，介绍提升GNSS定位性能的非模型误差处理以及周跳探测与数据中断修复等关键技术。依托基础理论和关键技术的创新成果，介绍了同济大学GNSS团队近年来发展的4种多频多模GNSS高精度定位应用新技术，并展望了未来以GNSS为核心的高精度定位技术的发展趋势及其挑战。
- 整数模糊度 /
- 高精度定位 /
- 长距离RTK /
- 数据融合 /
- 海洋定位
Abstract:
High-precision global navigation satellite system(GNSS)positioning technology, as a hot spot in the field of satellite navigation, has expanded the in-depth development of the location-based service area, and has been widely used in smart cities, intelligent transportation, autonomous driving and other fields. First, this paper presents the current status and development of GNSS positioning technologies.Then, it explains the common basic theories such as integer ambiguity fixation, functional model and stochastic model fusion involved in multi-frequency multi-GNSS high-precision positioning, as well as the key techniques for improving GNSS positioning performance such as the non-model error processing, the cycle slip detection and data interruption repair. Based on the innovations of basic theories and key techniques, this paper introduces four new technologies for multi-frequency multi-GNSS high-precision positioning applications developed by Tongji University GNSS team in recent years. Finally, we remark the future development trend and challenges of GNSS-based high-precision positioning.
- integer ambiguity /
- high-precision positioning /
- long-distance RTK /
- data fusion /
- ocean positioning

HTML全文

电离层是日-地空间系统的重要组成部分，会对无线电波产生折射、散射、极化面旋转等影响，对基于无线电波的对地观测技术造成延迟误差，如GPS的电离层延迟^[1]、合成孔径雷达（synthetic aperture radar，SAR）的相位超前、群延迟以及法拉第旋转（Faraday rotation，FR）等^[2]。此外，电离层变化与地震也存在一定关系^[3-4]。因此，研究电离层不仅可以了解整个日-地空间系统，而且可以估计空间环境效应对无线电通信的影响。

总电子含量（total electron content，TEC）和电子密度是研究电离层的两个关键参数。TEC表示穿过电离层的单位截面柱体中电子数之和，反映了电离层的二维空间信息；而电子密度则描述了电离层三维空间结构信息，不仅包含电离层的水平结构信息，还包含了垂直结构信息。目前，GPS通过发射不同频率的信号获取垂直总电子含量（vertical total electron content，VTEC）^[5-6]；电离层与气候星座观测系统（the constellation observing system for meteorology，ionosphere，and climate，COSMIC）通过掩星事件获取电子密度剖线^[7]；非相干散射雷达（incoherent scattering radar，ISR）利用散射信号获取连续时间段内的电子密度剖线^[8]；电离层测高仪通过发射高频脉冲波并测量反射回波延迟获取F2层临界频率（foF2）等电离层参数^[9]；国际参考电离层（international reference ionosphere，IRI） ^[10]和差分整合移动平均自回归模型 ^[11]等也可获取VTEC和电子密度。然而，上述方法都存在空间分辨率不足的问题，而星载SAR凭借全天时、全天候、空间分辨率高等优点，已成为研究高空间分辨率电离层参数的重要手段。

文献[12]根据FR角与TEC之间的关系，利用全极化SAR数据计算了地磁北极及加科纳地区的TEC，证明了使用星载SAR数据反演TEC的可能性；文献[13]提出了利用距离分频法获取TEC的方法，将获取高空间分辨率TEC的方法拓展到单极化SAR领域；文献[14]提出了利用双波段路径延迟获取TEC的方法，进一步证实了分频法估计TEC的可行性；文献[15]利用全极化先进陆地观测卫星（advanced land observing satellite，ALOS）/相控合成孔径雷达（phased array type L-band synthetic aperture radar，PALSAR）数据，分别计算了高、中、低纬度地区的TEC分布，并分析了不同纬度的电离层特征；基于传统双波段路径延迟的方法，文献[16]提出了三波段路径延迟法，该方法可获取去除多重散射误差后的TEC；文献[17]提出了利用方位向子波段获取hmF2以及漂移速度场等电离层参数的方法，并使用ALOS-1/2数据进行实验，获取了相应的电离层参数结果；文献[18]使用全极化ALOS PALSAR数据反演了3组VTEC结果，并将其与ISR在几乎同一时空的实测数据进行比较，首次利用实测数据验证了利用全极化SAR反演VTEC的有效性。

目前利用SAR估计电离层参数只能获取二维的VTEC分布，难以获取精细化的三维电子密度分布。基于此，本文利用全极化SAR计算得到的VTEC联合IRI的电子密度剖线信息，提出了一种三维电子密度分布估计方法。

1 本文方法

本文方法的具体流程如图 1所示，首先利用全极化SAR数据计算FR角；然后根据得到的FR角结合磁场信息计算VTEC；最后联合IRI模型的电子密度剖线求解三维空间电子密度分布。

图 1 本文方法流程图

Figure 1. Flowchart of the Proposed Method

下载: 全尺寸图片幻灯片

1.1 利用全极化SAR数据计算FR角

对于全极化SAR系统，观测值散射矩阵M可表示为^[19]：

[\begin{matrix} M_{H H} & M_{V H} \\ M_{H V} & M_{V V} \end{matrix}] = A e^{j φ} [\begin{matrix} 1 & δ_{2} \\ δ_{1} & f_{1} \end{matrix}] [\begin{matrix} c o s Ω & s i n Ω \\ - s i n Ω & c o s Ω \end{matrix}] [\begin{matrix} S_{H H} & S_{V H} \\ S_{H V} & S_{V V} \end{matrix}] [\begin{matrix} c o s Ω & s i n Ω \\ - s i n Ω & c o s Ω \end{matrix}] [\begin{matrix} 1 & δ_{3} \\ δ_{4} & f_{2} \end{matrix}] + [\begin{matrix} N_{H H} & N_{V H} \\ N_{H V} & N_{V V} \end{matrix}]

式中， $A$ 为雷达系统的总体增益； $j$ 为复数； $φ$ 为双程相位延迟； $δ_{1} 、 δ_{2} 、 δ_{3} 、 δ_{4}$ 为因发射与接收的非完全独立性导致的串扰； $f_{1} 、 f_{2}$ 为通道失配； $Ω$ 为单程FR角； $S$ 为真实散射系数； $N$ 为附加噪声；角标代表不同极化方式，HH（horizontal horizontal）表示水平发射水平接收的同向极化方式，VV（vertical vertical）表示垂直发射垂直接收的同向极化方式，VH（vertical horizontal）表示垂直发射水平接收的交叉极化方式，HV（horizontal vertical）表示水平发射垂直接收的交叉极化方式。经系统误差改正后的观测值散射矩阵为^[20-21]：

\begin{matrix} [\begin{matrix} M_{H H} & M_{V H} \\ M_{H V} & M_{V V} \end{matrix}] = \\ [\begin{matrix} c o s Ω & s i n Ω \\ - s i n Ω & c o s Ω \end{matrix}] [\begin{matrix} S_{H H} & S_{V H} \\ S_{H V} & S_{V V} \end{matrix}] [\begin{matrix} c o s Ω & s i n Ω \\ - s i n Ω & c o s Ω \end{matrix}] \end{matrix}

根据真实散射矩阵的散射互易性（S_HV=S_VH），式（2）可变为：

\{\begin{array}{l} M_{H H} = S_{H H} c o s^{2} Ω - S_{V V} s i n^{2} Ω \\ M_{V H} = S_{H V} + (S_{H H} + S_{V V}) s i n Ω c o s Ω \\ M_{H V} = S_{H V} - (S_{H H} + S_{V V}) s i n Ω c o s Ω \\ M_{V V} = S_{V V} c o s^{2} Ω - S_{H H} s i n^{2} Ω \end{array}

由于法拉第旋转效应，观测值散射矩阵的 $M_{H V} \neq M_{V H}$ ，因此可根据式（3）解算FR角 $Ω$ 。由式（3）可导出多种FR角估计器^[22-24]，其中Bickel and Bates估计器最为稳定，计算如下：

Ω = \frac{1}{4} a r g (Z_{12} Z_{21}^{*}), - \frac{π}{4} < Ω < \frac{π}{4}

[\begin{matrix} Z_{11} & Z_{12} \\ Z_{21} & Z_{22} \end{matrix}] = [\begin{matrix} 1 & j \\ j & 1 \end{matrix}] [\begin{matrix} M_{H H} & M_{V H} \\ M_{H V} & M {}_{V V} \end{matrix}] [\begin{matrix} 1 & j \\ j & 1 \end{matrix}]

式中，arg（）表示取幅角函数；*表示共轭数。

1.2 由法拉第旋转角反演VTEC

SAR信号穿过电离层产生的FR角可表示为^[13]：

Ω = \frac{2.365 \times 10^{4}}{f^{2}} \int n_{e} B c o s θ d s

式中， $f$ 为信号频率； $n_{e}$ 为电子密度； $B$ 为磁场强度； $θ$ 为磁场与SAR信号夹角； $\int n_{e} d s$ 表示沿信号传播路径对电子密度积分。考虑到积分路径（信号传播路径）上 $B$ 近似成线性变化，且 $c o s θ$ 几乎不变，因此取路径上的平均磁场强度 $B_{0}$ 作为 $B$ ，式（6）可变为：

Ω = \frac{2.365 \times 10^{4}}{f^{2}} B_{0} c o s θ s e c ϕ V T E C

式中， $ϕ$ 为SAR入射角。对式（7）进行变换后得：

V T E C = Ω \frac{f^{2}}{2.365 \times 10^{4} \times B_{0} c o s θ s e c ϕ}

由此可知，在磁场信息已知的情况下，可由FR角计算VTEC。

1.3 联合IRI电子密度剖线估计三维空间电子密度分布

IRI是根据真实观测数据结合电离层模式形成的经验模型，可提供高时空分辨率的全球电离层参数。然而，由于观测数据相对较少，电离层特性不稳定，IRI模型计算得到的VTEC以及电子密度的精度不理想。由于SAR数据计算的VTEC具有较高的可靠性，因此本文基于SAR数据计算的VTEC对IRI模型的电子密度进行改正，得到较高可靠性的三维空间电子密度分布。IRI可提供全球60~2 000 km的电子密度，根据VTEC的定义，可得到IRI模型的VTEC，计算如下：

V T E C_{I R I} = \sum_{h = H_{m i n}}^{H_{m a x}} N (h)

式中， $V T E C_{I R I}$ 为IRI模型的VTEC； $N (h)$ 为IRI高度为 $h$ （单位：km）处的电子密度； $H_{m a x}$ 、 $H_{m i n}$ 分别为计算VTEC时的最大、最小高度。由于IRI提供的电子密度存在误差，从而导致估计的VTEC也存在误差。本文利用SAR数据计算的VTEC对IRI电子密度进行改正，计算如下：

\hat{N} (h) = N (h) \times \frac{V T E C_{S A R}}{V T E C_{I R I}}

式中， $\hat{N} (h)$ 为改正后高度h（单位：km）处的电子密度； $V T E C_{S A R}$ 为SAR数据计算的VTEC。为了验证改正方法的合理性，对改正后的电子密度求和得到：

\sum_{h = H_{m i n}}^{H_{m a x}} \hat{N} (h) = \frac{V T E C_{S A R}}{V T E C_{I R I}} \sum_{h = H_{m i n}}^{H_{m a x}} N (h)

将式（9）代入式（11）中得到：

\sum_{h = H_{m i n}}^{H_{m a x}} \hat{N} (h) = V T E C_{S A R}

综上，经改正后，IRI模型VTEC与SAR计算的VTEC相等。

2 实验结果

2.1 数据及研究区域

为了验证本文方法的可行性，利用全极化的ALOS-1/PALSAR数据进行实验。实验区域位于美国阿拉斯加地区，分别采用升轨（实验一）和降轨（实验二）数据来验证VTEC和电子密度结果，数据详细参数如表 1所示。

表 1 实验使用的SAR影像

Table 1. SAR Images Used in the Experiment

实验	日期	入射角 $ϕ$ /(°)	飞行方向	轨道高度/km	轨道号	影像号	采集时间
实验一	2007-04-01	23.94	升轨	695	243	1250~1260	07:28
实验二	2009-08-03	23.98	降轨	695	618	2270~2310	21:08

下载: 导出CSV

| 显示表格

数据覆盖范围如图 2所示，褐色矩形为实验一研究区域，黑色矩形为实验二研究区域，红色星号为ISR测站（Poker Flat测站），红色三角形为GPS观测站所在的费尔班克斯城。为了验证实验结果，收集了SAR对应时间和位置的ISR数据。

图 2 研究区域

Figure 2. Study Area

下载: 全尺寸图片幻灯片

2.2 利用SAR数据计算FR角

本文利用收集到的全极化数据计算FR角，首先，读取全极化SAR数据并构建观测值散射矩阵M；其次，采用Bickel and Bates FR角估计器计算FR角，根据式（5）计算 $Z_{12} Z_{21}^{*}$ ；然后，对计算的 $Z_{12} Z_{21}^{*}$ 进行多视处理以减小运算量，本文实验中多视比距离向为2，方位向为14；最后，利用自适应光谱滤波器对多视后的 $Z_{12} Z_{21}^{*}$ 进行滤波，并根据式（4）计算FR角，结果如图 3所示。

图 3 研究区法拉第旋转角分布

Figure 3. Distribution of FR Angles in Study Area

下载: 全尺寸图片幻灯片

图 3（a）为实验一FR角分布图，可以看出明显的FR角异常现象。对数据进行统计可知FR角最大值为13.4°，最小值为3.2°，平均值为6.1°。根据法拉第效应分析，该研究区域上空的磁场和电离层的共同作用使得SAR信号发生了极化面的旋转，从而产生了FR角。图 3（b）为实验二FR角分布图。对FR角进行统计分析发现FR角最小值为2°，最大值为2.2°，平均值为2.1°。该研究区域FR角较为稳定，变化区间大小仅为0.2°，推断实验二没有电离层异常事件。

2.3 计算VTEC

首先，基于FR角，可由式（8）计算VTEC分布，获取国际参考地磁场（international geomagnetic reference field，IGRF）提供的磁场强度、磁倾角以及磁偏角，由于ALOS卫星飞行高度为700 km左右，地磁场在高度向近似成线性变化，且分析电离层模型得出电离层通常在300 km左右电子密度达到峰值，本文使用高度为300 km的值作为磁场信息平均值；其次，利用数字高程模型（digital elevation model，DEM）和SAR成像参数计算SAR入射角 $ϕ$ 的分布图；然后，将SAR入射角 $ϕ$ 以及磁场信息转换到SAR坐标系下，并计算磁场与SAR入射角 $ϕ$ 之间的夹角 $θ$ 的分布；最后，根据式（8）计算研究区域VTEC分布图，结果如图 4所示。

图 4 研究区域VTEC分布图

Figure 4. Distribution of VTEC in Study Area

下载: 全尺寸图片幻灯片

从图 4可以看出，实验一的VTEC最大值为33.4 TECU，最小值为7.9 TECU，平均值为15.2 TECU；实验二的VTEC最大值为5.3 TECU，最小值为4.8 TECU，平均值为5.2 TECU。结合图 3可以看出，VTEC分布与FR角分布趋势相似。这是因为VTEC由FR角和磁场信息共同得到，且本实验中，磁场信息的变化相对于FR角的变化较小，因此得到的VTEC和FR角高度相关。在实验一中，VTEC变化区间较大，VTEC变化可达20 TECU左右，推测存在电离层异常事件；在实验二中，VTEC变化较小，仅为0.5 TECU，不存在电离层异常事件。但在图 4（d）中，方位向4 000行左右出现了一个较小的沟壑，通过查看研究区域的卫星影像发现该位置为费尔班克斯城的水域，推测为该水域影响了SAR信号后向散射系数，导致VTEC出现误差。

2.4 联合IRI估计三维电子密度分布

首先根据IRI模型获取研究区域的电子密度剖线；然后由SAR数据计算的VTEC对电子密度剖线进行改正，从而获得整个区域三维电子密度分布。在计算IRI VTEC时，IRI模型的输入值为时间、经纬度以及高度范围，其中时间为2009年年积日第215天21：08，经纬度与实验二SAR数据覆盖范围相同，高度范围为80~700 km。IRI模型输出值为对应时空80~700 km的电子密度，将IRI模型输出的电子密度相加可获取VTEC。由于SAR飞行高度约为700 km，假设空间中每千米的电子密度分布均匀，且电离层通常位于80 km上方，因此式（10）中h为80~700 km，按每千米分层，约620层。考虑到后续验证的需要，这里只显示了实验二的三维电子密度。

图 5为实验二不同高度处的电子密度分布。由图 5可知，电子密度在300 km和400 km处较大，在其他高度则较小。

图 5 实验二的三维电子密度分布

Figure 5. Estimated 3D Electron Density Distribution in Experiment 2

下载: 全尺寸图片幻灯片

图 6为图 5中AA'连线的剖线，反映了不同高度的电子密度在方位向的变化趋势。由图 6可知，研究区域不同高度的电子密度在方位向有着相似的变化趋势，且该趋势近似于图 4（d）的VTEC变化趋势；同时由剖线可知，VTEC中的沟壑现象同样出现在了电子密度剖线中；在本文方法中，电子密度结果与使用的VTEC密切相关。

图 6 不同高度处水平电子密度剖线

Figure 6. Profiles of Electron Density at Different Altitudes

下载: 全尺寸图片幻灯片

3 误差分析

3.1 VTEC结果验证

为验证FR角估计VTEC的可靠性，分别利用GPS和ISR数据验证实验一和实验二结果。实验一中SAR获取位置和时刻无对应ISR数据，因此使用附近的GPS数据进行验证。图 7（a）为GPS在实验一SAR获取时间段内的轨迹，红色矩形为实验一研究区域，红色五角星为GPS测站，图 7（b）为GPS估计的VTEC数据，棕色虚线为SAR数据采集时间。由图 7（b）可知，在07：30左右，GPS VTEC突然上升，验证了之前对电离层异常的推测。此外，GPS在SAR采集时间的VTEC为18.3 TECU，SAR数据VTEC平均值为15.2 TECU，差值为3.1 VTEC。两者虽有一定差距，但考虑到GPS数据在时间和空间上与SAR数据范围并未完全对应，会导致两者存在误差，可以认为两者在电离层扰动情况下计算的VTEC有相当的一致性。对于实验二，SAR获取位置和时刻附近GPS轨迹相隔较远，因此利用精度更高的ISR数据进行验证。对实验二SAR卫星时刻的ISR电子密度进行积分，计算出ISR VTEC为5.5 TECU，SAR VTEC为5.3 TECU，两者相差0.2 TECU，进一步验证了SAR估计VTEC的可靠性。

图 7 GPS飞行轨迹以及实验一得到的VTEC

Figure 7. GPS Flight Trajectory and GPS-Derived VTEC at SAR-Acquired Time in Experiment 1

下载: 全尺寸图片幻灯片

3.2 三维电子密度结果验证

为验证本文方法估计三维电子密度的可靠性，使用ISR实测的电子密度对三维电子密度结果进行验证。由于在实验一的研究区域及时间没有对应的ISR数据，因此使用Poker Flat测站2009年年积日第215天21：06 ISR的电子密度数据验证实验二的电子密度结果。考虑到ISR数据与实验二SAR数据采集时间仅相差2 min，且该时间段电离层没有异常活动，因此认为可将ISR电子密度数据作为真实的电子密度结果与本文结果进行比较分析，结果如图 8所示。

图 8 SAR估计的电子密度误差分布

Figure 8. Errors Distribution of Electron Density Estimated by SAR

下载: 全尺寸图片幻灯片

图 8（a）为ISR、IRI及本文方法获取的电子密度数据，其中蓝色菱形符号为ISR数据，红色实线为IRI电子密度剖线，棕色虚线为经本文方法改正后的电子密度剖线。由图 8（a）可知，改正前IRI的电子密度普遍高于ISR电子密度，推测原因为IRI的VTEC估值偏高导致其电子密度也偏高。在改正后，电子密度偏高现象得到明显改善，分析原因为SAR数据计算的VTEC与真实的VTEC更为接近，因此使用SAR计算的VTEC进行改正后改善了原来电子密度偏高的现象。对误差进行统计发现，改正前IRI电子密度误差和为 $8.14 \times 10^{11} / m^{3}$ ，改正后电子密度误差和为 $5.41 \times 10^{11} / m^{3}$ ，整体误差减少了33.57%。

为进一步对比不同高度处的电子密度精度，将ISR获取的电子密度作为真值，计算了IRI与本文方法的误差，如图 8（b）所示，红色条形图为IRI与ISR电子密度差值的绝对值，蓝色条形图为本文方法与ISR电子密度差值的绝对值。由图 8（b）可知，对于低于地球表面133 km部分的电子密度结果，本文方法获取的电子密度误差更大；对于高于地球表面133 km部分的电子密度结果，本文方法获取的电子密度精度明显高于IRI模型。统计结果发现，IRI在133 km以下误差为7.2×10¹⁰/m³，133 km以上误差为7.42×10¹¹/m³；本文方法133 km以下误差为1.55×10¹¹/m³，133 km以上误差为3.86×10¹¹/m³，误差减少了47.98%。通过查询相关资料后发现，IRI模型在低空部分使用的是垂测站和火箭探测的数据，而在高空部分使用的是卫星探测数据^[25]。再考虑到在研究区域附近存在ELELSON垂测站，因此推测在该研究区域IRI模型低空部分的电子密度本身可靠性较高，而本文方法改正时对所有高度进行了等比例的调整，导致低空部分改正后误差增大。

4 结语

本文提出一种利用全极化SAR数据结合IRI模型估计高空间分辨率三维电子密度分布的方法，并选择覆盖阿拉斯加地区的ALOS-1全极化数据进行实验，对结果进行分析得出如下结论：

1）利用全极化SAR能够获取高空间分辨率的VTEC分布。基于FR角与VTEC之间的关系，利用全极化SAR数据计算了研究区域VTEC分布，并利用GPS和ISR数据验证可靠性。结果发现，实验一获取的含有电离层异常扰动的VTEC与GPS估计的VTEC相差3.1 TECU，实验二利用SAR估计的VTEC与ISR的VTEC相差0.2 TECU，证明了利用全极化SAR估计VTEC分布的可靠性。

2）基于SAR获取了高精度VTEC，并联合IRI电子密度剖线获取了研究区域三维电子密度分布。结果发现，在不同高度处的三维电子密度不同，300~400 km处较大，而在其他高度处相对较小，且不同高度的电子密度在水平方向具有相似的分布趋势。将结果与ISR数据对比后发现，本文方法获取的电子密度在整体误差上比IRI模型少33.57%，在低于133 km高度的误差比IRI模型大，在高于133 km高度的误差比IRI模型少47.98%，这与IRI模型所使用的数据相关。

图 1 GNSS定位技术的发展历程

Figure 1. Development of GNSS-based Positioning Concepts

下载: 全尺寸图片幻灯片

图 2 宽巷模糊度和双频窄巷模糊度N₁、N₂的分布图

Figure 2. Scatterplot of Wideline and Narrowlane N₁ and N₂ Ambiguities for Two Frequencies

下载: 全尺寸图片幻灯片

图 3 TRS和PPF周跳探测方法的RTK水平定位效果

Figure 3. RTK Horizontal Results with TRS and PPF Methods for ublox Dataset

下载: 全尺寸图片幻灯片

图 4 ORTK系统原型^[84]

Figure 4. Diagram of ORTK with BeiDou SMC^[84]

下载: 全尺寸图片幻灯片

图 5 海上动态定位实验与结果

Figure 5. ORTK Kinematic Experiment and Result

下载: 全尺寸图片幻灯片

图 6 松组合RTK与紧组合RTK原理示意图

Figure 6. Schematic Illustration of Loosely Combined RTK and TRTK

下载: 全尺寸图片幻灯片

图 7 GPS/BDS/Galileo/QZSS重叠频率松、紧组合RTK动态定位

Figure 7. GPS/BDS/Galileo/QZSS Loosely and Tightly Combined RTK Kinematic Positioning Results with Overlapping Frequencies

下载: 全尺寸图片幻灯片

图 8 240颗低轨卫星混合轨道倾角的星座优化设计

Figure 8. Constellation Optimization Design for 240 LEO Satellites with Mixed Orbital Inclinations

下载: 全尺寸图片幻灯片

图 9 城市场景示意图和不同区域下不同连续跟踪时长的卫星数

Figure 9. Schematic Overview of the Urban Canyon and the Number of Satellites with Different Continuous Tracking Durations Across Various Regions

下载: 全尺寸图片幻灯片

参考文献(101)

[1]	杨元喜. 综合PNT体系及其关键技术[J]. 测绘学报, 2016, 45(5): 505-510. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB201605001.htm Yang Yuanxi. Concepts of Comprehensive PNT and Related Key Technologies[J]. Acta Geodaetica et Cartographica Sinica, 2016, 45(5): 505-510. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB201605001.htm
[2]	Teunissen P, Montenbruck O. Springer Handbook of Global Navigation Satellite Systems[M]. Cham: Springer International Publishing, 2017.
[3]	Leick A. GPS Satellite Surveying[M]. 3rd ed. New York: John Wiley, 2004.
[4]	Hofmann-Wellenhof B, Lichtenegger H, Collins J. Global Positioning System: Theory and Practice[M]. 5th ed. New York: Springer Wien, 2001.
[5]	Verhagen S. The GNSS Integer Ambiguities: Estimation and Validation[D]. Delft : Delft University of Technology, 2004.
[6]	Teunissen P. Towards a Unified Theory of GPS Ambiguity Resolution[J]. Journal of Global Positioning Systems, 2003, 2(1): 1-12. doi: 10.5081/jgps.2.1.1
[7]	Feng Y, Li B. Three Carrier Ambiguity Resolution: Generalised Problems, Models, Methods and Performance Analysis Using Semi-generated Triple Frequency GPS Data[C]//ION GNSS, Savannah, USA, 2008.
[8]	Teunissen P. Integer Estimation in the Presence of Biases[J]. Journal of Geodesy, 2001, 75(7): 399-407.
[9]	Li B, Shen Y, Feng Y. Fast GNSS Ambiguity Resolution as an Ill-posed Problem[J]. Journal of Geodesy, 2010, 84(11): 683-698. doi: 10.1007/s00190-010-0403-5
[10]	Li B, Qin Y, Liu T. Geometry-based Cycle Slip and Data Gap Repair for Multi-GNSS and Multi-frequency Observations[J]. Journal of Geodesy, 2019, 93(3): 399-417. doi: 10.1007/s00190-018-1168-5
[11]	杨元喜. 弹性PNT基本框架[J]. 测绘学报, 2018, 47(7): 893-898. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB201807002.htm Yang Yuanxi. Resilient PNT Concept Frame[J]. Acta Geodaetica et Cartographica Sinica, 2018, 47(7): 893-898. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB201807002.htm
[12]	Shin E. Estimation Techniques for Low-Cost Inertial Navigation[D]. Calgary: University of Calgary, 2005.
[13]	张治腾. 多频多系统GNSS长距离RTK理论与方法[D]. 上海: 同济大学, 2021. Zhang Zhiteng. Multi-frequency and Multi-GNSS Long-Range RTK Theory and Methods[D]. Shanghai: Tongji University, 2021.
[14]	Ge H, Li B, Jia S, et al. LEO Enhanced Global Navigation Satellite System (LeGNSS): Progress, Opportunities, and Challenges[J]. Geo⁃spatial Information Science, 2022, 25(1): 1-13.
[15]	张小红, 马福建. 低轨导航增强GNSS发展综述[J]. 测绘学报, 2019, 48(9): 1073-1087. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB201909002.htm Zhang Xiaohong, Ma Fujian. Review of the Development of LEO Navigation-Augmented GNSS[J]. Acta Geodaetica et Cartographica Sinica, 2019, 48(9): 1073-1087. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB201909002.htm
[16]	Rogers R. Applied Mathematics in Integrated Navigation Systems[M]. 3rd ed. Reston, VA, USA: AIAA, 2007.
[17]	Bock Y, Abbot R, Counselman C, et al. Geodetic Accuracy of the Macrometer Model V-1000[J]. Bulletin Geodesique, 1984, 58(2): 211-221. doi: 10.1007/BF02520902
[18]	Bock Y, Abbot R, Counselman C, et al. Three-dimensional Geodetic Control by Interferometry with GPS: Processing of GPS Phase Observables[C]// The 1st International Symposium on Precise Positioning with the Global Positioning System, Rockville, Maryland, 1985.
[19]	Edwards S, Cross P, Barnes J, et al. A Methodology for Benchmarking Real-Time Kinematic GPS[J]. Survey Review, 1999, 35(273): 163-174. doi: 10.1179/sre.1999.35.273.163
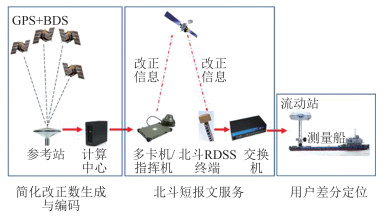
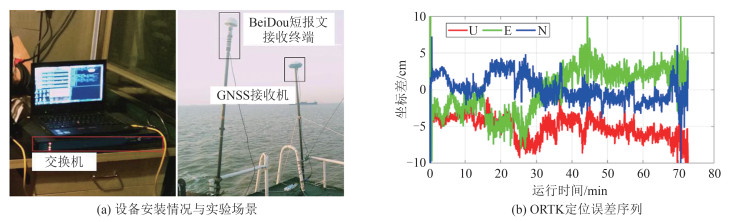
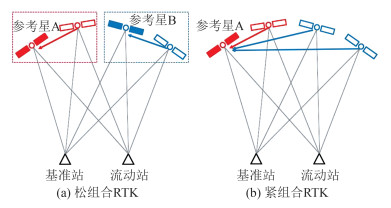
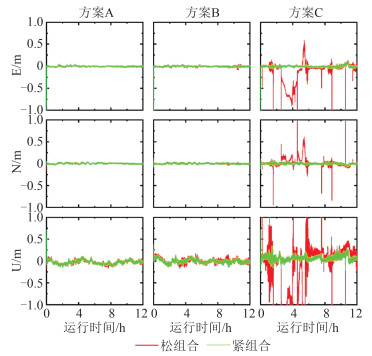
[20]	Han S. Carrier Phase-Based Long-Range GPS Kinematic Positioning[D]. Sydney, Australia : UNSW University, 1997.
[21]	Wanninger L. Improved Ambiguity Resolution by Regional Differential Modelling of the Ionosphere[C]//The 8th International Technical Meeting of the Satellite Division of The Institute of Navigation, Palm, USA, 1995.
[22]	高星伟. GPS/GLONASS网络RTK的算法研究与程序实现[D]. 武汉: 武汉大学, 2002. Gao Xingwei. The Algorithmic Research of GPS/GLONASS Network RTK and Its Program Realization[D]. Wuhan: Wuhan University, 2002.
[23]	Blewitt G. Carrier Phase Ambiguity Resolution for the Global Positioning System Applied to Geodetic Baselines up to 2 000 km[J]. Journal of Geophysical Research, 1989, 94(B8): 10187-10203. doi: 10.1029/JB094iB08p10187
[24]	Trimble. Trimble - VRS[OL]. [2010-01-21]. http://www.trimble.com/vrs.shtml.
[25]	Wübbena G, Bagge A, Seeber G, et al. Reducing Distance Dependent Errors for Real-Time Precise DGPS Applications by Establishing Reference Station Networks[C]//The 9th International Technical Meeting of the Satellite Division of the Institute of Navigation (ION GPS 1996), CityKansas, MO, 1996.
[26]	Leica Geosystems. White Paper: GPS SpiderNET - Take It to the MAX[OL]. [2005-03-22]. http://www.leica-geosystems.com.
[27]	Kouba J, Mireault Y, Lahaye F. IGS Orbit/Clock Combination and Evaluation[C]//International GPS Service for Geodynamics (IGS), California, USA, 1995.
[28]	Zumberge J, Heflin M, Jefferson D, et al. Precise Point Positioning for the Efficient and Robust Analysis of GPS Data from Large Networks[J]. Journal of Geophysical Research, 1997, 102(B3): 5005-5017. doi: 10.1029/96JB03860
[29]	刘经南, 叶世榕. GPS非差相位精密单点定位技术探讨[J]. 武汉大学学报(信息科学版), 2002, 27(3): 234-240. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH200203001.htm Liu Jingnan, Ye Shirong. GPS Precise Point Positioning Using Undifferenced Phase Observation[J]. Geomatics and Information Science of Wuhan University, 2002, 27(3): 234-240. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH200203001.htm
[30]	Ge M, Gendt G, Rothacher M, et al. Resolution of GPS Carrier-Phase Ambiguities in Precise Point Positioning (PPP) with Daily Observations[J]. Journal of Geodesy, 2008, 82(7): 389-399. doi: 10.1007/s00190-007-0187-4
[31]	Laurichesse D, Mercier F, Berthias J, et al. Integer Ambiguity Resolution on Undifferenced GPS Phase Measurements and Its Application to PPP and Satellite Precise Orbit Determination[J]. Annual of Navigation, 2007, 56(2): 135-149.
[32]	Collins P. Isolating and Estimating Undifferenced GPS Integer Ambiguities[C]//The 2008 National Technical Meeting of the Institute of Navigation, San Diego, USA, 2008.
[33]	Geng J, Chen X, Pan Y, et al. A Modified Phase Clock/Bias Model to Improve PPP Ambiguity Resolution at Wuhan University[J]. Journal of Geodesy, 2019, 93(10): 2053-2067. doi: 10.1007/s00190-019-01301-6
[34]	Wang S, Li B, Li X, et al. Performance Analysis of PPP Ambiguity Resolution with UPD Products Estimated from Different Scales of Reference Station Networks[J]. Advances in Space Research, 2018, 61(1): 385-401. doi: 10.1016/j.asr.2017.09.005
[35]	姚宜斌, 彭文飞, 孔建, 等. 精密单点定位模糊度固定效果分析[J]. 武汉大学学报(信息科学版), 2013, 38(11): 1281-1285. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201311006.htm Yao Yibin, Peng Wenfei, Kong Jian, et al. Analysis of Ambiguity Fixing in Precise Point Positioning[J]. Geomatics and Information Science of Wuhan University, 2013, 38(11): 1281-1285. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201311006.htm
[36]	Zhang X, Li X, Guo F. Satellite Clock Estimation at 1Hz for Real Time Kinematic PPP Applications[J]. GPS Solutions, 2011, 15(4): 315-324. doi: 10.1007/s10291-010-0191-7
[37]	Li X, Ge M, Dai X, et al. Accuracy and Reliability of Multi-GNSS Real-Time Precise Positioning: GPS, GLONASS, BeiDou, and Galileo[J]. Journal of Geodesy, 2015, 89(6): 607-635. doi: 10.1007/s00190-015-0802-8
[38]	姚宜斌, 冯鑫滢, 彭文杰, 等. 基于CORS的区域大气增强产品对实时PPP的影响[J]. 武汉大学学报(信息科学版), 2019, 44(12): 1739-1748. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201912001.htm Yao Yibin, Feng Xinying, Peng Wenjie, et al. Local Atmosphere Augmentation Based on CORS for Real-Time PPP[J]. Geomatics and Information Science of Wuhan University, 2019, 44(12): 1739-1748. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201912001.htm
[39]	Teunissen P, Khodabandeh A. Review and Principles of PPP-RTK Methods[J]. Journal of Geodesy, 2015, 89(3): 217-240. doi: 10.1007/s00190-014-0771-3
[40]	Wabbena G, Schmitz M, Bagge A. PPP-RTK: Precise Point Positioning Using State-Space Representation in RTK Networks[C]//The 18th International Technical Meeting of the Satellite Division of The Institute of Navigation, Long Beach, USA, 2005.
[41]	李博峰. 混合整数GNSS函数模型及随机模型参数估计理论与方法[D]. 上海: 同济大学, 2010. Li Bofeng. Theory and Method of Parameter Estimation for Mixed Integer GNSS Stochastic and Function Models[D]. Shanghai: Tongji University, 2010.
[42]	Teunissen P. An Optimality Property of the Integer Least-Squares Estimator[J]. Journal of Geodesy, 1999, 73(11): 587-593. doi: 10.1007/s001900050269
[43]	Xu P. Voronoi Cells, Probabilistic Bounds and Hypothesis Testing in Mixed Integer Linear Models[J]. IEEE Transactions on Information Theory, 2006, 52(7): 3122-3138. doi: 10.1109/TIT.2006.876356
[44]	Teunissen P. The Least-Squares Ambiguity Decorrelation Adjustment: A Method for Fast GPS Integer Ambiguity Estimation[J]. Journal of Geodesy, 1995, 70(1): 65-82.
[45]	Chang X, Yang X, Zhou T. MLAMBDA: A Modified LAMBDA Algorithm for Integer Least-Squares Estimation[J]. Journal of Geodesy, 2005, 79(9): 552-565. doi: 10.1007/s00190-005-0004-x
[46]	Xu P. Random Simulation and GPS Decorrelation[J]. Journal of Geodesy, 2001, 75(7): 408-423.
[47]	Teunissen P. Integer Aperture GNSS Ambiguity Resolution[J]. Artificial Satellites, 2003, 38(3): 79-88.
[48]	Teunissen P. GNSS Ambiguity Resolution with Optimally Controlled Failure-Rate[J]. Artificial Satellites, 2005, 40(4): 219-227.
[49]	Teunissen P. Theory of Integer Equivariant Estimation with Application to GNSS[J]. Journal of Geodesy, 2003, 77(7): 402-410.
[50]	Teunissen P. Best Integer Equivariant Estimation for Elliptically Contoured Distributions[J]. Journal of Geodesy, 2020, 94(9): 82. doi: 10.1007/s00190-020-01407-2
[51]	Shen Y, Li B. Regularized Solution to Fast GPS Ambiguity Resolution[J]. Journal of Survey Engineering, 2007, 133(4): 168-172. doi: 10.1061/(ASCE)0733-9453(2007)133:4(168)
[52]	李博峰, 沈云中. P范分布混合整数模型参数估计理论[J]. 测绘学报, 2010, 39(2): 141-145. Li Bofeng, Shen Yunzhong. Maximum Likelihood Estimation in Mixed Integer Linear Model with p-norm Distribution[J]. Acta Geodaetica et Cartographica Sinica, 2010, 39(2): 141-145.
[53]	Li B, Zhang Z, Miao W, et al. Improved Precise Positioning with BDS-3 Quad-Frequency Signals[J]. Satellite Navigation, 2020, 1(1): 1-10. doi: 10.1186/s43020-019-0006-0
[54]	Feng Y, Li B. A Benefit of Multiple Carrier GNSS Signals: Regional Scale Network-Based RTK with Doubled Inter-Station Distances[J]. Journal of Spatial Science, 2008, 53(2): 135-147. doi: 10.1080/14498596.2008.9635154
[55]	Li B. Review of Triple-Frequency GNSS: Ambiguity Resolution, Benefits and Challenges[J]. The Journal of Global Positioning Systems, 2018, 16(1): 1-15. doi: 10.1186/s41445-018-0010-y
[56]	Li B, Feng Y, Shen Y. Three Carrier Ambiguity Resolution: Distance-Independent Performance Demonstrated Using Semi-Generated Triple Frequency GPS Signals[J]. GPS Solutions, 2010, 14(2): 177-184. doi: 10.1007/s10291-009-0131-6
[57]	Li B, Feng Y, Gao W, et al. Real-Time Kinematic Positioning over Long Baselines Using Triple-Frequency BeiDou Signals[J]. IEEE Transactions on Aerospace and Electronic Systems, 2015, 51(4): 3254-3269. doi: 10.1109/TAES.2015.140643
[58]	Feng Y. GNSS Three Carrier Ambiguity Resolution Using Ionosphere-Reduced Virtual Signals[J]. Journal of Geodesy, 2008, 82(12): 847-862. doi: 10.1007/s00190-008-0209-x
[59]	Parkins A. Increasing GNSS RTK Availability with a New Single-epoch Batch Partial Ambiguity Resolution Algorithm[J]. GPS Solutions, 2011, 15(4): 391-402. doi: 10.1007/s10291-010-0198-0
[60]	Wang J, Feng Y. Reliability of Partial Ambiguity Fixing with Multiple GNSS Constellations[J]. Journal of Geodesy, 2013, 87(1): 1-14. doi: 10.1007/s00190-012-0573-4
[61]	Teunissen P, Odolinski R, Odijk D. Instantaneous BeiDou+GPS RTK Positioning with High Cut-off Elevation Angles[J]. Journal of Geodesy, 2014, 88(4): 335-350. doi: 10.1007/s00190-013-0686-4
[62]	Li B, Shen Y, Feng Y, et al. GNSS Ambiguity Resolution with Controllable Failure Rate for Long Baseline Network RTK[J]. Journal of Geodesy, 2014, 88(2): 99-112. doi: 10.1007/s00190-013-0670-z
[63]	李博峰, 沈云中, 张兴福. 纳伪概率可控的四舍五入法及其在RTK模糊度固定中的应用[J]. 测绘学报, 2012, 41(4): 483-489. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB201204004.htm Li Bofeng, Shen Yunzhong, Zhang Xingfu. Error Probability Controllable Integer Rounding Method and Its Application to RTK Ambiguity Resolution[J]. Acta Geodaetica et Cartographica Sinica, 2012, 41(4): 483-489. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB201204004.htm
[64]	Li B, Lou L, Shen Y. GNSS Elevation-Dependent Stochastic Modeling and Its Impacts on the Statistic Testing[J]. Journal of Surveying Engineering, 2016, 142(2): 04015012. doi: 10.1061/(ASCE)SU.1943-5428.0000156
[65]	Li B, Zhang L, Verhagen S. Impacts of BeiDou Stochastic Model on Reliability: Overall Test, w-test and Minimal Detectable Bias[J]. GPS Solutions, 2017, 21(3): 1095-1112. doi: 10.1007/s10291-016-0596-z
[66]	Tian Y, Ge M, Neitzel F, et al. Multi-Dimensional Particle Filter-Based Estimation of Inter-System Phase Biases for Multi-GNSS Real-Time Integer Ambiguity Resolution [J]. Journal of Geodesy, 2019, 93: 1073-1087. doi: 10.1007/s00190-018-01226-6
[67]	Zhang B, Liu T, Yuan Y. GPS Receiver Phase Biases Estimable in PPP-RTK Networks: Dynamic Characterization and Impact Analysis[J]. Journal of Geodesy, 2018, 92(6): 659-674. doi: 10.1007/s00190-017-1085-z
[68]	Geng J, Wen Q, Zhang Q, et al. GNSS Observable-specific Phase Biases for All-Frequency PPP Ambiguity Resolution[J]. Journal of Geodesy, 2022, 96(2): 11. doi: 10.1007/s00190-022-01602-3
[69]	Zang N, Li B, Nie L, et al. Inter-System and Inter-Frequency Code Biases: Simultaneous Estimation, Daily Stability and Applications in Multi-GNSS Single-Frequency Precise Point Positioning [J]. GPS Solutions, 2019, 24(1): 18.
[70]	Li B, Zang N, Ge H, et al. Single-Frequency PPP Models: Analytical and Numerical Comparison[J]. Journal of Geodesy, 2019, 93(12): 2499-2514. doi: 10.1007/s00190-019-01311-4
[71]	Teunissen P, Amiri-Simkooei A. Least-Squares Variance Component Estimation[J]. Journal of Geodesy, 2008, 82(2): 65-82. doi: 10.1007/s00190-007-0157-x
[72]	李博峰, 沈云中, 楼立志. 基于等效残差的方差-协方差分量估计[J]. 测绘学报, 2010, 39(4): 349-354. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB201101005.htm Li Bofeng, Shen Yunzhong, Lou Lizhi. Variance-Covariance Component Estimation Based on the Equivalent Residuals[J]. Acta Geodaetica et Cartographica Sinica, 2010, 39(4): 349-354. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB201101005.htm
[73]	李博峰, 沈云中. 基于等效残差积探测粗差的方差-协方差分量估计[J]. 测绘学报, 2011, 40(1): 10-14. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB201101005.htm Li Bofeng, Shen Yunzhong. Equivalent Residual Product Based Outlier Detection for Variance and Covariance Component Estimation[J]. Acta Geodaetica et Cartographica Sinica, 2011, 40(1): 10-14. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB201101005.htm
[74]	Li B, Shen Y, Lou L. Efficient Estimation of Variance and Covariance Components: A Case Study for GPS Stochastic Model Evaluation[J]. IEEE Transaction on Geosciences and Remote Sensing, 2011, 49(1): 203-210. doi: 10.1109/TGRS.2010.2054100
[75]	Li B, Shen Y, Xu P. Assessment of Stochastic Models for GPS Measurements with Different Types of Receivers[J]. Chinese Science Bulletin, 2008, 53(20): 3219-3225. doi: 10.1007/s11434-008-0293-6
[76]	Li B. Stochastic Modeling of Triple-Frequency BeiDou Signals: Estimation, Assessment and Impact Analysis[J]. Journal of Geodesy, 2016, 90(7): 593-610. doi: 10.1007/s00190-016-0896-7
[77]	Li B, Zhang Z, Shen Y, et al. A Procedure for the Significance Testing of Unmodeled Errors in GNSS Observations[J]. Journal of Geodesy, 2018, 92(10): 1171-1186. doi: 10.1007/s00190-018-1111-9
[78]	Zhang Z, Li B. Unmodeled Error Mitigation for Single-Frequency Multi-GNSS Precise Positioning Based on Multi-epoch Partial Parameterization[J]. Measurement Science and Technology, 2020, 31(2): 025008.
[79]	Zhang Z, Li B, Shen Y. Efficient Approximation to a Fully Populated Variance-Covariance Matrix for RTK Positioning[J]. Journal of Surveying Engineering, 2018, 144(4): 04018005.
[80]	Dach R, Hugentobler U, Fridez P, et al. Bernese GPS Software Version 5.0[R]. Bern: University of Bern, 2007.
[81]	Li B, Liu T, Nie L, et al. Single-Frequency GNSS Cycle Slip Estimation with Positional Polynomial Constraint[J]. Journal of Geodesy, 2019, 93(9): 1781-1803.
[82]	Zhang Z, Li B, Gao Y, et al. Asynchronous and Time-Differenced RTK for Ocean Applications Using the BeiDou Short Message Service[J]. Journal of Geodesy, 2023, 97(1): 7.
[83]	Dixon, K. StarFire: A Global SBAS for Sub-Decimeter Precise Point Positioning[C]//The 19th International Technical Meeting of the Satellite Division of the Institute of Navigation (ION GNSS 2006), Fort Worth, TX, USA, 2006.
[84]	Li B, Zhang Z, Zang N, et al. High-Precision GNSS Ocean Positioning with BeiDou Short-Message Communication[J]. Journal of Geodesy, 2019, 93(2): 125-139.
[85]	Julien O, Alves P, Cannon M E, et al. A Tightly Coupled GPS/Galileo Integration for Improved Ambiguity Resolution[C]//Proceedings of ENC-GNSS 2003, Graz, Austria, 2003.
[86]	Odijk D, Teunissen P, Huisman L. First Results of Mixed GPS+GIOVE Single-Frequency RTK in Australia[J]. Journal of Spatial Science, 2012, 57(1): 3-18.
[87]	Odijk D, Teunissen P. Estimation of Differential Inter-System Biases Between the Overlapping Frequencies of GPS, Galileo, BeiDou and QZSS[C]//The 4th International Colloquium Scientific and Fundamental Aspects of the Galileo Program, Prague, Czech Republic, 2013.
[88]	Chen G, Li B, Zhang Z, et al. Integer Ambiguity Resolution and Precise Positioning for Tight Integration of BDS-3, GPS, Galileo, and QZSS Overlapping Frequencies Signals[J]. GPS Solutions, 2021, 26(1): 26.
[89]	Geng T, Li Z, Xie X, et al. Real-Time Ocean Precise Point Positioning with BDS-3 Service Signal PPP-B2b[J]. Measurement, 2022, 203: 111911.
[90]	Ge H, Li B, Ge M, et al. Improving Low Earth Orbit (LEO) Prediction with Accelerometer Data[J]. Remote Sensing, 2020, 12(10): 1599.
[91]	Ge H, Wu T, Li B. Characteristics Analysis and Prediction of Low Earth Orbit (LEO) Satellite Clock Corrections by Using Least-squares Harmonic Estimation[J]. GPS Solutions, 2022, 27(1): 38.
[92]	Zangenehnejad F, Gao Y. GNSS Smartphones Positioning: Advances, Challenges, Opportunities, and Future Perspectives[J]. Satellite Navigation, 2021, 2(1): 24.
[93]	Humphreys T, Murrian M, Van Diggelen F, et al. On the Feasibility of cm-accurate Positioning via a Smartphone's Antenna and GNSS Chip[C]//2016 IEEE/ION Position, Location and Navigation Symposium (PLANS), Savannah, USA, 2016.
[94]	Zhang X, Tao X, Zhu F, et al. Quality Assessment of GNSS Observations from an Android N Smartphone and Positioning Performance Analysis Using Time-Differenced Filtering Approach[J]. GPS Solutions, 2018, 22(3): 70.
[95]	张小红, 陶贤露, 王颖喆, 等. 城市场景智能手机GNSS/MEMS融合车载高精度定位[J]. 武汉大学学报(信息科学版), 2022, 47(10): 1740-1749. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH202210020.htm Zhang Xiaohong, Tao Xianlu, Wang Yingzhe, et al. MEMS-Enhanced Smartphone GNSS High-Precision Positioning for Vehicular Navigation in Urban Conditions[J]. Geomatics and Information Science of Wuhan University, 2022, 47(10): 1740-1749. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH202210020.htm
[96]	张小红, 张元泰, 朱锋. 城市复杂场景下GNSS定位的因子图优化方法及其抗差性能分析[J]. 武汉大学学报(信息科学版), 2023, 48(7): 1050-1057. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH202307004.htm Zhang Xiaohong, Zhang Yuantai, Zhu Feng. Factor Graph Optimization for Urban Environment GNSS Positioning and Robust Performance Analysis[J]. Geomatics and Information Science of Wuhan University, 2023, 48(7): 1050-1057. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH202307004.htm
[97]	Miao W, Li B, Gao Y. The Superiority of Multi-GNSS L5/E5a/B2a Frequency Signals in Smartphones: Stochastic Modeling, Ambiguity Resolution and RTK Positioning[J]. IEEE Internet of Things Journal, 2023, 10(8): 7315-7326.
[98]	Li B, Miao W, Chen G, et al. Ambiguity Resolution for Smartphone GNSS Precise Positioning: Effect Factors and Performance[J]. Journal of Geodesy, 2022, 96(9): 63.
[99]	Engel J, Koltun V, Cremers D. Direct Sparse Odometry[J]. IEEE Transactions on Pattern Analysis and Machine Intelligence, 2018, 40(3): 611-625.
[100]	Latif Y, Cadena C, Neira J. Robust Loop Closing over Time for Pose Graph Slam [J]. International Journal of Robotics Research, 2013, 32(14): 1611-1626.
[101]	Liu T, Li B, Yang L. Phase Center Offset Calibration and Multipoint Time Latency Determination for UWB Location[J]. IEEE Internet of Things Journal, 2022, 9(18): 17536-17550.

施引文献(4)

期刊类型引用(1)

雷洋，朱武，孙全，杨文敬，张金敏. 顾及方位向偏移的光谱分频法InSAR电离层改正. 测绘科学. 2023(01): 81-90 .

百度学术

其他类型引用(3)

资源附件(0)

图(9)

计量

文章访问数: 853
HTML全文浏览量: 1138
PDF下载量: 357
被引次数: 4

1 本文方法
1.1 利用全极化SAR数据计算FR角
1.2 由法拉第旋转角反演VTEC
1.3 联合IRI电子密度剖线估计三维空间电子密度分布
2 实验结果
2.1 数据及研究区域
2.2 利用SAR数据计算FR角
2.3 计算VTEC
2.4 联合IRI估计三维电子密度分布
3 误差分析
3.1 VTEC结果验证
3.2 三维电子密度结果验证
4 结语

1 本文方法
1.1 利用全极化SAR数据计算FR角
1.2 由法拉第旋转角反演VTEC
1.3 联合IRI电子密度剖线估计三维空间电子密度分布
2 实验结果
2.1 数据及研究区域
2.2 利用SAR数据计算FR角
2.3 计算VTEC
2.4 联合IRI估计三维电子密度分布
3 误差分析
3.1 VTEC结果验证
3.2 三维电子密度结果验证
4 结语

参考文献(101)

施引文献(4)

资源附件(0)

多频多模GNSS高精度定位关键技术与挑战

作者简介: 李博峰，博士，教授，研究方向为测量数据处理理论、GNSS精密定轨定位、多传感器融合综合PNT。bofeng_li@tongji.edu.cn

计量

出版历程