BDS-3 Real-Time Filtered Precise Orbit Determination with Undifferenced Ambiguity Resolution
-
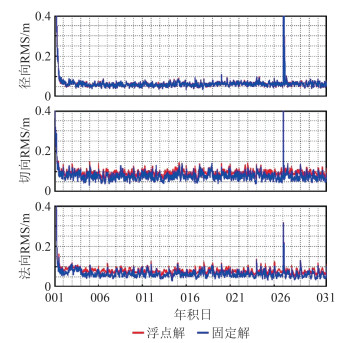
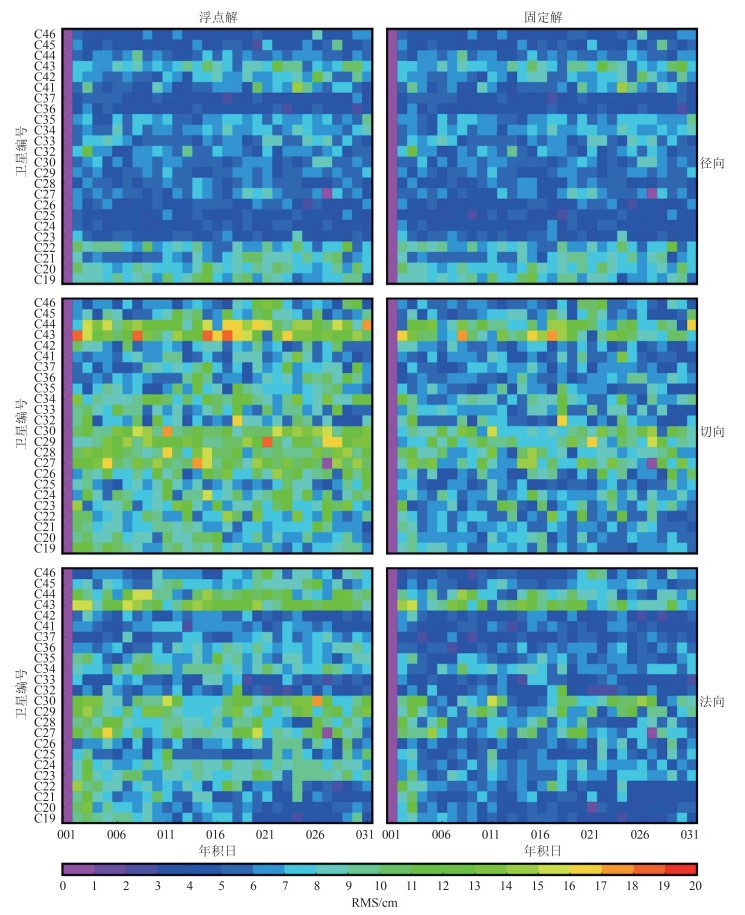
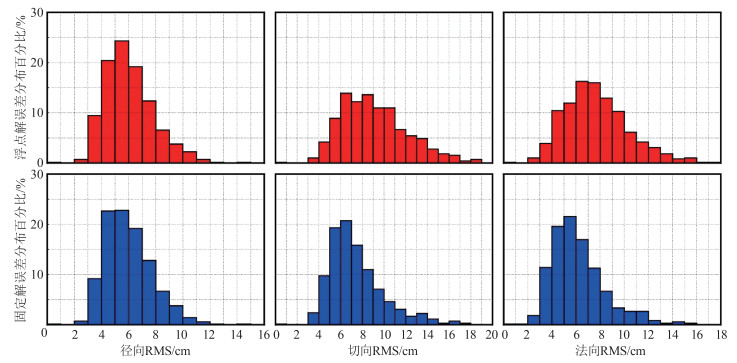
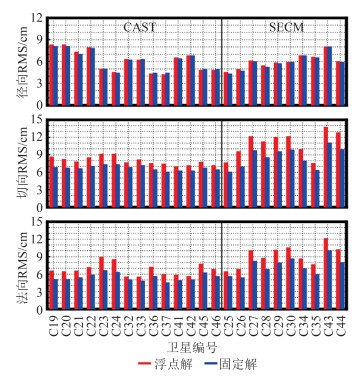
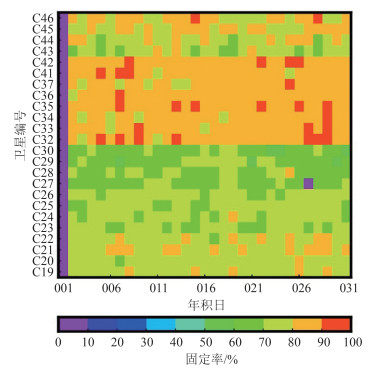
摘要: 模糊度固定是全球导航卫星系统（global navigation satellite system，GNSS）高精度数据处理的关键。不同于传统的双差模糊度固定，非差模糊度固定无需构建双差模糊度，更为简单高效。将非差模糊度固定引入北斗三号全球卫星导航系统(BeiDou-3 global navigation satellite system，BDS-3)中地球轨道卫星实时滤波定轨，分析非差模糊度固定对实时滤波轨道收敛速度及精度的影响。利用国际GNSS服务组织全球测站网观测数据进行实时滤波精密定轨实验，以德国地学研究中心的事后快速轨道为参考评定精度。结果表明：非差模糊度固定对收敛速度影响很小，但可以有效提升轨道切向、法向精度；相比浮点解轨道，固定解轨道径向、切向、法向精度分别提高1.0%、18.5%、19.5%，误差均方根分别达到6.0、7.4、6.2 cm；受切向、法向影响，中国空间技术研究院类型卫星轨道精度优于上海微小卫星工程中心类型卫星轨道；顾及窄巷固定率与轨道精度的相关性，窄巷固定率可以作为实时轨道质量的重要指标之一。实时滤波轨道精度的进一步提升有赖于BDS-3数据处理模型的持续精化。Abstract:Objectives Ambiguity resolution is the key for global navigation satellite system(GNSS) high-accuracy data processing. Different from traditional double-difference ambiguity resolution, the undifferenced ambiguity resolution does not need to construct double-difference ambiguity, which is simpler and more efficient.Methods The undifferenced ambiguity resolution is introduced into the BeiDou-3 satellite navigation system(BDS-3)medium Earth orbit satellite real-time filtered orbit determination, and the influence of undifferenced ambiguity resolution on the convergence speed and accuracy of the real-time filtered orbit is analyzed. With observation data from International GNSS Service global network stations, simulated real-time filtered precise orbit determination experiment is conducted with German Research Centre for Geosciences post-processed precise orbit for accuracy assessment.Results The results indicate that while undifferenced ambiguity resolution has little effect on the convergence, it can effectively improve the orbit tangential/normal accuracy; compared with the ambiguity-float orbit, the accuracy of ambiguity-fixed orbit is improved by 1.0%, 18.5%, 19.5% and the error root mean square reaches 6.0, 7.4, 6.2 cm for the radial, tangential, normal directions respectively. Affected by the tangential, normal direction, the orbit accuracy of China Academy of Space Technology type satellites is better than that of Shanghai Engineering Center for Microsatellites type satellites; considering the correlation between narrow lane ambiguity fixed rate and orbit accuracy, narrow lane fixed rate can be used as one of the important indicators of real-time orbit quality.Conclusions Further improvement of real-time filtered orbit relies on the continuous refinement of BDS-3 data processing models.
-
致谢: 感谢IGS提供观测数据，GFZ提供精密产品。
-
![]()
图 1 非差模糊度固定实时滤波定轨流程图
Figure 1. Flowchart of Real-Time Filtered Orbit Determination with Undifferenced Ambiguity Resolution
![]()
图 2 BDS-3实时滤波轨道误差星座均值RMS
Figure 2. BDS-3 Real-Time Filtered Orbit Error Constellation-Mean RMS
![]()
图 3 BDS-3实时滤波轨道误差单天均值RMS
Figure 3. BDS-3 Real-Time Filtered Orbit Error Daily-Mean RMS
![]()
图 4 BDS-3实时滤波轨道误差单天均值RMS分布
Figure 4. BDS-3 Real-Time Filtered Orbit Error Daily-Mean RMS Distribution
![]()
图 5 BDS-3实时滤波轨道误差径向/切向/法向平均RMS
Figure 5. BDS-3 Real-Time Filtered Orbit Error Radial/Tangential/Normal Average RMS
![]()
图 6 BDS-3实时滤波轨道窄巷单天均值固定率
Figure 6. BDS-3 Real-Time Filtered Orbit Narrow Lane Daily-Mean Fixed Rate
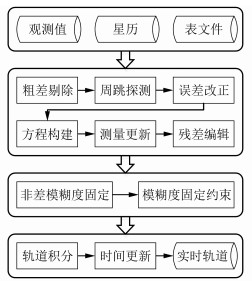
表 1 BDS-3实时滤波定轨处理策略
Table 1 Processing Strategy of BDS-3 Real-Time Filtered Orbit Determination
项目 模型 观测值 非差IF组合伪距、相位，截止高度角10°，采样率30 s 定权策略 原始伪距、相位噪声为0.3 m、0.3 cm，高度角权函数为1/sine 天线相位 igs14.atx[26]，接收机端采用GPS相近频率数值替代 卫星姿态 建模 对流层 干延迟：Saastamoinen公式[27]；气压：GPT2w模型[28]；湿延迟：估计，随机游走模型，Vienna投影函数[29]；水平梯度：不考虑 电离层 一阶项：IF组合消除；高阶项：不考虑 潮汐位移 固体潮、海潮、极潮，IERS Conventions 2010[30]，FES2004海潮模型[31] 相位缠绕 改正[32] 接收机钟差 估计，白噪声模型 卫星钟差 估计，白噪声模型 相位模糊度 估计，弧段常数 地球引力 EGM2008模型[33]，IERS Conventions 2010[30] 三体引力 日月以及其他行星，点质量模型，JPL DE405行星星历 潮汐摄动 固体潮、海潮、极潮，IERS Conventions 2010[30]，FES2004海潮模型[31] 相对论效应 IERS Conventions 2010[30] 太阳辐射压 5参数ECOM模型[34] 地球辐射压 不考虑 天线反推力 根据IGS卫星元数据计算[35] 测站坐标 固定为IGS SINEX解 EOP 固定为IERS Bulletin A解 数值积分器 RKF7(8)，30 s步长 参数估计器 扩展卡尔曼滤波 
下载: 导出CSV
表 2 BDS-3实时滤波定轨参数设置
Table 2 Parameter Setting of BDS-3 Real-Time Filtered Orbit Determination
参数 初始状态 初始方差 过程噪声 卫星位置 GFZ事后解 1×104 m2 0 m2 卫星速度 GFZ事后解 1×10－4 (m/s)2 1×10－16×30 (m/s)2 SRP系数 D0为－100.0 nm/s2，
其余为0.0 nm/s21×104 (nm/s2) 2 1×10－7×30 (nm/s2)2 卫星钟差 GFZ事后解 1×104 m2 接收机钟差 伪距单点定位 1×104 m2 测站ZWD 0.0 m 0.25 m2 3×10－8×30 m2 相位模糊度 对齐伪距 1×106 m2 0 m2 卫星宽巷UPD 0.0周 1×102周2 1×30周2 接收机宽巷UPD 0.0周 1×102周2 1×30周2 卫星窄巷UPD 0.0周 1×102周2 1×30周2 接收机窄巷UPD 0.0周 1×102周2 1×30周2
下载: 导出CSV
-
[1] Zumberge J F, Heflin M B, Jefferson D C, et al. Precise Point Positioning for the Efficient and Robust Analysis of GPS Data from Large Networks[J]. Journal of Geophysical Research: Solid Earth, 1997, 102(B3): 5005-5017. doi: 10.1029/96JB03860
[2] 曹新运, 沈飞, 李建成, 等. BDS-3/GNSS非组合精密单点定位[J]. 武汉大学学报(信息科学版), 2023, 48(1): 92-100. doi: 10.13203/j.whugis20210198 Cao Xinyun, Shen Fei, Li Jiancheng, et al. BDS-3/GNSS Uncombined Precise Point Positioning[J]. Geomatics and Information Science of Wuhan University, 2023, 48(1): 92-100. doi: 10.13203/j.whugis20210198
[3] Johnston G, Riddell A, Hausler G. The International GNSS Service[M]//Teunissen P J G, Montenbruck O. Springer Handbook of Global Navigation Satellite Systems. Cham: Springer International Publishing, 2017.
[4] Lou Y D, Dai X L, Gong X P, et al. A Review of Real-Time Multi-GNSS Precise Orbit Determination Based on the Filter Method[J]. Satellite Navigation, 2022, 3(1): 15. doi: 10.1186/s43020-022-00075-1
[5] Choi K K, Ray J, Griffiths J, et al. Evaluation of GPS Orbit Prediction Strategies for the IGS Ultra-Rapid Products[J]. GPS Solutions, 2013, 17(3): 403-412. doi: 10.1007/s10291-012-0288-2
[6] Ye F, Yuan Y B, Zhang B C. Impact Analysis of Arc Length in Multi-GNSS Ultra-Rapid Orbit Determination Based on the One-Step Method[J]. Measurement Science and Technology, 2020, 31(5): 055012. doi: 10.1088/1361-6501/ab69d4
[7] Li X X, Chen X H, Ge M R, et al. Improving Multi-GNSS Ultra-rapid Orbit Determination for Real-Time Precise Point Positioning[J]. Journal of Geodesy, 2019, 93(1): 45-64. doi: 10.1007/s00190-018-1138-y
[8] Bertiger W, Bar-Sever Y, Dorsey A, et al. GipsyX/RTGX, a New Tool Set for Space Geodetic Operations and Research[J]. Advances in Space Research, 2020, 66(3): 469-489. doi: 10.1016/j.asr.2020.04.015
[9] Kuang K, Li J, Zhang S, et al. Improve Real-Time GNSS Orbit with Epoch-Independent Undifferenced Ambiguity Resolution[J]. Advances in Space Research, 2021, 68(11): 4544-4555. doi: 10.1016/j.asr.2021.08.021
[10] Dai X L, Gong X P, Li C L, et al. Real-Time Precise Orbit and Clock Estimation of Multi-GNSS Satellites with Undifferenced Ambiguity Resolution[J]. Journal of Geodesy, 2022, 96(10): 73. doi: 10.1007/s00190-022-01664-3
[11] Ge M, Gendt G, Dick G, et al. Improving Carrier-Phase Ambiguity Resolution in Global GPS Network Solutions[J]. Journal of Geodesy, 2005, 79(1): 103-110.
[12] Li Z N, Li M, Shi C, et al. Impact of Ambiguity Resolution with Sequential Constraints on Real-Time Precise GPS Satellite Orbit Determination[J]. GPS Solutions, 2019, 23(3): 85. doi: 10.1007/s10291-019-0878-3
[13] Ge M, Gendt G, Rothacher M, et al. Resolution of GPS Carrier-Phase Ambiguities in Precise Point Positioning (PPP) with Daily Observations[J]. Journal of Geodesy, 2008, 82(7): 389-399. doi: 10.1007/s00190-007-0187-4
[14] Laurichesse D, Mercier F, Berthias J P, et al. Integer Ambiguity Resolution on Undifferenced GPS Phase Measurements and Its Application to PPP and Satellite Precise Orbit Determination[J]. Navigation, 2009, 56(2): 135-149. doi: 10.1002/j.2161-4296.2009.tb01750.x
[15] Collins P, Bisnath S, Lahaye F, et al. Undifferenced GPS Ambiguity Resolution Using the Decoupled Clock Model and Ambiguity Datum Fixing[J]. Navigation, 2010, 57(2): 123-135. doi: 10.1002/j.2161-4296.2010.tb01772.x
[16] Chen H, Jiang W P, Ge M R, et al. An Enhanced Strategy for GNSS Data Processing of Massive Networks[J]. Journal of Geodesy, 2014, 88(9): 857-867. doi: 10.1007/s00190-014-0727-7
[17] Ruan R G, Wei Z Q. Between-Satellite Single-Difference Integer Ambiguity Resolution in GPS/GNSS Network Solutions[J]. Journal of Geodesy, 2019, 93(9): 1367-1379. doi: 10.1007/s00190-019-01251-z
[18] Geng J H, Mao S Y. Massive GNSS Network Analysis Without Baselines: Undifferenced Ambiguity Resolution[J]. Journal of Geophysical Research: Solid Earth, 2021, 126(10): e2020JB021558.
[19] 邓志国, 王君刚, 葛茂荣. GBM快速轨道产品及非差模糊度固定对其精度的改进[J]. 测绘学报, 2022, 51(4): 544-555. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB202204007.htm Deng Zhiguo, Wang Jungang, Ge Maorong. The GBM Rapid Product and the Improvement from Undifferenced Ambiguity Resolution[J]. Acta Geodaetica et Cartographica Sinica, 2022, 51(4): 544-555. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB202204007.htm
[20] Geng J H, Meng X L, Dodson A H, et al. Integer Ambiguity Resolution in Precise Point Positioning: Method Comparison[J]. Journal of Geodesy, 2010, 84(9): 569-581. doi: 10.1007/s00190-010-0399-x
[21] Lannes A, Prieur J L. Calibration of the Clock-phase Biases of GNSS Networks: The Closure-Ambiguity Approach[J]. Journal of Geodesy, 2013, 87(8): 709-731. doi: 10.1007/s00190-013-0641-4
[22] Dong D N, Bock Y. Global Positioning System Network Analysis with Phase Ambiguity Resolution Applied to Crustal Deformation Studies in California[J]. Journal of Geophysical Research: Solid Earth, 1989, 94(B4): 3949-3966. doi: 10.1029/JB094iB04p03949
[23] Steigenberger P, Deng Z, Guo J, et al. BeiDou-3 Orbit and Clock Quality of the IGS Multi-GNSS Pilot Project[J]. Advances in Space Research, 2023, 71(1): 355-368. doi: 10.1016/j.asr.2022.08.058
[24] Wang N B, Li Z S, Duan B B, et al. GPS and GLONASS Observable-Specific Code Bias Estimation: Comparison of Solutions from the IGS and MGEX Networks[J]. Journal of Geodesy, 2020, 94(8): 74. doi: 10.1007/s00190-020-01404-5
[25] Deng Z, Nischan T, Bradke M. Multi-GNSS Rapid Orbit-, Clock- & EOP-Product Series[EB/OL]. (2017-01-01)[2023-03-15]. https://doi.org/10.5880/GFZ.1.1.2017.002.
[26] Rebischung P, Schmid R. IGS14/igs14. atx: A New Framework for the IGS Products[C]// AGU Fall Meeting, San Francisco, CA, USA, 2016.
[27] Davis J L, Herring T A, Shapiro I I, et al. Geodesy by Radio Interferometry: Effects of Atmospheric Modeling Errors on Estimates of Baseline Length[J]. Radio Science, 1985, 20(6): 1593-1607. doi: 10.1029/RS020i006p01593
[28] Böhm J, Möller G, Schindelegger M, et al. Development of an Improved Empirical Model for Slant Delays in the Troposphere (GPT2w)[J]. GPS Solutions, 2015, 19(3): 433-441. doi: 10.1007/s10291-
[29] Boehm J, Werl B, Schuh H. Troposphere Mapping Functions for GPS and very Long Baseline Interfero‑ metry from European Centre for Medium-range Weather Forecasts Operational Analysis Data[J]. Journal of Geophysical Research: Solid Earth, 2006, 111(B2): B02406.
[30] Petit G, Luzum B. IERS Conventions(2010)(IERS Technical Note No. 36)[EB/OL]. (2019-04-26)[2023-03-15]. http://iers-conventions.obspm.fr/conventions_versions.php#official_target.
[31] Lyard F, Lefevre F, Letellier T, et al. Modelling the Global Ocean Tides: Modern Insights from FES2004[J]. Ocean Dynamics, 2006, 56(5): 394-415.
[32] Wu J T, Wu S C, Hajj G A, et al. Effects of Antenna Orientation on GPS Carrier Phase[J]. Manuscripta Geodaetica, 1993, 18: 91-98.
[33] Pavlis N K, Holmes S A, Kenyon S C, et al. The Development and Evaluation of the Earth Gravitational Model 2008 (EGM2008)[J]. Journal of Geophysical Research: Solid Earth, 2012, 117(B4): B04406.
[34] Arnold D, Meindl M, Beutler G, et al. CODE's New Solar Radiation Pressure Model for GNSS Orbit Determination[J]. Journal of Geodesy, 2015, 89(8): 775-791.
[35] 阮仁桂, 贾小林, 冯来平, 等. 北斗三号MEO卫星非保守力建模[J]. 测绘学报, 2022, 51(9): 1862-1869. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB202209003.htm Ruan Rengui, Jia Xiaolin, Feng Laiping, et al. Non-conservative Force Modeling of BeiDou-3 MEO Satellite[J]. Acta Geodaetica et Cartographica Sinica, 2022, 51(9): 1862-1869. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB202209003.htm
-
期刊类型引用(3)
1. 周永章，陈川，张旗，王功文，肖凡，沈文杰，卞静，王亚，杨威，焦守涛，刘艳鹏，韩枫. 地质大数据分析的若干工具与应用. 大地构造与成矿学. 2020(02): 173-182 . 
百度学术
2. 王叶晨梓，杜震洪，张丰，刘仁义. 面向分片地图的多分辨率格点数据统一存取方法. 浙江大学学报(理学版). 2017(05): 584-590 . 百度学术
3. 朱建章，石强，陈凤娥，史晓丹，董泽民，秦前清. 遥感大数据研究现状与发展趋势. 中国图象图形学报. 2016(11): 1425-1439 . 百度学术
其他类型引用(6)
计量
- 文章访问数: 367
- HTML全文浏览量: 74
- PDF下载量: 80
- 被引次数: 9
