GNSS Precise Time-Frequency Receiver Clock Steering Model and Parameter Design Method
-
摘要: 实时精密单点定位（real time precise point positioning, RT-PPP）技术可实现亚纳秒级、天稳定度达到1×10－14量级的全球导航卫星系统（global navigation satellite system, GNSS）单向授时。时钟调控直接影响输出时频信号的稳定度性能，但调控参数不合适时将破坏振荡器的短期稳定度。面向振荡器短期稳定度和RT-PPP长期稳定度最优组合，构建了RT-PPP时频接收机的锁相环时钟调控模型，分析了调控系统噪声，提出了一种二阶锁相环调控参数设计方法。利用RT-PPP时频接收机对所提出的调控模型和参数设计方法进行了实测验证，结果表明，利用所设计的时钟调控模型和参数，RT-PPP时频接收机的频率稳定度可达秒稳4.344 4×10－12，天稳1.102 9×10－14，在时间间隔小于300 s时与铷钟自由振荡稳定度基本一致，大于300 s时与RT-PPP稳定度基本一致，能很好地组合振荡器短期稳定度和RT-PPP长期稳定度优势。Abstract:Objectives Real time precise point positioning(RT-PPP) technology can realize one-way timing with sub-nanosecond precision and daily stability of 1×10－14 level. Clock steering affects the stability of the receiver's output time-frequency signal, however, inappropriate clock steering parameters can degrade the short-term stability of the receiver's oscillator.Methods To combine the short-term stability of the oscillator and the long-term stability of the RT-PPP, we propose a phase-locked loop clock steering model for the RT-PPP time-frequency receiver. The noises of the system are analyzed. And the parameter design method of the second-order phase-locked loop is proposed. Experiments were carried out with the clock steering model and designed parameters used in the RT-PPP time-frequency receiver.Results Experimental results show that the frequency stability of the RT-PPP time-frequency receiver is 4.344 4×10－12 at 1 s, 1.102 9×10－14 at 1 d, the frequency stability of the time intervals shorter than 300 s can get close to the free-running Rubidium clock, the frequency stability of the time intervals longer than 300 s can get close to the RT-PPP.Conclusions The proposed clock steering model and parameter design method can well realize the combination of the oscillator's short-term stability and the RT-PPP's long-term stability.
-
Keywords:
- RT-PPP /
- phase-locked loop /
- noise analysis /
- frequency stability /
- parameter design
-
随着实时精密单点定位[1-2]（real time precise point positioning，RT-PPP）技术在时频领域的应用，全球导航卫星系统（global navigation satellite system，GNSS）授时精度得到了显著提升，逐步形成了一种高精度低成本、节点数不受限、实时单向授时技术，其授时精度可达亚纳秒级、天稳定度达到1×10－14量级[3]。RT-PPP维持着长期稳定的时频基准，相比时频接收机的振荡器在长期稳定度上有明显优势。时频接收机中通常是成本低且体积小的温补晶振、恒温晶振和铷钟，这些振荡器生成的时频信号会随着时间发散，表现出较差的长期频率稳定度[4]。GNSS精密时频接收机利用RT-PPP估计出本地振荡器的时间偏差，定期对振荡器进行调控，从而抑制振荡器的发散，可以有效改善接收机输出时频信号的长期稳定度。文献[5]实现了一种RT-PPP时频接收机，时钟调控后恒温晶振的长期稳定度可达天稳定度2×10－14。文献[6]实验证明利用RT-PPP差分驯服恒温晶振后，稳定度性能优于高水平工业铯钟。
在时频接收机中，调控可以显著改善振荡器的长期频率稳定度，但同时可能对振荡器的短期频率稳定度造成破坏。从文献来看，文献[5]的RT-PPP时频接收机调控后输出时频信号的短期频率稳定度约为10 s稳定度9×10－11，相比其中恒温晶振自由振荡的10 s稳定度2×10－12有明显损失，这种振荡器短期稳定度损失通常是由不合理的时钟调控造成的。对于短期稳定度较高的振荡器，在一定时间间隔内，振荡器自由振荡的时频抖动小于RT-PPP观测时频抖动，此时过度调控将导致接收机输出时频的短期频率稳定度性能比振荡器自由振荡差[7-8]。因此，如何设计合理的调控算法，充分结合RT-PPP时频接收机本地振荡器短期稳定度优势和RT-PPP长期稳定度优势，是时频接收机的核心问题和关键技术。
时频接收机中振荡器的时钟调控属于动态系统的反馈控制问题，各种通用的控制器都可以应用于时钟调控。文献[9]首次利用线性二次高斯（linear quadratic Gaussian，LQG）算法将远程氢钟同步到由美国海军天文台产生的协调世界时作为备份，比较了3组LQG调控参数设计的影响，其中一组调控参数被应用于实际远程时钟调控，在实现纳秒级同步精度的同时没有破坏被控氢钟的短期稳定度。2008年，美国国家标准与技术研究院发布了一款基于锁相环（phase locked loop，PLL）调控技术的GPS驯服振荡器，该产品实测的稳定度指标与铯钟相当，被推荐作为标定和计量实验室的主要频率标准[10]。美国国家标准与技术研究院于2010年发布了基于比例、积分和微分（proportional integral derivative，PID）3项控制器的GPS共视驯服器，PID调控参数可以由使用者配置，该产品除了可以为标定和计量实验室提供频率，还可以提供美国国家标准与技术研究院产生的协调世界时，24 h间隔平均下的频率不确定度约为1×10－14，时间不确定度约为10 ns[11]。按照反馈控制理论[12]，上述控制器中LQG调控属于基于状态空间设计的现代控制方法，PLL和PID属于通过频域变换分析设计的经典控制方法，都属于经典的通用控制器。这些文献对实现系统的性能进行了实际测试，但对调控算法的参数设计方法没有具体的理论分析，公布的调控参数设计不能直接应用于其他时频系统和实际场景。
在时钟调控参数设计理论方面，文献[13]认为控制理论在时钟调控中的潜力还有待挖掘，其仿真实验结果中同一控制器在不同调控参数下时频信号的稳定度性能相差较大，不同控制器在选择合适的调控参数后，时频信号的稳定度性能相近。在时频应用中，实现稳定度最优组合的重要理论是系统噪声分析。文献[14]分析了锁相环中的加性噪声和振荡器相位噪声，指出锁相环带宽应合理权衡以实现最小相位抖动。为利用铯钟和氢钟实现最优稳定度组合的时间基准，文献[8]分析利用二阶数字锁相环和二阶卡尔曼滤波的等效关系，将二阶数字锁相环的系统闭环传递函数和误差传递函数交点频率设计为氢钟和铯钟相位噪声交点频率。文献[15]面向原子钟内基准和振荡器稳定度最优组合的问题，从基准和振荡器频率稳定度交点时间间隔出发，提出了LQG调控最优控制的调控参数设计方法。这些文献主要针对时频实验室原子钟频率合成，以实现频率稳定度最优组合为目标，对调控系统噪声进行了理论分析和参数设计，但均是利用仿真实验进行验证，缺乏实测验证。同时，时频实验室原子钟频率合成的信道较为理想，而RT-PPP授时接收机时钟调控场景面临的系统噪声有所不同，RT-PPP参考时频信号经过了卫星无线信号传播，传播噪声不可忽略。文献[16]采用乒乓算法将芯片级原子钟与GNSS系统时间同步误差控制在±7.5 ns，驾驭后芯片级原子钟1 h频率准确度为8.5×10－13，平均时间为10 000 s时的频率稳定度为3×10－13，该驾驭算法直接利用芯片级原子钟和GNSS时间的频率稳定度交点时间间隔作为乒乓算法驾驭时间，在短期频率稳定度损失上，相比芯片级原子钟演示软件的驾驭损失更小，但相比芯片级原子钟自由振荡仍有明显的损失。
本文针对RT-PPP时频接收机，建立了一种锁相环时钟调控模型，以最优稳定度输出为目标，进行了系统噪声分析，推导了二阶锁相环的参数设计公式，通过实测实验对调控性能进行了分析验证。
1 锁相环时频接收机时钟调控模型
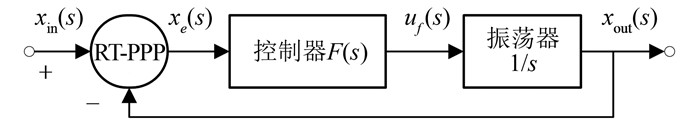
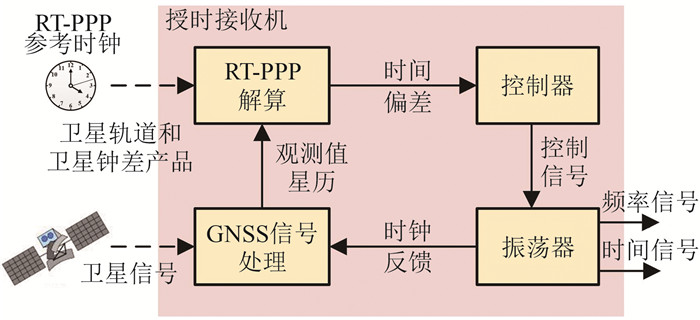
如图 1所示，RT-PPP时频接收机系统主要包括4个部分：振荡器、GNSS信号处理、RT-PPP解算和控制器。振荡器是系统时钟调控的核心部分，生成的时频信号输出供给用户并同时反馈到GNSS信号处理部分；GNSS信号处理部分基于振荡器产生的时频信号处理接收的GNSS卫星信号，输出广播星历、伪距和载波观测值；RT-PPP解算部分利用网络接收的RT-PPP精密卫星轨道和卫星钟差产品[17]，对广播星历、伪距和载波观测值进行解算处理，估计出接收机时间偏差。控制器利用接收机时间偏差，根据时钟调控算法生成对振荡器频率的控制信号，使得本地时频对齐RT-PPP参考时频。
上述系统处理流程中，RT-PPP时频接收机对振荡器的控制过程可以视作锁相环[18]，GNSS信号处理和RT-PPP解算部分整体等效为锁相环的相位鉴别器，控制器等效为环路滤波器，等效后系统的锁相环结构如图 2所示。
![]()
图 2 RT-PPP时频接收机锁相环结构框图Figure 2. Phase-Locked Loop Structure of RT-PPP Time-Frequency Receiver图 2中信号和模块在拉普拉斯变换域表示，$ s $是拉普拉斯变换复频率，$ {x}_{\mathrm{i}\mathrm{n}}\left(s\right) $是输入RT-PPP参考时间，$ {x}_{\mathrm{o}\mathrm{u}\mathrm{t}}\left(s\right) $是输出振荡器时间，$ {x}_{e}\left(s\right)={x}_{\mathrm{i}\mathrm{n}}\left(s\right)-{x}_{\mathrm{o}\mathrm{u}\mathrm{t}}\left(s\right) $是RT-PPP模块输出的参考时间和振荡器时间之差，控制器$ F\left(s\right) $是锁相环环路滤波器的传递函数，环路滤波器输入鉴相结果、输出对振荡器的频率控制量$ {u}_{f}\left(s\right) $。
RT-PPP参考时钟特性由发布该产品的分析中心的基准时钟约束策略决定，一种常见的RT-PPP参考时钟满足初始约束[19]：
$$ {\mathit{\boldsymbol{u}}}^{\mathrm{T}}\bullet \mathit{\boldsymbol{t}}=0 $$ (1) 式中，$ \mathit{\boldsymbol{u}}={\left[\begin{array}{cc}\begin{array}{cc}1& 1\end{array}& \begin{array}{cc}\cdots & 1\end{array}\end{array}\right]}^{\mathrm{T}} $是全1向量，长度为所采用导航系统的卫星数；$ \mathit{\boldsymbol{t}}={\left[\begin{array}{cc}\begin{array}{cc}{t}^{{s}_{1}}& {t}^{{s}_{2}}\end{array}& \begin{array}{cc}\cdots & {t}^{{s}_{n}}\end{array}\end{array}\right]}^{\mathrm{T}} $是该导航系统卫星钟差向量，$ {t}^{{s}_{1}} $表示卫星$ {s}_{1} $的卫星钟差，不同的卫星系统或精密产品引入不同的参考时钟。RT-PPP解算给出参考时间和振荡器时间之差，在RT-PPP解算的时域观测方程中，对应RT-PPP解算得到的接收机钟差估计结果$ {\widehat{t}}_{r} $[20]可表示为：
$$ {\widehat{t}}_{r}={t}_{r}-{t}^{0}+{\varepsilon }_{r} $$ (2) 式中，$ {t}_{r} $是本地振荡器时钟；$ {t}^{0} $是RT-PPP参考时钟；$ {\varepsilon }_{r} $表示估计噪声。
控制器$ F\left(s\right) $决定锁相环跟踪滤波性能。本地振荡器和参考之间通常存在初始相位偏差和频率偏差，对于铷钟等较好的振荡器，在调控时间间隔较小时，频率漂移可以忽略，二阶锁相环可以满足跟踪对齐需求。二阶锁相环环路滤波器的传递函数为$ F\left(s\right)=2\xi {\omega }_{n}+{\omega }_{n}^{2}/s $，其中$ {\omega }_{n} $为系统的特征频率，$ \xi $为阻尼系数，$ {\omega }_{n} $和$ \xi $取值决定二阶锁相环的滤波性质，需要根据实际系统需求设计。RT-PPP时频接收机等效为锁相环后，时频接收机时钟调控参数设计可以利用锁相环的分析理论[14]。
2 时钟调控噪声分析与参数设计
本文参数设计目标是频率稳定度[21]指标，频率稳定度在时域可以由阿伦（Allan）方差$ {\sigma }_{y}^{2}\left(\tau \right) $描述，在频域可以由频率抖动功率谱密度$ {S}_{y}\left(f\right) $描述，两者满足[22]：
$$ {\sigma }_{y}^{2}\left(\tau \right)=2{\int }_{0}^{{f}_{h}}{S}_{y}\left(f\right)\frac{\mathrm{s}\mathrm{i}{\mathrm{n}}^{4}\left(\mathrm{\pi }\tau f\right)}{{\left(\mathrm{\pi }\tau f\right)}^{2}}\mathrm{d}f $$ (3) 式中，$ \tau $是Allan方差平均时间；$ f $是以Hz为单位的单边带频率；$ {f}_{h} $是测量的上截止频率。Allan方差长期稳定度对应功率谱密度低频，Allan方差短期稳定度对应功率谱密度高频，利用Allan方差实现RT-PPP长期稳定度和振荡器短期稳定度最优组合时，对应功率谱密度在低频表现为RT-PPP噪声功率谱密度，在高频表现为振荡器噪声功率谱密度。本文频率稳定度目标下锁相环参数设计适合频域分析，将利用功率谱密度分析噪声并设计参数。
RT-PPP参考时间是远程、非物理存在的纸面时钟，时频接收机的时钟调控可以看作远程锁相，不同的接收机锁定到相同参考时间，实现广域时间同步，但GNSS信号在无线传播过程中，引入了传播噪声和电离层、对流层等大气误差的影响，使得在考虑参考和本地振荡器噪声来源以外，还应该考虑参考时频传递过程引入的观测噪声。将系统中的噪声源划分为3类：RT-PPP参考时钟噪声、RT-PPP观测噪声和振荡器自由振荡噪声。由锁相环噪声传播[23-24]，可以推导输出时间抖动功率谱密度为：
$$ {S}_{x}^{\mathrm{o}\mathrm{u}\mathrm{t}}\left(f\right)=\left({S}_{x}^{\mathrm{r}\mathrm{e}\mathrm{f}}\left(f\right)+{S}_{x}^{\mathrm{o}\mathrm{b}\mathrm{s}}\left(f\right)\right){\left|H\left(f\right)\right|}^{2}+\\\ \ \ \ \ \ \ \ \ \ {S}_{x}^{\mathrm{o}\mathrm{s}\mathrm{c}}\left(f\right){\left|{H}_{e}\left(f\right)\right|}^{2} $$ (4) 式中，$ {S}_{x}^{\mathrm{r}\mathrm{e}\mathrm{f}}\left(f\right) $、$ {S}_{x}^{\mathrm{o}\mathrm{b}\mathrm{s}}\left(f\right) $、$ {S}_{x}^{\mathrm{o}\mathrm{s}\mathrm{c}}\left(f\right) $分别是RT-PPP参考时钟、RT-PPP观测和振荡器自由振荡的时间抖动功率谱密度；$ H\left(f\right) $是系统闭环传递函数；$ {H}_{e}\left(f\right)=1-H\left(f\right) $是系统误差传递函数。利用时间抖动和频率抖动功率谱密度的关系[22]：$ {S}_{x}\left(f\right)={S}_{y}\left(f\right)/{\left(2\mathrm{\pi }f\right)}^{2} $，可以将式（4）中的时间抖动功率谱密度$ {S}_{x}\left(f\right) $等效替换为频率抖动功率谱密度$ {S}_{y}\left(f\right) $。
对于本文考虑的3种噪声，由于RT-PPP参考时钟和RT-PPP观测噪声在式（4）描述的稳定度问题中是叠加的，可以将两种噪声合并，统称为RT-PPP噪声。RT-PPP噪声长期稳定度比振荡器高，对应其低频抖动更小，所以低频时有$ {S}_{y}^{\mathrm{r}\mathrm{e}\mathrm{f}}\left(f\right)+{S}_{y}^{\mathrm{o}\mathrm{b}\mathrm{s}}\left(f\right) < {S}_{y}^{\mathrm{o}\mathrm{s}\mathrm{c}}\left(f\right) $；RT-PPP噪声的短期稳定度较铷钟等振荡器低，对应高频抖动较大，所以高频时有$ {S}_{y}^{\mathrm{r}\mathrm{e}\mathrm{f}}\left(f\right)+{S}_{y}^{\mathrm{o}\mathrm{b}\mathrm{s}}\left(f\right) > {S}_{y}^{\mathrm{o}\mathrm{s}\mathrm{c}}\left(f\right) $；则$ {S}_{y}^{\mathrm{r}\mathrm{e}\mathrm{f}}\left(f\right)+{S}_{y}^{\mathrm{o}\mathrm{b}\mathrm{s}}\left(f\right) $和$ {S}_{y}^{\mathrm{o}\mathrm{s}\mathrm{c}}\left(f\right) $有交点频率$ {f}_{c} $满足：
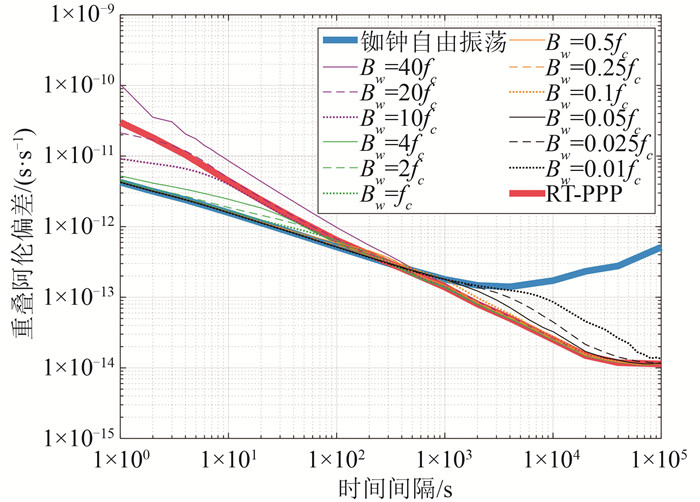
$$ {S}_{y}^{\mathrm{r}\mathrm{e}\mathrm{f}}\left({f}_{c}\right)+{S}_{y}^{\mathrm{o}\mathrm{b}\mathrm{s}}\left({f}_{c}\right)={S}_{y}^{\mathrm{o}\mathrm{s}\mathrm{c}}\left({f}_{c}\right) $$ (5) 简化考虑系统闭环传递函数$ H\left(f\right) $为理想低通滤波，锁相环带宽用$ {B}_{w} $表示，对$ f < {B}_{w} $，有$ \left|H\left(f\right)\right|=1 $且$ \left|{H}_{e}\left(f\right)\right|=0 $；对$ f > {B}_{w} $，有$ \left|H\left(f\right)\right|=0 $且$ \left|{H}_{e}\left(f\right)\right|=1 $。为使式（4）系统输出功率谱密度在低频表现为RT-PPP噪声功率谱密度，在高频表现为振荡器噪声功率谱密度，应将带宽$ {B}_{w} $取为交点频率$ {f}_{c} $，此时系统输出$ {S}_{y}^{\mathrm{o}\mathrm{u}\mathrm{t}}\left(f\right) $在$ f < {B}_{w} $时取到较小的$ {S}_{y}^{\mathrm{r}\mathrm{e}\mathrm{f}}\left(f\right)+{S}_{y}^{\mathrm{o}\mathrm{b}\mathrm{s}}\left(f\right) $，在$ f > {B}_{w} $时取到较小的$ {S}_{y}^{\mathrm{o}\mathrm{s}\mathrm{c}}\left(f\right) $，实现RT-PPP长期稳定度和振荡器短期稳定度组合；当带宽$ {B}_{w} $取值大于交点频率$ {f}_{c} $时，更多的高频RT-PPP噪声传递到输出，造成系统输出短期稳定度变差；当带宽$ {B}_{w} $取值小于交点频率$ {f}_{c} $时，更多的低频振荡器噪声传递到输出，造成系统输出长期稳定度变差。RT-PPP长期稳定度和振荡器短期稳定度优势组合的关键是将带宽参数$ {B}_{w} $设计在交点频率$ {f}_{c} $。
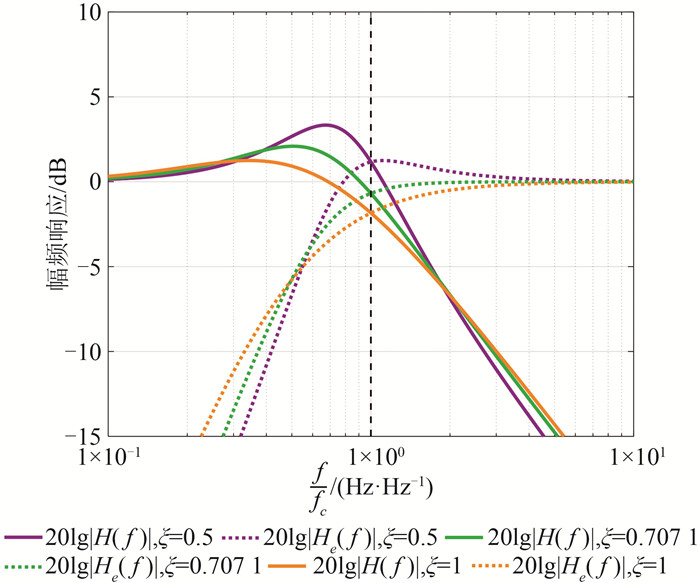
实际二阶锁相环系统传递函数不是理想低通滤波，存在不同的带宽定义，考虑式（4）中系统传递函数和误差传递函数对稳定度指标的影响，本文将带宽$ {B}_{w} $定义为系统传递函数和系统误差传递函数幅频响应的交点[8]，依据二阶锁相环系统传递函数[18]，可以推导得到用$ {\omega }_{n} $和$ \xi $描述的带宽公式为：
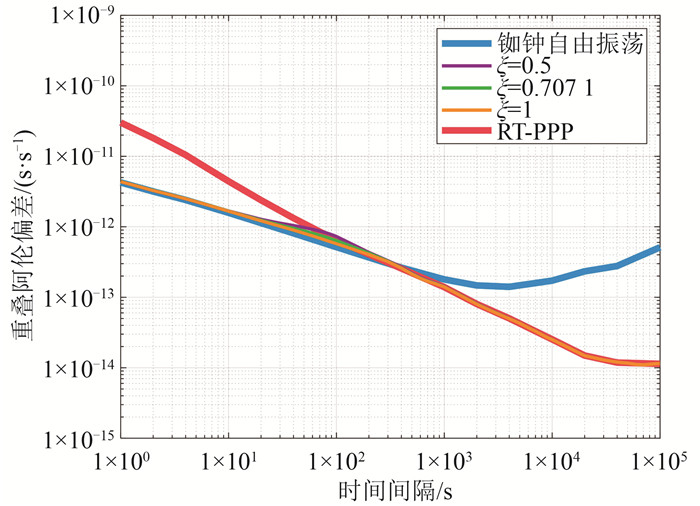
$$ {B}_{w}=\frac{{\omega }_{n}}{2\mathrm{\pi }\sqrt[]{\sqrt[]{1+4{\xi }^{4}}-2{\xi }^{2}}} $$ (6) 令$ {B}_{w}={f}_{c} $可以约束锁相环$ {\omega }_{n} $和$ \xi $参数关系，但不能唯一确定两个参数。频率稳定度指标优化是一个噪声滤波问题，带宽设计占主要影响，但阻尼系数会通过幅频响应曲线形状影响滤波。相同带宽$ {B}_{w} $，不同阻尼系数下，系统传递函数和误差传递函数幅频响应如图 3所示。在交点频率$ {f}_{c} $附近，阻尼系数较大时，系统传递函数和系统误差传递函数的幅频响应都较小，使式（4）输出信号抖动的功率谱密度减小，具有更好的频率稳定度，但较大的阻尼系数会使系统在阶跃响应过程中反应迟缓，需要权衡两个指标。
![]()
图 3 不同阻尼系数系统传递函数和误差传递函数幅频响应Figure 3. Amplitude Response of System Transfer Function and System Error Transfer Function with Various Damping Ratios由于阻尼系数对于滤波效果的影响不占主导，而对于阶跃响应相关的指标影响较大，本文更加推荐取阻尼系数为经验常用[18]的$ \sqrt[]{2}/2 $。阻尼系数$ \xi =\sqrt[]{2}/2 $时，根据$ {B}_{w}={f}_{c} $计算的系统特征频率$ {\omega }_{n}\approx 4.043\mathrm{ }8{f}_{c} $。
3 实验与结果分析
为了验证所提出的时钟调控模型和参数设计性能，本文利用铷钟（FE-5650A）作为本地振荡器的RT-PPP时频接收机进行实验。实验平台如图 4所示，RT-PPP时频接收机连接楼顶GNSS天线和以太网，RT-PPP解算采用了武汉大学实时精密星历和GPS L1/L2无电离层组合观测值；氢钟（CH1-75A）为相位噪声探测仪3120A提供测量参考频率，其频率稳定度为秒稳定度2×10－13和天稳定度7×10－16；电脑用于配合相位噪声探测仪测量时频信号。
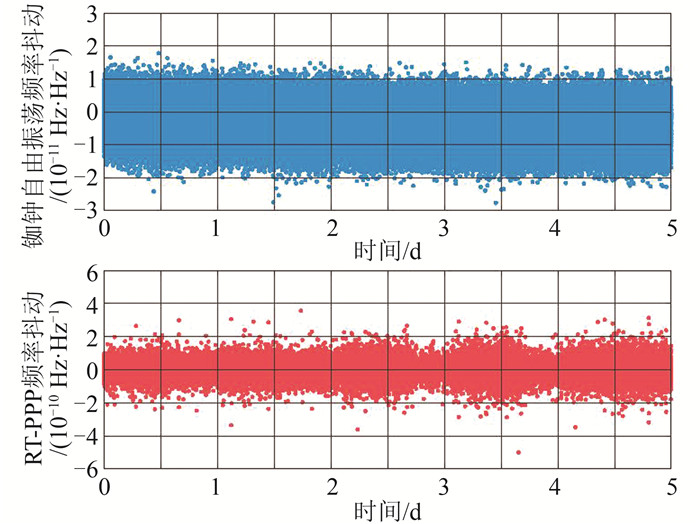
首先，3120A以氢钟为参考采集铷钟自由振荡的时频抖动序列，作为接收机本地振荡器的噪声序列；其次，将时频接收机外接氢钟采集RT-PPP解算的时频抖动序列作为RT-PPP噪声序列。采集的铷钟自由振荡和RT-PPP的频率抖动序列如图 5所示，两个序列频率计算的平均时间为1 s，可以看出，铷钟自由振荡频率抖动序列的主要范围是±2×10－11，RT-PPP频率抖动序列的主要范围是±2×10－10，在1 s的平均时间尺度下，铷钟频率的抖动小于RT-PPP；铷钟自由振荡频率抖动序列的中心频率随时间缓慢漂移，而RT-PPP的中心频率几乎保持不变，可以预计在长时间尺度的平滑平均下，铷钟频率的抖动将大于RT-PPP。
![]()
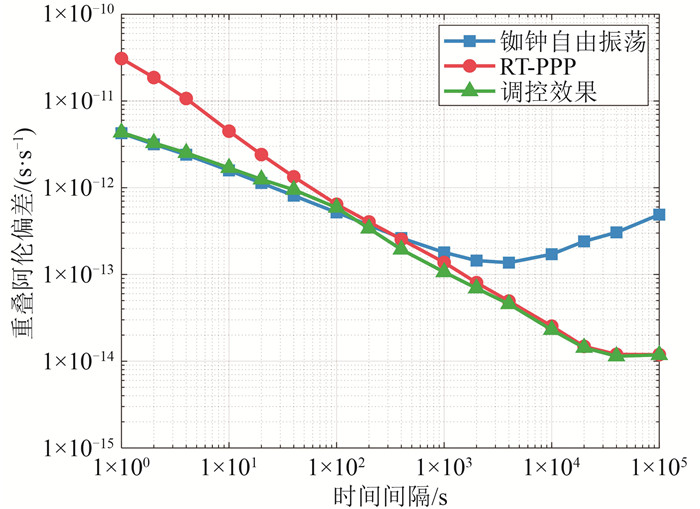
图 5 实测铷钟自由振荡和RT-PPP频率抖动Figure 5. Measured Frequency Fluctuations of Free-Running Rubidium Clock and RT-PPP对铷钟自由振荡和RT-PPP的频率抖动序列统计重叠Allan偏差，频率稳定度情况如图 6所示，在时间间隔小于300 s的短期稳定度上，自由振荡的铷钟具有更优良的频率稳定度，而在时间间隔大于300 s的长期稳定度上，RT-PPP具有更优良的频率稳定度。接收机时钟调控的目的正是通过算法和参数设计实现两者的组合。
![]()
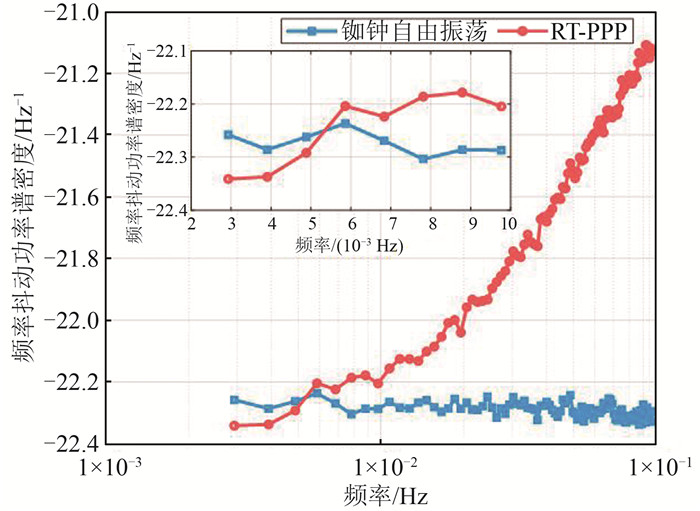
图 6 实测铷钟自由振荡和RT-PPP频率稳定度Figure 6. Measured Frequency Stability of Free-Running Rubidium Clock and RT-PPP铷钟自由振荡和RT-PPP频率抖动序列计算的功率谱密度$ \mathrm{l}\mathrm{g}\left({S}_{y}\left(f\right)\right) $结果如图 7所示。本文频率抖动序列是相对标称频率的相对频率偏差，功率谱密度单位为1/Hz[22]。铷钟自由振荡功率谱密度曲线的斜率近似为0，表示铷钟自由振荡在该频段内调频白噪声占主导；RT-PPP功率谱密度曲线的斜率为正，表示RT-PPP在该频段内调相噪声占主导；在低频段RT-PPP的功率谱密度更小，而高频段铷钟的功率谱密度更小，两条曲线的交点频率为$ {f}_{c}\approx 0.005\mathrm{ }5\mathrm{ }\mathrm{H}\mathrm{z} $。
![]()
图 7 实测铷钟自由振荡和RT-PPP频率抖动功率谱密度Figure 7. Measured Power Spectral Density of Frequency Fluctuations of Free-Running Rubidium Clock and RT-PPP为了快速验证调控参数设计的影响，本文首先用采集的铷钟自由振荡和RT-PPP时频抖动序列设计了仿真实验，铷钟自由振荡频率抖动序列控制系统频率和时间发散，RT-PPP时间抖动序列作为参考时钟和观测噪声叠加结果，统计不同调控参数下系统输出频率序列的重叠Allan偏差。首先固定系统阻尼系数$ \xi =0.707\mathrm{ }1 $，带宽围绕理论值$ {B}_{w}={f}_{c} $多组取值进行对比，结果如图 8所示。当带宽$ {B}_{w} $较大时，100 s以内的重叠Allan偏差较大，偏离铷钟自由振荡，短期稳定度较差，而大于1 000 s的重叠Allan偏差较小，与RT-PPP基本保持一致，长期稳定度较好；相反，当带宽$ {B}_{w} $较小时，100 s以内的重叠Allan偏差与铷钟自由振荡基本保持一致，短期稳定度较好，而大于1 000 s的重叠Allan偏差偏离RT-PPP，长期稳定度较差；当带宽取值接近理论值$ {B}_{w}={f}_{c} $时，调控后输出的频率稳定度对比铷钟自由振荡短期稳定度和RT-PPP长期稳定度都没有明显的损失。
![]()
图 8 仿真不同带宽下RT-PPP时频接收机频率稳定度结果Figure 8. Simulated Frequency Stability of RT-PPP Time-Frequency Receiver with Various Bandwidths固定带宽$ {B}_{w}={f}_{c} $，阻尼系数多组取值对比结果如图 9所示。不同阻尼系数对于频率稳定度指标影响在时间间隔100 s附近，较大的阻尼系数表现出更好的频率稳定度，但总体而言，不同阻尼系数对于频率稳定度的影响较小。
![]()
图 9 仿真不同阻尼系数下RT-PPP时频接收机频率稳定度结果Figure 9. Simulated Frequency Stability of RT-PPP Time-Frequency Receiver with Various Damping Ratios本文还利用RT-PPP时频接收机进行了实测验证，二阶锁相环参数取$ {B}_{w}={f}_{c} $、$ \xi =\sqrt[]{2}/2 $，实测RT-PPP时频接收机调控效果如图 10所示。图 10中内符合相位抖动序列是调控过程中RT-PPP解算的接收机钟差序列，序列在0附近波动；外符合相位抖动序列是3120A测量时频接收机相对氢钟的相位偏差，5 d相位波动范围不超过4 ns；外符合频率抖动序列是3120A测量时频接收机相对氢钟的频率偏差，相比图 5铷钟自由振荡中心频率随时间缓慢漂移，调控后中心频率漂移不明显，可以预计调控后长期频率稳定度的改善。
![]()
图 10 实测RT-PPP时频接收机时间频率抖动Figure 10. Measured Time Fluctuations and Frequency Fluctuations of RT-PPP Time-Frequency Receiver调控前后RT-PPP时频接收机频率稳定度对比如图 11所示，调控后的重叠Allan偏差在小于300 s时贴近铷钟自由振荡，在大于300 s时贴近RT-PPP，与仿真结果基本一致。由此可以认为RT-PPP时频接收机有效结合了铷钟的短期稳定度和RT-PPP的长期稳定度。根据本文实测的RT-PPP时频接收机调控后的频率抖动序列，统计得到的频率稳定度达到秒稳定度4.344 4×10－12，天稳定度1.102 9×10－14。
![]()
图 11 实测RT-PPP时频接收机频率稳定度Figure 11. Measured Frequency Stability of RT-PPP Time-Frequency Receiver4 结语
为提高GNSS高精度时频接收机的稳定度指标，本文建立了RT-PPP时频接收机的时钟调控模型，分析了时钟调控系统中的噪声来源及其传播特性，提出了基于噪声分析的二阶锁相环调控参数设计方法，利用采集的噪声数据仿真比较了参数设计的影响，并基于铷钟RT-PPP时频接收机对本文方法设计的调控模型和参数进行实测验证。结果表明，利用本文提出的调控模型和设计的参数，铷钟RT-PPP时频接收机稳定度达到秒稳定度4.344 4×10－12，天稳定度1.102 9×10－14，在小于300 s的短期稳定度上表现为铷钟自由振荡的稳定度，大于300 s的长期稳定度上表现为RT-PPP的稳定度，能够很好地结合本地振荡器短期稳定度和RT-PPP长期稳定度优势。
-
![]()
图 2 RT-PPP时频接收机锁相环结构框图
Figure 2. Phase-Locked Loop Structure of RT-PPP Time-Frequency Receiver
![]()
图 3 不同阻尼系数系统传递函数和误差传递函数幅频响应
Figure 3. Amplitude Response of System Transfer Function and System Error Transfer Function with Various Damping Ratios
![]()
图 5 实测铷钟自由振荡和RT-PPP频率抖动
Figure 5. Measured Frequency Fluctuations of Free-Running Rubidium Clock and RT-PPP
![]()
图 6 实测铷钟自由振荡和RT-PPP频率稳定度
Figure 6. Measured Frequency Stability of Free-Running Rubidium Clock and RT-PPP
![]()
图 7 实测铷钟自由振荡和RT-PPP频率抖动功率谱密度
Figure 7. Measured Power Spectral Density of Frequency Fluctuations of Free-Running Rubidium Clock and RT-PPP
![]()
图 8 仿真不同带宽下RT-PPP时频接收机频率稳定度结果
Figure 8. Simulated Frequency Stability of RT-PPP Time-Frequency Receiver with Various Bandwidths
![]()
图 9 仿真不同阻尼系数下RT-PPP时频接收机频率稳定度结果
Figure 9. Simulated Frequency Stability of RT-PPP Time-Frequency Receiver with Various Damping Ratios
![]()
图 10 实测RT-PPP时频接收机时间频率抖动
Figure 10. Measured Time Fluctuations and Frequency Fluctuations of RT-PPP Time-Frequency Receiver
-
[1] 宋伟伟, 赵新科, 楼益栋, 等. 北斗三号PPP-B2b服务性能评估[J]. 武汉大学学报(信息科学版), 2023, 48(3): 408-415. doi: 10.13203/j.whugis20200686 Song Weiwei, Zhao Xinke, Lou Yidong, et al. Performance Evaluation of BDS-3 PPP-B2b Service[J]. Geomatics and Information Science of Wuhan University, 2023, 48(3): 408-415 doi: 10.13203/j.whugis20200686
[2] 周要宗, 楼益栋, 张卫星, 等. 新一代实时对流层映射函数精度及PPP性能评估[J]. 武汉大学学报(信息科学版), 2021, 46(12): 1881-1888. doi: 10.13203/j.whugis20210238 Zhou Yaozong, Lou Yidong, Zhang Weixing, et al. On the Accuracy and PPP Performance Evaluation of the Latest Generation of Real Time Tropospheric Mapping Function[J]. Geomatics and Information Science of Wuhan University, 2021, 46(12): 1881-1888 doi: 10.13203/j.whugis20210238
[3] 谭俊雄. 基于PPP的高精度GNSS授时接收机技术[D]. 武汉: 武汉大学, 2019. Tan Junxiong. Research on GNSS High Precision Timing Receiver Based on PPP Technology[D]. Wuhan: Wuhan University, 2019
[4] Lei Y, Tan J X, Guo W F, et al. Time-Domain Evaluation Method for Clock Frequency Stability Based on Precise Point Positioning[J]. IEEE Access, 2019, 7: 132413-132422. doi: 10.1109/ACCESS.2019.2940515
[5] Guo W F, Song W W, Niu X J, et al. Foundation and Performance Evaluation of Real-Time GNSS High-Precision One-Way Timing System[J]. GPS Solutions, 2019, 23(1): 23. doi: 10.1007/s10291-018-0811-1
[6] Rønningen O P, Danielson M. A Novel PPP Disciplined Oscillator[C]//Joint Conference of the IEEE International Frequency Control Symposium and European Frequency and Time Forum, Orlando, USA, 2019.
[7] Matsakis D. The Effects of Proportional Steering Strategies on the Behavior of Controlled Clocks[J]. Metrologia, 2019, 56(2): 025007. doi: 10.1088/1681-7575/ab0614
[8] Wu Y W, Gong H, Zhu X W, et al. A DPLL Method Applied to Clock Steering[J]. IEEE Transactions on Instrumentation and Measurement, 2016, 65(6): 1331-1342. doi: 10.1109/TIM.2016.2526699
[9] Koppang P, Leland R. Linear Quadratic Stochastic Control of Atomic Hydrogen Masers[J]. IEEE Transactions on Ultrasonics, Ferroelectrics, and Frequency Control, 1999, 46(3): 517-522. doi: 10.1109/58.764838
[10] Lombardi M A. The Use of GPS Disciplined Oscillators as Primary Frequency Standards for Calibration and Metrology Laboratories[J]. NCSLI Measure, 2008, 3(3): 56-65. doi: 10.1080/19315775.2008.11721437
[11] Lombardi M A. A NIST Disciplined Oscillator: Delivering UTC(NIST) to the Calibration Laboratory[J]. NCSLI Measure, 2010, 5(4): 46-54. doi: 10.1080/19315775.2010.11721537
[12] Franklin G F, David Powell J, Emami-Naeini A. Feedback Control of Dynamic Systems[M]. NJ: Prentice Hall, 2002.
[13] Farina M, Galleani L, Tavella P, et al. A Control Theory Approach to Clock Steering Techniques[J]. IEEE Transactions on Ultrasonics, Ferroelectrics, and Frequency Control, 2010, 57(10): 2257-2270. doi: 10.1109/TUFFC.2010.1687
[14] Gardner F M. Phaselock Techniques[M]. NJ: Wiley-Interscience, 2005.
[15] Mishagin K G, Lysenko V A, Medvedev S Y. A Practical Approach to Optimal Control Problem for Atomic Clocks[J]. IEEE Transactions on Ultrasonics, Ferroelectrics, and Frequency Control, 2020, 67(5): 1080-1087. doi: 10.1109/TUFFC.2019.2957650
[16] 乔广欣, 张慧君, 李博, 等. GNSS驯服芯片级原子钟方法研究[J]. 导航定位与授时, 2022, 9(2): 153-159. https://www.cnki.com.cn/Article/CJFDTOTAL-DWSS202202019.htm Qiao Guangxin, Zhang Huijun, Li Bo, et al. Research on the Method of GNSS-Disciplined Chip-Scale Atomic Clock[J]. Navigation Positioning and Timing, 2022, 9(2): 153-159 https://www.cnki.com.cn/Article/CJFDTOTAL-DWSS202202019.htm
[17] 赵齐乐, 戴志强, 王广兴, 等. 利用非差观测量估计北斗卫星实时精密钟差[J]. 武汉大学学报(信息科学版), 2016, 41(5): 686-691. doi: 10.13203/j.whugis20150314 Zhao Qile, Dai Zhiqiang, Wang Guangxing, et al. Real-Time Precise BDS Clock Estimation with the Undifferenced Observation[J]. Geomatics and Information Science of Wuhan University, 2016, 41(5): 686-691 doi: 10.13203/j.whugis20150314
[18] 谢钢. GPS原理与接收机设计[M]. 北京: 电子工业出版社, 2009. Xie Gang. Principles of GPS and Receiver Design[M]. Beijing: Publishing House of Electronics Industry, 2009
[19] Yang X H, Gu S F, Gong X P, et al. Regional BDS Satellite Clock Estimation with Triple-Frequency Ambiguity Resolution Based on Undifferenced Observation[J]. GPS Solutions, 2019, 23(2): 33. doi: 10.1007/s10291-019-0828-0
[20] Guo W F, Zuo H M, Mao F Y, et al. On the Satellite Clock Datum Stability of RT-PPP Product and Its Application in One-Way Timing and Time Synchronization[J]. Journal of Geodesy, 2022, 96(8): 52. doi: 10.1007/s00190-022-01638-5
[21] 伍贻威, 杨斌, 肖胜红, 等. 原子钟模型和频率稳定度分析方法[J]. 武汉大学学报(信息科学版), 2019, 44(8): 1226-1232. doi: 10.13203/j.whugis20180058 Wu Yiwei, Yang Bin, Xiao Shenghong, et al. Atomic Clock Models and Frequency Stability Analyses[J]. Geomatics and Information Science of Wuhan University, 2019, 44(8): 1226-1232 doi: 10.13203/j.whugis20180058
[22] Riley W J. Handbook of Frequency Stability Analysis[M]. Boulder: National Institute of Standards and Technology, 2008.
[23] Kroupa V. Noise Properties of PLL Systems[J]. IEEE Transactions on Communications, 1982, 30(10): 2244-2252.
[24] Hajimiri A. Noise in Phase-Locked Loops[C]//Southwest Symposium on Mixed-Signal Design, Austin, USA, 2002.
-
期刊类型引用(12)
1. 贺延伟，贾小林，刘家龙，许瑾，张锐斌，伏军胜，朱永兴. BDS-3与BDS-2基本服务性能对比分析. 导航定位学报. 2022(01): 20-28+75 . 
百度学术
2. 刘家龙，贾小林，陶清瑞，伏军胜，张奋. 多系统广播电离层模型精度评估. 测绘通报. 2022(01): 66-71+78 . 百度学术
3. 姚宜斌，高鑫. GNSS电离层监测研究进展与展望. 武汉大学学报(信息科学版). 2022(10): 1728-1739 . 百度学术
4. 袁运斌，李敏，霍星亮，李子申，王宁波. 北斗三号全球导航卫星系统全球广播电离层延迟修正模型(BDGIM)应用性能评估. 测绘学报. 2021(04): 436-447 . 百度学术
5. 杨毅，胡洪，解雪峰，田香勇. BDS-3/GPS在遮挡环境下定位性能分析. 全球定位系统. 2021(03): 104-110 . 百度学术
6. 杨秋莲，赵镇，胡志刚. 北斗三号SBAS B1c格网电离层算法修正分析. 大地测量与地球动力学. 2021(09): 916-919 . 百度学术
7. 邱封钦，潘雄，罗小敏，赖祖龙，蒋可，王广兴. 综合半参数核估计和自回归补偿的全球电离层总电子含量预报模型. 地球物理学报. 2021(09): 3021-3029 . 百度学术
8. 高清文，赵国忱. CEEMD与GRNN神经网络电离层TEC预报模型. 全球定位系统. 2021(04): 76-84 . 百度学术
9. 赵建，安家春，艾松涛，王泽民，朱李忠，宋翔宇. 北斗三号系统在环南极地区的信号质量与基本定位服务性能分析. 极地研究. 2021(03): 337-350 . 百度学术
10. 宋伟伟，何成鹏，辜声峰. 不同纬度区域电离层增强PPP-RTK性能分析. 武汉大学学报(信息科学版). 2021(12): 1832-1842 . 百度学术
11. 方欣颀，范磊. BDS-2/BDS-3伪距单点定位精度分析. 全球定位系统. 2020(01): 19-25 . 百度学术
12. 周金宁，赵齐乐，胡志刚，蔡洪亮，周仁宇. 北斗不同电离层模型精度分析. 测绘通报. 2020(08): 71-75 . 百度学术
其他类型引用(15)
下载:
计量
- 文章访问数: 561
- HTML全文浏览量: 155
- PDF下载量: 114
- 被引次数: 27
