Equal Districting for Emergency Planning of Citywide Emergency Test
-
摘要:
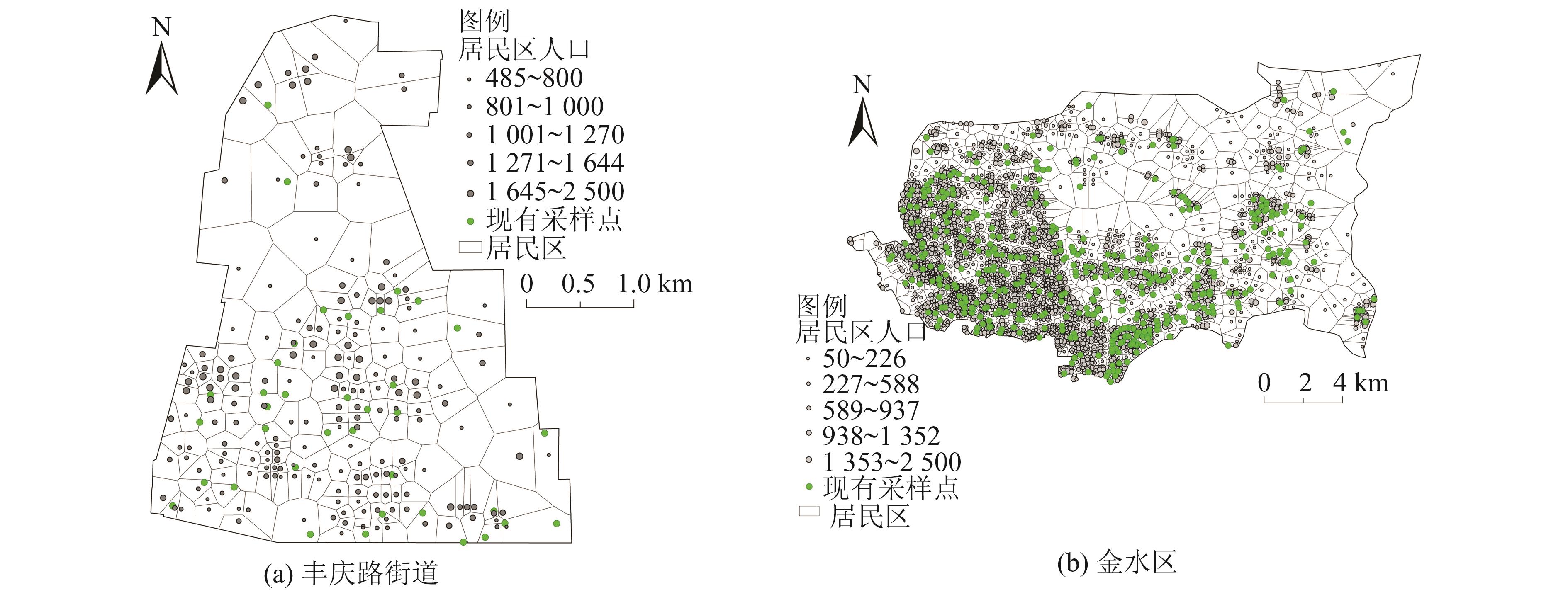
突发公共卫生事件中的全员应急检测是落实动态清零的关键环节,通常采用分片包干的形式组织实施。而全员应急检测具有任务繁重、时间紧急和专业人员短缺等特点,且存在人员聚集风险。因此,以医护人员工作量均等、居民方便参与、减少人员流动为目标,使用均等分区模型解决服务划片问题,为快速有效地完成应急检测任务提供保障。以中国河南省郑州市丰庆路街道和金水区两个尺度的区域为例,验证了基于均等分区模型进行服务划片的可行性和优势。结果表明,所提模型能够有效划定服务区域,且在责任区的平等性和服务空间可达性方面均优于现有规划。均等分区模型不需要遴选候选设施区位,根据人力资源确定分片数量,适合分片包干类型的应急服务规划,具有较高的实用价值。
Abstract:ObjectivesThe citywide emergency test is widely used to identify all the infected persons, and thus contributes to realize "dynamic zero" during the public health emergency. Citywide emergency test is usually implemented by assigning nurses and material resources to the service areas. However, citywide emergency test is often characterized by the extremely heavy task covering millions of residents, the very limited time such as within one day or even 6 hours, and the shortage of nurses, laboratory professionals and equipment. It is also a possible risk factor that causes infectious disease. Therefore, the design of the service areas is crucial for successful citywide emergency test.
MethodsThis paper proposes the equal districting model to design the equal, compact and contiguous areas as service areas for emergency test. The proposed model is tested in two urban areas in different scales, which are Fengqing road subdistrict and Jinshui district in Zhengzhou, Henan Province, China.
ResultsThe results show that the service areas can be effectively delineated, and are much better than the existing planning models in terms of the equality of responsibility districts and the spatial accessibility of service.
ConclusionsSince the delineation of service areas does not depend on the candidate locations that used in classical location models, the proposed equal districting approach has application potentials in some scenarios of emergency planning.
-
建成区是人类活动的重要场所,受到人口增长和社会经济发展的影响,展现出多种变化,如新增、拆除和重建。准确地获取建成区的变化信息,尤其是在较高的空间分辨率下,对可持续的城市发展和生态环境保护有着重要意义。然而,常用的全监督变化检测方法严重依赖大量高质量的像素级样本,这些样本通常具有较高的获取代价。对此,引入3种弱样本,即无样本、图像级样本(一张图像对应一个标签)和众源样本,开展高分辨率建成区变化检测方法研究。采用资源三号高分辨率多视角卫星,制作了对应3种弱样本的变化检测数据集,用于训练和测试。主要研究内容和结论如下:
1)提出了平面和垂直特征融合的无监督建成区变化检测方法。在无样本场景下,传统方法依赖人工设计特征,而这些特征通常仅从空间或时间维度出发,较难全面描述建成区。针对这一问题,提出了平面和垂直特征融合的策略。此外,引入多时序影像(每年一景),设计了面向对象的时序改正算法,获取到时空一致的多时序特征。并提出了基于二阶差分的多时序变化检测方法,实现了自动的建成区变化区域及变化时间检测。实验结果表明,所提方法在建成区变化区域检测上获取到约90%的F1值,在变化时间检测上达到了约92%的总体精度(一年容忍度匹配)。实例说明,所提方法有潜力应用于建成区的自动监测。
2)提出了多尺度特征融合的图像级监督建成区变化检测方法。在图像级样本场景下,针对现有的图像级语义分割方法通常依赖单尺度的深层特征,未充分利用建成区的多尺度特性的问题,提出多尺度类激活图算法,获取到像素级建成区伪标签,并对该伪标签进行在线噪声改正,以稳健地训练建筑提取网络。该网络被迁移到多时序影像上以生成变化伪标签,用于优化建成区变化检测网络。实验结果显示,所提方法在建成区变化检测上达到了78.6%(上海)和82.4%(北京)的F1值,与常用的分类后比较方法相比,分别提升了14.0%和11.4%。实例说明,所提方法能够较好地利用图像级样本实现像素级的建成区变化检测,有潜力应用于像素级样本有限的场景。
3)提出了全层特征融合的众源监督建筑变化检测方法。首先,在众源样本场景下,针对来自高德地图的众源样本包含噪声、极易降低网络的泛化性能的问题,提出了噪声稳健的网络训练策略,以优化建筑提取网络参数。然后,将该参数迁移至多时序影像,生成可靠的变化伪标签,以优化全层特征融合的建筑变化检测网络。实验结果表明,所提方法在建筑变化检测上获取到78.3%(上海)和81.7%(北京)的F1值,与常用的变化向量法相比,分别提升了16.1%和13.6%。实例说明,众源样本能够缓解参考样本获取代价较高的问题,有潜力应用于参考样本有限或者完全缺乏的场景。
http://ch.whu.edu.cn/cn/article/doi/10.13203/j.whugis20220222 -
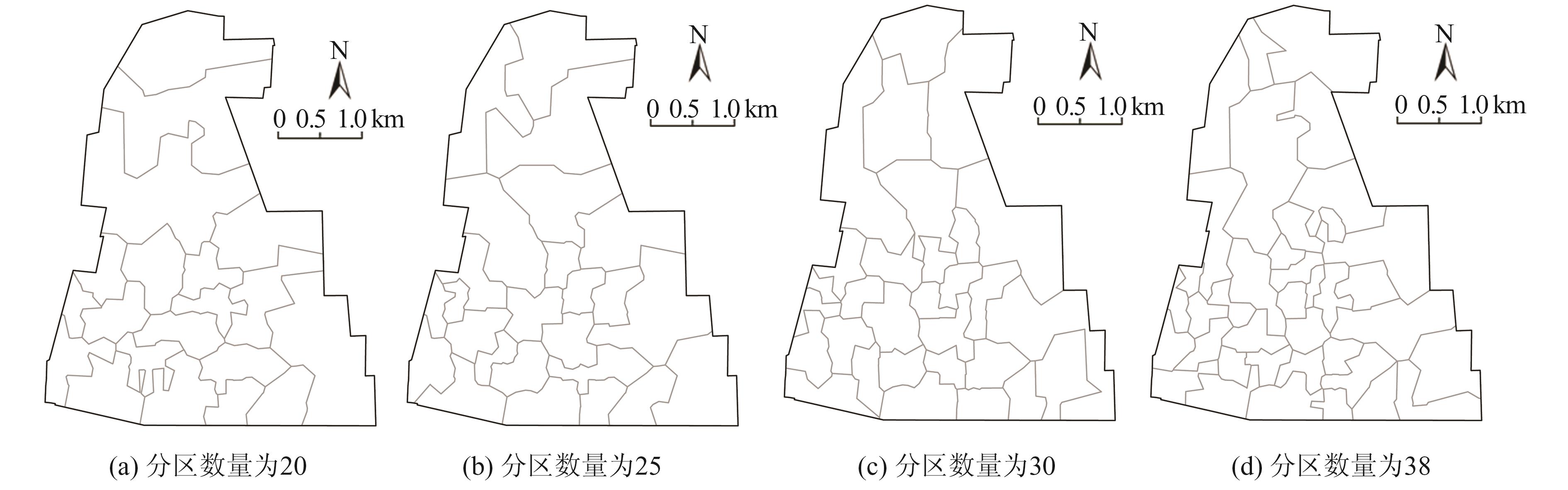
表 1 丰庆路街道均等分区结果
Table 1 Statistics of Solutions in Fengqing Road Subdistrict
分区数量 平均距离/m 最小片区人口数 最大片区人口数 平均偏差/% 计算时间/s 15 232.28 13 231 16 081 6.03 2.40 20 231.98 9 968 12 067 6.91 2.50 25 183.66 8 086 9 670 5.00 1.76 30 175.33 6 707 8 076 4.44 3.96 35 167.79 5 676 6 916 4.50 4.58 38 162.61 5 230 6 354 3.94 4.69 40 147.97 5 000 6 034 4.68 5.05 表 2 金水区均等分区结果
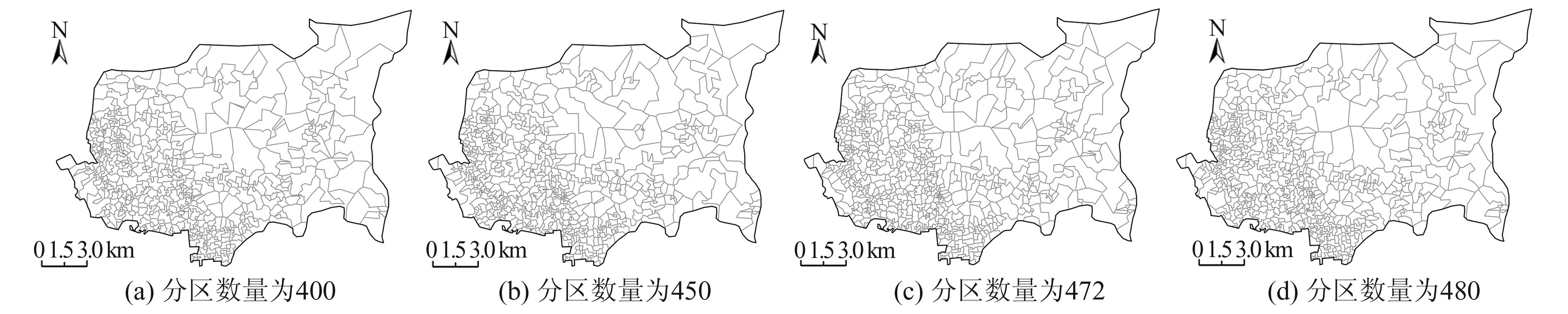
Table 2 Statistics of Solutions in Jinshui District
分区数量 平均距离/m 最小片区人口数 最大片区人口数 平均偏差/% 计算时间/s 400 195.82 4 374 6 000 4.94 835.94 410 187.91 4 000 5 808 5.29 860.80 420 187.92 4 000 6 000 5.68 699.65 430 186.34 3 800 5 258 5.44 859.48 440 181.95 3 800 5 310 5.43 835.94 450 172.19 3 734 5 048 5.09 940.77 460 168.63 3 528 5 285 4.62 821.54 470 167.47 3 256 5 050 4.65 620.80 472 166.78 3 231 5 000 5.41 870.88 480 164.49 3 500 5 048 5.40 960.43 -
[1] YANIK S, BOZKAYA B. A Review of Districting Problems in Health Care[M]//International Series in Operations Research & Management Science. Cham: Springer, 2020: 31-55.
[2] 於家, 温家洪, 陈芸, 等. 基于应急疏散智能体模型模拟的城市避难所空间配置: 以上海市静安区为例[J]. 地理学报, 2017, 72(8): 1458-1475. YU Jia, WEN Jiahong, CHEN Yun, et al. Spatial Configuration of Urban Shelters Based on Simulation Using Emergency Evacuation Agent-Based Model: A Case Study in Jing’an District, Shanghai[J]. Acta Geographica Sinica, 2017, 72(8): 1458-1475.
[3] TRIVEDI A, SINGH A. A Hybrid Multi-Objective Decision Model for Emergency Shelter Location-Relocation Projects Using Fuzzy Analytic Hierarchy Process and Goal Programming Approach[J]. International Journal of Project Management, 2017, 35(5): 827-840.
[4] 卢永华, 李爽. 改进G2SFCA的深圳市室内应急避难场所空间可达性研究[J]. 武汉大学学报(信息科学版), 2019, 44(9): 1391-1398. LU Yonghua, LI Shuang. Spatial Accessibility of Indoor Emergency Shelters Based on Improved G2SFCA in Shenzhen City[J]. Geomatics and Information Science of Wuhan University, 2019, 44(9): 1391-1398.
[5] WEI L, LI W P, LI K L, et al. Decision Support for Urban Shelter Locations Based on Covering Model[J]. Procedia Engineering, 2012, 43: 59-64.
[6] TASSONE J, CHOUDHURY S. A Comprehensive Survey on the Ambulance Routing and Location Problems[EB/OL]. [2020-05-28]. https://arxiv.org/abs/2001.05288v1.
[7] LEKNES H, AARTUN E S, ANDERSSON H, et al. Strategic Ambulance Location for Heterogeneous Regions[J]. European Journal of Operational Research, 2017, 260(1): 122-133.
[8] SCHMID V, DOERNER K F. Ambulance Location and Relocation Problems with Time-Dependent Travel Times[J]. European Journal of Operational Research, 2010, 207(3): 1293-1303.
[9] YAO J, ZHANG X X, MURRAY A T. Location Optimization of Urban Fire Stations: Access and Service Coverage[J]. Computers, Environment and Urban Systems, 2019, 73: 184-190.
[10] YANG L L, JONES B F, YANG S H. A Fuzzy Multi-Objective Programming for Optimization of Fire Station Locations Through Genetic Algorithms[J]. European Journal of Operational Research, 2007, 181(2): 903-915.
[11] SAVSAR M. Fire Station Location Analysis in a Metropolitan Area[J]. International Journal of Industrial and Systems Engineering, 2014, 16(3): 365.
[12] AKTAŞ E, ÖZAYDıN Ö, BOZKAYA B, et al. Optimizing Fire Station Locations for the Istanbul Metropolitan Municipality[J]. Interfaces, 2013, 43(3): 240-255.
[13] TOREGAS C, SWAIN R, REVELLE C, et al. The Location of Emergency Service Facilities[J]. Operations Research, 1971, 19(6): 1363-1373.
[14] CHURCH R, VELLE C R. The Maximal Covering Location Problem[J]. Papers in Regional Science, 1974, 32(1): 101-118.
[15] HAKIMI S L. Optimum Locations of Switching Centers and the Absolute Centers and Medians of a Graph[J]. Operations Research, 1964, 12(3): 450-459.
[16] HAKIMI S L. Optimum Distribution of Switching Centers in a Communication Network and some Related Graph Theoretic Problems[J]. Operations Research, 1965, 13(3): 462-475.
[17] KONG Y F. A Matheuristic Algorithm for the Single-Source Capacitated Facility Location Problem and Its Variants[EB/OL]. [2021-11-02]. https://arxiv.org/abs/2112.12974v1.
[18] SITEPU R, PUSPITA F M, ROMELDA S, et al. Set Covering Models in Optimizing the Emergency Unit Location of Health Facility in Palembang[J]. Journal of Physics: Conference Series, 2019, 1282(1): 012008.
[19] DENG X C. Location-Routing Problem of Emergency Facilities Under Uncertain Demand by Branch-Price and Cut[J]. Journal of Mathematics, 2021, 2021(1): 9152989.
[20] MOADI S, MOHAYMANY A S, BABAEI M. Application of Imperialist Competitive Algorithm to the Emergency Medical Services Location Problem[J]. International Journal of Artificial Intelligence & Applications, 2011, 2(4): 137-147.
[21] 张彤, 马帅, 沈宏. 面向公平分配的时变应急服务覆盖优化[J]. 武汉大学学报(信息科学版), 2017, 42(12): 1681-1687. ZHANG Tong, MA Shuai, SHEN Hong. Equity-Oriented Time-Varying Emergency Management Service Coverage Optimization[J]. Geomatics and Information Science of Wuhan University, 2017, 42(12): 1681-1687.
[22] HUANG B, LIU N, CHANDRAMOULI M. A GIS Supported Ant Algorithm for the Linear Feature Covering Problem with Distance Constraints[J]. Decision Support Systems, 2006, 42(2): 1063-1075.
[23] Fattahi P, Bagheri H, BabaeiMorad S. A Mathematical Model for Fire Station Locating with Maximal Covering Location and Multi Period Approach [J]. Modern Research in Decision Making, 2017, 2(3): 193-213.
[24] AYTUG H, SAYDAM C. Solving Large-Scale Maximum Expected Covering Location Problems by Genetic Algorithms: A Comparative Study[J]. European Journal of Operational Research, 2002, 141(3): 480-494.
[25] LEE Y H, KEUM H J, HAN K Y, et al. A Hierarchical Flood Shelter Location Model for Walking Evacuation Planning[J]. Environmental Hazards, 2021, 20(4): 432-455.
[26] 王玉璟, 孔云峰. 设施服务分区问题的求解算法框架设计[J]. 武汉大学学报(信息科学版), 2021, 46(5): 726-735. WANG Yujing, KONG Yunfeng. An Algorithm Framework for Facility Service Districting Problem[J]. Geomatics and Information Science of Wuhan University, 2021, 46(5): 726-735.
[27] TASNÁDI A. The Political Districting Problem: A Survey[J]. Society and Economy, 2011, 33(3): 543-554.
[28] HESS S W, SAMUELS S A. Experiences with a Sales Districting Model: Criteria and Implementation[J]. Management Science, 1971, 18(4): 41-54.
[29] ZHANG Y, BROWN D E. Police Patrol Districting Method and Simulation Evaluation Using Agent- Based Model & GIS[J]. Security Informatics, 2013, 2(1): 7.
[30] KEANE M. The Size of the Region-Building Problem[J]. Environment and Planning A: Economy and Space, 1975, 7(5): 575-577.
[31] d’AMICO S J, WANG S J, BATTA R, et al. A Simulated Annealing Approach to Police District Design[J]. Computers & Operations Research, 2002, 29(6): 667-684.
[32] BAÇÃO F, LOBO V, PAINHO M. Applying Genetic Algorithms to Zone Design[J]. Soft Computing, 2005, 9(5): 341-348.
[33] KONG Y F. A Hybrid Algorithm for the Equal Districting Problem[C]// Spatial Data and Intelligence. Cham: Springer International Publishing, 2021: 110-120.
[34] RONG P J, ZHENG Z C, KWAN M P, et al. Evaluation of the Spatial Equity of Medical Facilities Based on Improved Potential Model and Map Service API: A Case Study in Zhengzhou, China[J]. Applied Geography, 2020, 119: 102192.





 下载:
下载: