Relationship and Geometric Interpretation of Adjustment Models
-
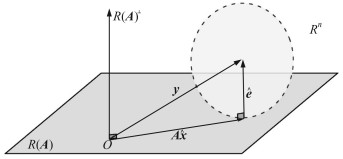
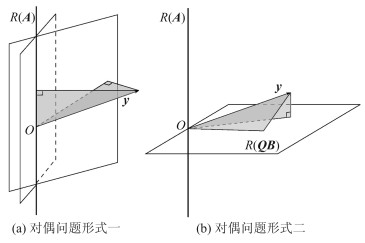
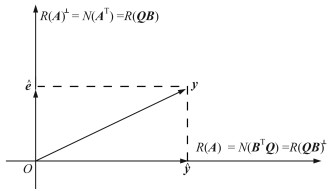
摘要: 高斯马尔可夫模型（间接平差模型）、条件平差模型和高斯赫尔默特模型是3种经典平差模型，通常根据参数个数的不同从模型形式上予以表述，但该方式不能反映模型间的本质关系。首先，以矩阵分析理论为基础，依据系数矩阵的半正交性实现了高斯马尔可夫模型与高斯赫尔默特模型的转换，完善了3种平差函数模型之间的数学转换关系，揭示了模型间内在的数学联系。然后，基于线性空间和投影算子，解释了高斯马尔可夫模型和条件平差模型的最小二乘估计，阐述了两类模型的最小二乘目标函数的等价性，并结合勾股定理和对偶性将两种经典目标函数形式扩展至4种等效目标函数，说明了其在空间意义上的构造原理，揭示了目标函数之间的几何关系和内涵，对于平差模型及其估计的数学本质的理解具有理论和应用价值。Abstract:Objectives Gauss-Markov model, the model with condition equations and Gauss-Helmert model are three classical adjustment models. Gauss-Helmert model can be seen as the combined form of the other two models. They are usually distinguished by the number of unknown parameters. However, this classification method cannot reflect the essential relationship between them. In addition, the connections between Gauss-Markov model and the conditional model has been intensively investigated, while that of Gauss-Helmert model has been ignored.Methods Some equivalent objective functions of the adjustment of the least-square in the context of Gauss-Markov model and the conditional model are proposed based on the projection theory and the dual optimization technique. Based on the theory of matrix analysis, this paper realizes the conversion between Gauss-Markov model and Gauss-Helmert model according to partial orthogonality of coefficient matrices, thus completing the structure of these models and revealing the mathematical relationships.Results The least-square adjustment within Gauss-Markov model and the model with condition equations are explained geometrically by employing the linear space and projector. The equivalence of the objective functions of these two models are also expounded. Combining the Pythagorean theorem and duality theory, we extend them to four equivalent objective functions and illustrate the construction principle. The transformation between Gauss-Markov model and Gauss-Helmert model are achieved by the so-called partial-orthogonality.Conclusions The geometric reasoning of the least-squares adjustment is analyzed once more. Two objection functions are newly proposed, and the geometric relation and connotation between objective functions are revealed. Gauss-Helmert model is not only the combined model in terms of the model appearance, these three adjustment models can actually be mutually transformed. As a result, the understanding of the mathematical nature of adjustment models and the corresponding estimation in this paper has theoretical and practical value.
-
Keywords:
- adjustment /
- least-square estimate /
- orthogonal projection /
- duality
-
目前，北斗导航卫星系统(BDS)已实现局域覆盖，随着系统建设的不断完善和应用的不断拓展，与之相关的各类数据处理软件的开发成为重要的研究内容。因此，自主开发北斗高精度数据处理软件，成为发展高精度位置服务的迫切任务[1-8]。因北斗导航卫星系统与GPS在星座构造、坐标框架、时间系统、信号频率等方面具有明显差异[9-15]，现有的高精度GPS数据处理软件无法直接处理北斗数据。本文针对北斗高精度数据处理的系统设计、数据流、功能模块及高精度算法实现等进行了研究，研制开发了一套高精度北斗基线解算软件BGO(BeiDou Navigation Satellite System/Global Positioning System Office)，并将其用于高速铁路高精度控制测量建网。通过与商业软件TGO(Trimble Geomatics Office)和TBC(Trimble Business Center)，及高精度科研软件Bernese进行对比测试、性能分析，验证了该软件的正确性和有效性。
1 系统的设计与模块算法的实现
1.1 系统设计与数据流分析
北斗和GPS基线解算软件主要包含北斗基线处理、GPS基线处理及联合基线处理3大模块。各模块间相互独立，但使用相同的数据结构，且数据流基本一致。数据处理流程如图 1所示。
基线解算之前，需选择有效双频观测数据，具体包含低高度角卫星剔除、观测值粗差剔除、星历未获取观测数据剔除等。剔除质量较差的观测数据可通过可视化的方式实现。通过双频数据组合有效消除电离层延迟影响，伪距消电离组合能算出测站精确至10 m内的概略位置，从而形成网络拓扑图，便于用户查看站点的平面分布。基线解算时，北斗与GPS独立系统数据处理算法相同；联合处理需选择统一的坐标和时间框架，随着多余观测数的增加，还需设置合理的模糊度固定限值。基线解算后，进行网平差，应剔除不合格基线，直至平差结果满足要求。
1.2 高精度基线解算算法实现
高精度基线解算利用双差观测量建立误差方程，北斗双差观测量构造如式(1)：
$$ \mathit{\Delta} \nabla L^{{C_m}{C_n}}_{{S_i}{S_j}} = \left( {L^{{C_n}}_{{S_j}} - L^{{C_n}}_{{S_i}}} \right) - \left( {L^{{C_m}}_{{S_j}} - L^{{C_m}}_{{S_i}}} \right) $$ (1) 式中，Δ▽L表示双差观测量；Si和Sj表示任意站点；Cm和Cn表示任意北斗卫星。
依据式(1)构建的双差观测量，建立误差方程，如式(2)：
$$ \left[ \begin{array}{l} \mathit{\Delta} \nabla \boldsymbol{\varPhi} \\ \mathit{\Delta} \nabla \boldsymbol{P} \end{array} \right] = \boldsymbol{BX} + \boldsymbol{A}\mathit{\Delta} \nabla \boldsymbol{N} + \boldsymbol{V} $$ (2) 式中，Δ▽Φ和Δ▽P分别表示卫星载波相位和伪距双差观测量；X表示基线向量；Δ▽N表示双差整周模糊度；B和A为系数阵；V为残差向量。
利用式(2)构建的误差方程，解算基线向量和双差整周模糊度浮点解。利用LAMBAD方法[16, 17]固定双差整周模糊度后去除。再利用载波相位观测值获取高精度基线向量结果。基线解算过程中，主要利用抗差估计的切比雪夫多项式拟合法[18]及MW-GF组合法[19]探测与修复周跳。
对北斗和GPS双系统基线解算，只需将各系统的双差观测量误差方程叠加后平差计算，即可实现双系统联合基线解算。但需注意，星间差分需选择同一系统卫星，否则会引入系统间信号硬件延迟[20]，影响双差整周模糊度的固定。另外，北斗和GPS在时间框架、坐标框架等存在一定差异，双系统联合解算需保证框架的统一。
北斗和GPS时间转换公式如式(3)：
$$ {t_C} = {t_G}-14\;{\rm{s}} $$ (3) 式中，tC和tG分别表示北斗时和GPS时，两者均为原子时，起算原点不同[13]。
北斗和GPS坐标转换公式如式(4)：
$$ \begin{array}{c} \left[ {\begin{array}{*{20}{c}} {{X_C}}\\ {{Y_C}}\\ {{Z_C}} \end{array}} \right] = \left[ {\begin{array}{*{20}{c}} {{X_G}}\\ {{Y_G}}\\ {{Z_G}} \end{array}} \right] + \left[ {\begin{array}{*{20}{c}} {{T_X}}\\ {{T_Y}}\\ {{T_Z}} \end{array}} \right] + \\ \left[ {\begin{array}{*{20}{c}} D&{ - {R_Z}}&{{R_Y}}\\ {{R_Z}}&D&{ - {R_X}}\\ { - {R_Y}}&{{R_X}}&D \end{array}} \right]\left[ {\begin{array}{*{20}{c}} {{X_G}}\\ {{Y_G}}\\ {{Z_G}} \end{array}} \right] \end{array} $$ (4) 式中，北斗坐标(XC，YC，ZC)与GPS坐标(XG，YG，ZG)可通过七参数TX、TY、TZ、D、RX、RY、RZ进行转换。北斗CGCS2000坐标系采用ITRF97框架2000历元的坐标和速度场，当前GPS WGS84坐标和ITRF08基本一致。因此，可利用ITRF97框架2000历元与ITRF08间转换的七参数(ITRF网站公布)实现北斗与GPS坐标框架的统一[11, 12]。
2 BGO数据处理实例与性能测试
2.1 高速铁路CPI控制网基线解算
处理高速铁路CPI控制网时，通过读取观测文件和星历文件，单点定位生成控制网的基线网络拓扑图，如图 2所示。基线解算前，设置相关参数包括卫星截止高度角、误差限差参数、框架、对流层模型、电离层模型、模糊度Ratio值、同步最小观测历元数等。设置完成后，可选择北斗、GPS、联合3种模式进行基线解算。基线解算完成后，软件界面中将显示解算的基线分量及其精度，并可显示残差向量检核基线解算效果。
2.2 BGO、TGO、Bernese软件处理GPS基线结果比较
为了测试BGO解算GPS基线的正确性，将其与TGO和Bernese软件处理结果进行了比较，得到57条GPS基线(基线最长6 667 m，最短446 m)的比较结果，如图 3所示。
![]()
图 3 BGO、TGO、Bernese软件处理GPS基线分量比较Figure 3. Comparing GPS Baseline Components from BGO, TGO and Bernese Software图 3(a)、3(b)分别表示BGO软件与TGO、Bernese软件处理GPS基线分量的差值ΔX、ΔY、ΔZ。图 3(a)中，BGO和TGO有52条基线在X、Y、Z方向的分量差值均在2 cm内，有48条基线各分量差值在mm级。TGO解算少量基线验后方差分量超限，与BGO基线分量差值较大。图 3(b)中，BGO和Bernese有55条基线在X、Y、Z方向的分量差值均在2 cm内，有49条基线各分量差值在mm级。
图 4(a)~4(c)分别表示BGO、TGO、Bernese软件处理GPS基线的内符合精度σX、σY、σZ(BGO、TGO、Bernese软件基线解算精度分别精确至0.1 mm、1 mm和0.1 mm)。整体上，约90%的基线3个软件的解算精度相当。
![]()
图 4 BGO、TGO、Bernese的GPS基线内符合精度比较Figure 4. Comparing GPS Baseline Precision from BGO, TGO and Bernese Software2.3 BGO、TBC软件处理北斗与GPS联合基线结果
为了测试BGO解算北斗与GPS联合基线的性能，本文选用美国Trimble的商业软件TBC与之进行比较。同上57条基线，每条基线观测数据均包含北斗与GPS观测数据。图 5展示了BGO和TBC处理北斗与GPS联合基线分量的差值ΔX、ΔY、ΔZ。图 5可见，98%的基线分量差值分布在mm级，表明BGO软件处理联合基线能达到与TBC软件相当的水平。另外，两者内符合精度绝大部分均在mm级，故图 5中未加以比较。
![]()
图 5 BGO与TBC软件处理北斗与GPS联合基线分量比较Figure 5. Comparing BDS and GPS Combined Baseline Components from BGO and TBC Software由此可知，BGO软件处理GPS基线、北斗与GPS联合基线的内外符合精度能达到TGO、Bernese、TBC相当的水平。因此，以BGO软件处理GPS、北斗与GPS联合基线结果为参考值，分析该软件处理北斗基线结果的正确性和可靠性，如图 6和图 7所示。图 6比较了北斗与GPS、联合基线分量的差值，图 7比较了北斗、GPS、联合基线解算的内符合精度。
![]()
图 6 BGO软件处理北斗与GPS、联合基线分量比较Figure 6. Comparing BDS, GPS and BDS/GPS Combined Baseline Components from BGO Software![]()
图 7 北斗、GPS、联合基线解的内符合精度统计Figure 7. The Statistics of Precision of BDS, GPS and BDS/GPS Combined Baseline Solutions图 6(a)表示BGO软件处理北斗与GPS基线分量的差值ΔX、ΔY、ΔZ，其中有43条基线在X、Y、Z方向上的分量差值Δx、Δy、Δz在2 cm内，有31条基线在X、Y、Z方向上的分量差值在mm级。图 6(b)表示BGO软件处理北斗与联合基线分量的差值，其中有54条基线在X、Y、Z方向上的分量差值在2 cm内，有38条基线在X、Y、Z方向上的分量差值在mm级(图 6中第6条基线北斗为浮点解，各分量差值结果较大，图中置为0)。
图 7中，93%的联合基线在X、Y、Z方向上的分量精度分别优于0.5 mm、1 mm、0.5 mm；约90%的北斗基线和95%的GPS基线在X、Y、Z方向上的分量精度分别优于1 mm、2 mm、1 mm。由北斗、GPS、联合基线3者精度比较可知，在北斗试运行阶段，GPS基线内符合精度略优于北斗，北斗与GPS联合系统基线内符合精度明显高于独立系统。
2.4 BGO基线网平差及其精度分析
BGO具备网平差功能，根据网平差后的基线分量改正数、相对中误差、点位精度等判断基线解算结果的可靠性。对上述解算的北斗、GPS、联合基线分别进行无约束网平差。
北斗、GPS、联合基线无约束网平差的平差改正数δX、δY、δZ绝大部分在±1 cm内，如图 8(a)~8(c)所示。最弱边相对中误差优于5.5 ppm(规范限值)，具体见表 1。据图 8、表 1及《高速铁路工程测量规范》[21]可知，BGO能合理稳定地解算北斗、GPS及联合基线，解算结果中的基线向量改正数、最弱边相对中误差、最弱点点位精度均满足CPI控制测量要求，各系统解算均能精确获得24个CPI控制点坐标。
![]()
图 8 GPS、北斗、联合无约束网平差基线向量改正数Figure 8. Baseline Vector Corrections from GPS, BDS and BDS/GPS Combined Unconstrained Adjustment表 1 GPS、北斗、联合无约束平差结果统计Table 1. The Statistics of GPS, BDS and BDS/GPS Combined Unconstrained Adjustment Results解算模式 独立基线 多余观测数 控制点个数 最弱边相对中误差/ppm 最弱点点位精度/mm GPS 55 66 24 3.6 23.6 北斗 51 57 24 3.1 26.9 联合 57 72 24 3.7 17.9 3 结语
本文系统地研究了北斗与GPS联合基线解算的算法，自主开发了北斗高精度基线解算软件BGO。通过实测高铁CPI控制网的数据处理测试表明：软件能进行高精度地处理北斗与GPS数据, 以及北斗与GPS联合数据处理；GPS基线解算性能与天宝TGO软件相当，能达到与Bernese软件一致的精度；北斗与GPS基线处理能达到与TBC相当的水平。BGO最大的优势在于能对北斗和GPS进行联合解算，从而提高北斗或GPS单系统的基线解算合格率和精度。经高速铁路CPI控制网实例测试，证明该软件处理基线结果可用于高精度北斗和GPS测量控制网的数据处理。
-
![]()
图 1 投影示意图（度量由$ \boldsymbol{P} $定义）
Figure 1. Projection Diagram(the Metric is Defined by $ \boldsymbol{P} $)
-
[1] Gauss C F. Méthode Des Moindres Carrés: Mémoires Sur la Combinaison Des Observations[M]. Paris : Mallet-Bachelier, 1855.
[2] Aitken A C. On Least Squares and Linear Combination of Observations[J]. Proceedings of the Royal Society of Edinburgh, 1936, 55: 42-48. doi: 10.1017/S0370164600014346
[3] Bose R C. Mathematical Theory of the Symmetrical Factorial Design[J]. Sankhyā: The Indian Journal of Statistics, 1947, 8(2): 107-166.
[4] Rao C R. Unified Theory of Linear Estimation[J]. Sankhyā: The Indian Journal of Statistics, 1971, 33(4): 371-394.
[5] Haasbroek N D. Investigation of the Accuracy of Krayenhoff's Triangulation in Belgium, the Netherlands and a Part of North Western Germany[M]. Delft: Rijkscommissie voor Geodesie, 1972.
[6] Tienstra J M. Theory of the Adjustment of Normally Distributed Observations[M]. Amsterdam: Argus, 1956.
[7] Helmert F R. Die Ausgleichungsrechnung Nach Der Methode Der Kleinsten Quadrate, Mit Anwendungen Auf Die Geodäsie Und Die Theorie Der Messinstrumente[M]. Leipzig: BG Teubner, 1872.
[8] Neitzel F. Generalization of Total Least-Squares on Example of Unweighted and Weighted 2D Similarity Transformation[J]. Journal of Geodesy, 2010, 84(12): 751-762. doi: 10.1007/s00190-010-0408-0
[9] 方兴, 曾文宪, 刘经南, 等. 三维坐标转换的通用整体最小二乘算法[J]. 测绘学报, 2014, 43(11): 1139-1143. doi: 10.13485/j.cnki.11-2089.2014.0193 Fang Xing, Zeng Wenxian, Liu Jingnan, et al. A General Total Least Squares Algorithm for Three-Dimensional Coordinate Transformations[J]. Acta Geodaetica et Cartographica Sinica, 2014, 43(11): 1139-1143. doi: 10.13485/j.cnki.11-2089.2014.0193
[10] 姚宜斌, 黄书华, 孔建, 等. 空间直线拟合的整体最小二乘算法[J]. 武汉大学学报(信息科学版), 2014, 39(5): 571-574. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201405013.htm Yao Yibin, Huang Shuhua, Kong Jian, et al. Total Least Squares Algorithm for Fitting Spatial Straight Lines[J]. Geomatics and Information Science of Wuhan University, 2014, 39(5): 571-574. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201405013.htm
[11] 姚宜斌, 黄书华, 陈家君. 求解自回归模型参数的整体最小二乘新方法[J]. 武汉大学学报(信息科学版), 2014, 39(12): 1463-1466. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201412014.htm Yao Yibin, Huang Shuhua, Chen Jiajun. A New Method of TLS to Solving the Autoregressive Model Parameter[J]. Geomatics and Information Science of Wuhan University, 2014, 39(12): 1463-1466. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201412014.htm
[12] 姚宜斌, 黄书华, 张良, 等. 求解三维坐标转换参数的整体最小二乘新方法[J]. 武汉大学学报(信息科学版), 2015, 40(7): 853-857. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201507001.htm Yao Yibin, Huang Shuhua, Zhang Liang, et al. A New Method of TLS for Solving the Parameters of Three-Dimensional Coordinate Transformation[J]. Geomatics and Information Science of Wuhan University, 2015, 40(7): 853-857. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201507001.htm
[13] 方兴, 曾文宪, 刘经南, 等. 基于非线性高斯-赫尔默特模型的混合整体最小二乘估计[J]. 测绘学报, 2016, 45(3): 291-296. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB201603007.htm Fang Xing, Zeng Wenxian, Liu Jingnan, et al. Mixed LS-TLS Estimation Based on Nonlinear Gauss-Helmert Model[J]. Acta Geodaetica et Cartographica Sinica, 2016, 45(3): 291-296. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB201603007.htm
[14] Fang X. Weighted Total Least Squares: Necessary and Sufficient Conditions, Fixed and Random Parameters[J]. Journal of Geodesy, 2013, 87(8): 733-749.
[15] Fang X. Weighted Total Least-Squares with Constraints: A Universal Formula for Geodetic Symmetrical Transformations[J]. Journal of Geodesy, 2015, 89(5): 459-469.
[16] 黄令勇, 吕志平, 任雅奇, 等. 多元总体最小二乘在三维坐标转换中的应用[J]. 武汉大学学报(信息科学版), 2014, 39(7): 793-798. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201407008.htm Huang Lingyong, Lü Zhiping, Ren Yaqi, et al. Application of Multivariate Total Least Square in Three-Dimensional Coordinate Transformation[J]. Geomatics and Information Science of Wuhan University, 2014, 39(7): 793-798. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201407008.htm
[17] 常国宾, 张书毕, 刘志平. 从信息论角度理解间接平差[J]. 武汉大学学报(信息科学版), 2021, DOI: 10.13203/j.whugis20210383. Chang Guobin, Zhang Shubi, Liu Zhiping. Understanding the Adjustment from an Information Theoretic Perspective[J]. Geomatics and Information Science of Wuhan University, 2021, DOI: 10.13203/j.whugis20210383.
[18] Krarup T. A Contribution to the Mathematical Foundation of Physical Geodesy[M]// Heidelberg, Berlin: Springer, 2006.
[19] Vaníček P. Tensor Structure and the Least Squares[J]. Bulletin Géodésique, 1979, 53(3): 221-225.
[20] Blaha G. Tensor Structure Applied to the Least-Squares Method, Revisited[J]. Bulletin Géodésique, 1984, 58(1): 1-30.
[21] Meissl P. Least Squares Adjustment: A Modern Approach[M]. Gdańsk : Geodätische Institute der Technischen Universität Graz, 1982.
[22] Teunissen P J G. The Geometry of Geodetic Inverse Linear Mapping and Non-linear Adjustment[M]. Netherlands : Netherlands Geodetic Commission, 1985.
[23] Teunissen P J G. Adjustment Theory: An Introduction[M]. Delft: Delft University Press, 2000
[24] Strang G. Introduction to Applied Mathematics[M]. Wellesley: Wellesley-Cambridge Press, 1986.
[25] Strang G, Borre K. Linear Algebra, Geodesy, and GPS[M]. Wellesley: Wellesley-Cambridge Press, 1997.
[26] Hu Y, Fang X. Linear Estimation Under the Gauss-Helmert Model: Geometrical Interpretation and General Solution[J]. Journal of Geodesy, 2023, 97(5): 44.
[27] Rao C R, Mitra S. Further Contributions to the Theory of Generalized Inverse of Matrices and Its Applications[J]. Sankhyā: The Indian Journal of Statistics, 1971, 33(4): 289-300.
-
期刊类型引用(6)
1. 柯文清 ，陈业滨 ，赵志刚 ，韩德志 ，郭仁忠 . 基于文献计量的新世纪地图可视化研究演变和热点分析. 地理与地理信息科学. 2025(01): 15-23 . 
百度学术
2. 于峰一泽，汤国安，陆鼎阳，林晓芬，胡光辉，沈婕，吴明光. 语言学视角下的地图演化. 地理学报. 2024(01): 171-186 . 百度学术
3. 韩德志，郭仁忠，陈业滨，赵志刚，柯文清. 基于可视化维度理论的泛地图知识推荐方法. 地球信息科学学报. 2024(01): 110-120 . 百度学术
4. 刘强，刘金花，王磊斌，陈鑫，赵志斌，赵晓艳，李英奎. 使用虚拟现实技术提高冰川地貌表达维度的研究. 冰川冻土. 2024(03): 1087-1098 . 百度学术
5. 邓志钢，郭仁忠，陈业滨，马丁，赵志刚，朱维. 面向轨迹可视化的泛地图表达维度关联方法及应用. 测绘通报. 2024(11): 56-60+96 . 百度学术
6. 柯婷，杨品福，任福，李连营，杨晨. 内河航行参考图地图符号形式化表达. 地理空间信息. 2024(12): 102-105 . 百度学术
其他类型引用(4)
下载:
计量
- 文章访问数: 534
- HTML全文浏览量: 92
- PDF下载量: 156
- 被引次数: 10
