Bias Characteristics and Accuracy Analysis of GPS Ultra-Long Baseline Solution
-
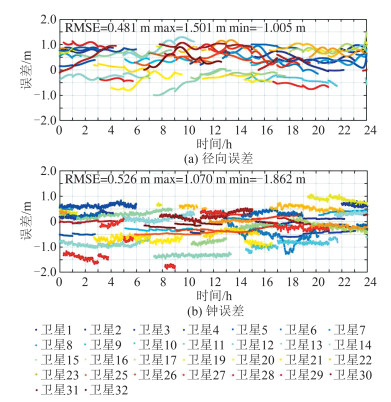
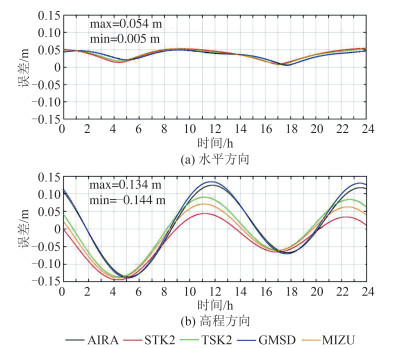
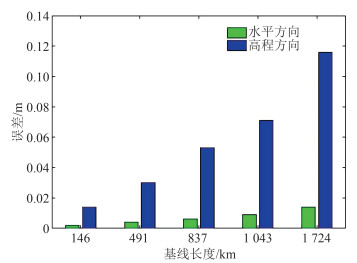
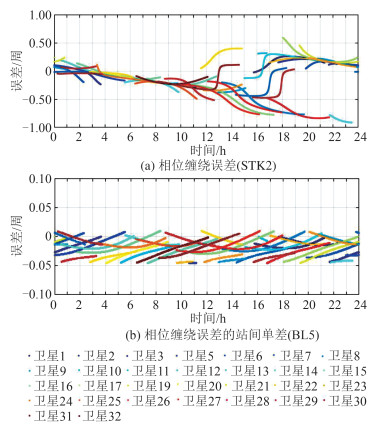
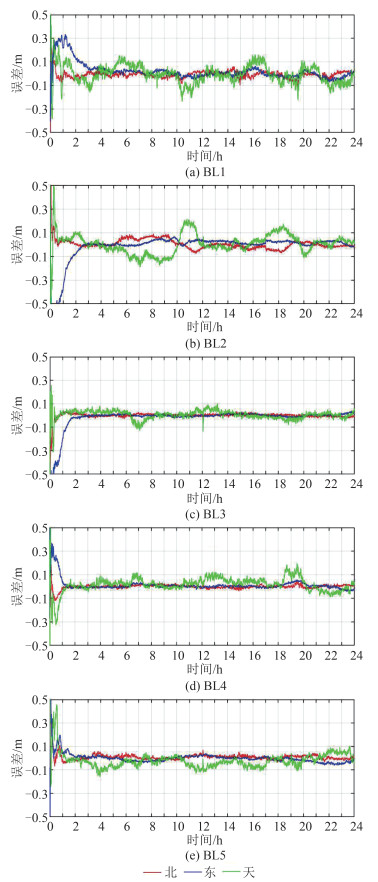
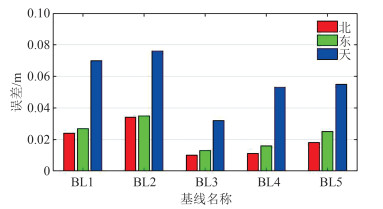
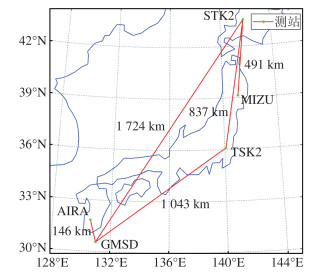
摘要: 基于单基站的超长基线定位技术在地壳形变监测、高精度授时等领域具有广泛应用，但仍有诸多因素制约着超长基线解算精度。从观测方程出发，利用单差观测值对长（超长）基线(146~1 724 km)解算中的卫星轨道误差、对流层延迟误差、地球潮汐误差和相位缠绕误差等误差特性进行了详细分析。分析结果表明，当基线小于500 km时广播星历误差可忽略不计；超过500 km时需要采用精密星历, 同时需要考虑地球潮汐误差的影响；利用参数估计法同时估计基线两端的天顶对流层延迟误差可获得1~2 cm精度; 相位缠绕误差对基线小于2 000 km的解算影响可忽略。基于估计天顶对流层延迟的方法解算了5条长(超长)基线（146 km、491 km、837 km、1 043 km和1 724 km）。实验结果表明，当基线小于500 km时，采用广播星历可获得水平方向优于0.05 m、高程方向优于0.08 m的定位精度；当基线小于2 000 km时，采用超快速精密星历可获得水平方向优于0.025 m、高程方向优于0.055 m的定位精度。解算的初始收敛时间随着基线长度增加而缩短。Abstract:Objectives Considering the advantages of low cost, simple algorithm model and high accuracy, the ultra-long baseline positioning technology was widely used in the fields of crustal deformation monitoring and high-precision timing. However, there are still many factors that may reduce the accuracy of the ultra-long baseline solution, such as satellite orbit error, tropospheric delay error and solid earth tide, etc.Methods In this contribution, characteristics of various errors and bias (such as broadcast ephemeris orbit error, tropospheric delay error, earth tide and phase windup) were analyzed based on ionosphere-free combination model. The rule of error changing with baseline length was analyzed using single difference observations of long(ultra-long) baselines (146-1 724 km).Results The results show that broadcast ephemeris error could be neglected while baseline is less than 500 km. When baseline length exceeded 500 km, precise ephemeris was needed to reduce the influence of orbital errors on positioning. The accuracy of the zenith tropospheric delay calculated by GPT2+Saastamoninen model ranged from centimeter to decimetre, and the method of simultaneously estimating zenith tropospheric delays at both stations could provide accuracy of 1-2 cm. Compared with the horizontal direction, the vertical direction of long baseline was more significantly affected by solid Earth tides which must be corrected in the solution of long baseline. Phase wind-up error could be neglected while baseline is less than 2 000 km.Conclusions The experimental results of five long(ultra-long) baselines (146 km, 491 km, 837 km, 1 043 km and 1 724 km) solutions were presented using estimating zenith tropospheric delays at both stations of baseline. While baseline is less than 500 km, the positioning precision based on broadcast ephemeris is better than 0.05 m and 0.08 m in horizontal and vertical direction, respectively. While baseline is less than 2 000 km, the positioning precision based on ultra-rapid precise ephemeris is better than 0.025 m and 0.055 m in horizontal and vertical directions, respectively. The initial convergence time of the baseline solution decreased as the lengths of baseline increased.
-
Keywords:
- ultra-long baseline /
- zenith tropospheric delay /
- solid Earth tides /
- phase wind-up
-
-
![]()
图 2 TSK2站广播星历轨道径向误差和钟差
Figure 2. Broadcast Ephemeris Radial Error and Clock Offset at TSK2 Site
![]()
图 3 不同基线中星历轨道误差对站间单差的影响
Figure 3. Effects of Broadcast Ephemeris Orbit Error on Single Difference Observation in Each Baseline
![]()
图 4 不同方法计算的天顶对流层延迟对比
Figure 4. Comparison of Zenith Tropospheric Delay Calculated by Different Methods
![]()
图 6 各基线中潮汐误差的站间单差最大值
Figure 6. Max Single Differences of Tides Error Between Stations in Each Baseline
![]()
图 7 STK2站相位缠绕误差和站间单差
Figure 7. Antenna Phase Wind-Up Error at STK2 Site and Single Difference at BL5
![]()
图 8 各条基线在北、东、天方向的误差变化
Figure 8. Error Variation of Each Baseline Solution at North, East and Up Components
表 1 基线名称及长度
Table 1 Name and Length for Each Baseline
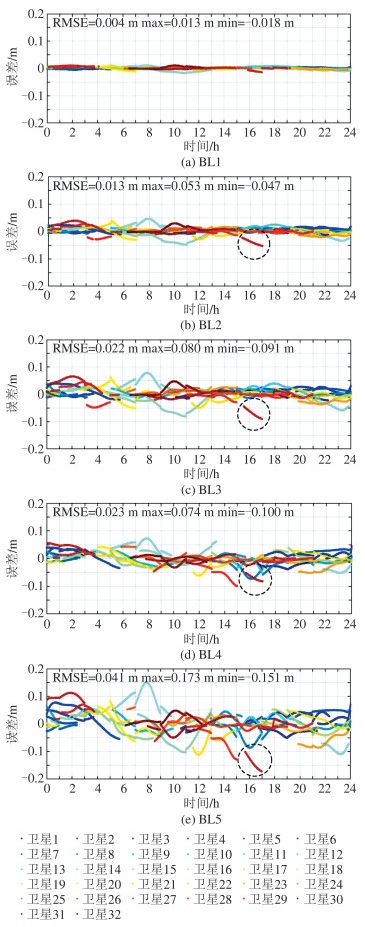
名称 测站 长度/km BL1 AIRA-GMSD 146 BL2 MIZU-STK2 491 BL3 STK2-TSK2 837 BL4 TSK2-GMSD 1 043 BL5 GMSD-STK2 1 724 
下载: 导出CSV
表 2 广播星历轨道误差对单差的影响
Table 2 Effects of Broadcast Orbit Error on Single Difference
名称 长度/km 实测误差/m 理论误差/m BL1 146 0.004 0.004 BL2 491 0.013 0.012 BL3 837 0.022 0.021 BL4 1 043 0.023 0.026 BL5 1 724 0.041 0.043
下载: 导出CSV
表 3 天顶对流层延迟的估计偏差/m
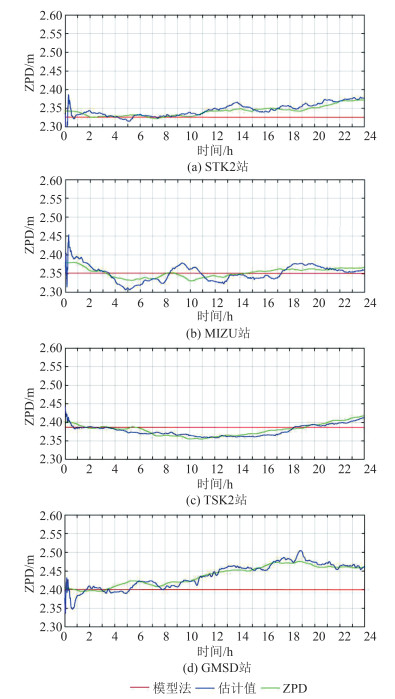
Table 3 Bias Between ZPD and Estimated ZTD/m
测站名 STK2 MIZU TSK2 GMSD Bias 0.004 -0.001 -0.003 0.000 RMSE 0.008 0.015 0.007 0.012
下载: 导出CSV
表 4 基线解算的策略
Table 4 Baseline Solution Strategies
项目 处理策略 观测频率 GPS L1+L2 参数估计 Kalman滤波 截止高度角/（°) 10 采样率/s 30 模糊度参数 参数估计(浮点解) 坐标参数 参数估计 电离层延迟 无电离层组合 天顶对流层干延迟 Saastamoninen改正 天顶对流层湿延迟 GMF+随机游走模型估计 卫星星历 BL1、BL2采用广播星历，BL3、BL4、BL5采用超快速精密星历 天线相位改正 igs14.atx 地球固体潮改正 IERS Conventions 2010
下载: 导出CSV
-
[1] 刘刚, 谭凯, 彭懋磊, 等. 用超长基线解算分析汶川地震动态形变特征[J]. 大地测量与地球动力学, 2011, 31(5): 14-19. doi: 10.3969/j.issn.1671-5942.2011.05.004 Liu Gang, Tan Kai, Peng Maolei, et al. Using Ultra Long Baseline to Measure Co-seismic Kinematic Deformations of Wenchuan Earthquake[J]. Journal of Geodesy and Geodynamics, 2011, 31(5): 14-19. doi: 10.3969/j.issn.1671-5942.2011.05.004
[2] 张钰玺, 张小红, 刘全海, 等. 航空测量场景下的中长基线动态定位方法[J]. 测绘学报, 2019, 48(7): 871-878. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB201907009.htm Zhang Yuxi, Zhang Xiaohong, Liu Quanhai, et al. A Method of Dynamic Positioning with the Medium and Long Baseline for Aerial Measurement Scenarios[J]. Acta Geodaetica et Cartographica Sinica, 2019, 48(7): 871-878. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB201907009.htm
[3] 成枢, 冯子帆. 中长基线的定位精度分析[J]. 测绘地理信息, 2018, 43(1): 59-62. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXG201801014.htm Cheng Shu, Feng Zifan. Accuracy Analysis of Medium-Long Baseline Positioning[J]. Journal of Geomatics, 2018, 43(1): 59-62. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXG201801014.htm
[4] Baybura T, Tiryakioğlu İ, Uğur M A, et al. Examining the Accuracy of Network RTK and Long Base RTK Methods with Repetitive Measurements[J]. Journal of Sensors, 2019, DOI: 10.1155/2019/3572605.
[5] Choi B K, Roh K M, Lee S J. Long Baseline GPS RTK with Estimating Tropospheric Delays[J]. Journal of Positioning, Navigation, and Timing, 2014, 3(3): 123-129. doi: 10.11003/JPNT.2014.3.3.123
[6] 安向东, 陈华, 姜卫平, 等. 长基线GLONASS模糊度固定方法及实验分析[J]. 武汉大学学报(信息科学版), 2019, 44(5): 690-698. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201905009.htm An Xiangdong, Chen Hua, Jiang Weiping, et al. GLONASS Ambiguity Resolution Method Based on Long Baselines and Experimental Analysis[J]. Geomatics and Information Science of Wuhan University, 2019, 44(5): 690-698. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201905009.htm
[7] Abidin H Z, Haroen T S, Adiyanto F H, et al. On the Establishment and Implementation of GPS CORS for Cadastral Surveying and Mapping in Indonesia[J]. Survey Review, 2015, 47(340): 61-70. doi: 10.1179/1752270614Y.0000000094
[8] Yanase T, Tanaka H, Ohashi M, et al. Long Baseline Relative Positioning with Estimating Ionosphere and Troposphere Gradients[C]//The 23rd International Technical Meeting of the Satellite Division of the Institute of Navigation, Portland, USA, 2010.
[9] Blewitt G. Carrier Phase Ambiguity Resolution for the Global Positioning System Applied to Geodetic Baselines up to 2 000 km[J]. Journal of Geophysical Research: Solid Earth, 1989, 94(B8): 10187-10203. doi: 10.1029/JB094iB08p10187
[10] 高旺, 高成发, 潘树国, 等. 基于部分固定策略的多系统长距离基准站间模糊度快速解算[J]. 武汉大学学报(信息科学版), 2017, 42(4): 558-562. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201704020.htm Gao Wang, Gao Chengfa, Pan Shuguo, et al. Fast Ambiguity Resolution Between GPS/GLONASS/BDS Combined Long-Range Base Stations Based on Partial-Fixing Strategy[J]. Geomatics and Information Science of Wuhan University, 2017, 42(4): 558-562. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201704020.htm
[11] 高星伟, 陈锐志, 赵春梅. 网络RTK算法研究与实验[J]. 武汉大学学报(信息科学版), 2009, 34(11): 1350-1353. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH200911023.htm Gao Xingwei, Chen Ruizhi, Zhao Chunmei. A Network RTK Algorithm and Its Test[J]. Geomatics and Information Science of Wuhan University, 2009, 34(11): 1350-1353. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH200911023.htm
[12] Li B F, Shen Y Z, Feng Y M, et al. GNSS Ambiguity Resolution with Controllable Failure Rate for Long Baseline Network RTK[J]. Journal of Geodesy, 2014, 88(2): 99-112.
[13] 祝会忠, 李军, 蔚泽然, 等. 长距离GPS/BDS参考站网多频载波相位整周模糊度解算方法[J]. 测绘学报, 2020, 49(3): 300-311. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB202003006.htm Zhu Huizhong, Li Jun, Yu Zeran, et al. The Algorithm of Multi-frequency Carrier Phase Integer Ambiguity Resolution with GPS/BDS Between Long Range Network RTK Reference Stations[J]. Acta Geodaetica et Cartographica Sinica, 2020, 49(3): 300-311. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB202003006.htm
[14] 李博峰, 沈云中, 楼立志. GPS中长基线观测值随机特性分析[J]. 武汉大学学报(信息科学版), 2010, 35(2): 176-180. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201002014.htm Li Bofeng, Shen Yunzhong, Lou Lizhi. Analysis of the Stochastic Characteristics for Medium and Long Baseline GPS Measurements[J]. Geomatics and Information Science of Wuhan University, 2010, 35(2): 176-180. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201002014.htm
[15] Jin S G, Luo O, Ren C. Effects of Physical Correlations on Long-Distance GPS Positioning and Zenith Tropospheric Delay Estimates[J]. Advances in Space Research, 2010, 46(2): 190-195.
[16] Xu Y, Wu C, Li L, et al. GPS/BDS Medium/Long-Range RTK Constrained with Tropospheric Delay Parameters from NWP Model[J]. Remote Sensing, 2018, 10(7): 1113.
[17] Cohenour C, Graas F. GPS Orbit and Clock Error Distributions[J]. Navigation, 2011, 58(1): 17-28.
[18] Montenbruck O, Steigenberger P, Hauschild A. Broadcast Versus Precise Ephemerides: A Multi-GNSS Perspective[J]. GPS Solutions, 2015, 19(2): 321-333.
[19] Kazmierski K, Sośnica K, Hadas T. Quality Assessment of Multi-GNSS Orbits and Clocks for Real-Time Precise Point Positioning[J]. GPS Solutions, 2018, 22(1), DOI: 10.1007/s10291-017-0678-6.
[20] Han S. Carrier Phase-Based Long-Range GPS Kinematic Positioning[D]. Sydney, Australia: University of New South Wales, 1997.
[21] 陈俊平, 王君刚, 王解先, 等. SHAtrop: 基于陆态网GNSS数据的中国大陆区域ZTD模型[J]. 武汉大学学报(信息科学版), 2019, 44(11): 1588-1595. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201911002.htm Chen Junping, Wang Jungang, Wang Jiexian, et al. SHAtrop: Empirical ZTD Model Based on CMONOC GNSS Network[J]. Geomatics and Information Science of Wuhan University, 2019, 44(11): 1588-1595. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201911002.htm
[22] Yin H T, Li J, Ma P F, et al. Effect of Solid-Earth-Tide on GPS Time Series[J]. Geodesy and Geodynamics, 2010, 1(1): 64-69.
[23] 王嘉琛, 刘根友, 郭爱智, 等. 相对定位双差模型中的天线相位缠绕误差分析[J]. 武汉大学学报(信息科学版), 2020, 45(1): 38-45. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH202001006.htm Wang Jiachen, Liu Genyou, Guo Aizhi, et al. Antenna Phase Wind-Up Error Analysis of GNSS Relative Positioning Double-Difference Model[J]. Geomatics and Information Science of Wuhan University, 2020, 45(1): 38-45. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH202001006.htm
[24] 叶世榕, 夏凤雨, 赵乐文, 等. 偏航姿态对北斗精密单点定位的影响分析[J]. 测绘学报, 2017, 46(8): 971-977. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB201708007.htm Ye Shirong, Xia Fengyu, Zhao Lewen, et al. Impact Analysis of Yaw Attitude on BDS Precise Point Positioning[J]. Acta Geodaetica et Cartographica Sinica, 2017, 46(8): 971-977. https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB201708007.htm
[25] 王晨, 郭靖, 赵齐乐. 偏航姿态对GPS和GLONASS精密轨道和钟差的影响[J]. 武汉大学学报(信息科学版), 2017, 42(5): 624-629. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201705009.htm Wang Chen, Guo Jing, Zhao Qile. Impact of Yaw Attitude on GPS/GLONASS Orbit and Clock Solutions[J]. Geomatics and Information Science of Wuhan University, 2017, 42(5): 624-629. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201705009.htm
[26] Dilssner F. GPS IIF-1 Satellite: Antenna Phase Center and Attitude Modeling[J]. Inside GNSS, 2010, 5(6): 59-64.
计量
- 文章访问数: 730
- HTML全文浏览量: 291
- PDF下载量: 150
