-
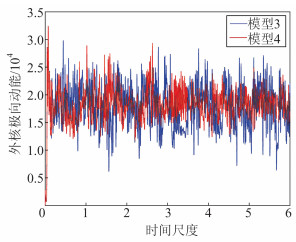
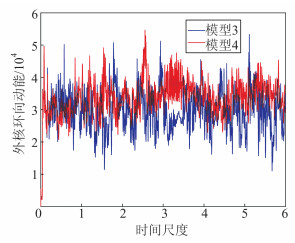
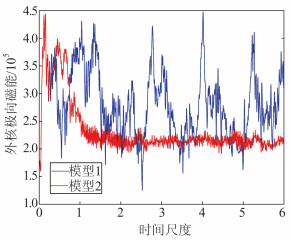
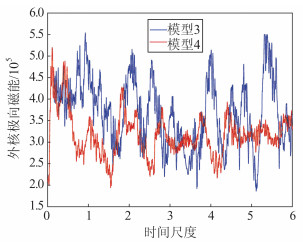
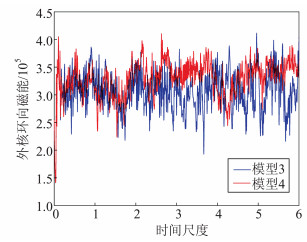
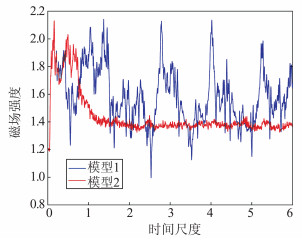
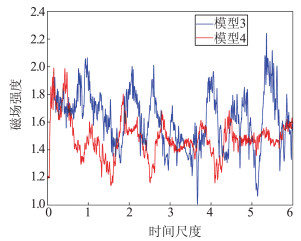
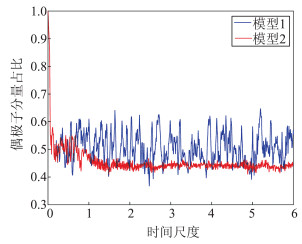
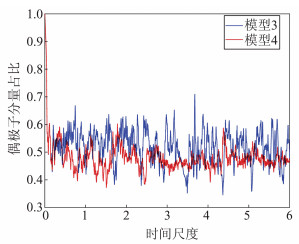
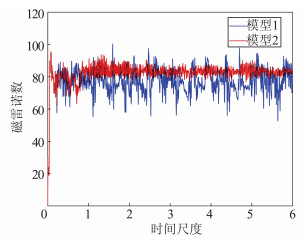
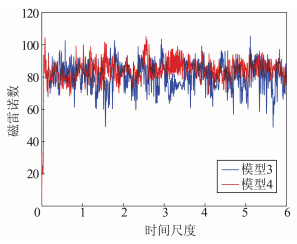
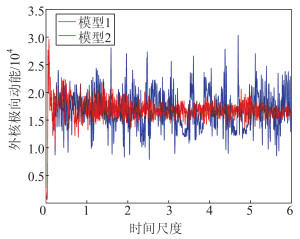
摘要: 在地球外核磁流体发电机数值模拟研究中,与地轴进动相关的庞加莱力通常视其影响极小而在求解发电机方程组中将其忽略,但实际上地轴进动的周期相对地球磁场的倒转周期来说仍是一个值得考虑的项。基于地球发电机数值模拟基准模型,通过引入周期为25 960 a的地轴进动速度,比较分析不同埃克曼数和瑞利数参数组合模型中地轴进动对发电机数值模型的作用。结果表明,地轴进动使得球壳磁流体的动能及磁能波动稳定在一个较原始模型更小的范围内,但增大球壳磁流体的环向动能10%~20%以上,这导致磁场西向漂移速率明显加快;通过比较各模型间的核-幔边界磁场强度和磁雷诺数,发现地轴进动项的引入使得磁流体的能量更倾向于向动能转换;通过比较核-幔边界的偶极性,发现地轴进动项的引入会降低磁流体发电机数值模型的偶极性,但对于所选的原始模型,偶极性的减小程度还不足以使其成为非类地发电机模型。通过数值模拟研究,发现地轴进动项的加入很可能会导致埃克曼数量级较小的类地发电机模型变为多极子主导的发电机模型,因此,在更为准确的磁流体发电机数值模拟研究中,必须考虑地轴进动项。Abstract:Objectives The Poincaré force related to the precession of Earth's axis is usually omitted in the solution of the dynamo equations as its effects are minimal. But in fact, the period of Earth's axial precession is still a term that is worth considering compared to the period of Earth's magnetic field reversal.Methods This paper compares and analyzes different dynamo models with Ekman number and Rayleigh number by introducing Earth's axis precession velocity with a period of 25 960 years.Results It is found that Earth's axial precession stabilizes the kinetic energy and magnetic energy fluctuation of spherical shell magnetic fluid in a smaller range than the benchmark dynamo, and increases the toroidal kinetic energy of spherical shell magnetic fluid by more than 10%-20%, which leads to significantly accelerated westward drift rate of the magnetic field. Based on the magnetic field strength and magnetic Reynolds number at the core-mantle boundary of those dynamos, it is found that the introduction of the precession item of Earth's axis makes magnetic energy more tend to kinetic energy conversion. And by comparing the core-mantle boundary dipolarity, it is found that the introduction of the precession item will reduce the dipolarity. But for the dynamos in this article, the influence is not strong enough to make it become less Earth-like.Conclusions From the comparison and analysis of the dynamo models, the introduction of Earth's axis precession would possibly change the dynamo model with a small Ekman number to a multipole dominant dynamo model. It can be inferred that the term of Earth's axis precession should be considered in more precise research of the numerical dynamo simulation for more.
-
Keywords:
- geomagnetic field /
- geodynamo /
- numerical simulation /
- the Earth's axis precessio
-
致谢: 本文使用的MagIC代码由Thomas Gastine博士提供,黄曦博士和林玉峰副教授在使用MagIC代码中提供了帮助;本文的数值计算得到了武汉大学超级计算中心和武汉大学测绘学院网络与数据处理中心的计算支持和帮助,在此一并表示感谢。
-
表 1 模型1~4在6个粘性时间尺度的西向漂移圈数
Table 1 Circles of West Drifts of 4 Models in 6 Viscous Time Scales
模型 西向漂移圈数 1 32±3 2 49±3 3 35±3 4 44±3 -
[1] Baron G C. A Discourse on the Revolutions of the Surface of the Globe, and the Changes Thereby Produced in the Animal Kingdom[M]. Philadelphia: Lea&Blanchard, 1831
[2] Glatzmaier G A, Roberts P H. A Three-Dimensional Self-Consistent Computer Simulation of a Geomagnetic Field Reversal[J]. Nature, 1995, 377(6546): 203-209 doi: 10.1038/377203a0
[3] Christensen U R, Aubert J, Hulot G. Conditions for Earth-Like Geodynamo Models[J]. Earth and Planetary Science Letters, 2010, 296(3): 486-496
[4] Kageyama A, Sato T. Computer Simulation of a Magnetohydrodynamic DynamoⅡ[J]. Physics of Plasmas, 1995, 2(5): 1421-1431 doi: 10.1063/1.871485
[5] Kuang W, Bloxham J. An Earth-Like Numerical Dynamo Model[J]. Nature, 1997, 389: 371-374 doi: 10.1038/38712
[6] Stefani F, Albrecht T, Gerbeth G, et al. Towards a Precession Driven Dynamo Experiment[J]. Magnetohydrodynamics, 2014, 51(2): 275-283
[7] 邓洪涛. 地球内核与磁场[J]. 武汉大学学报·信息科学版, 2010, 35(7): 854-856 http://ch.whu.edu.cn/article/id/989 Deng Hongtao. The Inner Core and Geomagnetic Field[J]. Geomatics and Information Science of Wuhan University, 2010, 35(7): 854-856 http://ch.whu.edu.cn/article/id/989
[8] Glatzmaier G A. Introduction to Modelling Convection in Planets and Stars[M]. Princeton: Princeton University Press, 2013
[9] Christensen U R, Aubert J, Cardin P, et al. A Numerical Dynamo Benchmark[J]. Physics of the Earth and Planetary Interiors, 2001, 128(1): 24-34
[10] Thomas G, Johannes W, Ankit B, et al. Welcome—Magic 5.6 Documentation[EB/OL]. [2019-3-29]. https://magic-sph.github.io/
[11] Lauren W, Jessica I. Reconciling the Hemispherical Structure of Earth's Inner Core with Its Super-Rotation[J]. Nature Geoscience, 2011, 4(4): 264-267 doi: 10.1038/ngeo1083
[12] Wicht J. Inner-Core Conductivity in Numerical Dynamo Simulations[J]. Physics of the Earth and Planetary Interiors, 2002, 132(4): 281-302 doi: 10.1016/S0031-9201(02)00078-X





 下载:
下载: