An Improved Model for Inter-System Bias Estimation Based on BDS-2/BDS-3 Combined Precise Orbit Determination
-
摘要: 针对BDS-2与BDS-3卫星之间的差异性，为实现观测数据深度融合，对BDS-2/BDS-3联合定轨中系统偏差(inter-satellite bias, ISB)模型进行研究。首先，基于奇异值分解提取相邻历元观测信息以增加数据利用率，提高ISB解算精度与可靠性；其次，分析了BDS-2/BDS-3联合定轨中ISB特性，发现新信号与BDS-2之间存在明显与接收机相关偏差；随后，利用定轨法方程求解ISB与轨道相关性，结果显示，ISB对北斗轨道精度影响较GPS（global positioning system）显著；最后，通过对ISB时间序列建立短期预报模型，并将预报值作为约束条件引入超快速定轨中。实验结果表明，针对BDS-2/BDS-3超快速轨道，利用提出的ISB的估计与预报模型，可分别改善BDS-2与BDS-3轨道18 h重叠弧段精度（一维）-0.4~1.0 cm与0.8~4.1 cm。因此，所提出的改进的ISB处理模型对优化BDS-2/BDS-3联合定轨策略具有一定参考意义。
-
关键词:
- BDS-2/BDS-3联合定轨 /
- 系统偏差 /
- 奇异值分解 /
- 短期预报 /
- 超快速轨道
Abstract:Objectives To integrate processing of BDS-2 and BDS-3 satellites observations and to conduct the deeply merged strategy for enhancing the parameter solutions, the differences between BDS-2 and BDS-3 should be considered and analyzed.Methods Given the differences of BDS-2 and BDS-3 satellites, to fully utilize and fuse the observations, the inter-system bias (ISB) model of BDS-2/BDS-3 combined precise orbit determination is analyzed and improved. Firstly, to improve the accuracy and reliability of ISB, the singular value decomposition is introduced to extract the observation information of adjacent epoch. Secondly, the characteristics of ISB are analyzed, which indicts that the ISBs are significantly presented and related with the types of receivers based on the new signals of BDS-3 and BDS-2. Then, the correlations between ISB and orbit parameters are analyzed; the results suggest that the BeiDou orbit accuracy is affected more significantly than that of GPS. Finally, based on the estimated ISB series, the short-term prediction of ISB model is established by spectral analysis, which is inserted into the ultra-rapid precise orbit determination.Results With ultra-rapid orbit determination experiments, the proposed ISB prediction and estimation strategies can improve -0.4–1.0 cm and 0.8–4.1 cm for BDS-2 and BDS-3 orbit one-day overlapping, respectively.Conclusions Therefore, it is meaningful for improving the BDS-2/BDS-3 ultra-rapid combined precise orbit determination with the improved ISB estimation and prediction model. -
北斗卫星导航系统正按照先试验、后区域、再全球的三步走战略稳步推进[1]，即导航验证系统（BDS-1）、区域服务系统（BDS-2）和全球覆盖系统（BDS-3）3个阶段[2]。自2015年3月，第一颗BDS-3试验卫星（BDS-3e）入轨，标志北斗导航系统由区域向全球发展。截至2018年12月，共4颗BDS-3试验卫星（C18、C19、C28、C31）和19颗工作卫星（C20~C27、C29~C37、C47、C48）在轨工作。于2020年实现30颗BDS-3组网星服务：3颗GEO（geostationary orbit satellite）、3颗IGSO（inclined geosynchronous orbit）、24颗MEO（medium earth orbit），真正实现了中国北斗卫星导航系统由区域向全球服务的飞跃[3]。
考虑到BDS-2与BDS-3为北斗系统建设的不同阶段，其相应的精密定轨研究重点不尽相同。针对BDS-2轨道研究主要集中于3个方面：（1）基于区域跟踪网[4-5]，研究地面跟踪站受限条件下的定轨策略；（2）卫星几何和物理模型[6-8]，如姿态模型等；（3）多卫星导航系统联合定轨[9-10]。评估表明，BDS-2的MEO/IGSO和GEO一天重叠弧段不符值分别由0.5 m和3.0 m提升至优于0.2 m与1.0 m[11-12]。而BDS-3定轨热点主要包括3点：（1）卫星观测数据、卫星钟差以及轨道测定精度等分析[13-14]；（2）评估星间链路对轨道、钟差测定精度影响[3, 15]；（3）融合星间与星地观测数据的BDS-2/BDS-3联合定轨技术研究[16]。结果显示，BDS-3试验卫星轨道重叠弧段径向与切向分别由10.0 cm和25.0 cm提升至3.7 cm与7.9 cm，且星载原子钟日稳定性较BDS-2提高了一个量级[14, 17]。
综上，BDS-3新卫星、新技术与新信号的引入，使BDS-3卫星具备更强性能，也对北斗卫星精密定轨提出了新挑战：卫星几何形状的改变将可能导致现有几何与物理模型不再适用，BDS-3卫星潜在的优势与价值尚未充分挖掘利用。考虑当前北斗系统建设现状，未来一段时间内北斗系统将由BDS-3与BDS-2共同提供服务，因此，研究BDS-3/BDS-2联合精密定轨，充分利用BDS-2卫星资源，挖掘BDS-3新技术优势，是当前北斗导航系统数据处理的有效途径之一。
BDS-2与BDS-3卫星之间的差异将不可避免地导致不同卫星以及观测数据之间产生与接收机相关的偏差[18]。GNSS（global navigation satellite system）数据融合处理中，将不同卫星系统存在于观测数据中的偏差定义为系统偏差（inter-satellite bias，ISB），这是GNSS数据融合的关键问题之一[2, 19-21]。由于BDS-3尚处于建设阶段，BDS-2与BDS-3之间系统偏差缺少深入研究，其将直接影响BDS-2与BDS-3深度融合与兼容互操作，因此，深入研究BDS-2与BDS-3之间系统偏差是实现联合定轨的必要前提。
研究表明，ISB是GNSS观测数据联合处理必须考虑的参数，且BDS-2不同类型卫星（GEO、IGSO、MEO）之间同样存在偏差[22]。为降低ISB对多系统数据融合处理的影响，主要有3种策略：（1）星间单差实现多系统松组合[23]；（2）非差观测模型估计ISB参数估计，实现多系统的紧组合[24]；（3）将ISB参数与接收机钟差、模糊度以及伪距残差合并，简化数据处理模型[25]。BDS-2/BDS-3联合定轨中，Li等[17]通过iGMAS（international GNSS monitoring and assessment system）跟踪站的B1I/B3I数据验证了BDS-3e与BDS-2间无明显偏差；Hu等[26]基于两步法，通过引入七参数消除BDS-2与BDS-3e之间的系统偏差。
针对北斗不同类型卫星联合其他多系统数据处理时，ISB处理策略可归为：忽略ISB参数、同一参数及不同参数。需要注意的是，所有的处理方法都是以ISB天稳定参数为前提。在考虑BDS-2/BDS-3联合定轨ISB未充分研究且尚未实现观测数据的深度融合的前提下，ISB处理策略存在诸多不合理之处，尤其对于轨道精度与计算时效性有严格要求的超快速产品[27]。超快速精密定轨受时效性与跟踪站等限制，无法利用足够的观测数据实现参数高精度解算。研究发现[28]，不施加约束条件下，超快速轨道边界存在发散现象，其将进一步降低ISB参数的解算精度。本文将从BDS-2/BDS-3联合定轨角度出发，研究与分析ISB参数特性及其处理方法。
1 联合定轨ISB处理模型
1.1 联合定轨ISB参数估计原理
由于北斗跟踪站受限，BDS-2/BDS-3联合定轨过程中引入GPS（global positioning system）数据以提高参数解算可靠性。式（1）、式（2）分别表示载波相位和伪距观测方程，设非差消电离层组合GPS/BDS-2/BDS-3联合定轨观测方程为[17]：
$$ \left\{\begin{array}{l}{\varphi }_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}}={\mathit{\boldsymbol{\psi }}}_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}}\times ({\mathit{\pmb{\Phi }}}_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}}\times {\mathit{\boldsymbol{o}}}_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}}-\mathit{\boldsymbol{r}})-\mathrm{\Delta }{t}_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}}+\mathrm{\Delta }t+{\lambda }_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}\times ({b}_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}}-{p}_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}}+{N}_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}})+m\times Z+{\mathit{\pmb{\epsilon }}}_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}}\\ {\varphi }_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2}}={\mathit{\boldsymbol{\psi }}}_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2}}\times ({\mathit{\pmb{\Phi }}}_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2}}\times {\mathit{\boldsymbol{o}}}_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2}}-\mathit{\boldsymbol{r}})-\mathrm{\Delta }{t}_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2}}+\mathrm{\Delta }t+{\lambda }_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2}}\times ({b}_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2}}-{p}_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2}}+{N}_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2}})+m\times Z+{\mathit{\pmb{\epsilon }}}_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2}}\\ {\varphi }_{\mathrm{G}\mathrm{P}\mathrm{S}}={\mathit{\boldsymbol{\psi }}}_{\mathrm{G}\mathrm{P}\mathrm{S}}\times ({\mathit{\pmb{\Phi }}}_{\mathrm{G}\mathrm{P}\mathrm{S}}\times {\mathit{\boldsymbol{o}}}_{\mathrm{G}\mathrm{P}\mathrm{S}}-\mathit{\boldsymbol{r}})-\mathrm{\Delta }{t}_{\mathrm{G}\mathrm{P}\mathrm{S}}+\mathrm{\Delta }t+{\lambda }_{\mathrm{G}\mathrm{P}\mathrm{S}}\times ({b}_{\mathrm{G}\mathrm{P}\mathrm{S}}-{p}_{\mathrm{G}\mathrm{P}\mathrm{S}}+{N}_{\mathrm{G}\mathrm{P}\mathrm{S}})+m\times Z+{\mathit{\pmb{\epsilon }}}_{\mathrm{G}\mathrm{P}\mathrm{S}}\end{array}\right. $$ (1) $$ \left\{\begin{array}{l}{\rho }_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}}={\mathit{\boldsymbol{\psi }}}_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}}\times ({\mathit{\pmb{\Phi }}}_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}}\times {\mathit{\boldsymbol{o}}}_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}}-\mathit{\boldsymbol{r}})-\mathrm{\Delta }{t}_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}}+\mathrm{\Delta }t+c\times ({d}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}-{u}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3})+m\times Z+{\mathit{\pmb{e}}}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}\\ {\rho }_{{}_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2}}}={\mathit{\boldsymbol{\psi }}}_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2}}\times ({\mathit{\pmb{\Phi }}}_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2}}\times {\mathit{\boldsymbol{o}}}_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2}}-\mathit{\boldsymbol{r}})-\mathrm{\Delta }{t}_{{}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2}}+\mathrm{\Delta }t+c\times ({d}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2}-{u}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2})+m\times Z+{\mathit{\pmb{e}}}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2}\\ {\rho }_{\mathrm{G}\mathrm{P}\mathrm{S}}={\mathit{\boldsymbol{\psi }}}_{\mathrm{G}\mathrm{P}\mathrm{S}}\times ({\mathit{\pmb{\Phi }}}_{\mathrm{G}\mathrm{P}\mathrm{S}}\times {\mathit{\boldsymbol{o}}}_{\mathrm{G}\mathrm{P}\mathrm{S}}-\mathit{\boldsymbol{r}})-\mathrm{\Delta }{t}_{\mathrm{G}\mathrm{P}\mathrm{S}}+\mathrm{\Delta }t+c\times ({d}_{\mathrm{G}\mathrm{P}\mathrm{S}}-{u}_{\mathrm{G}\mathrm{P}\mathrm{S}})+m\times Z+{\mathit{\pmb{e}}}_{\mathrm{G}\mathrm{P}\mathrm{S}}\end{array}\right. $$ (2) 式中，Ψ为星地方向向量；λ、Φ、o、r分别表示波长、旋转矩阵向量、卫星初始状态向量和测站位置向量；$ \mathrm{\Delta }{t}_{\mathrm{G}\mathrm{P}\mathrm{S}} $、$ \mathrm{\Delta }t $分别表示接收机钟差与卫星钟差参数；c、m、Z、N分别为光速、对流层映射函数、对流层天顶延迟参数和模糊度参数；$ {d}_{\mathrm{G}\mathrm{P}\mathrm{S}}\mathrm{、}{u}_{\mathrm{G}\mathrm{P}\mathrm{S}} $与$ {b}_{\mathrm{G}\mathrm{P}\mathrm{S}}\mathrm{、}{p}_{\mathrm{G}\mathrm{P}\mathrm{S}} $分别表示接收机与卫星对应的伪距和相位硬件延迟；e、ε分别表示伪距、载波相位观测方程残差向量。令：
$$ \left\{\begin{array}{l}\mathrm{\Delta }{\overline{t}}_{\mathrm{G}\mathrm{P}\mathrm{S}}=\mathrm{\Delta }t+c\times {d}_{\mathrm{G}\mathrm{P}\mathrm{S}}\\ \mathrm{\Delta }{\overline{t}}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2}=\mathrm{\Delta }t+c\times {d}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2}=\mathrm{\Delta }{\overline{t}}_{\mathrm{G}\mathrm{P}\mathrm{S}}+{I}_{\mathrm{G}\mathrm{P}\mathrm{S}\_\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2}\\ \mathrm{\Delta }{\overline{t}}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}=\mathrm{\Delta }t+c\times {d}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}=\mathrm{\Delta }{\overline{t}}_{\mathrm{G}\mathrm{P}\mathrm{S}}+{I}_{\mathrm{G}\mathrm{P}\mathrm{S}\_\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}\end{array}\right. $$ (3) 式中，$ {I}_{\mathrm{G}\mathrm{P}\mathrm{S}\_\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2} $与$ {I}_{\mathrm{G}\mathrm{P}\mathrm{S}\_\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}\mathrm{分}\mathrm{别} $表示GPS与BDS-2和BDS-3之间的偏差。设模糊度参数为：
$$ \left\{\begin{array}{l}{\overline{N}}_{\mathrm{G}\mathrm{P}\mathrm{S}}={b}_{\mathrm{G}\mathrm{P}\mathrm{S}}-{p}_{\mathrm{G}\mathrm{P}\mathrm{S}}+{N}_{\mathrm{G}\mathrm{P}\mathrm{S}}\\ {\overline{N}}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2}={b}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2}-{p}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2}+{N}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2}\\ {\overline{N}}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}={b}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}-{p}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}+{N}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}\end{array}\right. $$ (4) 参数处理过程中，为避免法方程秩亏，需对ISB参数构建零均值约束条件，即：
$$ \left\{\begin{array}{l}({I}_{\mathrm{G}\mathrm{P}\mathrm{S}\_\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2}{)}_{1}+({I}_{\mathrm{G}\mathrm{P}\mathrm{S}\_\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2}{)}_{2}+\cdots +({I}_{\mathrm{G}\mathrm{P}\mathrm{S}\_\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2}{)}_{n}=0\\ ({I}_{\mathrm{G}\mathrm{P}\mathrm{S}\_\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}{)}_{1}+({I}_{\mathrm{G}\mathrm{P}\mathrm{S}\_\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}{)}_{2}+\cdots +({I}_{\mathrm{G}\mathrm{P}\mathrm{S}\_\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}{)}_{n}=0\end{array}\right. $$ (5) 式中，n表示测站数。设某测站，BDS-2与BDS-3之间系统偏差表示为：
$$ {I}_{\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3\_\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2}={I}_{\mathrm{G}\mathrm{P}\mathrm{S}\_\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}3}-{I}_{\mathrm{G}\mathrm{P}\mathrm{S}\_\mathrm{B}\mathrm{D}\mathrm{S}\mathrm{-}2} $$ (6) 上述参数处理中，引入GPS观测数据以提高参数解算精度。但是，BDS-2/BDS-3联合定轨中ISB处理模型仍存在明显不足：（1）基于ISB天稳定的前提，其稳定性主要表现为观测弧段中间部分，两端易出现波动现象；（2）未充分挖掘前后历元观测信息，导致观测数据受限条件下，参数估计可靠性降低。
1.2 改进的联合定轨ISB处理模型
针对联合定轨中ISB存在的问题，可通过优化定轨策略，如对测站坐标、对流层、钟差等参数施加强约束条件；充分利用ISB参数稳定特性，实现参数估计随机模型精化。而对于观测信息利用不充分，可通过观测值域内奇异值分解，将历元间观测信息进行有效传递。
为提高BDS-2/BDS-3联合定轨中ISB参数解算精度，准确分析偏差参数特性，本文提出一种改进的联合定轨ISB估计方法，充分提取历元间观测信息，并对数据中断与观测弧段两端ISB参数进行短期预报。具体可参见文献[29]，为便于论述，将式（1）与式（2）简化，即第k个历元为：
$$ {\mathit{\boldsymbol{l}}}_{k}=\left[\begin{array}{c}{\mathit{\boldsymbol{\phi }}}_{k}\\ {\mathit{\boldsymbol{\rho }}}_{k}\end{array}\right]={\mathit{\boldsymbol{G}}}_{k}{\mathit{\boldsymbol{\beta }}}_{k}+{\mathit{\boldsymbol{\eta }}}_{k} $$ (7) 式中，β中包含卫星轨道、ISB等定轨待估参数；η为残差矩阵。首先，对系数矩阵Gk进行分解：
$$ {\mathit{\boldsymbol{G}}}_{\mathrm{k}}={\mathit{\boldsymbol{U}}}_{k}^{\mathrm{T}}\left[\begin{array}{cc}{\mathit{\boldsymbol{D}}}_{k}& 0\\ 0& 0\end{array}\right]{\mathit{\boldsymbol{V}}}_{k}={\left[\begin{array}{c}{\mathit{\boldsymbol{U}}}_{k, 1}\\ {\mathit{\boldsymbol{U}}}_{k, 2}\end{array}\right]}^{\mathrm{T}}\left[\begin{array}{cc}{\mathit{\boldsymbol{D}}}_{k}& 0\\ 0& 0\end{array}\right]\left[\begin{array}{c}{\mathit{\boldsymbol{V}}}_{k, 1}\\ {\mathit{\boldsymbol{V}}}_{k, 2}\end{array}\right]=\\ \;\;\;\;{\mathit{\boldsymbol{U}}}_{k, 1}^{\mathrm{T}}{\mathit{\boldsymbol{D}}}_{k}{\mathit{\boldsymbol{V}}}_{k, 1} $$ (8) 式中，Dk为对角矩阵。令$ {\mathit{\boldsymbol{\theta }}}_{k}=\left[\begin{array}{c}{\mathit{\boldsymbol{\theta }}}_{k, 1}\\ {\mathit{\boldsymbol{\theta }}}_{k, 2}\end{array}\right]=\left[\begin{array}{c}{\mathit{\boldsymbol{V}}}_{k, 1}{\mathit{\boldsymbol{\beta }}}_{k}\\ {\mathit{\boldsymbol{V}}}_{k, 2}{\mathit{\boldsymbol{\beta }}}_{k}\end{array}\right] $，且$ {\mathit{\boldsymbol{\beta }}}_{k}={\mathit{\boldsymbol{V}}}_{k}^{\mathrm{T}}{\mathit{\boldsymbol{\theta }}}_{k}={\mathit{\boldsymbol{V}}}_{k, 1}^{\mathrm{T}}{\widehat{\mathit{\boldsymbol{\theta }}}}_{k, 1}+{\mathit{\boldsymbol{V}}}_{k, 2}^{\mathrm{T}}{\widehat{\mathit{\boldsymbol{\theta }}}}_{k, 2} $，则式（7）表示为：
$$ \left[\begin{array}{c}{\mathit{\boldsymbol{U}}}_{k, 1}{\mathit{\boldsymbol{l}}}_{k}\\ {\mathit{\boldsymbol{U}}}_{k, 2}{\mathit{\boldsymbol{l}}}_{k}\end{array}\right]=\left[\begin{array}{c}{\mathit{\boldsymbol{D}}}_{k}{\mathit{\boldsymbol{\theta }}}_{k, 1}\\ 0\times {\mathit{\boldsymbol{\theta }}}_{k, 2}\end{array}\right]+\left[\begin{array}{c}{\mathit{\boldsymbol{U}}}_{k, 1}{\mathit{\boldsymbol{\eta }}}_{k}\\ {\mathit{\boldsymbol{U}}}_{k, 2}{\mathit{\boldsymbol{\eta }}}_{k}\end{array}\right] $$ (9) 将Uk，1分解为Uk，1，1与Uk，1，2，则参数θk，1解可表示为：
$$ {\widehat{\mathit{\boldsymbol{\theta }}}}_{k, 1}={\mathit{\boldsymbol{D}}}_{k}^{-1}{\mathit{\boldsymbol{U}}}_{k, 1}{\mathit{\boldsymbol{l}}}_{k}={\mathit{\boldsymbol{D}}}_{k}^{-1}\left[\begin{array}{cc}{\mathit{\boldsymbol{U}}}_{k, \mathrm{1, 1}}& {\mathit{\boldsymbol{U}}}_{k, \mathrm{1, 2}}\end{array}\right]\left[\begin{array}{c}{\mathit{\boldsymbol{\phi }}}_{k}\\ {\mathit{\boldsymbol{\rho }}}_{k}\end{array}\right] $$ (10) 其对应协因数矩阵为：
$$ \begin{array}{l}{\mathit{\boldsymbol{Q}}}_{{\mathit{\boldsymbol{\theta }}}_{1}{\mathit{\boldsymbol{\theta }}}_{1}, k}=\mathrm{c}\mathrm{o}\mathrm{v}\left[\mathrm{d}{\widehat{\mathit{\boldsymbol{\theta }}}}_{k, 1}\right]=({\mathit{\boldsymbol{U}}}_{k, \mathrm{1, 1}}^{\mathrm{T}}{\mathit{\boldsymbol{D}}}_{k}^{-1}{\mathit{\boldsymbol{Q}}}_{\mathit{\boldsymbol{\epsilon }}\mathit{\boldsymbol{\epsilon }}, k}^{-1}{\mathit{\boldsymbol{D}}}_{k}^{-1}{\mathit{\boldsymbol{U}}}_{k, \mathrm{1, 1}}+\\\;\;\;\;\;\;\;\; \mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }{\mathit{\boldsymbol{U}}}_{k, \mathrm{1, 2}}^{\mathrm{T}}{\mathit{\boldsymbol{D}}}_{k}^{-1}{\mathit{\boldsymbol{Q}}}_{\mathit{\boldsymbol{e}}\mathit{\boldsymbol{e}}, k}^{-1}{\mathit{\boldsymbol{D}}}_{k}^{-1}{\mathit{\boldsymbol{U}}}_{k, \mathrm{1, 2}}{)}^{-1}\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\end{array} $$ (11) 式中，Qεε与Qee分别对应式（1）与式（2）观测噪声协因数阵。为讨论历元间相关性，设第k历元与第j卫星对应的相位观测方程为：
$$ {\mathit{\boldsymbol{\phi }}}_{k, j}={\mathit{\boldsymbol{\alpha }}}_{k, j}^{\mathrm{T}}{\mathit{\boldsymbol{\beta }}}_{k}+\lambda \times {\mathit{\boldsymbol{N}}}_{j}+{\mathit{\boldsymbol{\epsilon }}}_{k, j} $$ (12) 式中，α为参数对应的系数矩阵；N为模糊度；ε为模型噪声。对式（12）求历元间差分，消除模糊度参数及相关常量可得：
$$ \mathrm{\delta }{\mathit{\boldsymbol{\phi }}}_{k, j}={\mathit{\boldsymbol{\alpha }}}_{k, j}^{\mathrm{T}}{\mathit{\boldsymbol{\beta }}}_{k}-{\mathit{\boldsymbol{\alpha }}}_{k-1, j}^{\mathrm{T}}{\mathit{\boldsymbol{\beta }}}_{k-1}+{\mathit{\boldsymbol{\epsilon }}}_{k, j}-{\mathit{\boldsymbol{\epsilon }}}_{k-1, j} $$ (13) 则当前相位观测方程可表示为：
$$ \begin{array}{l}{\tilde{\mathit{\boldsymbol{\phi }}}}_{k, j}=\mathrm{\delta }{\mathit{\boldsymbol{\phi }}}_{k, j}+{\mathit{\boldsymbol{\alpha }}}_{k-1, j}^{\mathrm{T}}{\widehat{\mathit{\boldsymbol{\beta }}}}_{k-1}={\mathit{\boldsymbol{\alpha }}}_{k, j}^{\mathrm{T}}{\widehat{\mathit{\boldsymbol{\beta }}}}_{k}+\\ {\mathit{\boldsymbol{\alpha }}}_{k-1, j}^{\mathrm{T}}\mathrm{d}{\widehat{\mathit{\boldsymbol{\beta }}}}_{k-1}+{\mathit{\boldsymbol{\epsilon }}}_{k, j}-{\mathit{\boldsymbol{\epsilon }}}_{k-1, j}\end{array} $$ (14) 类似式（12）~式（14），可得伪距历元间差分观测方程。则当前历元观测方程可表示为：
$$ {\mathit{\boldsymbol{y}}}_{k}={\mathit{\boldsymbol{A}}}_{k}{\mathit{\boldsymbol{\beta }}}_{k}+{\mathit{\boldsymbol{A}}}_{k-1}\mathrm{d}{\widehat{\mathit{\boldsymbol{\beta }}}}_{k-1}+{\mathit{\boldsymbol{\omega }}}_{k}-{\mathit{\boldsymbol{\omega }}}_{k-1} $$ (15) 将式（10）代入式（15），可得：
$$ \begin{array}{l}{\mathit{\boldsymbol{y}}}_{k}={\mathit{\boldsymbol{A}}}_{k}{\mathit{\boldsymbol{\beta }}}_{k}+{\mathit{\boldsymbol{A}}}_{k-1}{\mathit{\boldsymbol{V}}}_{k-\mathrm{1, 1}}^{\mathrm{T}}\mathrm{d}{\widehat{\mathit{\boldsymbol{\theta }}}}_{k-\mathrm{1, 1}}+\\ {\mathit{\boldsymbol{A}}}_{k-1}{\mathit{\boldsymbol{V}}}_{k-\mathrm{1, 2}}^{\mathrm{T}}\mathrm{d}{\widehat{\mathit{\boldsymbol{\theta }}}}_{k-\mathrm{1, 2}}+{\mathit{\boldsymbol{\omega }}}_{k}-{\mathit{\boldsymbol{\omega }}}_{k-1}={\mathit{\boldsymbol{A}}}_{k}{\mathit{\boldsymbol{\beta }}}_{k}+{\mathit{\boldsymbol{\mu }}}_{k}\end{array} $$ (16) 式中，模型误差协因素为：
$$ \begin{array}{l}\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }{\mathit{\boldsymbol{Q}}}_{\mathit{\boldsymbol{\mu }}\mathit{\boldsymbol{\mu }}, k}=\mathrm{c}\mathrm{o}\mathrm{v}\left[{\mathit{\boldsymbol{\mu }}}_{k}\right]={\mathit{\boldsymbol{A}}}_{k-1}{\mathit{\boldsymbol{V}}}_{k-\mathrm{1, 1}}^{\mathrm{T}}{\mathit{\boldsymbol{Q}}}_{{\mathit{\boldsymbol{\theta }}}_{1}{\mathit{\boldsymbol{\theta }}}_{1}, k-1}{\mathit{\boldsymbol{V}}}_{k-\mathrm{1, 1}}{\mathit{\boldsymbol{A}}}_{k-1}^{\mathrm{T}}+{\mathit{\boldsymbol{A}}}_{k-1}{\mathit{\boldsymbol{V}}}_{k-\mathrm{1, 2}}^{\mathrm{T}}{\mathit{\boldsymbol{Q}}}_{{\mathit{\boldsymbol{\theta }}}_{2}{\mathit{\boldsymbol{\theta }}}_{2}, k-1}{\mathit{\boldsymbol{V}}}_{k-\mathrm{1, 2}}{\mathit{\boldsymbol{A}}}_{k-1}^{\mathrm{T}}+\\ \mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }{\mathit{\boldsymbol{Q}}}_{\mathit{\boldsymbol{\omega }}\mathit{\boldsymbol{\omega }}, k}+{\mathit{\boldsymbol{Q}}}_{\mathit{\boldsymbol{\omega }}\mathit{\boldsymbol{\omega }}, k-1}-{\mathit{\boldsymbol{A}}}_{k-1}{\mathit{\boldsymbol{V}}}_{k-\mathrm{1, 1}}^{\mathrm{T}}{\mathit{\boldsymbol{C}}}_{k-1}-{\mathit{\boldsymbol{C}}}_{k-1}^{\mathrm{T}}{\mathit{\boldsymbol{V}}}_{k-\mathrm{1, 1}}{\mathit{\boldsymbol{A}}}_{k-1}^{\mathrm{T}}\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\end{array} $$ (17) 式中，$ {\mathit{\boldsymbol{C}}}_{k-1} $为：
$$ {\mathit{\boldsymbol{C}}}_{k-1}=\mathrm{c}\mathrm{o}\mathrm{v}[\mathrm{d}{\widehat{\mathit{\boldsymbol{\theta }}}}_{k-\mathrm{1, 1}}, {\mathit{\boldsymbol{\omega }}}_{k-1}] $$ (18) 为便于讨论，令式（9）中：
$${\widehat{\mathit{\boldsymbol{\theta }}}}_{k, 2}=0 且 {\mathit{\boldsymbol{Q}}}_{{\mathit{\boldsymbol{\theta }}}_{2}{\mathit{\boldsymbol{\theta }}}_{2}, k}=0 $$ (19) 则式（17）化简为：
$$ \begin{array}{l}{\mathit{\boldsymbol{Q}}}_{\mu \mu , k}={\mathit{\boldsymbol{A}}}_{k-1}{\mathit{\boldsymbol{V}}}_{k-\mathrm{1, 1}}^{\mathrm{T}}{\mathit{\boldsymbol{Q}}}_{{\mathit{\boldsymbol{\theta }}}_{1}{\mathit{\boldsymbol{\theta }}}_{1}, k-1}{\mathit{\boldsymbol{V}}}_{k-\mathrm{1, 1}}{\mathit{\boldsymbol{A}}}_{k-1}^{\mathrm{T}}+{\mathit{\boldsymbol{Q}}}_{\mathit{\boldsymbol{\omega }}\mathit{\boldsymbol{\omega }}, k}+\\ {\mathit{\boldsymbol{Q}}}_{\mathit{\boldsymbol{\omega }}\mathit{\boldsymbol{\omega }}, k-1}-{\mathit{\boldsymbol{A}}}_{k-1}{\mathit{\boldsymbol{V}}}_{k-\mathrm{1, 1}}^{\mathrm{T}}{\mathit{\boldsymbol{C}}}_{k-1}-{\mathit{\boldsymbol{C}}}_{k-1}^{\mathrm{T}}{\mathit{\boldsymbol{V}}}_{\mathit{\boldsymbol{k}}-\mathrm{1, 1}}{\mathit{\boldsymbol{A}}}_{k-1}^{\mathrm{T}}\end{array} $$ (20) 同时：
$$ {\mathit{\boldsymbol{Q}}}_{\mathit{\boldsymbol{\beta }}\mathit{\boldsymbol{\beta }}, k}=\mathrm{c}\mathrm{o}\mathrm{v}\left[\mathrm{d}{\widehat{\mathit{\boldsymbol{\beta }}}}_{k}\right]=({\mathit{\boldsymbol{A}}}_{k}^{\mathrm{T}}{\mathit{\boldsymbol{Q}}}_{\mathit{\boldsymbol{\mu }}\mathit{\boldsymbol{\mu }}, k}^{-1}{\mathit{\boldsymbol{A}}}_{k}{)}^{-1} $$ (21) 因此，参数解为：
$$ {\widehat{\mathit{\boldsymbol{\beta }}}}_{k}={\mathit{\boldsymbol{Q}}}_{\mathit{\boldsymbol{\beta }}\mathit{\boldsymbol{\beta }}, k}{\mathit{\boldsymbol{A}}}_{k}^{\mathrm{T}}{\mathit{\boldsymbol{Q}}}_{\mathit{\boldsymbol{\mu }}\mathit{\boldsymbol{\mu }}, k}^{-1}{\mathit{\boldsymbol{y}}}_{k} $$ (22) 采用上述参数估计策略，顾及历元间相关性，可实现ISB参数解算过程中观测信息的充分利用。同时，针对定轨过程中边界弧段发散现象[28]（由于轨道、钟差及ISB参数之间具有相关性，同样会导致ISB参数发散），有必要对两端ISB参数重新进行处理。本文采用多项式模型，对两端ISB进行约束处理。研究表明，一天内ISB趋于稳定。本文基于多项式模型对ISB进行拟合与预报，设当前历元ISB为γ，则由多项式模型可得：
$$ \widehat{\mathit{\boldsymbol{\gamma }}}\left(t\right)={\mathit{\boldsymbol{\nu }}}_{0}+{\mathit{\boldsymbol{\nu }}}_{1}t+\cdots +{\mathit{\boldsymbol{\nu }}}_{s}{t}^{s}+{\mathit{\boldsymbol{\varsigma }}}_{m}^{\text{'}} $$ (23) 式中，ν为多项式系数；t为历元标记；$ {\mathit{\boldsymbol{\varsigma }}}_{\mathrm{m}}^{\mathrm{\text{'}}} $为模型拟合残差；s为模型阶数，可由赤池准则[30]确定。式（23）可简化为：
$$ \mathit{\boldsymbol{\gamma }}=\mathit{\boldsymbol{R}}\mathit{\boldsymbol{\nu }}+\mathrm{d}\mathit{\boldsymbol{\nu }} $$ (24) 式中，R为模型系数矩阵。ISB函数模型系数为：
$$ \widehat{\mathit{\boldsymbol{\nu }}}=({\mathit{\boldsymbol{R}}}^{\mathrm{T}}{\mathit{\boldsymbol{R}})}^{-1}{\mathit{\boldsymbol{R}}}^{\mathrm{T}}\mathit{\boldsymbol{\gamma }} $$ (25) 设当前历元t =0，则当前历元ISB延迟量为：
$$ \overline{\mathit{\boldsymbol{\gamma }}}\left(t\right)={\widehat{\mathit{\boldsymbol{\nu }}}}_{0} $$ (26) 通过式（26）逐历元求解观测弧段边界ISB参数，实现整个弧段内ISB参数的准确求解。下面将利用对比实验进行BDS-2/BDS-3联合定轨中ISB参数分析。
2 联合定轨ISB参数实验与分析
2.1 联合定轨ISB参数估计结果
为探讨BDS-2/BDS-3联合定轨中ISB参数特性，要先通过联合定轨实验进行相应分析。
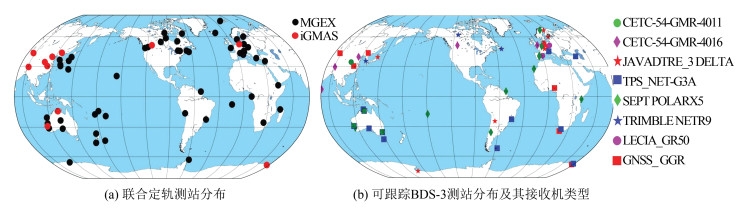
实验利用2018年的年积日第110天至第130天的MGEX（multi-GNSS experiment）与iGMAS观测数据（测站分布见图 1（a））进行BDS-2、BDS-3e、BDS-3和GPS卫星联合定轨；定轨采用单天弧段，其他策略参考文献[26]。
![]()
图 1 联合定轨MGEX与iGMAS测站分布Figure 1. Distribution of MGEX and iGMAS Stations of Combined Orbit Determination实验中，分别基于B1I/B3I与B1C/B2a进行相应的验证，并且ISB设置为一天对应一个常数解。
联合定轨实验步骤如下：
1）利用北斗B1I/B3I观测数据分别进行GPS+BDS-2、GPS+BDS-3e与GPS+BDS-3联合定轨实验，对各定轨组合中ISB参数接收机进行分类统计分析。
2）增加基于B1C/B2a数据进行GPS+BDS-3联合定轨实验，针对不同类型接收机提取ISB参数。实验中，由于可跟踪B1C/B2a信号较少，为提高参数解算的可靠性，需要先固定站坐标、地球自转参数、对流层等。
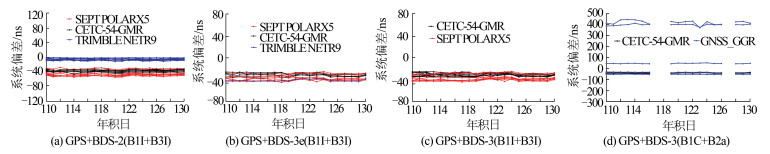
基于连续21 d的不同组合条件下GPS与BDS之间的ISB参数解算结果如图 2所示。为具体分析北斗不同卫星以及不同观测数据条件下BDS-2/BDS-3/BDS-3e之间的系统偏差特性，表 1统计了不同类型接收机BDS-2与BDS-3/BDS-3e系统偏差（通过对GPS+BDS-2、GPS+BDS-3e与GPS+BDS-3联合定轨中估计的系统偏差参数进行作差处理）。
![]()
图 2 北斗不同频率BDS/GPS联合定轨ISB时间序列Figure 2. ISB Time Series Between GPS and BeiDou Combined Orbit Determination表 1 联合定轨中BDS之间的系统偏差统计/nsTable 1. Results of ISB Parameters Between BDS-2, BDS-3 and BDS-3e of Combined Orbit Determination/ns测站 接收机类型 BDS-2/BDS-3e偏差
(B1I/B3I)BDS-2/BDS-3偏差
(B1I/B3I)BDS-2/BDS-3偏差
(B1C/B2a)BJF1 CETC-54-GMR-4016 0.71 0.58 27.28 BRCH 0.73 0.70 30.00 LHA1 1.03 0.63 28.37 WHU1 0.75 0.80 35.15 CLGY 0.74 0.98 37.03 ICUK 0.85 0.87 31.35 CANB CETC-54-GMR-4011 0.82 0.85 30.01 DWIN 0.75 0.84 39.00 PETH 0.85 0.83 24.16 ZHON 0.69 0.77 35.93 ABJA GNSS_GGR 0.81 0.81 23.66 HMNS -2.04 -1.85 22.99 XIA1 -0.32 -0.32 22.70 GUA1 SEPT POLARX5 0.71 0.63 43.05 CHU1 0.75 0.79 23.98 ARUC -0.96 0.43 ‒ STR1 -1.05 -0.10 ‒ DARW -0.99 0.76 ‒ HOB2 -1.01 0.29 ‒ CHPI -0.95 0.10 ‒ DAV1 -0.92 -0.67 ‒ TID1 -0.84 0.41 ‒ 1）GPS与BDS-2/BDS-3e/BDS-3之间的系统偏差可通过联合定轨估计得到；
2）不同类型接收机系统偏差稳定性存在较大差异，且系统偏差参数以接收机类型分群；
3）基于B1I/B3I观测数据，BDS-2与BDS-3e、BDS-2与BDS-3之间偏差参数较小；
4）BDS-2基于B1I/B3I，而BDS-3利用B1C/B2a观测数据，结果显示，BDS-2与BDS-3间存在明显的系统偏差。
实验时间段内，可跟踪BDS-3新信号（B1C/B2a）的MGEX测站多为单频数据（见图 1（b））。需要注意的是，实验过程中主要存在两个因素影响ISB参数估计：
1）实验是基于一天弧段内ISB具有较好的稳定性的前提，通过设置一个ISB参数全弧段估计；
2）北斗新信号可跟踪的测站数据有限，且数据预处理过程中对质量较差的数据进行剔除，势必影响ISB参数估计。
2.2 联合定轨中ISB参数相关性分析
为实现BDS-2/BDS-3联合定轨，需对ISB进行合理的处理：基于参数之间的相关性原理对ISB与轨道参数之间的相关性进行分析；基于联合定轨法方程，分析不同测站对应的ISB参数与卫星轨道参数之间的相关性。计算公式为：
$$ r={\sigma }_{12} / \sqrt{{\sigma }_{1}{\sigma }_{2}}$$ (27) 式中，σ1、σ2、σ12分别对应参数方差及其协方差。
为便于讨论，实验中卫星选取G11与C11卫星，测站选取iGMAS测站（WHU1）与MGEX测站（DARW），统计年积日第110天相应测站ISB参数与卫星轨道的相关系数，结果如表 2所示。
表 2 联合定轨中ISB与卫星轨道参数之间相关系数统计Table 2. Correlation Factors Between ISB and GPS/BDS Satellite Orbit Parameters Based on Combined Orbit Determinations测站 卫星 X Y Z VX VY VZ WHU1 G11 2.73×10-5 -1.55×10-9 -1.50×10-9 2.10×10-5 9.34×10-2 -1.42×10-2 WHU1 C11 1.98×10-3 4.70×10-7 1.03×10-7 -1.74×10-5 7.34×10-2 9.60×10-2 DARW C11 2.76×10-5 -1.44×10-9 -1.76×10-9 2.14×10-5 7.21×10-2 -1.35×10-2 DARW C11 1.99×10-3 4.71×10-7 1.03×10-7 -1.75×10-3 7.15×10-2 9.62×10-2 由表 2中的实验结果可知，GPS与BDS-2/BDS-3联合定轨中，ISB与北斗轨道各参数之间较GPS相关系数高两个量级，这是由于北斗跟踪站数据有限，依赖单站的观测数据较GPS更强。单站ISB与轨道之间相关系数量级较小，这是由于定轨过程受参与解算的所有测站以及力学模型共同作用所致。
为进一步分析ISB参数对联合定轨精度的影响，设计3组对比实验进行分析说明。（1）方案一：联合GPS+BDS-2+BDS-3+BDS-3e定轨中不考虑ISB参数，即定轨过程中不设置任何ISB参数，实验中北斗基于B1I/B3I观测数据进行。（2）方案二：基于方案一，联合定轨中整体考虑一类ISB参数，即不考虑北斗卫星类型差异，对于同时跟踪GPS和北斗卫星的测站只考虑一个系统偏差参数。（3）方案三：基于方案二，分别考虑不同类型卫星的ISB参数。对于同时跟踪GPS和北斗卫星的测站，依据卫星类型（BDS-2/BDS-3/BDS-3e）设置ISB参数。
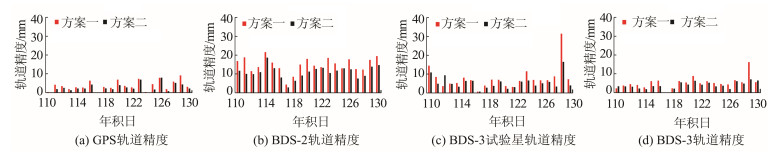
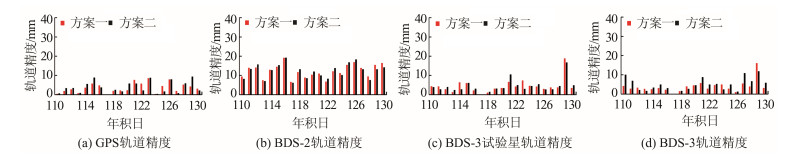
基于§2.1定轨观测数据，统计3个定轨方案轨道精度。由于无法获得BDS-3参考轨道，本文仅统计轨道相对变化量，即以方案三为参考，分别统计其他两种方案的轨道相对变化量，其结果如图 3所示。同时，为说明北斗不同观测数据引起的定轨差异，同样基于上述实验方案，BDS-2/BDS-3e与BDS-3分别基于B1I/B3I和B1C/B2a进行联合定轨实验。精度统计以方案三作为参考，其相应统计结果如图 4所示。
![]()
图 3 基于B1I/B3I频率(BDS-3)不同ISB参数模式下精密定轨精度相对变化Figure 3. Accuracy Variation of Precise Orbit Determination Based on Different ISB Models Under B1I/B3I![]()
图 4 基于B1C/B2a频率(BDS-3)不同ISB参数模式下精密定轨精度相对变化Figure 4. Accuracy Variation of Precise Orbit Determination Based on Different ISB Models Under B1C/B2a通过图 3与图 4对比实验结果可知：（1）基于B1I/B3I定轨过程中采用不同的ISB估计模式，GPS轨道精度受影响较小，而BDS-2受影响较大，同时，定轨过程中不考虑ISB参数对轨道的影响较设置为一类参数大。BDS-3轨道受影响较小，这主要是可跟踪BDS-3的测站有限，观测数据对轨道精度影响较ISB参数明显。（2）定轨方案中BDS-3采用B1C/B2a进行GPS/BDS-2/BDS-3/BDS-3e联合定轨，实验结果表明，两种方案对GPS/BDS-2/BDS-3e轨道影响无明显差异。而对于BDS-3，在不估计ISB参数情况下轨道影响小于估计ISB情况下的轨道，同样是由于可获得观测数据较少导致在待估参数增加的情况下整体参数精度降低，其具体原因有待进一步分析。上述实验间接说明引入新信号、新卫星，联合定轨策略中需要考虑北斗各类卫星之间的系统偏差参数。
2.3 联合定轨ISB参数建模分析
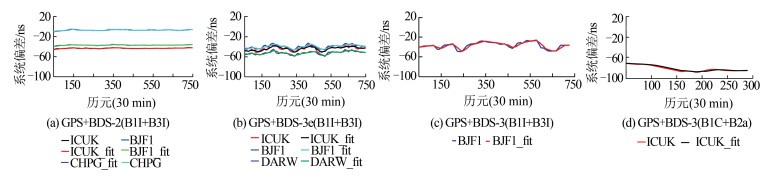
为分析联合定轨过程中ISB参数特性，利用§1.2中提出的顾及前后历元相关性的ISB解算模型，进行连续两周（2018年年积日第111天至第124天）的联合定轨实验。定轨过程中将测站坐标、地球自转参数以及对流层等参数施加强约束，以降低其他参数对ISB解算的影响；实验中ISB以30 min历元间隔分段估计。定轨方案中采用GPS+BDS-2、GPS+BDS-3、GPS+BDS-3e解算北斗各类卫星与GPS之间的系统偏差参数。实验中BDS-2与BDS-3e采用B1I/B3I观测数据，BDS-3同时对B1C/B2a进行实验分析。不同测站联合定轨ISB序列及其拟合曲线如图 5所示。
![]()
图 5 不同测站联合定轨ISB序列及其拟合曲线Figure 5. ISB Series and Its Corresponding Fit Results of Different Stations Based on Combined Orbit Determination由于ISB参数存在与接收机类型相关的分群现象，图 5中选取具有代表性的测站画出了其随历元变化的趋势（由于B1C/B2a数据质量问题，实验中只选取了连续10 d的结果）。需要注意的是，由于无法获取ISB参数相应的参考值，尚不能对ISB参数估计精度作出对比分析，本文只讨论利用估计出的ISB时间序列建模的效果。
图 5中，估计出的ISB时间序列结果表明：（1）不同频率、不同类型卫星估计出的ISB存在明显差异，这主要是由于卫星类型和频率之间差异导致的；（2）ISB参数存在明显周期特性，且同类卫星、同频率所估计出的ISB具有近似的周期和振幅。因此，针对联合定轨过程中不同类型北斗间偏差归为一类参数且定轨弧段内作为天稳定参数处理显然不合理，有必要针对不同接收机、不同卫星类型以及不同观测信号构建ISB时间序列模型。
为分析ISB变化趋势，基于接收机和卫星类型，采用文献[31]提出的函数模型，对不同接收机、卫星建立相应的ISB序列函数模型，即：
$$ \mathit{\boldsymbol{I}}=\mathit{\boldsymbol{A}}\times {t}^{2}+\mathit{\boldsymbol{B}}\times t+\mathit{\boldsymbol{C}}+\sum\limits _{i=1}^{n}({\mathit{\boldsymbol{D}}}_{i}\times \mathrm{c}\mathrm{o}\mathrm{s}(\frac{2\mathrm{\pi }t}{{\mathit{\boldsymbol{P}}}_{i}})+\\ \;\;\;\;\;\;{\mathit{\boldsymbol{E}}}_{i}\times \mathrm{s}\mathrm{i}\mathrm{n}(\frac{2\mathrm{\pi }t}{{\mathit{\boldsymbol{P}}}_{i}}\left)\right) $$ (28) 式中，A、B、C为趋势项系数；Di、Ei为周期项振幅；Pi为周期；t为相对起始时刻的时间间隔。各系数可通过最小二乘求解，周期则利用谱分析获得。
为验证上述ISB建模的可行性，利用式（28）分析不同联合定轨方案中不同测站、不同类型卫星对应的ISB曲线，如图 5所示。与之类似，由于同一类型卫星对应的ISB具有分群现象且趋势基本一致，图 5中仅绘出了具有代表性结果。同时，表 3中具体给出了对应于图 5中各测站ISB拟合残差的最大值、最小值、平均值与均方根误差（root mean square error，RMSE）。
表 3 各测站ISB拟合残差统计/nsTable 3. Fitting Residuals of ISB of Different Stations /ns频率 卫星 测站 最大值 最小值 平均值 RMSE B1I/B3I GPS+BDS-2 ICUK 0.608 0.001 0.013 0.008 BJF1 0.410 0.000 0.301 0.012 CHPG 0.809 0.002 0.286 0.224 GPS+BDS-3 ICUK 6.544 -0.005 0.298 1.204 BJF1 4.309 0.022 0.449 0.642 DARW 5.251 -0.014 0.155 0.993 GPS+BDS-3e BJF1 5.694 0.003 1.396 1.311 B1C/B2a GPS+BDS-3 ICUK 2.334 -0.102 0.063 0.159 由表 3中的实验结果可知，利用式（28）进行ISB建模，利用B1I/B3I观测数据解算出的BDS-2偏差参数建模，其误差可忽略不计；但BDS-3误差明显较大，无法实现准确建模。综上，针对BDS-2解算的ISB参数可较好地利用模型（28）进行描述，其RMSE优于0.25 ns；而BDS-3则受iGMAS测站受限等因素共同影响，出现个别测站拟合残差较大的情况，整体精度优于1.4 ns。通过对联合定轨中ISB参数准确建模，在时效性与精度严格要求的超快速轨道定轨中，可加入短期预报的ISB参数约束，提高超快速联合定轨精度。
2.4 超快速联合定轨实验
超快速轨道作为实时或近实时应用的重要产品，其精度与时效性是参数解算的核心指标。为顾及轨道参数解算的时效性，减少观测方程中参数，降低法方程维数，以及增加参数解算间隔等策略已探索应用。本文对GPS/BDS-2/BDS-3联合超快速轨道确定中ISB参数进行约束处理，以降低ISB参数对轨道的影响，提高参数解算的可靠性与精度。
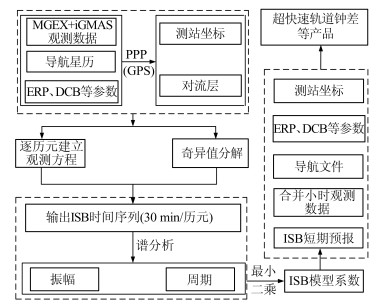
首先，基于提出的改进的联合定轨ISB解算模型，对解算的ISB序列进行准确建模与短期预报；其次，将预报的ISB参数作为约束条件加入超快速联合定轨中，实现顾及超快速轨道时效性与精度的指标。为验证超快速联合定轨中以预报ISB参数为约束条件的可行性，利用与§2.1相同的实验数据，设计了超快速联合定轨实验，具体实验步骤如图 6所示。
![]()
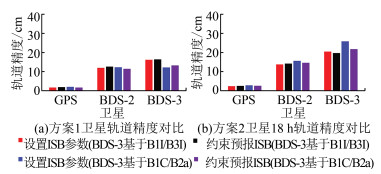
图 6 基于预报ISB的超快速轨道确定流程Figure 6. Flowchart of Ultra-Rapid Orbit Determination Based on Predicted ISB基于图 6的超快速轨道定轨流程，进行连续10 d（2018年年积日第110天至第119天）定轨实验，分析基于实时估计与约束预报ISB参数对定轨结果的影响。为充分分析不同ISB处理模型，设计了两套定轨实验方案。方案1：基于3天定轨弧段，分别利用实时估计（BDS-2与BDS-3相对于GPS设置不同的ISB参数）与约束预报的ISB参数进行GPS+BDS-2+BDS-3联合定轨，通过对比一天的重叠弧段分析ISB参数处理效果，其轨道精度如图 7（a）所示。方案2：如图 6所示，基于超快速定轨策略，分别约束预报的ISB与实时估计ISB参数进行超快速单天弧段联合轨道确定，对比18 h重叠弧段轨道不符值，其轨道精度如图 7（b）所示。
![]()
图 7 方案1与方案2轨道重叠弧段（1D RMSE）对比Figure 7. Accuracies Comparison of Overlapping Orbit Based on Scheme 1 and Scheme 2 (1D RMSE)上述两组对比实验分析了实时估计ISB参数与约束预报ISB对不同定轨模式（3天弧段与单天弧段）影响。图 7实验结果表明，观测数据充足的条件下（方案1），采用实时估计ISB参数的定轨策略获得的轨道重叠弧段精度优于约束预报ISB的定轨结果；而在观测数据较少条件下（方案2），预报单天ISB参数作为约束条件带入定轨中获得的超快速轨道重叠弧段精度略优于实时估计ISB参数方案结果。上述两组实验方案间接地证明了超快速联合定轨过程中，采用约束预报ISB参数方案，可一定程度上改善定轨的时效性与精度。为更具体描述轨道实验结果，表 4统计了各方案所对应ISB处理模式以及定轨精度。
表 4 不同定轨方案轨道重叠弧段不符值(1D RMSE) /cmTable 4. Accuracy of Orbit Overlapping (1D RMSE) Based on Different Schemes /cm卫星 方案 BDS-3/BDS-2(B1I B3I)/GPS(L1L2) BDS-2(B1IB3I)/ BDS-3(B1CB2a)/ GPS(L1L2) ISB估计 ISB预报 ISB估计 ISB预报 GPS 方案1 1.6 1.8 1.9 1.6 方案2 2.3 2.4 2.7 2.5 BDS-2 方案1 11.9 12.5 12.2 11.4 方案2 13.7 14.1 15.5 14.5 BDS-3 方案1 16.1 16.3 12.1 13.2 方案2 20.4 19.6 25.8 21.7 从表 4统计结果中可知，不同定轨方案以及ISB处理策略中，GPS轨道精度受影响较小，原因是GPS观测数据较充足，不同的定轨模式无明显差别。BDS-2超快速轨道精度变化为-0.4~1.0 cm。BDS-3轨道精度变化如下：方案1中采用B1I/B3I观测数据，其轨道重叠弧段不符值无明显差别；而采用B1C/B2a观测数据时，约束预报ISB参数进行联合定轨进一步降低轨道精度达1.1 cm。需要注意的是，方案2中约束预报ISB参数的超快速定轨精度明显优于设置ISB参数实时估计策略，其精度提升0.8~4.1 cm，主要是由于可跟踪BDS‑3测站较少，超快速定轨过程中无法准确求解各参数，引入高精度预报的ISB参数间接提高了轨道等其他参数解算精度。
综上，在GPS/BDS-2/BDS-3联合定轨过程中有必要考虑系统偏差；且在超快速轨道确定过程中，有必要采用约束短期预报ISB参数策略。
3 结语
本文针对BDS-2与BDS-3共存以及卫星间存在差异的现状，研究联合定轨中系统偏差参数处理策略。连续21 d联合定轨ISB参数解算结果表明，ISB参数存在以接收机类型分群现象，且BDS-2与BDS-3间存在明显的偏差，尤其是BDS-3新信号。
为提高ISB参数估计的准确性与可靠性，本文提出了一种改进的联合定轨ISB参数估计模型，即通过奇异值分解与历元间差分，充分保留相邻历元有益的观测信息，实现观测数据充分利用。联合定轨实验结果显示，在B1I/B3I观测信号条件下，BDS-2/BDS-3不存在明显的系统偏差。而当BDS-3采用B1C/B2a观测信号则存在较大的偏差，有必要对GPS/BDS-2/BDS-3联合定轨过程中ISB参数进行精化处理。
为分析ISB参数对联合定轨的影响，首先，基于参数之间的相关性分析发现ISB参数与北斗轨道各参数之间较GPS轨道参数相关性系数高两个量级；通过3组联合定轨对比实验验证了引入BDS-3新信号、新卫星条件下，联合定轨中需要对BDS-2/BDS-3间的偏差具体考虑。然后，基于估计的ISB时间序列，通过谱分析与最小二乘构建ISB模型，实现对ISB时间序列建模分析。最后，基于构建的ISB模型，通过将短期预报的ISB参数作为约束条件带入超快速联合定轨模型中，实现顾及超快速轨道时效性与精度的定轨策略。
通过两组定轨对比方案发现，GPS轨道受ISB参数影响较小，而北斗受影响较为明显。通过约束预报的ISB参数，超快速定轨结果显示可分别提高BDS-2与BDS-3轨道重叠弧段不符值-0.4 ~ 1.0 cm与0.8 ~ 4.1 cm。
综上，在GPS/BDS-2/BDS-3联合定轨过程中有必要考虑BDS-2/BDS-3间系统偏差，且在超快速轨道确定过程中采用约束短期预报ISB参数是有效的策略之一。
致谢: 感谢江苏省双创团队项目支持和国际GNSS监测评估系统（iGMAS）提供的数据产品。 -
![]()
图 1 联合定轨MGEX与iGMAS测站分布
Figure 1. Distribution of MGEX and iGMAS Stations of Combined Orbit Determination
![]()
图 2 北斗不同频率BDS/GPS联合定轨ISB时间序列
Figure 2. ISB Time Series Between GPS and BeiDou Combined Orbit Determination
![]()
图 3 基于B1I/B3I频率(BDS-3)不同ISB参数模式下精密定轨精度相对变化
Figure 3. Accuracy Variation of Precise Orbit Determination Based on Different ISB Models Under B1I/B3I
![]()
图 4 基于B1C/B2a频率(BDS-3)不同ISB参数模式下精密定轨精度相对变化
Figure 4. Accuracy Variation of Precise Orbit Determination Based on Different ISB Models Under B1C/B2a
![]()
图 5 不同测站联合定轨ISB序列及其拟合曲线
Figure 5. ISB Series and Its Corresponding Fit Results of Different Stations Based on Combined Orbit Determination
![]()
图 6 基于预报ISB的超快速轨道确定流程
Figure 6. Flowchart of Ultra-Rapid Orbit Determination Based on Predicted ISB
![]()
图 7 方案1与方案2轨道重叠弧段（1D RMSE）对比
Figure 7. Accuracies Comparison of Overlapping Orbit Based on Scheme 1 and Scheme 2 (1D RMSE)
表 1 联合定轨中BDS之间的系统偏差统计/ns
Table 1 Results of ISB Parameters Between BDS-2, BDS-3 and BDS-3e of Combined Orbit Determination/ns
测站 接收机类型 BDS-2/BDS-3e偏差
(B1I/B3I)BDS-2/BDS-3偏差
(B1I/B3I)BDS-2/BDS-3偏差
(B1C/B2a)BJF1 CETC-54-GMR-4016 0.71 0.58 27.28 BRCH 0.73 0.70 30.00 LHA1 1.03 0.63 28.37 WHU1 0.75 0.80 35.15 CLGY 0.74 0.98 37.03 ICUK 0.85 0.87 31.35 CANB CETC-54-GMR-4011 0.82 0.85 30.01 DWIN 0.75 0.84 39.00 PETH 0.85 0.83 24.16 ZHON 0.69 0.77 35.93 ABJA GNSS_GGR 0.81 0.81 23.66 HMNS -2.04 -1.85 22.99 XIA1 -0.32 -0.32 22.70 GUA1 SEPT POLARX5 0.71 0.63 43.05 CHU1 0.75 0.79 23.98 ARUC -0.96 0.43 ‒ STR1 -1.05 -0.10 ‒ DARW -0.99 0.76 ‒ HOB2 -1.01 0.29 ‒ CHPI -0.95 0.10 ‒ DAV1 -0.92 -0.67 ‒ TID1 -0.84 0.41 ‒ 
下载: 导出CSV
表 2 联合定轨中ISB与卫星轨道参数之间相关系数统计
Table 2 Correlation Factors Between ISB and GPS/BDS Satellite Orbit Parameters Based on Combined Orbit Determinations
测站 卫星 X Y Z VX VY VZ WHU1 G11 2.73×10-5 -1.55×10-9 -1.50×10-9 2.10×10-5 9.34×10-2 -1.42×10-2 WHU1 C11 1.98×10-3 4.70×10-7 1.03×10-7 -1.74×10-5 7.34×10-2 9.60×10-2 DARW C11 2.76×10-5 -1.44×10-9 -1.76×10-9 2.14×10-5 7.21×10-2 -1.35×10-2 DARW C11 1.99×10-3 4.71×10-7 1.03×10-7 -1.75×10-3 7.15×10-2 9.62×10-2
下载: 导出CSV
表 3 各测站ISB拟合残差统计/ns
Table 3 Fitting Residuals of ISB of Different Stations /ns
频率 卫星 测站 最大值 最小值 平均值 RMSE B1I/B3I GPS+BDS-2 ICUK 0.608 0.001 0.013 0.008 BJF1 0.410 0.000 0.301 0.012 CHPG 0.809 0.002 0.286 0.224 GPS+BDS-3 ICUK 6.544 -0.005 0.298 1.204 BJF1 4.309 0.022 0.449 0.642 DARW 5.251 -0.014 0.155 0.993 GPS+BDS-3e BJF1 5.694 0.003 1.396 1.311 B1C/B2a GPS+BDS-3 ICUK 2.334 -0.102 0.063 0.159
下载: 导出CSV
表 4 不同定轨方案轨道重叠弧段不符值(1D RMSE) /cm
Table 4 Accuracy of Orbit Overlapping (1D RMSE) Based on Different Schemes /cm
卫星 方案 BDS-3/BDS-2(B1I B3I)/GPS(L1L2) BDS-2(B1IB3I)/ BDS-3(B1CB2a)/ GPS(L1L2) ISB估计 ISB预报 ISB估计 ISB预报 GPS 方案1 1.6 1.8 1.9 1.6 方案2 2.3 2.4 2.7 2.5 BDS-2 方案1 11.9 12.5 12.2 11.4 方案2 13.7 14.1 15.5 14.5 BDS-3 方案1 16.1 16.3 12.1 13.2 方案2 20.4 19.6 25.8 21.7
下载: 导出CSV
-
[1] China Satellite Navigation Project Center. Compass/BeiDou Navigation Satellite System Development[R]. Beijing: China Satellite Navigation Project Center, 2009
[2] Yang Y, Li J, Xu J, et al. Contribution of the Compass Satellite Navigation System to Global PNT Users[J]. Chinese Science Bulletin, 2011, 56(26): 2 813-2 819 doi: 10.1007/s11434-011-4627-4
[3] Yang Y. Performance Analysis of BDS-3 Demonstration System[C]. International Symposium on GNSS, Hong Kong, China, 2017
[4] Zhou S, Hu X, Wu B, et al. Orbit Determination and Time Synchronization for a GEO/IGSO Satellite Navigation Constellation with Regional Tracking Network [J]. Science China: Physics, Mechanics and Astronomy, 2011, 54(6): 1 089-1 097 doi: 10.1007/s11433-011-4342-9
[5] Zhao Q, Wang C, Guo J, et al. Enhanced Orbit Determination for BeiDou Satellites with FengYun-3C Onboard GNSS Data[J]. GPS Solutions, 2017, 21(3): 1 179-1 190 doi: 10.1007/s10291-017-0604-y
[6] Guo J, Zhao Q. Analysis of Precise Orbit Determination for BeiDou Satellite During Yaw Maneuvers[C].The 5th China Satellite Navigation Conference, Nanjing, China, 2014
[7] Steigenberger P, Hugentobler U, Hauschild A, et al. Orbit and Clock Analysis of Compass GEO and IGSO Satellites[J]. Journal of Geodesy, 2013, 87(6): 515-525 doi: 10.1007/s00190-013-0625-4
[8] Dai X, Ge M, Lou Y, et al. Estimating the Yaw-Attitude of BDS IGSO and MEO Satellites[J]. Journal of Geodesy, 2015, 89(10): 1 005-1 018 doi: 10.1007/s00190-015-0829-x
[9] Shi C, Zhao Q, Min L, et al. Precise Orbit Determination of BeiDou Satellites with Precise Positioning[J]. Science China: Earth Sciences, 2012, 55(7): 1 079-1 086 doi: 10.1007/s11430-012-4446-8
[10] Wang Q, Zhang K, Wu S, et al. A Method for Identification of Optimal Minimum Number of Multi-GNSS Tracking Stations for Ultra-Rapid Orbit and ERP Determination[J]. Advances in Space Research, 2019, 63(9): 2 877-2 888 doi: 10.1016/j.asr.2017.12.006
[11] Ge M, Zhang H, Jia X, et al. What is Achievable with the Current Compass Constellation?[J]. GPS World, 2012, 23(11): 331-339 http://smartsearch.nstl.gov.cn/paper_detail.html?id=a00b348db5d18438d6c222c03ffb1ffe
[12] Montenbruck O, Hauschild A, Steigenberger P, et al. Initial Assessment of the COMPASS/BeiDou-2 Regional Navigation Satellite System[J]. GPS Solutions, 2013, 17(2): 211-222 doi: 10.1007/s10291-012-0272-x
[13] Tan B, Yuan Y, Wen M, et al. Initial Results of the Precise Orbit Determination for the New-Generation BeiDou Satellites (BeiDou-3) Based on the iGMAS Network[J]. International Society for Photogrammetry and Remote Sensing (ISPRS) International Journal of Geo-information, 2016, 5(11), DOI: 10.3390/ijgi5110196
[14] Zhao Q, Wang C, Guo J, et al. Precise Orbit and Clock Determination for BeiDou-3 Experimental Satellites with Yaw Attitude Analysis[J]. GPS Solutions, 2018, 22(1), DOI: org/10.1007/s10291-017-0673-y
[15] Ren X, Yang Y, Zhu J, et al. Orbit Determination of the Next-Generation BeiDou Satellites with Intersatellite Link Measurements and a Priori Orbit Constraints[J]. Advances in Space Research, 2017, 60(10): 2 155-2 165 doi: 10.1016/j.asr.2017.08.024
[16] Wen Y, Zhu J, Zhi L, et al. Simulation and Analysis of Integrated Orbit Determination of Satellites Constellation[J]. Journal of Astronautics, 2009, 30(1): 155-163 http://www.researchgate.net/publication/287760572_Simulation_and_analysis_of_integrated_orbit_determination_of_satellites_constellation
[17] Li X, Yuan Y, Zhu Y, et al. Precise Orbit Determination for BDS3 Experimental Satellites Using iGMAS and MGEX Tracking Networks[J]. Journal of Geodesy, 2019, 93(1): 103-117 doi: 10.1007/s00190-018-1144-0
[18] Zeng A, Yang Y, Ming F, et al. BDS-GPS Inter-system Bias of Code Observation and Its Preliminary Analysis[J]. GPS Solutions, 2017, 21(2): 1 573-1 581
[19] Odijk D, Teunissen P. Characterization of Between-Receiver GPS-Galileo Inter-system Biases and Their Effect on Mixed Ambiguity Resolution[J]. GPS Solutions, 2013, 17(4): 521-533 doi: 10.1007/s10291-012-0298-0
[20] Lou Y, Zheng F, Gu S, et al. Multi-GNSS Precise Point Positioning with Raw Single-Frequency and Dual-Frequency Measurement Models[J]. GPS Solutions, 2016, 20(4): 849-862 doi: 10.1007/s10291-015-0495-8
[21] Paziewski J, Wielgosz P. Accounting for GALILEO-GPS Inter-System Biases in Precise Satellite Positioning[J]. Journal of Geodesy, 2015, 89(1): 81-93 doi: 10.1007/s00190-014-0763-3
[22] Nadarajah N, Teunissen P, Raziq N. BeiDou Inter-Satellite-Type Bias Evaluation and Calibration for Mixed Receiver Attitude Determination[J]. Sensors, 2013, 13(7): 9 435-9 463 doi: 10.3390/s130709435
[23] Rabbou M, EI-Rabbany A. Performance Analysis of Precise Point Positioning Using Multi-constellation GNSS: GPS, GLONASS, Galileo and BeiDou[J]. Survey Review, 2017, 49(352): 39-50 doi: 10.1080/00396265.2015.1108068
[24] Liu T, Yuan Y, Zhang B, et al. Multi-GNSS Precise Point Positioning (MGPPP) Using Raw Observations[J]. Journal of Geodesy, 2017, 91(10): 253-268 doi: 10.1007/s00190-016-0960-3
[25] Chen J, Zhang Y, Wang J, et al. A Simplified and Unified Model of Multi-GNSS Precise Point Positioning[J]. Advances in Space Research, 2015, 55(1): 125-134 doi: 10.1016/j.asr.2014.10.002
[26] Hu C, Wang Q, Wang Z, et al. New-Generation BeiDou (BDS-3) Experimental Satellite Precise Orbit Determination with an Improved Cycle-Slip Detection and Repair Algorithm[J]. Sensors, 2018, 18(5), DOI: 10.3390/s18051402
[27] 胡超, 王潜心, 王中元, 等. 一种基于观测方程GDOP值的优化选站模型[J]. 武汉大学学报·信息科学版, 2017, 42(6): 838-844 doi: 10.13203/j.whugis20150550 Hu Chao, Wang Qianxin, Wang Zhongyuan, et al. An Optimal Stations Selected Model Based on the GDOP Value of Observation Equation [J]. Geomatics and Information Science of Wuhan University, 2017, 42(6): 838-844 doi: 10.13203/j.whugis20150550
[28] 胡超, 王潜心, 毛亚. 一种基于DOP值的GNSS超快速观测轨道精化模型[J]. 武汉大学学报·信息科学版, 2020, 45(1): 28-37 doi: 10.13203/j.whugis20180310 Hu Chao, Wang Qianxin, Mao Ya. An Improved Model for the Observed GNSS Ultra-Rapid Orbit Based on DOP Values[J]. Geomatics and Information Science of Wuhan University, 2020, 45(1): 28-37 doi: 10.13203/j.whugis20180310
[29] 骆飞. GNSS位置域载波平滑伪距技术研究[D]. 徐州: 中国矿业大学, 2019 Luo Fei. Research on GNSS Position-Domain Carrier-Smooth-Code Technology[D]. Xuzhou: China University of Mining and Technology, 2019
[30] Akaike H. Infomation Theory and an Extension of the Maximum Likelihood Principle[C]. The Second International Symposium on Information Theory, Budapest, Hungarian, 1973
[31] Jiang N, Xu Y, Xu T, et al. GPS/BDS Short-Term ISB Modelling and Prediction[J]. GPS Solutions, 2015, 21(1), DOI: 10.1007/s10291-015-0513-x
-
期刊类型引用(5)
1. 花奋奋，王萌萌，于洋，卢丽君，黄国满. 融合枝切法和最小费用流的多级相位解缠方法. 测绘科学. 2022(12): 138-146+183 . 
百度学术
2. 唐桂彬，周波. 基于遥感GIS的地震应急信息质量控制系统设计. 计算机测量与控制. 2021(10): 98-102 . 百度学术
3. 景贵飞. 遥感可信度及可信系统互操作分析. 遥感信息. 2020(02): 1-7 . 百度学术
4. 黄国满，程春泉，赵争，卢丽君，杨书成. 机载SAR遥感测图技术及应用. 测绘科学. 2019(06): 105-113 . 百度学术
5. 金鼎坚，王建超，吴芳，高子弘，韩亚超，李奇. 航空遥感技术及其在地质调查中的应用. 国土资源遥感. 2019(04): 1-10 . 百度学术
其他类型引用(1)
计量
- 文章访问数: 1343
- HTML全文浏览量: 335
- PDF下载量: 118
- 被引次数: 6
