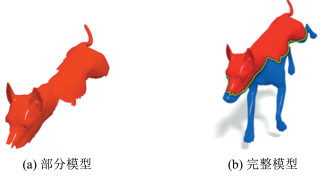
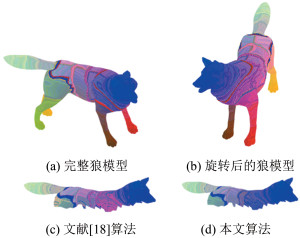
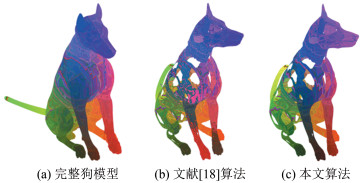
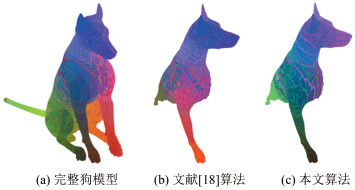
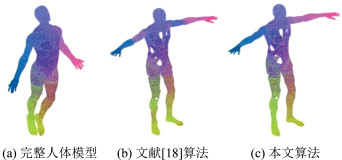
-
摘要: 针对不同姿态下的三维等距部分模型与完整模型对应关系计算问题，提出了一种结合局部函数映射和局部流形谐波(localized manifold harmonics，LMH)算子计算三维模型特征描述符并构建模型间对应关系的新方法。首先，通过改进的Laplace算子的谱分解构造局部基产生LMH算子，并计算模型的特征描述符；其次，通过局部函数映射理论构建部分模型与完整模型间的初始对应关系；然后，交替迭代计算部分模型与完整模型间的稠密对应关系；最后，利用贪心算法优化对应关系直至收敛。实验结果表明，以局部流形谐波产生的新算子与局部函数映射方法计算得到的稀疏对应关系为基础，能构建更为准确的稠密对应关系，并在一定程度上减少等距误差。和已有算法相比，采用LMH算子构建的特征描述符比由Laplace-Beltrami算子构建的特征描述符更能体现出部分模型的本征属性，计算出的对应关系也更加准确。Abstract:Objectives Constructing meaningful correspondence between two or more models is a very important basic research work. Regards the calculation issue of the correspondence between the partial 3D isometric shape and full shape, a new method for constructing the correspondence among shapes by computing 3D shape feature descriptors with partial functional map and localized manifold harmonics (LMH) operators is proposed.Methods Firstly, the LMH operator is generated by the spectral decomposition from the improved Laplace and the feature descriptor of the shape is calculated. Secondly, the initial correspondence between the partial shape and the full shape is constructed by the partial functional map theory. Then the dense correspondence between the partial shape and the full shape is iteratively calculated. Finally, the correspondence can be optimized by the greedy algorithm till convergence.Results Compared with the existing algorithms in TOSCA dataset, the feature descriptors constructed by the LMH operator used in this paper better reflects the intrinsic properties of some shapes than the feature descriptors constructed by Laplace-Beltrami operators, and the calculated correspondences between full and partial shapes (some with holes) are more accurate as well.Conclusions The new operator generated by the local manifold harmonics and the sparse correspondence calculated by the partial functional map method can be used to construct a more accurate dense correspondence and therefore it can reduce the isometric error to some extent.
-
-
![]()
图 1 Laplace–Beltrami算子在三角网格上的离散化
Figure 1. Discretization of the Laplace-Beltrami Operator on a Triangular Mesh
![]()
图 3 完整狼模型与带孔洞狼模型的对应关系比较
Figure 3. Comparison of the Constructed Correspondence Between the Full and the Holed Wolf Shapes
![]()
图 4 完整狼模型和部分狼模型的对应关系比较
Figure 4. Comparison of the Constructed Correspondence Between the Full and the Partial Wolf Shapes
![]()
图 5 完整狗模型与带孔洞狗模型的对应关系比较
Figure 5. Comparison of the Constructed Correspondence Between the Full and the Holed Dog Shapes
![]()
图 6 完整狗模型与部分狗模型的对应关系比较
Figure 6. Comparison of the Constructed Correspondence Between the Full and the Partial Dog Shapes
![]()
图 7 完整马模型与带孔洞马模型的对应关系比较
Figure 7. Comparison of the Constructed Correspondence Between the Full and the Holed Horse Shapes
![]()
图 8 完整马模型与部分马模型的对应关系比较
Figure 8. Comparison of the Constructed Correspondence Between the Full and the Partial Horse Shapes
![]()
图 9 完整人体模型与带孔洞人体模型的对应关系比较
Figure 9. Comparison of the Constructed Correspondence Between the Full and the Holed Man Shapes
![]()
图 10 完整人体模型与部分人体模型的对应关系比较
Figure 10. Comparison of the Constructed Correspondence Between the Full and the Partial Man Shapes
表 1 两种算法的部分模型与完整模型间等距误差的比较
Table 1 Comparison of the Isometric Errors for Calculating the Shape Correspondences Between Partial Shape and Full Shape of Two Algorithms
模型 等距误差 文献[18]算法 本文算法 带孔洞狼模型 0.098 724 0.087 807 部分狼模型 0.137 226 0.116 253 带孔洞狗模型 0.042 952 0.034 749 部分狗模型 0.033 408 0.032 362 带孔洞马模型 0.087 351 0.085 248 部分马模型 0.087 298 0.069 958 带孔洞人体模型 0.061 264 0.048 689 部分人体模型 0.057 785 0.041 543 
下载: 导出CSV
-
[1] VanKaick O, Zhang H, Hamarneh G, et al. A Survey on Shape Correspondence[J]. Computer Graphics Forum, 2011, 30(6): 1 681-1 707 doi: 10.1111/j.1467-8659.2011.01884.x
[2] Golovinskiy A, Funkhouser T. Consistent Segmentation of 3D Models[J]. Computers and Graphics, 2009, 33(3): 262-269 doi: 10.1016/j.cag.2009.03.010
[3] Kilian M, Mitra N J, Pottmann H. Geometric Modeling in Shape Space[J]. ACM Transactions on Graphics, 2007, 26(3), DOI: 10.1145/1276377.1276457
[4] Schreiner J, Asirvatham A, Praun E, et al. Inter-Surface Mapping[J]. ACM Transactions on Graphics, 2004, 23(3): 870-877 doi: 10.1145/1015706.1015812
[5] Jain V, Zhang H. A Spectral Approach to Shape-Based Retrieval of Articulated 3D Models[J]. Computer-Aided Design, 2007, 39(5): 398-407 doi: 10.1016/j.cad.2007.02.009
[6] Raviv D, Bronstein A M, Bronstein M M, et al. Equi-Affine Invariant Geometry for Shape Analysis[J]. Journal of Mathematical Imaging and Vision, 2014, 50(1/2): 144-163
[7] Sahillioglu Y, Yemez Y. Multiple Shape Correspondence by Dynamic Programming[J]. Computer Graphics Forum, 2015, 33(7): 121-130
[8] Lipman Y, Funkhouser T. Mobius Voting for Surface Correspondence[J]. ACM Transactions on Graphics, 2009, 28(3), DOI: 10.1145/1576246.1531378
[9] Aigerman N, Poranne R, Lipman Y. Seamless Surface Mappings[J]. ACM Transactions on Graphics, 2015, 34(4), DOI: 10.1145/2766921
[10] Aigerman N, Lipman Y. Hyperbolic Orbifold Tutte Embeddings[J]. ACM Transaction on Graphics, 2016, 35(6), DOI: 10.1145/1576246.153137
[11] Ovsjanikov M, Ben-Chen M, Solomon J, et al. Functional Maps: A Flexible Representation of Maps Between Shapes[J]. ACM Transactions on Graphics, 2012, 31(4), DOI: 10.1145/1576246.1531378
[12] Sun J, Ovsjanikov M, Guibas L. A Concise and Provably Informative Multi-scale Signature Based on Heat Diffusion[J]. Computer Graphics Forum, 2009, 28(5): 1 383-1 392 doi: 10.1111/j.1467-8659.2009.01515.x
[13] Aubry M, Schlickewei U, Cremers D. The Wave Kernel Signature: A Quantum Mechanical Approach to Shape Analysis[C]. 2011 IEEE International Conference on Computer Vision Workshops, Barcelona, Spain, 2011
[14] 杨军, 闫寒, 王茂正. 融合特征描述符约束的3维等距模型对应关系计算[J]. 中国图象图形学报, 2016, 21(5): 628-635 https://www.cnki.com.cn/Article/CJFDTOTAL-ZGTB201605010.htm Yang Jun, Yan Han, Wang Maozheng. Calculation of Correspondences Between Three-Dimensional Isometric Shapes with the Use of a Fused Feature Descriptor[J]. Journal of Image and Graphics, 2016, 21(5): 628-635 https://www.cnki.com.cn/Article/CJFDTOTAL-ZGTB201605010.htm
[15] Melzi S, Rodolà E, Castellani U, et al. Localized Manifold Harmonics for Spectral Shape Analysis[J]. Computer Graphics Forum, 2018, 37(6): 20-34 doi: 10.1111/cgf.13309
[16] Kim V, Lipman Y, Funkhouser T. Blended Intrinsic Maps[J]. ACM Transactions on Graphics, 2011, 30(4), DOI: 10.1145/2010324.1964974
[17] 杨军, 闫寒. 校准三维模型基矩阵的函数映射的对应关系计算[J]. 武汉大学学报·信息科学版, 2018, 43(10): 1 518-1 525 doi: 10.13203/j.whugis20160493 Yang Jun, Yan Han. An Algorithm for Calculating Shape Correspondences Using Functional Maps by Calibrating Base Matrix of 3D Shapes[J]. Geomatics and Information Science of Wuhan University, 2018, 43(10): 1 518-1 525 doi: 10.13203/j.whugis20160493
[18] Rodolà E, Cosmo L, Bronstein M M, et al. Partial Functional Correspondence[J]. Computer Graphics Forum, 2017, 36(1): 222-236 doi: 10.1111/cgf.12797
[19] Litany O, Bronstein A M, Bronstein M M, et al. Non-rigid Puzzles[C]. Symposium on Geometry Processing, Eurographics Association, Berlin, Germany, 2016
[20] Litany O, Rodolà E, Bronstein A M, et al. Fully Spectral Partial Shape Matching[J]. Computer Graphics Forum, 2017, 36(2): 247-258 doi: 10.1111/cgf.13123
计量
- 文章访问数: 1091
- HTML全文浏览量: 269
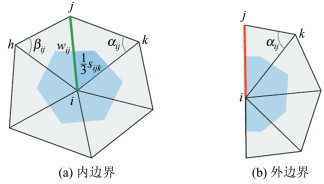
- PDF下载量: 51
