Tropospheric Delay Prediction Based on Phase Space Reconstruction and Gaussian Process Regression
-
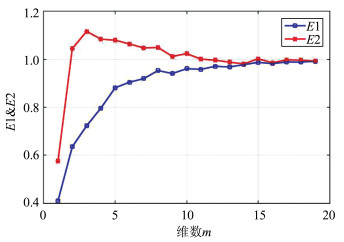
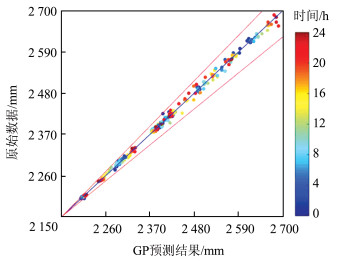
摘要: 天顶对流层延迟(zenith tropospheric delay，ZTD)是影响GPS定位精度的关键因素，为了提高ZTD的预测精度，提出一种基于相空间重构的高斯过程回归预测模型。针对ZTD时间序列的混沌特性，利用国际GNSS服务(International GNSS Service，IGS)站提供的ZTD数据，采用Cao方法确定嵌入维数，对ZTD数据进行相空间重构，探究高斯过程(Gaussian process，GP)模型对12个位于南、北半球不同纬度等级IGS站的ZTD预测精度和准确性。为了验证GP模型的有效性，将预测结果分别与原始数据和反向传播(back propagation，BP)神经网络模型预测结果作对比分析，进一步探究不同时间对ZTD预测精度的影响，并分析了经度和海拔对ZTD预测精度的影响。结果表明，GP模型预测结果的均方根误差(root mean square error，RMSE)达到mm级，GP模型与理论值的相关性达到0.997，预测精度指标明显优于BP神经网络模型；GP模型在南半球的预测精度高于北半球，且在高纬地区的RMSE小于3.6 mm，更适用于高纬地区的对流层延迟预测；在研究时域内，GP模型在大部分站点对晚上的预测精度高于白天，经度对ZTD预测精度的影响不明显，海拔与ZTD预测精度呈正比。Abstract: Zenith tropospheric delay (ZTD) is a key factor affecting global positioning system (GPS) positioning accuracy. In order to improve the prediction accuracy of ZTD, a Gaussian process(GP) regression prediction model based on phase space reconstruction is proposed.In view of the chaotic characteristics of ZTD time series, using the ZTD data provided by the International Global Navigation Satellite System Service (IGS) stations.Firstly, the embedded dimension is determined using Cao method, phase space reconstruction of ZTD data is carried out, and the precision and accuracy of ZTD using GP model for 12 IGS ststions at different latitude levels in the southern and northern hemisphere are explored.Then, in order to verify the effectiveness of GP model, the prediction results are compared with the original data and prediction results of the back propagation (BP) neural network model, and the influence of different time on the prediction accuracy of ZTD is further explored. Finally, the influence of longitude and altitude on the prediction accuracy of ZTD is analyzed.The results show that the root mean square error (RMSE) of GP model prediction results reaches mm level, the correlation between GP model and theoretical value reaches 0.997, and the prediction accuracy index is obviously better than that of BP neural network model. The prediction accuracy of GP model in the southern hemisphere is higher than that in the northern hemisphere, and RMSE in the high latitude area is less than 3.6 mm, which is more suitable for the tropospheric delay prediction in the high latitude area. In the time domain of the study, the prediction accuracy of GP model at night is higher than that in the day at most sites, the longitude has no obvious influence on the prediction accuracy of ZTD, and the altitude is proportional to the prediction accuracy of ZTD. Therefore, GP model has better practicability and feasibility for the prediction of tropospheric delay.
-
在移动测量系统，如车载或机载的移动摄影测量系统和激光扫描系统中，除了需要载体的位置信息外，还需要知道载体的姿态信息[1-2]。获取姿态信息最直接的方法是采用惯性导航系统(inertial navigation system, INS)，然而，INS价格昂贵，且存在姿态误差随时间积累等不足因素[3-4]，实际应用中还需要利用其他设备对其进行辅助[5-6]。近年来，随着全球卫星导航系统(glo-bal navigation satellite system, GNSS)动态定位技术的发展，利用多天线的GNSS测量载体姿态的方法受到越来越多的关注[7-11]。多天线GNSS定姿经济方便，使用灵活，并且姿态精度稳定，误差不会随时间积累，具有一定的优势。利用两副天线可以测量载体的航向角和俯仰角，同时测量载体的3个姿态角，至少需要3副天线。
利用多天线GNSS定姿的方法一般有两类，一类是先解算天线间的基线向量，然后利用基线向量解算载体的姿态[12-13]，这类定姿方法相对直观，并且可以利用基线长度辅助模糊度固定，提高基线解算质量；还有一类是将载体的姿态4元素或姿态角作为待解参数，与整周模糊度一起求解[14-17]，这类方法需要姿态的初始值，如果初始值精度较差，则难以收敛。
由基线向量解算姿态的方法主要有直接法和基于最小二乘法的迭代法[18-19]。迭代法是在有初始姿态的情况下，利用每个历元天线间的基线向量在载体系和导航系的坐标迭代计算载体姿态[18], 这种方法需要事先测量天线在载体系的坐标，解算过程中需要进行矩阵求逆，存在奇异性问题[19]，并且当初始姿态精度较差时，容易发散[20]；直接法是直接利用天线间的基线向量在导航系的坐标解算载体的姿态，此方法计算简便，利用两条基线向量就可以解算姿态，不存在奇异性问题[19]。但由于这种方法直接解算姿态角，需要对姿态角的象限进行判断，存在多值性问题。
本文主要研究基于三天线GNSS的直接法定姿，给出姿态解算公式，针对航向角存在的多值性问题，给出一种简便的方法确定航向角的取值。为了检验本文定姿方法的精度和可靠性，用车载三天线GNSS获取的实测数据进行姿态解算，并用同平台的高精度INS对三天线GNSS定姿的精度和可靠性进行评估。
1 三天线GNSS定姿方法
姿态指载体相对于参考坐标系的角位置。在导航和移动测量中，参考坐标系一般取导航坐标系，则载体的姿态即为载体系(b系)相对于导航系(n系)的角位置。n系至b系的变换可通过3次旋转来实现：n系先绕Z轴顺时针旋转γ角，再绕X轴逆时针旋转β角，最后绕Y轴逆时针旋转α角。γ、β、α构成一组欧拉角，称为载体的姿态角，其中α为横滚角，β为俯仰角，γ为航向角。载体的姿态除了可用姿态角表示之外，亦可用姿态矩阵表示，n系至b系的坐标变换矩阵Cnb定义为载体的姿态矩阵。姿态矩阵和姿态角之间的关系可表示为[21]:
$$ \begin{array}{*{20}{c}} {\mathit{\boldsymbol{C}}_n^b = {\mathit{\boldsymbol{R}}_2}\left( \alpha \right){\mathit{\boldsymbol{R}}_1}\left( \beta \right){\mathit{\boldsymbol{R}}_3}\left( { - \gamma } \right) = }\\ {\left[ {\begin{array}{*{20}{c}} {\cos \alpha }&0&{ - \sin \alpha }\\ 0&1&0\\ {\sin \alpha }&0&{\cos \alpha } \end{array}} \right]\left[ {\begin{array}{*{20}{c}} 1&0&0\\ 0&{\cos \beta }&{\sin \beta }\\ 0&{ - \sin \beta }&{\cos \beta } \end{array}} \right]\left[ {\begin{array}{*{20}{c}} {\cos \gamma }&{ - \sin \gamma }&0\\ {\sin \gamma }&{\cos \gamma }&0\\ 0&0&1 \end{array}} \right] = }\\ {\left[ {\begin{array}{*{20}{c}} {\cos \alpha \cos \gamma + \sin \alpha \sin \beta \sin \gamma }&{ - \cos \alpha \sin \gamma + \sin \alpha \sin \beta \cos \gamma }&{ - \sin \alpha \cos \beta }\\ {\cos \beta \sin \gamma }&{\cos \beta \cos \gamma }&{\sin \beta }\\ {\sin \alpha \cos \gamma - \cos \alpha \sin \beta \sin \gamma }&{ - \sin \alpha \sin \gamma - \cos \alpha \sin \beta \cos \gamma }&{\cos \alpha \cos \beta } \end{array}} \right]} \end{array} $$ (1) 由于n系和b系均为直角坐标系，姿态矩阵为正交矩阵[21]，即(Cnb)T=(Cnb)－1，b系至n系的坐标变换矩阵记为Cbn，有Cbn=(Cnb)－1=(Cnb)T。
将3副天线固定于车顶，取天线1为主天线，其相位中心的位置作为载体系的原点，天线1与天线2的连线与车辆纵轴平行并指向车头方向，构成载体系的Y轴，X轴在车顶平面内垂直于Y轴，Z轴垂直于车顶指向天顶方向，并与X轴和Y轴构成右手坐标系，将此坐标系记为载体坐标系O-XbYbZb，如图 1所示。则天线1、2、3在载体系的坐标分别为(0, 0, 0)、(0, L12, 0)、(L13sinθ, L13cosθ, 0)。
在每个观测历元，通过精密单点定位可以获得天线1在WGS-84系的坐标，通过动态基线解算可以获得WGS-84系下天线1至天线2、天线1至天线3的基线向量，分别用s12, e、s 13, e表示：
$$ {\mathit{\boldsymbol{s}}_{12,e}} = \left[ {\begin{array}{*{20}{c}} {{x_{12,e}}}\\ {{y_{12,e}}}\\ {{z_{12,e}}} \end{array}} \right] = \left[ {\begin{array}{*{20}{c}} {{x_{2,e}} - {x_{1,e}}}\\ {{y_{2,e}} - {y_{1,e}}}\\ {{z_{2,e}} - {z_{1,e}}} \end{array}} \right] $$ (2) $$ {\mathit{\boldsymbol{s}}_{13,e}} = \left[ {\begin{array}{*{20}{c}} {{x_{13,e}}}\\ {{y_{13,e}}}\\ {{z_{13,e}}} \end{array}} \right] = \left[ {\begin{array}{*{20}{c}} {{x_{3,e}} - {x_{1,e}}}\\ {{y_{3,e}} - {y_{1,e}}}\\ {{z_{3,e}} - {z_{1,e}}} \end{array}} \right] $$ (3) 将其变换到导航系，有：
$$ {\mathit{\boldsymbol{s}}_{12,n}} = \left[ {\begin{array}{*{20}{c}} {{x_{12,n}}}\\ {{y_{12,n}}}\\ {{z_{12,n}}} \end{array}} \right] = \mathit{\boldsymbol{C}}_e^n{\mathit{\boldsymbol{s}}_{12,e}} $$ (4) $$ {\mathit{\boldsymbol{s}}_{13,n}} = \left[ {\begin{array}{*{20}{c}} {{x_{13,n}}}\\ {{y_{13,n}}}\\ {{z_{13,n}}} \end{array}} \right] = \mathit{\boldsymbol{C}}_e^n{\mathit{\boldsymbol{s}}_{13,e}} $$ (5) Cen可由天线1的WGS-84坐标计算，得：
$$ \mathit{\boldsymbol{C}}_e^n{\rm{ = }}\left[ {\begin{array}{*{20}{c}} { - \sin \lambda }&{\cos \lambda }&0\\ { - \cos \lambda \sin \mathit{\Phi }}&{ - \sin \lambda \sin \mathit{\Phi }}&{\cos \mathit{\Phi }}\\ {\cos \lambda \cos \mathit{\Phi }}&{\sin \lambda {\cos}\mathit{\Phi }}&{\sin \mathit{\Phi }} \end{array}} \right] $$ (6) λ和Φ为天线1的经度和纬度，由精密单点定位获得。两基线向量在载体系的坐标为：
$$ {\mathit{\boldsymbol{s}}_{12,b}} = \left[ {\begin{array}{*{20}{c}} 0\\ {{L_{12}}}\\ 0 \end{array}} \right] $$ (7) $$ {\mathit{\boldsymbol{s}}_{13,b}} = \left[ {\begin{array}{*{20}{c}} {{L_{13}}\sin \theta }\\ {{L_{13}}\cos \theta }\\ 0 \end{array}} \right] $$ (8) 天线1至天线2的基线向量在载体系和导航系的坐标之间的关系为：
$$ {\mathit{\boldsymbol{s}}_{12,n}} = \mathit{\boldsymbol{C}}_b^n{\mathit{\boldsymbol{s}}_{12,b}} = {\left( {\mathit{\boldsymbol{C}}_b^n} \right)^{\rm{T}}}{\mathit{\boldsymbol{s}}_{12,b}} $$ (9) 将式(1)、式(4)、式(7)代入式(9)得：
$$ \left[ {\begin{array}{*{20}{c}} {{x_{12,n}}}\\ {{y_{12,n}}}\\ {{z_{12,n}}} \end{array}} \right] = \left[ {\begin{array}{*{20}{c}} {\cos \beta \sin \gamma }\\ {\cos \beta \cos \gamma }\\ {\sin \beta } \end{array}} \right]{L_{12}} $$ (10) 由式(10)可得航向角为：
$$ \gamma = \arctan \left( {\frac{{{x_{12,n}}}}{{{y_{12,n}}}}} \right) $$ (11) 俯仰角为:
$$ \beta = \arctan \left( {\frac{{{z_{12,n}}}}{{\sqrt {x_{12,n}^2 + y_{12,n}^2} }}} \right) $$ (12) 天线1至天线3的基线向量在载体系和导航系的坐标之间的关系为：
$$ {\mathit{\boldsymbol{s}}_{13,b}} = \mathit{\boldsymbol{C}}_n^b{\mathit{\boldsymbol{s}}_{13,n}} $$ (13) 将式(1)、式(5)、式(8)代入式(13)得:
$$ \left[ {\begin{array}{*{20}{c}} {{L_{13}}\sin \theta }\\ {{L_{13}}\cos \theta }\\ 0 \end{array}} \right] = \\\left[ {\begin{array}{*{20}{c}} {\cos \alpha }&0&{ - \sin \alpha }\\ 0&1&0\\ {\sin \alpha }&0&{\cos \alpha } \end{array}} \right]\left[ {\begin{array}{*{20}{c}} {{x_{13,n}}\cos \gamma - {y_{13,n}}\sin \gamma }\\ {{x_{13,n}}\cos \beta \sin \gamma + {y_{13,n}}\cos \beta \cos \gamma + {z_{13,n}}\sin \beta }\\ { - {x_{13,n}}\sin \beta \sin \gamma - {y_{13,n}}\sin \beta \cos \gamma + {z_{13,n}}\cos \beta } \end{array}} \right] $$ (14) 由式(14)可得横滚角为:
$$ \alpha = - \arctan \left( {\frac{{{{z''}_{13,n}}}}{{{{x''}_{13,n}}}}} \right) $$ (15) 式中，
$$ {{x''}_{13,n}} = {x_{13,n}}\cos \gamma - {y_{13,n}}\sin \gamma $$ (16) $$ \begin{array}{l} {{z''}_{13,n}} = - {x_{13,n}}\sin \beta \sin \gamma - \\ \;\;\;\;\;\;\;\;{y_{13,n}}\sin \beta \cos \gamma + {z_{13,n}}\cos \beta \end{array} $$ (17) 2 航向角取值判定
从式(11)、式(12)及式(15)可以看出，3个姿态角的表达式均为反正切函数，反正切函数的取值范围为－90°~90°，而航向角的取值为0°~360°，俯仰角的取值为－90°~90°，横滚角的取值为－180°~180°。因此俯仰角不存在多值性问题，横滚角在实际应用中由于载体的运动特性会受限于一定的取值范围之内，也不存在多值性问题，下面给出航向角取值的判定方法。
航向角为导航系(n系)至载体系(b系)的第一次旋转形成的角度，此旋转绕Zn轴进行。旋转后n系及b系的位置、天线1至天线2的基线向量旋转前后的位置如图 2所示。
由式(11)可知，航向角只与天线1至天线2的基线向量在n系的坐标分量x12, n和y12, n有关。图 2标出了航向角分别在4个象限时b系的位置及x12, n和y12, n的取值，由此，即可通过x12, n和y12, n的符号来判定航向角的象限，如表 1所示，γ0为直接由航向角的表达式计算得到的初始值。
表 1 航向角符号与x12, n、y12, n的关系Table 1. Relationship Between the Sign of the Yaw Angle and x12, n, y12, nx12, n的符号 y12, n的符号 象限 航向角γ取值 + + 0°~90° γ0 + - 90°~180° γ0+180° - - 180°~270° γ0+180° - + 270°~360° γ0+360° 3 实验与结果分析
为了检验三天线GNSS直接法定姿的精度和可靠性，进行了车载实验。实验车搭载了一套NovAtel公司的三天线GNSS，采样频率为1 Hz，用于姿态测量。同时随车搭载了一套高精度的INS，陀螺漂移为0.006°/h，采样频率为200 Hz，用于获取姿态参考值，对三天线GNSS定姿结果进行外符合精度检验。3副GNSS天线按照图 1所示固定于车顶，天线1和天线2之间的基线长度为2.641 m，天线1和天线3之间的基线长度为2.820 m，实验共采集了3 h的动态观测数据，车速40 km/h左右。
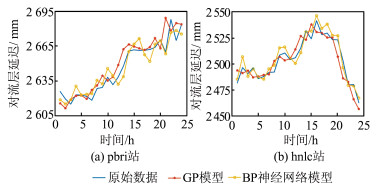
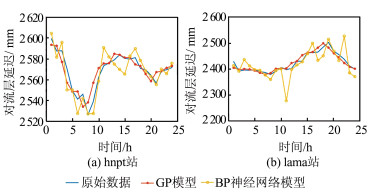
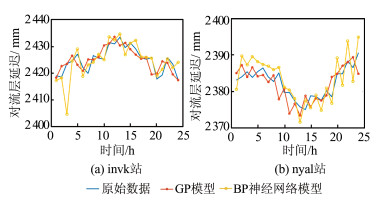
数据处理时，先利用精密星历和精密钟差解算天线1的WGS-84坐标，再解算天线1至天线2和天线1至天线3之间的动态基线。然后利用天线1的WGS-84坐标将基线坐标变换到导航系，利用导航系下的基线坐标解算载体姿态角，并用本文给出的方法判定航向角的取值。解得的姿态角与高精度INS的比较如图 3~5所示。
![]()
图 3 三天线GNSS测得的航向角与高精度INS的比较Figure 3. Comparison of the Yaw Angle Determined from Three-antenna GNSS and INS![]()
图 4 三天线GNSS测得的俯仰角与高精度INS的比较Figure 4. Comparison of the Pitch Angle Determined from Three-antenna GNSS and INS![]()
图 5 三天线GNSS测得的横滚角与高精度INS的比较Figure 5. Comparison of the Roll Angle Determined from Three-antenna GNSS and INS从图 3~5可以看出，在动态条件下，三天线GNSS定姿法能够很好地捕捉到载体姿态的变化，并与高精度INS给出的姿态参考值符合较好，姿态精度稳定。只有456 000~457 500 s这段时间精度较差，与观测条件较差有关。通过数据分析可以发现，三天线GNSS测得的航向角和横滚角与高精度INS之间存在一定的系统差，航向角差0.546°，横滚角差0.678°，这一系统差主要来源于GNSS天线与INS在安装时产生的系统偏差。去掉这一系统差之后，三天线GNSS定姿的误差如图 6所示。
从式(11)、式(12)及式(15)可以看出，基线向量的精度对姿态角的精度起着决定性的作用。航向角和俯仰角的精度和天线1至天线2之间的基线精度相关，而横滚角的精度和天线1至天线3之间的基线精度相关。基线解算精度与天线接收到的卫星信号质量及卫星分布相关。除此之外，姿态解算精度还与天线布局有关。从图 6可以看出，三天线直接法定姿得到的航向角误差最小，俯仰角误差稍大，横滚角误差最大，这是因为航向角和俯仰角的精度与天线1和天线2之间的基线长度成正比，横滚角的精度与天线2至天线3之间的基线长度在横轴方向的投影成正比。并且从式(11)、式(12)及式(15)分析可以发现，在计算航向角时用的是基线坐标的比值，计算俯仰角时进行了乘方和开方运算，放大了噪声，而计算横滚角时用到了航向角与俯仰角，所以噪声也会更大。3个姿态角的误差统计如表 2所示。
表 2 姿态角误差统计/(°)Table 2. Statistics of the Attitude Error/(°)航向角 俯仰角 横滚角 最大值 1.121 3.999 12.651 最小值 -1.898 -5.666 -16.013 标准差 0.168 0.397 0.974 4 结语
本文研究了三天线GNSS直接法动态定姿的方法，针对直接法定姿存在的航向角多值性问题，给出一种判定航向角取值的简便方法。利用车载实测数据进行了姿态解算，对本文的方法进行了验证。车载实验时两条基线长度分别为2.641 m和2.820 m，利用本文定姿方法解算得到的姿态外符合精度分别为航向角0.168°，俯仰角0.397°，横滚角0.974°。实验结果表明，三天线GNSS定姿法能很好地追踪移动载体的姿态变化，并且精度稳定，可靠性高。在良好的观测条件下，可以长时间保持较高的精度，相对于低精度的INS系统具有一定的优势。若建立实时通讯系统，还可以为移动测量系统提供实时的姿态信息。
-
![]()
图 1 Cao方法确定pbri站点的最小嵌入维数
Figure 1. Minimum Embedded Dimension for the pbri Site Using Cao Method
![]()
图 2 两种模型在北半球低纬地区的ZTD预测结果对比
Figure 2. Comparison of ZTD Prediction Results for Two Models in Low Latitude Areas of the Northern Hemisphere
![]()
图 3 两种模型在北半球中纬地区的ZTD预测结果对比
Figure 3. Comparison of ZTD Prediction Results for Two Models in Mid-latitude Areas of the Northern Hemisphere
![]()
图 4 两种模型在北半球高纬地区的ZTD预测结果对比
Figure 4. Comparison of ZTD Prediction Results for Two Models in High Latitude Areas of the Northern Hemisphere
![]()
图 5 两种模型在南半球低纬地区的ZTD预测结果对比
Figure 5. Comparison of ZTD Prediction Results for Two Models in Low Latitude Areas of the Southern Hemisphere
![]()
图 6 两种模型在南半球中纬地区的ZTD预测结果对比
Figure 6. Comparison of ZTD Prediction Results for Two Models in Mid-latitude Areas of the Southern Hemisphere
![]()
图 7 两种模型在南半球高纬区的ZTD预测结果对比
Figure 7. Comparison of ZTD Prediction Results for Two Models in High Latitude Areas of the Southern Hemisphere
![]()
图 8 GP模型预测结果与原始数据及其线性拟合结果
Figure 8. Prediction Results of GP Model and Original Data and Their Linear Fitting Results
![]()
图 10 GP模型对不同海拔地区ZTD的预测结果
Figure 10. Prediction Results of ZTD at Different Altitude Areas Using GP Model
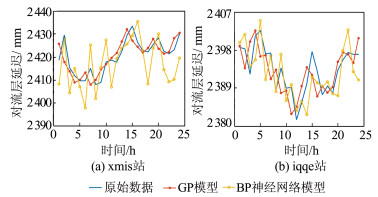
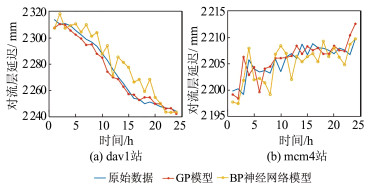
表 1 12个IGS站点的纬度值
Table 1 Latitude Values for 12 IGS Sites
站点 纬度 pbri 11.6°N hnlc 21.3°N hnpt 38.5°N lama 53.9°N invk 68.3°N nyal 78.9°N xmis 10.4°S iqqe 20.3°S mobs 37.8°S parc 53.1°S dav1 68.6°S mcm4 77.8°S 
下载: 导出CSV
表 2 北半球两种模型预测结果的RMSE和MRE值
Table 2 RMSE and MRE of Prediction Results of Two Models in the Northern Hemisphere
纬度地区 站点 RMSE/mm MRE/% GP模型 BP模型 GP模型 BP模型 低纬 pbri 9.340 10.812 0.256 0.279 hnlc 8.793 10.213 0.287 0.350 中纬 hnpt 7.435 12.262 0.221 0.397 lama 11.348 39.822 0.361 1.100 高纬 invk 2.960 5.108 0.098 0.146 nyal 3.096 4.635 0.102 0.161
下载: 导出CSV
表 3 南半球两种模型预测结果的RMSE和MRE值
Table 3 RMSE and MRE of Prediction Results of Two Models in the Southern Hemisphere
纬度地区 站点 RMSE/mm MRE/% GP模型 BP模型 GP模型 BP模型 低纬 xmis 4.322 10.413 0.138 0.368 iqqe 4.096 5.817 0.143 0.201 中纬 mobs 3.127 4.491 0.103 0.156 parc 4.522 5.644 0.157 0.185 高纬 dav1 3.557 10.514 0.127 0.361 mcm4 2.271 2.610 0.073 0.101
下载: 导出CSV
表 4 北半球各站点的昼夜平均绝对误差/mm
Table 4 Mean Absolute Errors of Day and Night at All Stations in the Northern Hemisphere/mm
站点 白天 晚上 pbri 5.90 7.73 hnlc 7.55 6.82 hnpt 3.79 7.56 lama 9.03 8.51 invk 2.56 2.18 nyal 2.76 2.11
下载: 导出CSV
表 5 南半球各站点的昼夜平均绝对误差/mm
Table 5 Mean Absolute Errors of Day and Night at All Stations in the Southern Hemisphere/mm
站点 白天 晚上 xmis 4.01 2.69 iqqe 2.58 4.26 mobs 2.93 1.99 parc 3.90 3.36 dav1 3.68 2.10 mcm4 2.11 1.12
下载: 导出CSV
表 6 不同经度地区ZTD的预测结果
Table 6 Prediction Results of ZTD at Different Longitude Areas
组别 站点 经度 纬度 海拔/m 预测中误差/mm 第1组 nrc1 284.38°W 45.45°N 82.5 6.46 mikl 31.97°E 46.97°N 94.7 7.98 第2组 chan 125.44°E 43.79°N 268.3 10.11 tlse 1.48°E 43.56°N 207.2 9.17 第3组 iqqe 289.87°W 20.27°S 38.9 4.10 nium 190.07°W 19.08°S 90.1 5.33
下载: 导出CSV
-
[1] 张超, 戴吾蛟, 石强, 等.电离层延迟对单频GPS点的影响及改正方法研究[J].武汉大学学报·信息科学版, 2018, 43(3):471-477 doi: 10.13203/j.whugis20150546 Zhang Chao, Dai Wujiao, Shi Qiang, et al.Influence of Ionosphere Delay on Single Frequency GPS Point and Its Correction Method[J].Geomatics and Information Science of Wuhan University, 2018, 43(3):471-477 doi: 10.13203/j.whugis20150546
[2] 吉绪发, 卢辰龙.GPS多路径误差滤波方法比较研究[J].测绘通报, 2015(4):10-13 https://www.cnki.com.cn/Article/CJFDTOTAL-CHTB201504004.htm Ji Xufa, Lu Chenlong.Comparing Different Filtering Methods for Mitigation of GPS Multipath Error[J].Bulletin of Surveying and Mapping, 2015(4):10-13 https://www.cnki.com.cn/Article/CJFDTOTAL-CHTB201504004.htm
[3] 王勇, 张立辉, 杨晶.基于BP神经网络的对流层延迟预测研究[J].大地测量与地球动力学, 2011, 31(3):134-137 https://www.cnki.com.cn/Article/CJFDTOTAL-DKXB201103032.htm Wang Yong, Zhang Lihui, Yang Jing.Study on Prediction of Zenith Tropospheric Delay by Use of BP Neural Network[J].Journal of Geodesy and Geodynamics, 2011, 31(3):134-137 https://www.cnki.com.cn/Article/CJFDTOTAL-DKXB201103032.htm
[4] 李剑锋, 王永前, 郭俊元.预测模型在对流层延迟计算中的应用研究[J].测绘科学技术学报, 2015, 32(5):450-454 doi: 10.3969/j.issn.1673-6338.2015.05.003 Li Jianfeng, Wang Yongqian, Guo Junyuan.Research on Tropospheric Delay Calculation with Prediction Model[J].Journal of Geomatics Science and Technology, 2015, 32(5):450-454 doi: 10.3969/j.issn.1673-6338.2015.05.003
[5] 吕慧珠, 黄文德, 闻德保.一种基于频谱分析和AR补偿的对流层延迟预报模型[J].大地测量与地球动力学, 2015, 35(2):283-286 https://www.cnki.com.cn/Article/CJFDTOTAL-DKXB201502026.htm Lü Huizhu, Huang Wende, Wen Debao.A Tropospheric Delay Prediction Model Based on Spectrum Analysis and the AR Compensation[J].Journal of Geodesy and Geodynamics, 2015, 35(2):283-286 https://www.cnki.com.cn/Article/CJFDTOTAL-DKXB201502026.htm
[6] 尹为松, 陶庭叶, 邓清军, 等.遗传算法优化的GPS对流层延迟内插算法[J].测绘科学, 2016, 41(1):180-184 https://www.cnki.com.cn/Article/CJFDTOTAL-CHKD201601035.htm Yin Weisong, Tao Tingye, Deng Qingjun, et al.Interpolation Algorithm of GPS Tropospheric Delay Based on GA-BP[J].Science of Surveying and Mapping, 2016, 41(1):180-184 https://www.cnki.com.cn/Article/CJFDTOTAL-CHKD201601035.htm
[7] 任超, 刘中流, 梁月吉, 等.基于EEMD-SARIMA的对流层延迟预测模型研究[J].大地测量与地球动力学, 2018, 38(9):953-957 https://www.cnki.com.cn/Article/CJFDTOTAL-DKXB201809014.htm Ren Chao, Liu Zhongliu, Liang Yueji, et al.Research on Tropospheric Delay Prediction Model Based on EEMD-SARIMA[J].Journal of Geodesy and Geodynamics, 2018, 38(9):953-957 https://www.cnki.com.cn/Article/CJFDTOTAL-DKXB201809014.htm
[8] 肖恭伟, 欧吉坤, 刘国林, 等.基于改进的BP神经网络构建区域精密对流层延迟模型[J].地球物理学报, 2018, 61(8):3 139-3 148 https://www.cnki.com.cn/Article/CJFDTOTAL-DQWX201808004.htm Xiao Gongwei, Ou Jikun, Liu Guolin, et al.Construction of a Regional Precise Tropospheric Delay Model Based on Improved BP Neural Network[J].Chinese Journal of Geophysics, 2018, 61(8):3 139-3 148 https://www.cnki.com.cn/Article/CJFDTOTAL-DQWX201808004.htm
[9] 张艳兰, 栾元重, 尹燕运, 等.混沌时间序列相空间重构及特性识别[J].测绘科学, 2016, 41(4):15-18 https://www.cnki.com.cn/Article/CJFDTOTAL-CHKD201604004.htm Zhang Yanlan, Luan Yuanzhong, Yin Yanyun, et al.The Deformation Monitoring of Chaotic Time Series Phase Space Reconstruction and Feature Recognition[J].Science of Surveying and Mapping, 2016, 41(4):15-18 https://www.cnki.com.cn/Article/CJFDTOTAL-CHKD201604004.htm
[10] Takens F.Detecting Strange Attractors in Turbulence[J].Lecture Notes in Mathematics, 1981, 898:366-381
[11] 王建民, 张锦.基于高斯过程回归的变形智能预测模型及应用[J].武汉大学学报·信息科学版, 2018, 43(2):248-254 doi: 10.13203/j.whugis20160075 Wang Jianmin, Zhang Jin.Deformation Intelligent Prediction Model Based on Gaussian Process Regression and Application[J].Geomatics and Information Science of Wuhan University, 2018, 43(2):248-254 doi: 10.13203/j.whugis20160075
[12] 李军, 张友鹏.基于高斯过程的混沌时间序列单步与多步预测[J].物理学报, 2011, 60(7):143-152 https://www.cnki.com.cn/Article/CJFDTOTAL-WLXB201107021.htm Li Jun, Zhang Youpeng.Single-Step and Multiple-Step Prediction of Chaotic Time Series Using Gaussian Process Model[J].Acta Physica Sinica, 2011, 60(7):143-152 https://www.cnki.com.cn/Article/CJFDTOTAL-WLXB201107021.htm
[13] Rasmussen C E, Williams C K I.Gaussian Processes for Machine Learning[M].Massachusetts:The MIT Press, 2006
[14] Cao L.Practical Method for Determining the Minimum Embedding Dimension of a Scalar Time Series[M].Amsterdam:Elsevier Science Publishers, 1997
[15] 陈永潮.北半球区域对流层延迟模型研究[D].南京: 东南大学, 2017 Chen Yongchao.Research on Tropospheric Delay Model in the Northern Hemisphere[D].Nanjing: Southeast University, 2017
[16] 黄良珂, 刘立龙, 文鸿雁, 等.亚洲地区EGNOS天顶对流层延迟模型单站修正与精度分析[J].测绘学报, 2014, 43(8):808-817 https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB201408007.htm Huang Liangke, Liu Lilong, Wen Hongyan, et al.Single-Site Improvement and Accuracy Analysis for Zenith Tropospheric Delay of EGNOS Model over Asia Area[J].Acta Geodaetica et Cartographica Sinica, 2014, 43(8):808-817 https://www.cnki.com.cn/Article/CJFDTOTAL-CHXB201408007.htm
-
期刊类型引用(3)
1. 刘春科，徐工，石志敏，张英琦，王辰晨. 基于GNSS天线阵列的定姿方法研究. 全球定位系统. 2022(04): 113-121 . 
百度学术
2. 韦永僧，贺凯飞，邱立杰，刘笃学. 陆地导航中GNSS单天线姿态测量的误差及精度分析. 大地测量与地球动力学. 2021(10): 1014-1017+1050 . 百度学术
3. 杨鸿毅，王潜心，胡超，毛亚，柴大帅. GPS/BDS组合多天线定姿与精度分析. 合肥工业大学学报(自然科学版). 2020(06): 818-822+854 . 百度学术
其他类型引用(6)
计量
- 文章访问数:
- HTML全文浏览量:
- PDF下载量:
- 被引次数: 9
