-
摘要: 尺度在地学中无处不在,地理信息科学也不例外。自Abler 1987年将尺度列为地理信息的几个重要科学问题之一,30年来,各国学者为尺度问题做了大量的研究工作。试图对这30年的研究作一简单的回顾,明析已解决的部分问题和尚需进一步努力的方向。讨论的重点是Goodchild等提出的5大关键问题,即尺度不变量、改变尺度的能力、尺度效应的度量、尺度作为过程模型中的参数及多尺度方法的实施。通过讨论发现,尺度效应得到了很好的研究,但主要是针对数据尺度,多种尺度(如数据尺度和分析尺度)的联合效应研究仍然严重贫乏;现在有很多转换模型可供使用,但是有些方法的理论基础不够完美;"尺度作为过程模型中的参数"这一问题最任重道远;尺度变换引起的信息量变化缺乏系统研究。这些问题需要引起高度的重视。Abstract: Scale is everywhere in all geosciences, with geographic information science being unexceptional. It has been 30 years since Abler published an article in 1987 which lists scale as one of several key research topics in geographic information science. Over the past 30 years, a lot of researches on this topic has been carried out by researchers from different countries. It seems to be appropriate time to have a close examination into the issues of this topic. This paper attempts to make a brief review of these researches. The discussions will be focused on the five key issues raised by Goodchild and his collaborators, i.e., scale invariants, ability to change scale, measure of the impact of scale, scale as a parameter in process models and implementation of multiscale approaches. It has been found that the impact of scale has been well studied, but the joint effect of scales at multiple stages (e.g., data scale and analysis scale) is still poorly investigated; various transformation models have become available, but the theoretical basis of some methods is not sound enough; the issue of "scale as a parameter in process model" has a long way to go. It is also noted that the effect of scale on information is rarely touched. All these issues deserve great attention from geo-information community.
-
Keywords:
- geographic information science /
- scale issue /
- scale-driven /
- multi-scale
-
1 尺度:地理信息科学中的关键主题之一
尺度在地学领域中是一个比较古老的科学问题。在地理学中,可塑性面积单元问题(modifiable areal unit problem, MAUP)早在1934年就由Gehlke等[1]提出。自Openshaw[2]于1984年发表《The Modifiable Areal Unit Problem》后,这个问题受到越来越广泛的关注。在地图学中,地图综合是一个地图的尺度问题。从文献[3]中可知,早在1866年,Emil von Sydow就对地图综合作了定义[4]。自20世纪60年代中起,地图综合进入了数字化时代。如何从最近更新的大比例尺地图中通过地图综合来自动更新小比例尺地图是现阶段的一个热门课题。
事实上,在泛地学领域中,尺度无处不在。也就是说,所有地学科学中都存在着类似的尺度问题,如地形学[5]、海洋学[6]、土壤学[7]、生物学[8]、社会科学[9]、水文学[10]、环境科学[11]等等。
在地理信息科学领域,Abler 1987年发表的文章中[12]介绍道,早在1983年,美国国家航空和宇宙航行局(National Aeronautics and Space Administration,NASA)召集部分著名学者讨论关键科学问题,空间数据的多尺度表达是其中之一;而且多尺度表达已被列入该研究中心(80年代初成立)的首批12个课题中。在遥感领域,Woodcock等[13]也于1987年发表了该领域的第一篇关于尺度的文章,文中通过实验分析了尺度对影像信息表达的影响。
本文认为,文献[12]吹响了地理信息科学领域尺度问题研究的进军号,具有非常重要的历史意义。此后,多尺度表达便成为国际地理信息学界的研究热点[14],欧盟自然科学基金会也将多尺度表达和地图综合列为主要研究课题[15];世界各国(加拿大、中国、英国、法国、德国、荷兰、瑞典、瑞士等)都对尺度问题极为重视。尺度问题也受到有关国际学术组织的特别关注,国际摄影测量与遥感学会(International Society for Photogrammetry and Remote Sensing,ISPRS)于2000年设立空间数据多尺度表达工作组;国际地图制图学会(International Cartographic Association,ICA)于2003年将地图综合自动化工作组升级为委员会。美国的大学地理信息科学联合会(University Consortium for Geographic Information Science, UCGIS)一直将尺度列为重点研究课题之一[16],其最新推荐的课题包括空间本体论(spatial ontologies)、地理表达(geographic representation)、空间数据获取与集成(spatial data acquisition and integration)、尺度(scale)、空间认知(spatial cognition)、空间及时空分析与建模(space and space/time analysis and modeling)、地理信息的不确定性(uncertainty in geographic information)、可视化(visualization)、地理信息与社会(GIS and society)以及地理信息工程,包括分布式计算、空间信息基础设施、空间数据挖掘等。
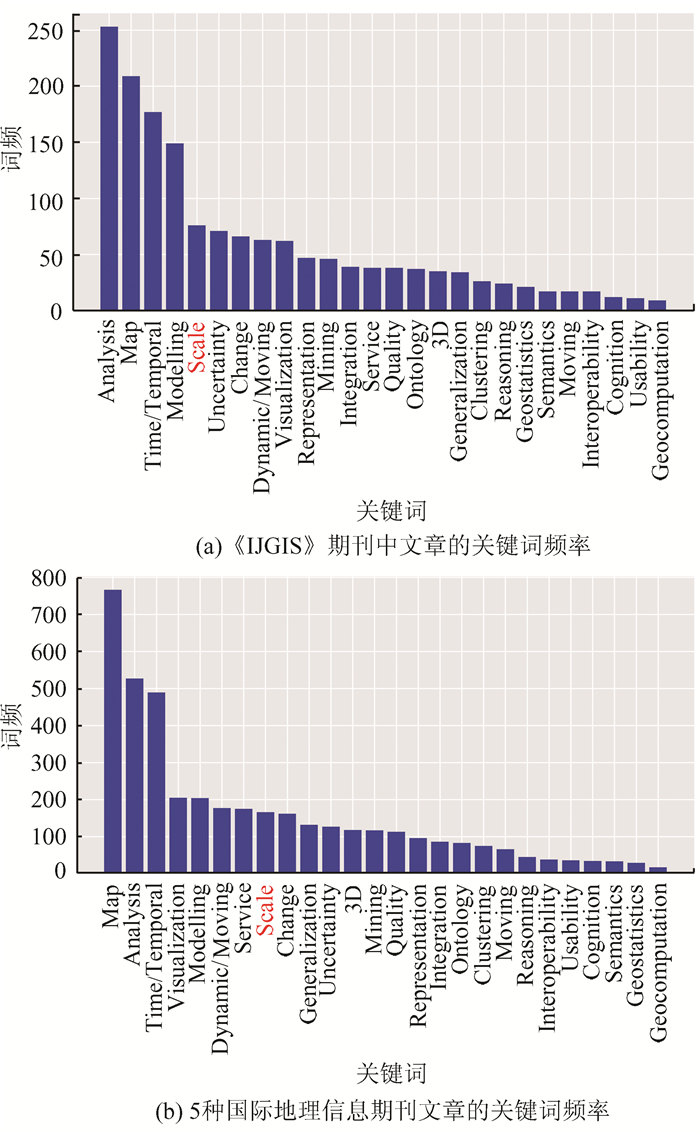
自1987年以来,关于尺度的文章不断涌现,而尺度至今仍然是地理信息领域的热点问题。图 1是对1987-2017年这30年在地理信息领域的主要国际期刊发表的文章题目中关键词做的统计。这些期刊包括《International Journal of Geographical Information Science》(IJGIS)《ISPRS International Journal of Geo-Information》《Transactions in GIS》《GeoInformatica》及《Cartography and Geographic Information Science》。这些关键词源于UCGIS的研究议程及ISPRS的地理信息有关委员会的研究内容。
自1996年以来,国际上关于尺度研究的专辑和专著也不断涌现。专辑如《Scaling up in Hydrology Using Remote Sensing》[17]《Scale in Remote Sensing and GIS》[18]《Scale Dependence and Scale Invariance in Hydrology》[19]《Modelling Scale in Geographical Information Science》[20]《Scale and Geographic Inquiry: Nature, Society, and Method》[21]《Generalization of Geogra-phic Information: Cartographic Modelling and Applications》[22]《Scale Issues in Remote Sen-sing》[23]《Integrating Scale in Remote Sensing and GIS》[24],专著如《Algorithmic Foundation of Multi-scale Spatial Representation》[25]《Scale in Spatial Information and Analysis》[26]。
近年来,国内关于尺度的研究也十分活跃。如艾廷华等[27]提出了4种空间数据的多尺度表达的技术策略;刘学军等[28]、汤国安等[29]对数字高程模型中的尺度问题进行了综述;刘凯等[30]探讨了地理信息尺度的概念及转换; 李小文等[31]分别对尺度问题和遥感中的尺度问题进行了综述;邓敏等[32]提出了一种融合尺度特征的预测方法;李霖等[33]就空间尺度的基础性问题展开研究;李德仁等[34]认为在地理国情监测中,尺度是关键参数之一。也有地理信息尺度相关的论文集面世,如《数字地图综合进展》[35]。特别值得一提的是北京师范大学地理学与遥感科学学院于2014年2月21日召开的“遥感尺度效应与尺度转换”学术研讨会。会上,李小文、刘昌明、傅伯杰等十几位学者各自发表了对尺度问题的见解。如刘昌明提出“研究尺度不一样,分析结果就不一样。地理现象在一定比例尺上表现的信息量是不一样的”;傅伯杰指出“地理现象在时间、空间尺度的表征,随着观测时空分辨率的变化,获得的信息也在变化;不同的研究对象有其对应的时间空间尺度,需要研究与发现其时空尺度特征;观测尺度与地理现象的时空尺度要匹配”[36]。
综上所述,自Abler于1987年将尺度列为地理信息的几个重要科学问题之一到今天,已经过了30年。本文试图对地理信息科学中尺度问题研究之30年作一简单的回顾。
2 地理信息科学中尺度研究的4个主要方面
通常来说,地理信息科学中尺度问题的内容包括尺度本体、尺度变换、尺度效应及最佳尺度等4个主要方面。实际上每个领域对这4个方面的侧重点有所不同。比如地图综合的侧重点是尺度变换,即怎样将大比例尺地图变换成小比例尺的地图。
针对这4个方面的研究,许多学者做了综述,如孟斌和王劲峰[37]、Li[4]、孙庆先等[38]、Marceau和Hay[39]、李小文等[31]、Wu和Li [40]、Achanta等[41]、Im等[42]、杜世宏等[43]。§1所列的专辑及专著也对这些问题做了系统的总结。
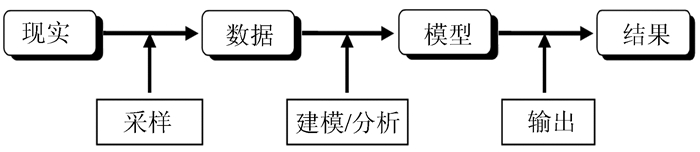
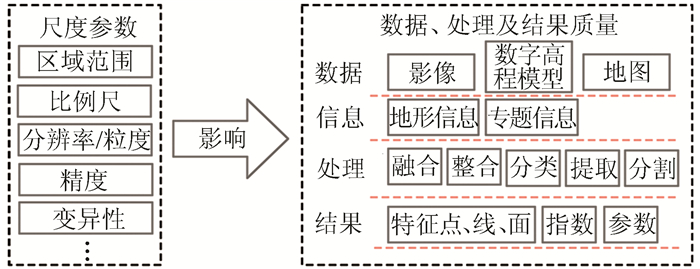
首先讨论尺度的内涵。尺度一词的含义非常广泛。Quattrochi和Goodchild[44]指出,在一定程度上讲,在所有具有科学意义的术语中,尺度定义最不清并且内容最超载;尺度是一个令人困惑的概念,经常被误解,并且根据背景和学科观点意味着不同的事物。因此,李志林[45]认为,尺度这一概念无法用单一参数来表示,需要用一组参数来表达,如区域的范围大小、地图比例尺、分辨率/粒度、精度和变异性等;同时,他还认为,在整个地理信息处理过程中,从现实、数据、模型到结果,经历采样、建模(分析)及输出等操作(图 2),只有当各环节的尺度一致时,得到的结果才有意义,否则就会出现由于不匹配引起的笑话,如2 000 002年前的化石、2 m精度的全球数字高程模型、250 m格网的1:50 000数字高程模型等。
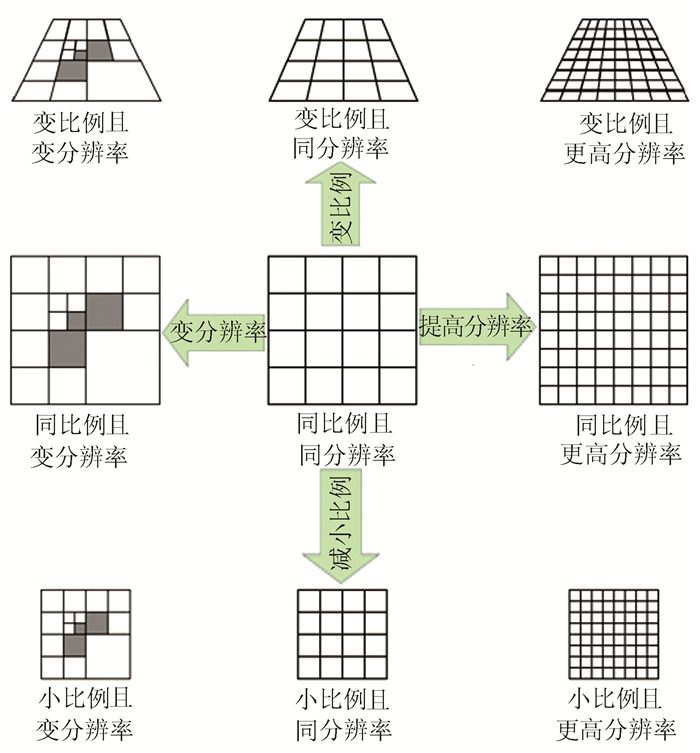
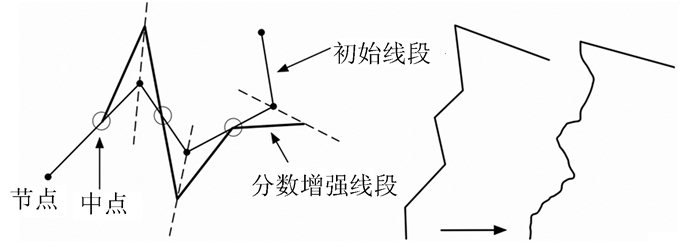
接下来讨论尺度变换。一个尺度由一个或多个尺度参数代表,任何一个或多个尺度参数的变化都会导致尺度变换。图 3中,可以通过改变比例尺(变比例尺与比例缩放)和分辨率(变分辨率与分辨率缩放)来实现9种尺度变换。根据尺度变换方向可以将其分为尺度上推和下推。尺度上推方法如小波变换[46]、傅里叶变换[47]、经验模态分解(emprical mode decomposition, EMD)[48-49]、重采样[50]、聚合[51-52]等。尺度下推方法如超分辨率或亚分辨率制图等[40]。但从本质上讲,尺度下推的方法基本上基于重采样理论,区别在于使用的先验知识和内插方法不同,如分形插值法[53]、克里金法(Kriging)[54]等。图 4是利用分数维来将线条尺度下推的示意图[55]。最近,基于机器学习的尺度变换开始流行[56]。同样地,尺度变换也会因精度或变异性的改变而发生,如平滑[57]、过滤[58]、特征保留[59]、融合[60]、压缩[61]等。
关于尺度效应的文章不断涌现,文章的内容可由图 5来概括。这里的尺度效应包括图 2中各环节的所有尺度参数对数据、处理过程以及结果呈现的影响。所以,这些文章包括有采样尺度对数据的影响、数据尺度对模型或结果的影响、分析尺度对模型或结果的影响、模型尺度对结果的影响等。典型例子如数字高程模型地图尺度和数据分辨率对基于地形的流域模型的影响[62]、模型分辨率对流域尺度亚热带大西洋模型中湾流预测结果的影响[63]、数字高程模型分析尺度对结果不确定性的规律[29]、城市土地利用变化模型中的尺度依赖性特征[64]、格网分辨率对土壤制图的影响[65]等。
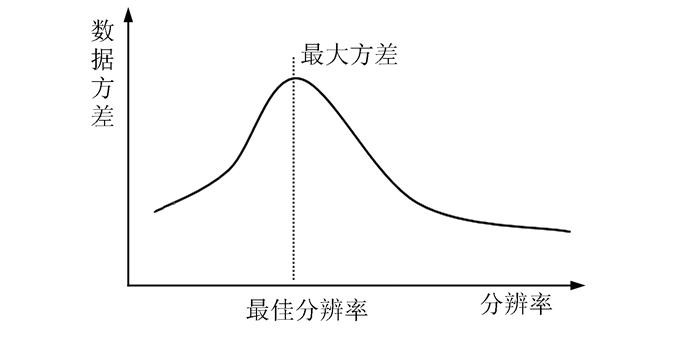
最佳尺度问题就是寻求尺度的最佳匹配,许多学者在这个问题上做了努力,已经有了许多方法,例如基于分形维数[66]、基于变异函数[67]、基于地理方差[68]、基于局部方差法[13]、基于信息熵[69-70]等。图 6是利用局部方差确定最佳尺度的示意图。这种方法的前提是,如果空间分辨率比区域中的对象精细得多,则大多数测量将与其邻居高度相关,因此局部方差将很低。反过来,如果所研究的物体大小接近分辨率,则这些测量值往往彼此不同,因此出现局部增大[71]。其他方法背后也有类似的基本假设。这些关于最佳尺度大部分是正对采样尺度或数据尺度,但也有许多学者开始研究最佳分析尺度[72-73]。
3 地理信息科学中尺度研究的5大关键问题
20年前,Goodchild等学者提出了尺度的5大关键问题。本文将以这5大问题为基础进行讨论。这5大问题的内容如下[18]。
1) 尺度不变量。什么尺度测度是数据中地理细节的不变特性,能在常规操作(如从模拟到数字转换或坐标变换)下保存下来,什么地理特性是尺度不变量。
2) 改变尺度的能力。有哪些类型的尺度变换可用于聚合或分解空间数据,并以一种合乎逻辑的、科学严谨的、理论坚实的方式进行;是否有可能开发出一套能符合人们对地球系统过程的理解的方式来分解粗粒度数据和聚合细粒度数据的通用方法。
3) 尺度效应的度量。是否有可能实现一些评估尺度变化影响的方法;过程的观察是如何受尺度变化影响的;如何能够衡量过程在不同尺度上的表现程度。
4) 尺度作为过程模型中的参数。尺度在参数化过程模型中如何表示;如何评估尺度参数对模型的影响。
5) 多尺度方法的实施。用集成工具来支持多尺度数据库以及相关建模和分析的潜力是什么;工具能否为多尺度数据提供兼容的框架;在整合不同尺度的数据时,哪些问题必须克服。
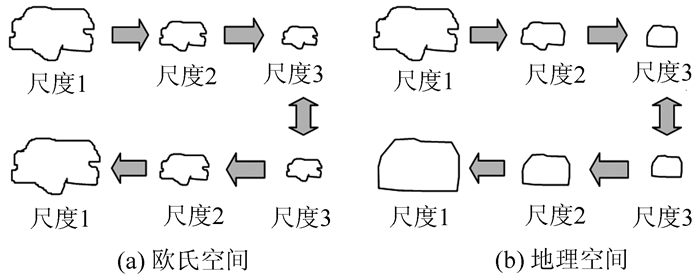
专门探讨尺度不变量的文献鲜有所见,但李志林[45]于2005年区别了欧氏空间与地理空间中的尺度问题,对寻找地理信息中的尺度不变量有所启发。在欧氏空间中,任何对象都相对于一个整数维,即一个点为0维,一条线为1维,一个平面为2维,一个体为3维。放大(或缩小)会导致2维空间中长度增大(或缩短)以及3维空间中体积增大(或缩小),但是对象的形状保持不变。图 7(a)是一个在2维欧氏空间中尺度缩小的例子,它表明这种变换是可逆的,因此基本的几何特性都是不随尺度变化而变化的。但是,地理空间中,量测不同比例尺地图上的同一条海岸线会得到不同的长度值[74]。事实上,在较小比例尺时,对象的复杂程度被减小(对应于小比例尺时观察到的地理目标)。这时,许多特性随尺度变化而变化。但当对象的表达从小比例尺下放大到原始尺寸时,其复杂程度却不能恢复。图 7(b)说明了在地理空间中尺度的增加与缩小,它表明这种变换是不可逆的。
![]() 图 7 二维空间的尺度缩放示意图[45]Figure 7. Scaling in 2D Spaces: Euclidean and Geographical
图 7 二维空间的尺度缩放示意图[45]Figure 7. Scaling in 2D Spaces: Euclidean and Geographical因此,分数维的概念被引入[74],即地理空间中维数并不是整数。在此空间内,一条线的维数在1.0~2.0之间,一个面的维数在2.0~3.0之间。因此可以推理,分数维是地理空间的一个尺度不变量。事实上,Xia等[66]一直建议用分数维来处理地理空间多尺度的问题,并取得了良好的效果。在影像的处理和分析中,常用到尺度不变特征,这种特征通过探测某种尺度空间的响应极值来对尺度变换保持不变,因此这类特征也是一种尺度不变量[75]。除此之外,还有许多问题值得思考,如是否存在其他尺度不变量;以及许多学者在尺度下推时利用了变异函数(variogram),变异函数是否是尺度不变量。
有关改变尺度的能力,在§2中提到,现在已有许多尺度变换可用于聚合或分解空间数据。也有人对尺度变换方法的影响做了系统的评估[70-76],但还没有见到对这些方法的科学严谨性和理论坚实性的系统研究。如变异函数已被用作尺度下推的理论基础,如果变异函数不是尺度不变量,那么在尺度下推时利用了变异函数是否合适。在聚合时,常用的窗口大小为3×3,3×3的理论基础是什么。在聚合数值数据时,聚合结果通常取窗口内的所有数值的某种统计值,为什么取这种统计值等。
对于改变尺度,至今仍难以全局把握,上下贯通。这是因为,一方面,尺度下推与上推的方法是分而治之的,因而没有形成一套符合人们对地理系统过程的理解方式来分解粗粒度数据和聚合细粒度数据的通用方法。另外,由于处理对象特征的差异性,很难说采用某种或如是否某一类方法来改变尺度就能达到目的。虽然现在机器学习中深度学习以模拟的方式实现多尺度转换取得了不错的结果,但是其仍然是个灰箱模型,缺乏理论基础,难以推广。
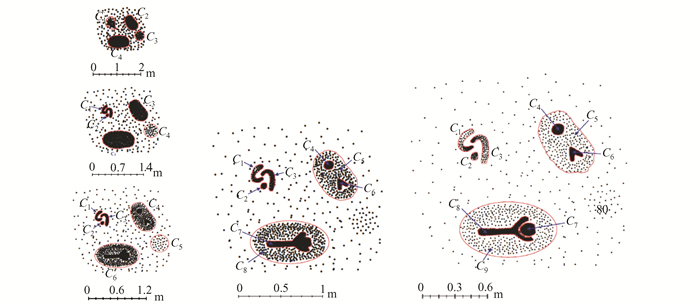
有关尺度作为过程模型中的参数,在大多数的尺度变换方法中,既没有直接将尺度作为模型参数,也没有通过尺度来计算模型参数。因此,这些方法虽然达到一定的效果,但所得结果的对应尺度是不明确的,包括那些基于尺度空间的方法。事实上,在基于尺度空间的方法中,模型的尺度参数是高斯函数中的σ,但是σ通常根据经验设定,而不是根据真实尺度计算而得,这样导致尺度结果无从理解。事实上,李志林等[77]一直呼吁基于自然法则的尺度驱动的变换方法,使结果与尺度直接挂钩[4, 78],在最近提出的尺度驱动的聚类理论就是一个这样的例子(图 8)[72]。
有关尺度效应的度量,在§2中提到,尺度的效应是研究最多的一个领域。学者们研究了各种尺度的效应以及评估方法,但是目前并没有一个统一的尺度效应理论。尺度变换引起的信息量变化还缺乏系统的研究。还有对多种尺度(如数据尺度和分析尺度)联合效应的研究也很少见。
有关多尺度方法的实施,目前虽然有许多多尺度分析的工具,但对这些工具的理论基础缺乏系统的研究。李志林等[77-80]一直坚持自然法则可以成为多尺度分析工具的理论基础,这样多尺度分析就会客观化。在整合不同尺度的数据及进行尺度变化时,信息量的变化是一个很少涉及的问题,只有极少几个学者[26, 79, 81]对这个问题进行了探讨。
4 地理信息科学中尺度研究的展望
总结文献可以发现,20年前,Goodchild等提出了尺度的5大问题,至今有些关键问题仍然缺乏完美的答案。
1) 对尺度和尺度问题性质的理解仍然不够深入;
2) 尺度效应得到了很好的研究,但主要是针对数据尺度,多种尺度(如数据尺度和分析尺度)的联合效应研究仍然严重贫乏;同时尺度变换引起的信息量变化缺乏系统研究,需要引起高度重视;
3) 现在有很多转换模型可供使用,但是将尺度作为模型中的显式参数仍显不足,有些方法的理论基础不够完美;
4) 多尺度的分析方法很多,但当中许多方法仍然缺乏严密的理论基础。
尺度无疑是地理信息科学中最基础的研究之一[18]。Cola [82]认为,尺度在空间数据的分析中是如此普遍的问题,以至于需要将它提升到基本维度的层面,与空间、时间和专题并列。这个看法类似于李志林之前所表达的观点[78, 81-82],但角度不同。并且Quattrochi与Goodchild[18]在其编写的专辑结语中也重复了类似的观点,当尺度是一个基础和不可避免的地理数据维度。因此,尺度是地理信息科学中的基本问题[83],尺度问题研究仍然需要大家努力。
-
图 7 二维空间的尺度缩放示意图[45]
Figure 7. Scaling in 2D Spaces: Euclidean and Geographical
-
[1] Gehlke C E, Biehl K. Certain Effects of Grouping upon the Size of the Correlation Coefficient in Census Tract Material[J]. Journal of the American Statistical Association, 1934, 29(185A):169-170 doi: 10.1080/01621459.1934.10506247
[2] Openshaw S. The Modifiable Areal Unit Problem[J]. Concepts and Techniques in Modern Geography, 1984, 38:41 http://d.old.wanfangdata.com.cn/OAPaper/oai_pubmedcentral.nih.gov_3245430
[3] Cebrykow P. Cartographic Generalization Yesterday and Today[J]. Polish Cartographical Review, 2017, 49(1):5-15 doi: 10.1515/pcr-2017-0001
[4] Li Zhilin. Digital Map Generalization at the Age of Enlightenment:A Review of the First Forty Years[J]. The Cartographic Journal, 2007, 44(1):80-93 doi: 10.1179/000870407X173913
[5] de Boer D H. Hierarchies and Spatial Scale in Process Geomorphology:A Review[J]. Geomorphology, 1992, 4(5):303-318 doi: 10.1016/0169-555X(92)90026-K
[6] Stommel H. Varieties of Oceanographic Experience[J]. Science, 1963, 139(3555):572-576 doi: 10.1126/science.139.3555.572
[7] Hillel D, Elrick D E. Scaling in Soil Physics:Principles and Applications[M]. Madison:Soil Science Society of America, 1990
[8] Haury L R, Mcgowan J A, Wiebe P H. Patterns and Processes in the Time-Space Scales of Plankton Distributions[M]//Steele J H. Spatial Pattern in Plankton Communities. Berlin: Springer, 1978: 277-327
[9] Dovers S R. A Framework for Scaling and Framing Policy Problems in Sustainability[J]. Ecological Economics, 1995, 12(2):93-106 doi: 10.1016/0921-8009(94)00042-T
[10] Blöschl G, Sivapalan M. Scale Issues in Hydrological Modelling:A Review[J]. Hydrological Processes, 1995, 9(3-4):251-290 doi: 10.1002/(ISSN)1099-1085
[11] Bian L. Multiscale Nature of Spatial Data in Scaling up Environmental Models[M]//Quattrochi D A, Goodchild M E. Scale in Remote Sensing and GIS. Florida, USA: CRC Press, 1997
[12] Abler R F. The National Science Foundation National Center for Geographic Information and Analysis[J]. International Journal of Geographical Information System, 1987, 1(4):303-326 doi: 10.1080/02693798708927819
[13] Woodcock C E, Strahler A H. The Factor of Scale in Remote Sensing[J]. Remote Sensing of Environment, 1987, 21(3):311-332 doi: 10.1016/0034-4257(87)90015-0
[14] Rhind D. A GIS Research Agenda[J]. International Journal of Geographical Information System, 1988, 2(1):23-28 doi: 10.1080/02693798808927876
[15] Arnaud A M, Craglia M, Masser I, et al. The Research Agenda of the European Science Foundation's GIS Data Scientific Programme[J]. International Journal of Geographical Information Science, 1993, 7(5):463-470 doi: 10.1080/02693799308901975
[16] Elmes G. The UCGIS Research Agenda-Driving Forward by Locating Challenges[OL]. https://www.directionsmag.com/article/3489, 2004
[17] Stewart J B. Scaling up in Hydrology Using Remote Sensing[M]. New Jersey:John Wiley and Sons, 1996
[18] Quattrochi D A, Goodchild M F. Scale in Remote Sensing and GIS[M]. Florida:CRC Press, 1997
[19] Sposito G. Scale Dependence and Scale Invariance in Hydrology[M]. Cambridge:Cambridge University Press, 2008
[20] Tate N, Atkinson P M. Modelling Scale in Geographical Information Science[M]. New Jersey:John Wiley and Sons, 2001
[21] Sheppard E, Mcmaster R B. Scale and Geographic Inquiry:Nature, Society, and Method[M]. New Jersey:John Wiley and Sons, 2008
[22] Mackaness W A, Ruas A, Sarjakoski L T. Genera-lisation of Geographic Information:Cartographic Modelling and Applications[M]. Amsterdam, Holland:Elsevier, 2011
[23] Wang Q. Scale Issues in Remote Sensing[M]. New Jersey:John Wiley and Sons, 2014
[24] Quattrochi D A, Wentz E, Lam N S N, et al. Integrating Scale in Remote Sensing and GIS[M]. Florida:CRC Press, 2017
[25] Li Zhilin. Algorithmic Foundation of Multi-scale Spatial Representation[M]. Florida:CRC Press, 2006
[26] Zhang J, Atkinson P M, Goodchild M F. Scale in Spatial Information and Analysis[M]. Florida:CRC Press, 2014
[27] 艾廷华, 成建国.对空间数据多尺度表达有关问题的思考[J].武汉大学学报·信息科学版, 2005, 30(5):377-382 http://ch.whu.edu.cn/CN/abstract/abstract2176.shtml Ai Tinghua, Cheng Jianguo. Key Issues of Multi-scale Representation of Spatial Data[J]. Geomatics and Information Science of Wuhan University, 2005, 30(5):377-382 http://ch.whu.edu.cn/CN/abstract/abstract2176.shtml
[28] 刘学军, 张平. DEM坡度、坡向的有效尺度范围[J].武汉大学学报·信息科学版, 2008, 33(12):1254-1258 http://ch.whu.edu.cn/CN/abstract/abstract1774.shtml Liu Xuejun, Zhang Ping. Effective Scale of Slope and Aspect Derived from Grid-Based Digital Elevation Model[J]. Geomatics and Information Science of Wuhan University, 2008, 33(12):1254-1258 http://ch.whu.edu.cn/CN/abstract/abstract1774.shtml
[29] 汤国安, 刘学军, 房亮, 等. DEM及数字地形分析中尺度问题研究综述[J].武汉大学学报·信息科学版, 2006, 31(12):1059-1066 http://ch.whu.edu.cn/CN/abstract/abstract2613.shtml Tang Guoan, Liu Xuejun, Fang Liang, et al. A Review on the Scale Issue in DEMs and Digital Terrain Analysis[J]. Geomatics and Information Science of Wuhan University, 2006, 31(12):1059-1066 http://ch.whu.edu.cn/CN/abstract/abstract2613.shtml
[30] 刘凯, 毋河海, 艾廷华, 等.地理信息尺度的三重概念及其变换[J].武汉大学学报·信息科学版, 2008, 33(11):1178-1181 http://ch.whu.edu.cn/CN/abstract/abstract1746.shtml Liu Kai, Wu Hehai, Ai Tinghua, et al. Three-Tiered Concepts of Scale of Geographical Information and Its Transformation[J]. Geomatics and Information Science of Wuhan University, 2008, 33(11):1178-1181 http://ch.whu.edu.cn/CN/abstract/abstract1746.shtml
[31] 李小文, 曹春香, 张颢.尺度问题研究进展[J].遥感学报, 2009, 13(s1):12-20 http://d.old.wanfangdata.com.cn/Periodical/dqkxjz200104016 Li Xiaowen, Cao Chunxiang, Zhang Hao. Progress in Scale Issues[J]. Journal of Remote Sensing, 2009, 13(s1):12-20 http://d.old.wanfangdata.com.cn/Periodical/dqkxjz200104016
[32] 邓敏, 陈倜, 杨文涛.融合空间尺度特征的时空序列预测建模方法[J].武汉大学学报·信息科学版, 2015, 40(12):1625-1632 http://ch.whu.edu.cn/CN/abstract/abstract3391.shtml Deng Min, Chen Ti, Yang Wentao. A New Method of Modeling Spatio-Temporal Sequence by Conside-ring Spatial Scale Characteristics[J]. Geomatics and Information Science of Wuhan University, 2015, 40(12):1625-1632 http://ch.whu.edu.cn/CN/abstract/abstract3391.shtml
[33] 李霖, 应申.空间尺度基础性问题研究[J].武汉大学学报·信息科学版, 2005, 30(3):199-203 http://ch.whu.edu.cn/CN/abstract/abstract2133.shtml Li Lin, Ying Shen. Fundamental Problem on Spatial Scale[J]. Geomatics and Information Science of Wuhan University, 2005, 30(3):199-203 http://ch.whu.edu.cn/CN/abstract/abstract2133.shtml
[34] 李德仁, 丁霖, 邵振峰.关于地理国情监测若干问题的思考[J].武汉大学学报·信息科学版, 2016, 41(2):143-147 http://ch.whu.edu.cn/CN/abstract/abstract3447.shtml Li Deren, Ding Lin, Shao Zhenfeng. Reflections on Issues in National Geographical Conditions Monitoring[J]. Geomatics and Information Science of Wuhan University, 2016, 41(2):143-147 http://ch.whu.edu.cn/CN/abstract/abstract3447.shtml
[35] 王家耀, 李志林, 武芳.数字地图综合进展[M].北京:科学出版社, 2011 Wang Jiayao, Li Zhilin, Wu Fang. Advances in Digital Map Generalization[M]. Beijing:Science Press, 2011
[36] 遥感学报编辑部. "遥感尺度效应和尺度转换"论坛简报[OL]. http://www.jors.cn/jrs/ch/reader/view_news.aspx?id=20140717-050026001, 2014 Editorial Department of Journal of Remote Sensing. Briefing of the "Remote Sensing Scale Effect and Scale Conversion" Forum[OL]. http://www.jors.cn/jrs/ch/reader/view_news.aspx?id=20140717050026001, 2014
[37] 孟斌, 王劲峰.地理数据尺度转换方法研究进展[J].地理学报, 2005, 60(2):277-288 doi: 10.3321/j.issn:0375-5444.2005.02.011 Meng Bin, Wang Jinfeng. A Review on the Methodology of Scaling with Geo-Data[J]. Acta Geographica Sinica, 2005, 60(2):277-288 doi: 10.3321/j.issn:0375-5444.2005.02.011
[38] 孙庆先, 李茂堂, 路京选, 等.地理空间数据的尺度问题及其研究进展[J].地理与地理信息科学, 2007, 23(4):53-56 doi: 10.3969/j.issn.1672-0504.2007.04.014 Sun Qingxian, Li Maotang, Lu Jingxuan, et al. Scale Issue and Its Research Progress of Geospatial Data[J]. Geography and Geo-Information Science, 2007, 23(4):53-56 doi: 10.3969/j.issn.1672-0504.2007.04.014
[39] Marceau D J, Hay G J. Remote Sensing Contributions to the Scale Issue[J]. Canadian Journal of Remote Sensing, 1999, 25(4):357-366 doi: 10.1080/07038992.1999.10874735
[40] Wu Hao, Li Zhilin. Scale Issues in Remote Sen-sing:A Review on Analysis, Processing and Mode-ling[J]. Sensors, 2009, 9(3):1768-1793 doi: 10.3390/s90301768
[41] Achanta R, Shaji A, Smith K, et al. SLIC Superpixels Compared to State-of-the-Art Superpixel Methods[J]. IEEE Transactions on Pattern Analy-sis and Machine Intelligence, 2012, 34(11):2274-2282 doi: 10.1109/TPAMI.2012.120
[42] Im J, Quackenbush L J, Li M, et al. Optimum Scale in Object-based Image Analysis[M]//Wen Qihao. Scale Issues in Remote Sensing. New Jersey: John Wiley and Sons, 2014: 197-214
[43] 杜世宏, 雒立群, 赵文智, 等.多尺度空间关系研究进展[J].地球信息科学学报, 2015, 17(2):135-146 http://d.old.wanfangdata.com.cn/Periodical/dqxxkx201502002 Du Shihong, Luo Liqun, Zhao Wenzhi, et al. Research Progress in Multi-scale Spatial Relations[J]. Geo-Information Science, 2015, 17(2):135-146 http://d.old.wanfangdata.com.cn/Periodical/dqxxkx201502002
[44] Quattrochi D A, Goodchild M F. Introduction: Scale, Multiscaling, Remote Sensing and GIS[M]//Quattrochi D A, Goodchild M F. Scale in Remote Sensing and GIS. Florida: CRC Press, 1997: 1-12
[45] 李志林.地理空间数据处理的尺度理论[J].地理信息世界, 2005, 3(2):1-5 doi: 10.3969/j.issn.1672-1586.2005.02.001 Li Zhilin. A Theoretical Discussion on the Scale Issue in Geospatial Data Handling[J]. Geomatics World, 2005, 3(2):1-5 doi: 10.3969/j.issn.1672-1586.2005.02.001
[46] Mallat S G. A Theory for Multiresolution Signal Decomposition:The Wavelet Representation[J]. IEEE Transactions on Pattern Analysis and Machine Intelligence, 1989, 11(7):674-693 doi: 10.1109/34.192463
[47] Lee S H, Lim S Y, Kim N, et al. Increasing the Storage Density of a Page-Based Holographic Data Storage System by Image Upscaling Using the PSF of the Nyquist Aperture[J]. Optics Express, 2011, 19(13):12053-12065 doi: 10.1364/OE.19.012053
[48] Chen S, Zhang R, Su H, et al. Scaling-up Transformation of Multisensor Images with Multiple Reso-lutions[J]. Sensors, 2009, 9(3):1370-1381 http://med.wanfangdata.com.cn/Paper/Detail/PeriodicalPaper_PM22573959
[49] Li Zhilin, Khoshelham K, Zheng Dawei, et al. Empirical Mode Decomposition (EMD) Transform for Spatial Analysis[M]//Li Z, Zhou Q, Kainz W, et al. Advances in Spatial Analysis and Decision Making. Florida: CRC Press, 2003: 19-30
[50] Kirchner M, Bohme R. Hiding Traces of Resampling in Digital Images[J]. IEEE Transactions on Information Forensics and Security, 2008, 3(4):582-592 doi: 10.1109/TIFS.2008.2008214
[51] Tan Shiteng, Xu Zhu, Ti Peng, et al. Morphology-Based Modeling of Aggregation Effect on the Patch Area Size for GlobeLand30 Data[J]. Transactions in GIS, 2018, 22(1):98-118 doi: 10.1111/tgis.12298/pdf
[52] Wu J, Shen W, Sun W, et al. Empirical Patterns of the Effects of Changing Scale on Landscape Metrics[J]. Landscape Ecology, 2002, 17(8):761-782 doi: 10.1023/A:1022995922992
[53] Kim G, Barros A P. Downscaling of Remotely Sensed Soil Moisture with a Modified Fractal Interpolation Method Using Contraction Mapping and Ancillary Data[J]. Remote Sensing of Environment, 2002, 83(3):400-413 doi: 10.1016/S0034-4257(02)00044-5
[54] Wang Q, Shi W, Atkinson P M, et al. Downsca-ling MODIS Images with Area-to-Point Regression Kriging[J]. Remote Sensing of Environment, 2015, 166:191-204 doi: 10.1016/j.rse.2015.06.003
[55] Dutton G H. Fractal Enhancement of Cartographic Line Detail[J]. The American Cartographer, 1981, 8(1):23-40 doi: 10.1559/152304081783948430
[56] Agrawal A. Application of Machine Learning to Computer Graphics[J]. IEEE Computer Graphics and Applications, 2018(4):93-96 http://ieeexplore.ieee.org/document/8402157/
[57] Shapiro R. Smoothing, Filtering, and Boundary Effects[J]. Reviews of Geophysics, 1970, 8(2):359-387 doi: 10.1029/RG008i002p00359
[58] Witkin A. Scale-Space Filtering: A New Approach to Multi-scale Description[C]. IEEE International Conference on Acoustics, Speech, and Signal Processing, San Diego, USA, 1984
[59] Zhang G X, Cheng M M, Hu S M. A Shape-Preserving Approach to Image Resizing[C].Computer Graphics Forum, Oxford, UK, 2009 http://www.researchgate.net/publication/220506024_A_Shape-Preserving_Approach_to_Image_Resizing
[60] Qian R, Lu Z. Different Focus Image Fusion Based on Multi-level Scaling[C]. The International Society for Optics and Photonics, USA, 2018
[61] Toderici G, Vincent D, Johnston N. Full Resolution Image Compression with Recurrent Neural Networks[C]. Computer Vision and Pattern Recognition, Maryland, USA, 2017 https://www.computer.org/csdl/proceedings/cvpr/2017/0457/00/0457f435-abs.html
[62] Wolock D M, Price C V. Effects of Digital Elevation Model Map Scale and Data Resolution on a Topography-Based Watershed Model[J]. Water Resources Research, 1994, 30(11):3041-3052 doi: 10.1029/94WR01971
[63] Hurlburt H E, Hogan P J. Impact of 1/8 to 1/64 Resolution on Gulf Stream Model-Data Comparisons in Basin-Scale Subtropical Atlantic Ocean Models[J]. Dynamics of Atmospheres and Oceans, 2000, 32(3-4):283-329 doi: 10.1016/S0377-0265(00)00050-6
[64] Jantz C A, Goetz S J. Analysis of Scale Dependencies in an Urban Land-Use-Change Model[J]. International Journal of Geographical Information Science, 2005, 19(2):217-241 doi: 10.1080/13658810410001713425
[65] Zhu A X, Burt J E, Smith M. Neighborhood Size of Terrain Derivatives and Its Impact on Digital Soil Mapping[C].The International Symposium on Terrain Analysis and Digital Terrain Modelling (TADTM 2006), Nanjing, China, 2006
[66] Xia Z G, Clarke K C. Approaches to Scaling of Geo-spatial Data[M]//Quattrochi D A, Goodchild M F. Scale in Remote Sensing and GIS. Florida: CRC Press, 1997
[67] Chang Y, Mohanty K. Scale-Up of Two-Phase Flow in Heterogeneous Porous Media[J]. Journal of Petroleum Science and Engineering, 1997, 18(1-2):21-34 doi: 10.1016/S0920-4105(97)00002-8
[68] Moellering H, Tobler W. Geographical Variances[J]. Geographical Analysis, 1972, 4(1):34-50 http://d.old.wanfangdata.com.cn/Periodical/rdzwxb201408001
[69] Han Peng, Gong Jianya, Li Zhilin, et al. Selection of Optimal Scale in Remotely Sensed Image Classification[J]. Journal of Remote Sensing, 2010, 14(3):507-518 http://en.cnki.com.cn/Article_en/CJFDTOTAL-YGXB201003011.htm
[70] Han Peng, Li Zhilin, Gong Jianya. Effects of Aggregation Methods on Image Classification[M]//Geospatial Technology for Earth Observation. Berlin: Springer, 2010
[71] Cao C, Lam N S N. Understanding the Scale and Resolution Effects in Remote Sensing and GIS[M]//Quattrochi D A, Goodchild M F. Scale in Remote Sensing and GIS. Florida: CRC Press, 1997: 72-86
[72] 李志林, 刘启亮, 唐建波.尺度驱动的空间聚类理论[J].测绘学报, 2017, 46(10):1534-1548 doi: 10.11947/j.AGCS.2017.20170275 Li Zhilin, Liu Qiliang, Tang Jianbo. Towards a Scale-Driven Theory for Spatial Clustering[J]. Acta Geodaetica et Cartographica Sinica, 2017, 46(10):1534-1548 doi: 10.11947/j.AGCS.2017.20170275
[73] Gonzalo-Martín C, Lillo-Saavedra M, Menasalvas E, et al. Local Optimal Scale in a Hierarchical Segmentation Method for Satellite Images[J]. Journal of Intelligent Information Systems, 2016, 46(3):517-529 doi: 10.1007/s10844-015-0365-4
[74] Mandelbrot B. How Long is the Coast of Britain? Statistical Self-Similarity and Fractional Dimension[J]. Science, 1967, 156(3775):636-638 doi: 10.1126/science.156.3775.636
[75] Lowe D G. Distinctive Image Features from Scale-invariant Keypoints[J]. International Journal of Computer Vision, 2004, 60(2):91-110 doi: 10.1023/B:VISI.0000029664.99615.94
[76] Tan Shiteng, Xu Zhu, Ti Peng. A Comparative Study on Effects of Spatial Aggregation for GlobeLand30[C]. IEEE 23rd International Conference on Geoinformatics, Wuhan, China, 2015 https://www.researchgate.net/publication/304292343_A_comparative_study_on_effects_of_spatial_aggregation_for_GlobeLand30
[77] Li Zhilin, Openshaw S. A Natural Principle for Objective Generalization of Digital Maps[J]. Carto-graphy and Geographic Information Systems, 1993, 20(1):19-29 doi: 10.1559/152304093782616779
[78] Li Zhilin. Transformation of Spatial Representation in Scale Dimension:A New Paradigm for Digital Generalization of Spatial Data[J]. International Archives of Photogrammetry and Remote Sensing, 1996, 31:453-458
[79] Li Zhilin, Huang P. Transformations of Spatial Information in Multi-scale Representation[C].The 20th International Cartographic Conference, Beijing, China, 2001
[80] Li Zhilin. Multi-scale Digital Terrain Modelling and Analysis[M]//Advances in Digital Terrain Analysis. Berlin, Heidelberg: Springer, 2008
[81] 艾廷华, 何亚坤, 杜欣. GIS数据尺度变换中的信息熵变化[J].地理与地理信息科学, 2015, 31(2):7-11 doi: 10.3969/j.issn.1672-0504.2015.02.002 Ai Tinghua, He Yakun, Du Xin. Information Entropy Change in GIS Data Scale Transformation[J]. Geography and Geo-Information Science, 2015, 31(2):7-11 doi: 10.3969/j.issn.1672-0504.2015.02.002
[82] de Cola L. Multiresolution Convariation Among Landsat and AVHRR Vegetation Indices[M]//Quattrochi D A, Goodchild M F. Scale in Remote Sensing and GIS. Florida: CRC Press, 1997: 73-91
[83] Li Zhilin. Scale: A Fundamental Dimension in Spatial Information Science[C].The 1st International Symposium on Digital Earth, Beijing, China, 1999 https://www.researchgate.net/publication/228729837_Scale_A_Fundamental_Dimension_in_Spatial_Representation
-
期刊类型引用(21)
1. 李连发,高茜琳,贺伟,陈淼淼,杨晓梅,王志华,张俊瑶,刘晓亮. 自然资源遥感智能解译中复杂场景样本抽样方法研究. 地球信息科学学报. 2025(02): 331-349 .  百度学术
百度学术
2. 周访滨,肖智文,刘学军,马国伟,张珊珊. 栅格DEM山顶点提取的坡向分布特征法. 武汉大学学报(信息科学版). 2024(03): 419-425 .  百度学术
百度学术
3. 罗松开,罗志军. 顾及尺度效应的矿粮复合区耕地非粮化空间异质性及其关联因素. 农业工程学报. 2024(01): 265-275 .  百度学术
百度学术
4. 张自强,刘涛,杜萍,杨国林. 典型建筑物群组模式的空间图卷积模型DGCNN识别方法. 武汉大学学报(信息科学版). 2024(05): 868-878 .  百度学术
百度学术
5. 耿云龙,汤玉奇,尹芝勇,邹滨,冯徽徽. 顾及斑块面积比的国产卫星土地覆盖产品尺度效应研究. 遥感学报. 2023(03): 810-820 .  百度学术
百度学术
6. 宗琴,邓鑫洁,刘艳霞,陈慧. 面实体多重表达下的谱特征量化. 北京测绘. 2023(06): 818-822 .  百度学术
百度学术
7. 刘万增,陈军,赵勇,李然,章炜,翟曦,王新鹏,王勇. 地理信息保密安全评估的基本问题与对策. 时空信息学报. 2023(04): 474-481 .  百度学术
百度学术
8. 艾廷华,余华飞. 从横向上的“一张图”到纵向上的“金字塔”:地理国情数据的多尺度表达. 北京测绘. 2023(12): 1573-1580 .  百度学术
百度学术
9. XIU Chunliang,JIN Ying. Issues with Spatial Scale in Urban Research. Chinese Geographical Science. 2022(03): 373-388 .  必应学术
必应学术
10. 李雪铭,徐梁,田深圳,杨俊,刘美含,刘贺. 基于地理尺度的中国人居环境研究进展. 地理科学. 2022(06): 951-962 .  百度学术
百度学术
11. 刘耀林,刘启亮,邓敏,石岩. 地理大数据挖掘研究进展与挑战. 测绘学报. 2022(07): 1544-1560 .  百度学术
百度学术
12. 艾廷华,张翔. 地理信息科学中尺度概念的诠释与表达. 测绘学报. 2022(07): 1640-1652 .  百度学术
百度学术
13. 钟其洋,郭庆胜,王勇,罗安. 基于空间粒度的矢量空间数据比例尺估算方法. 测绘通报. 2022(08): 117-122 .  百度学术
百度学术
14. 左其亭. 人水关系学的时空观及研究方法. 人民珠江. 2022(11): 1-7 .  百度学术
百度学术
15. 王迪,钱海忠,赵钰哲. 综述与展望:地理空间数据的管理、多尺度变换与表达. 地球信息科学学报. 2022(12): 2265-2281 .  百度学术
百度学术
16. 艾廷华. 深度学习赋能地图制图的若干思考. 测绘学报. 2021(09): 1170-1182 .  百度学术
百度学术
17. 徐丰,张琦,牛继强,李慧敏,陈时雨,张兵兵. 多尺度空间实体多目标相似性度量. 测绘科学技术学报. 2021(05): 514-520 .  百度学术
百度学术
18. 隆玺,桂志鹏,彭德华,吴华意,宋爱红. 基于低通保边滤波和尺度约束大津法的网格空间多尺度聚类方法. 地理与地理信息科学. 2020(01): 65-74 .  百度学术
百度学术
19. 董文钱,董良,向琳,陶海军,赵传虎,曲寒冰. 基于贝叶斯时空模型的城管事件时空变化分析. 地球信息科学学报. 2020(05): 1073-1082 .  百度学术
百度学术
20. 秦昆,林珲,胡迪,许刚,张晓祥,卢宾宾,叶信岳. 空间综合人文学与社会科学研究综述. 地球信息科学学报. 2020(05): 912-928 .  百度学术
百度学术
21. 邱鸿亮. 关于情境设问空间尺度与精细程度的整合探究——基于高考全国卷地理综合题的案例分析. 中学地理教学参考. 2019(23): 57-61 .  百度学术
百度学术
其他类型引用(21)





 下载:
下载: