Model Selection Method Based on ARIMA Model in Outliers Detection of Satellite Clock Offset
-
摘要: 由于各种不确定因素的干扰,人们获取的卫星钟差数据中经常会出现异常扰动,降低了卫星钟性能分析的可靠性,破坏了钟差建模和预报的有效性,影响了导航定位结果的精准度。对此,以求和自回归移动平均模型为基础,建立了钟差时间序列异常值探测模型;基于Bayes统计原理,将异常值的定位和定值问题转化为模型选择问题;通过模型后验概率的近似计算,构建了模型选择的度量标准,避免了复杂的迭代计算问题。通过全球定位系统和北斗导航卫星系统不同卫星钟差数据的仿真试验,验证了所提出的方法对于卫星钟差序列中异常影响的定位和定值的正确性和有效性。
-
关键词:
- 卫星钟差 /
- 异常值 /
- Bayes原理 /
- 求和自回归移动平均模型
Abstract: Due to the interference of various uncertain factors, the abnormal disturbances often occur in the satellite clock offset data, which reduces the reliability of the performance analysis of the satellite clock, destroys the validity of the modeling and prediction of clock offset, and affects the accuracy of the navigation positioning results. As to this problem, on the basis of the autoregressive integrated move average (ARIMA) model, this paper establishes an outlier detection model of clock offset time series. Based on the principle of Bayes statistics, the problems of outliers detection and the outliers magnitudes estimation are transformed into a model selection problem. Through the approximate calculation of the posterior probability of the model, the measurement standard of the model selection is derived so the complex iterative computation is avoided. Simulation Test examples of GPS and BeiDou illustrate that the proposed method can detect the outliers effectively and estimate the magnitudes of outliers accurately in the clock offset sequence; furthermore, it can obtain higher prediction precision when the method is applied in the medium and long term prediction of the satellite clock offset. -
-
表 1 不同异常值数量的异常扰动定位与定值结果
Table 1 Locations and Magnitudes of Abnormal Disturbances with Different Numbers of Outliers
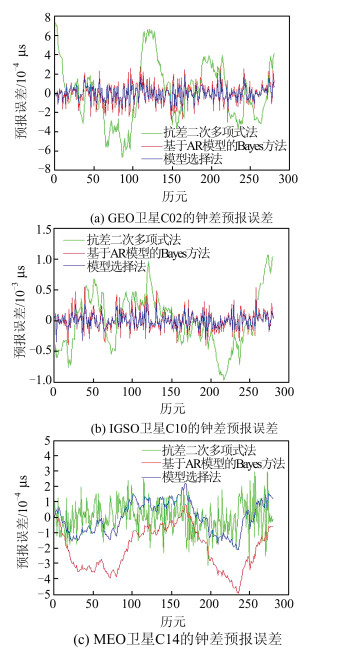
异常扰动项 i =0 i =1 i =2 i =3 τi 31 121 187 (31, 121) (31, 187) (121, 187) (31, 121, 187) wti/μs 2.745 2 -1.548 3 10.421 9 2.960 9,-2.973 4 2.840 6,6.054 2 -3.430 1,5.841 7 2.974 5,-2.826 3,6.040 1 MDO -1.337 7 -2.761 0 -2.756 5 -1.844 5 -2.535 3 -1.149 6 -1.143 3 22.052 1 表 2 3种方法的预报精度/ns
Table 2 Prediction Precisions of Three Methods/ns
预报方法 RMSEP Mean MAB 基于AR模型的Bayes方法 0.94 90.2 294 抗差二次多项式法 1.34 103.8 477 模型选择法 0.50 75.8 185 -
[1] 王宇谱. GNSS星载原子性能分析与卫星钟差建模预报研究[D].郑州: 信息工程大学, 2017 http://cdmd.cnki.com.cn/Article/CDMD-90005-1018702287.htm Wang Yupu. Research on Modeling and Prediction of the Satellite Clock Bias and Performance Evaluation of GNSS Satellite Clocks[D]. Zhengzhou: Information Engineering University, 2017 http://cdmd.cnki.com.cn/Article/CDMD-90005-1018702287.htm
[2] Winkler G M R. Introduction to Robust Statistics and Data Filtering[OL]. http://www.stable32.com/robstat.htm#INTRODUCTION, 1993
[3] Riley W J. The Calculation of Time Domain Frequency Stability[M/OL]. http://www.stable32.com/paper1ht.htm, 2002
[4] Riley W J. Handbook of Frequency Stability Analysis[M/OL]. http://www.stable32.com/Handbook.pdf, 2007
[5] 郭海荣.导航卫星原子钟时频特性分析理论与方法研究[D].郑州: 信息工程大学, 2006 http://d.wanfangdata.com.cn/Thesis_Y1032816.aspx Guo Hairong. Study on the Analysis Theories and Algorithms of the Time and Frequency Characterization for Atomic Clock of Navigation Satellites[D]. Zhengzhou: Information Engineering University, 2006 http://d.wanfangdata.com.cn/Thesis_Y1032816.aspx
[6] 黄观文. GNSS星载原子质量评价及精密钟差算法研究[M].北京:测绘出版社, 2017 Huang Guanwen. Research on Algorithms of Precise Clock Offset and Quality Evaluation of GNSS Satellite Clock[M]. Beijing:Surveying and Mapping Press, 2017
[7] Allan D W. The Statistics of Atomic Frequency Standards[J]. Proceedings of the IEEE, 1966, 54(2):221-230 http://d.old.wanfangdata.com.cn/OAPaper/oai_arXiv.org_0907.4849
[8] Steigenberger P, Hugentobler U, Hauschild A, et al. Orbit and Clock Analysis of Compass GEO and IGSO Satellites[J]. Journal of Geodesy, 2013, 87(6):515-525 doi: 10.1007/s00190-013-0625-4
[9] Steigenberger P, Hugentobler U, Loyer S, et al. Galileo Orbit and Clock Quality of the IGS Multi-GNSS Experiment[J]. Advances in Space Research, 2015, 55(1):269-281 doi: 10.1016/j.asr.2014.06.030
[10] Sesia I, Galleani L, Taavella P. Implementation of the Dynamic Allan Variance for the Galileo System Test Bed V2[C]. Frequency Control Symposium, 2007 Joint with 21st European Frequency and Time Forum, Geneva, Switzerland, 2007
[11] Sesia I, Galleani L, Taavella P. Application of the Dynamic Allan Variance for the Characterization of Space Clock Behavior[J]. IEEE Transactions on Aerospace and Electronic Systems, 2011, 47(2):884-895 doi: 10.1109/TAES.2011.5751232
[12] 于合理, 郝金明, 刘伟平, 等.一种卫星钟差异常实时监测算法[J].武汉大学学报·信息科学版, 2016, 41(1):106-110 http://ch.whu.edu.cn/CN/abstract/abstract3441.shtml Yu Heli, Hao Jinming, Liu Weiping, et al. A Real-Time Anomaly Monitoring Algorithm for Satellite Clock[J]. Geomatics and Information Science of Wuhan University, 2016, 41(1):106-110 http://ch.whu.edu.cn/CN/abstract/abstract3441.shtml
[13] 牛飞, 韩春好, 张义生, 等.导航卫星星载原子钟异常监测分析[J].武汉大学学报·信息科学版, 2009, 34(5):585-588 http://ch.whu.edu.cn/CN/abstract/abstract1261.shtml Niu Fei, Han Chunhao, Zhang Yisheng, et al. Analysis and Detection on Atomic Clock Anomaly of Navigation Satellites[J]. Geomatics and Information Science of Wuhan University, 2009, 34(5):585-588 http://ch.whu.edu.cn/CN/abstract/abstract1261.shtml
[14] Zhang Qianqian, Gui Qingming. Bayesian Methods for Outliers Detection in GNSS Time Series[J]. Journal of Geodesy, 2013, 87(7):609-627 doi: 10.1007/s00190-013-0640-5
[15] 张倩倩, 韩松辉, 杜兰, 等.星地时间同步钟差异常处理的Bayesian方法[J].武汉大学学报·信息科学版, 2016, 41(6):772-777 http://ch.whu.edu.cn/CN/abstract/abstract5462.shtml Zhang Qianqian, Han Songhui, Du Lan, et al. Bayesian Methods for Outliers Detection and Estimation in Clock Offset Measurements of Satellite-Ground Time Transfer[J]. Geomatics and Information Science of Wuhan University, 2016, 41(6):772-777 http://ch.whu.edu.cn/CN/abstract/abstract5462.shtml
[16] 李涛, 衡广辉, 归庆明. AR序列异常值探测的Bayes方法在卫星钟差预报中的应用[J].全球定位系统, 2010(4):15-20 doi: 10.3969/j.issn.1008-9268.2010.04.004 Li Tao, Heng Guanghui, Gui Qingming. The Application of Bayesian Method in Autoregressive Model Outliers Detecting of Satellite Clock Error Prediction[J]. GNSS World of China, 2010(4):15-20 doi: 10.3969/j.issn.1008-9268.2010.04.004
[17] 王宇谱, 吕志平, 陈正生, 等.一种新的钟差预处理方法及在WNN钟差中长期预报中的应用[J].武汉大学学报·信息科学版, 2016, 41(3):373-379 http://ch.whu.edu.cn/CN/abstract/abstract4578.shtml Wang Yupu, Lv Zhiping, Chen Zhengsheng, et al. A New Data Preprocessing Method for Satellite Clock Biased Its Application in WNN to Predict Medium-Term and Long-Term Clock Bias[J]. Geomatics and Information Science of Wuhan University, 2016, 41(3):373-379 http://ch.whu.edu.cn/CN/abstract/abstract4578.shtml
[18] 滕云龙, 师亦兵, 郑植.时间序列分析在周跳探测与修复中的应用[J].宇航学报, 2011, 32(3):543-548 doi: 10.3873/j.issn.1000-1328.2011.03.014 Teng Yunlong, Shi Yibing, Zheng Zhi. Time Series Analysis and Its Application in Detection and Correction of Cycle Slip[J]. Journal of Astronautics, 2011, 32(3):543-548 doi: 10.3873/j.issn.1000-1328.2011.03.014
[19] 郭海荣, 杨生, 杨元喜, 等.基于卫星双向时间频率传递进行钟差预报的方法研究[J].武汉大学学报·信息科学版, 2007, 32(1):43-46 http://ch.whu.edu.cn/CN/abstract/abstract1809.shtml Guo Hairong, Yang Sheng, Yang Yuanxi, et al. Numerical Prediction Methods for Clock Difference Based on Two-Way Satellite Time and Frequency Transfer Data[J]. Geomatics and Information Science of Wuhan University, 2007, 32(1):43-46 http://ch.whu.edu.cn/CN/abstract/abstract1809.shtml
[20] Koch K R. Bayesian Inference with Geodetic Application[M]. Berlin:Springer, 1990
[21] Box G E P, Jenkins G M. Time Series Analysis:Forecasting and Control[M]. 4th ed. New Jersey:Hoboken, 2011
[22] Claeskens G, Hjort N L. Model Selection and Model Averaging[M]. New York:North-Holland, 2008
[23] Schwarz G. Estimating the Dimension of a Model[J]. The Annals of Statistics, 1978, 6(2):461-464 doi: 10.1214/aos/1176344136
-
期刊类型引用(14)
1. 刘学习,朱守庆,陈国,张克非,郑南山,刘婧璇. 基于全球统一坐标框架的GNSS精密轨道与钟差产品一致性分析. 测绘学报. 2025(03): 432-447 .  百度学术
百度学术
2. 冯晓亮,陈欢,李厚芝. 不同观测环境中的多模GNSS数据质量自动化检测方法. 测绘工程. 2024(06): 56-61 .  百度学术
百度学术
3. 刘嘉伟,孙保琪,韩蕊,张喆,王侃,袁海波,杨旭海. GNSS多系统RTK授时性能分析. 导航定位与授时. 2023(03): 49-58 .  百度学术
百度学术
4. 王浩浩,郝明,庄文泉. GNSS实时卫星钟差估计在地震监测中的应用. 导航定位与授时. 2023(03): 108-116 .  百度学术
百度学术
5. 周长江,余海锋,王林伟,雷云平,岳彩亚. 无频间钟偏差改正的BDS-2三频非组合PPP随机模型优化. 测绘通报. 2023(12): 164-168 .  百度学术
百度学术
6. 潘丽静,刘翔,夏川茹,王雷雷. GNSS精密卫星钟差实时估计与分析. 城市勘测. 2021(06): 73-76 .  百度学术
百度学术
7. 郭磊,王甫红,桑吉章,张万威. 一种新的利用历元间位置变化量约束的GNSS导航算法. 武汉大学学报(信息科学版). 2020(01): 21-27 .  百度学术
百度学术
8. 陶钧,张柔. GPS/BeiDou/Galileo/GLONASS实时精密卫星钟差估计. 测绘地理信息. 2020(03): 102-106 .  百度学术
百度学术
9. 黄观文,王浩浩,谢威,曹钰. GNSS实时卫星钟差估计技术进展. 导航定位与授时. 2020(05): 1-9 .  百度学术
百度学术
10. 张浩,赵兴旺,陈佩文,谢毅. GPS/BDS卫星钟差融合解算模型及精度分析. 合肥工业大学学报(自然科学版). 2020(09): 1192-1196 .  百度学术
百度学术
11. 叶珍,李浩军. GNSS卫星钟差估计与结果分析. 导航定位与授时. 2019(03): 88-94 .  百度学术
百度学术
12. 盛剑锋,张彩红,谭凯. 一种全球导航卫星系统钟差估计优化方案的量化研究. 科学技术与工程. 2019(14): 14-21 .  百度学术
百度学术
13. 王尔申,赵珩,曲萍萍,庞涛,孙军. 基于拉格朗日插值法的卫星导航空间信号精度评估算法. 沈阳航空航天大学学报. 2019(04): 43-48 .  百度学术
百度学术
14. 李云,崔文刚. 精密单点定位技术发展及应用. 科学技术与工程. 2019(27): 1-11 .  百度学术
百度学术
其他类型引用(10)





 下载:
下载:


