A Method for Indoor Map Unified Mathematical Transformation Based on Transition Projecting Plane
-
摘要: 室内外统一的地图数学基础是室内外空间数据共享和使用的重要基础,是室内外一体化位置服务的重要保证,并且对于复杂室内及地下空间的安全管理与应急响应具有重要作用。室内地图通常采用自定义局部平面坐标系,造成室内与室外地图以及不同建筑室内地图之间数学基础的不一致。针对此,将地理坐标系作为室内地图统一遵循的数学基础,研究了室内地图从局部平面坐标系到地理坐标系的转换方法。分析了传统地图数学基础转换方法的适用性之后,提出了一种基于过渡投影面的室内地图统一数学基础转换方法。选取某住宅区的实测数据进行了转换试验,结果表明该方法可以显著提高室内地图统一数学基础转换的精度。Abstract: Unified mathematics basic of indoor and outdoor map is an important foundation for spatial data share and use between indoor and outdoor, and an important guarantee of the indoor and outdoor integrated LBS, which plays an important role in safety management and emergency response in complex indoor and underground space. Indoor map usually uses user-defined local plane coordinate system, which results in contradictions in terms of mathematics basic between indoor and outdoor map, and also among different building's indoor maps. Taking geographical coordinate system as the unified mathematics basic adopted by indoor map spatial data, this paper studied the method for transforming indoor map from local plane coordinate system into geographical coordinate system. Through analysis the applicability of traditional map mathematical transformation methods, this paper proposed a method for indoor map unified mathematical transformation based on transition projecting plane. Choosing a residential area's survey data to perform a transformation experiment, the results show that the method proposed in this paper can improve the accuracy of indoor map mathematical transformation efficiently in contrast to traditional methods.
-
人类80%~90%的时间生活在室内[1],随着大型公共建筑的不断出现及其内部结构的日益复杂,以室内地图为基础的室内位置服务逐渐成为导航与位置服务领域的研究热点[2-4]。室内地图是按一定的数学法则,以图形、文字相结合的符号系统表示封闭有限空间内的结构分布与地理信息的一种地图[5-8],其制图对象不仅包括商场、车站、机场等地上公共建筑,而且涵盖了地铁、防空洞、矿井等地下建筑空间。
室内外一体化是位置服务的重要特征和未来发展的必然趋势。目前,地图服务提供商通过链接或缩放的方式实现了室内外地图的切换或叠加显示[1],但并不具备室内外一体化路径分析与导航等功能,室内外的位置服务本质上是相互独立的。为了实现位置服务的室内外精准无缝融合,不仅需要高精度的室内外无缝定位技术[9],而且需要室内与室外空间数据的高精度无缝融合。
室内与室外空间数据高精度无缝融合的关键是地图数学基础的统一[10]。由于建筑一般相对独立和封闭,通常需要建立局部平面坐标系,造成室内外地图之间数学基础的差异。这不仅影响室内外空间数据的共享和使用,而且不利于购物中心、机场、地铁、矿井等复杂室内及地下空间的安全管理与应急响应[4]。
为了方便空间数据的共享和使用[11],室外地图通常采用地理坐标系作为统一的数学基础[12]。而室内地图采用的局部平面坐标系难以统一描述,本文将地理坐标系作为室内空间数据统一遵循的数学基础,以便同时实现室内外一体化的地图显示和路径分析等功能。此外,本文将室内地图从局部平面坐标系到地理坐标系的转换称为统一数学基础转换。
室内环境中难以获取大量共同点的精确地理坐标,而且室内地图的比例尺较大,数学基础转换的精度需求较高,导致传统地图数学基础转换方法的适用性较差。基于此,本文提出了一种基于过渡投影面的转换方法。
1 传统地图数学基础转换方法分析
传统的地图数学基础转换方法主要分为解析变换和数值变换。其中,数值变换主要包括多项式变换[13]、仿射变换[14-15]和相似变换[16-17]。由于室内地图的局部平面坐标系不具备严格的地图投影解析式,不能通过解析变换进行数学基础转换,因此,本文主要分析数值变换的几种方法。其中,(X,Y)代表室内地图局部平面坐标,(B,L)代表地理坐标。
1) 多项式变换
根据逼近函数的不同,多项式变换主要包括二元n次多项式和乘积型插值多项式[18],其中又分别以二元三次多项式和二元双二次多项式的应用居多[19]。
二元三次多项式表示为:
$$ \left\{ \begin{array}{l} B = {a_{00}} + {a_{10}}X + {a_{01}}Y + {a_{20}}{X^2} + {a_{11}}XY + \\ \;\;\;\;\;\;{a_{02}}{Y^2} + {a_{30}}{X^3} + {a_{21}}{X^2}Y + {a_{12}}X{Y^2} + {a_{03}}{Y^3}\\ L = {b_{00}} + {b_{10}}X + {b_{01}}Y + {b_{20}}{X^2} + {b_{11}}XY + \\ \;\;\;\;\;\;{b_{02}}{Y^2} + {b_{30}}{X^3} + {b_{21}}{X^2}Y + {b_{12}}X{Y^2} + {b_{03}}{Y^3} \end{array} \right. $$ (1) 二元双二次多项式表示为:
$$ \left\{ \begin{array}{l} B = {a_{11}} + {a_{21}}X + {a_{12}}Y + {a_{31}}{X^2} + {a_{22}}XY + \\ \;\;\;\;\;\;{a_{13}}{Y^2} + {a_{32}}{X^2}Y + {a_{23}}X{Y^2} + {a_{33}}{X^2}{Y^2}\\ L = {b_{11}} + {b_{21}}X + {b_{12}}Y + {b_{31}}{X^2} + {b_{22}}XY + \\ \;\;\;\;\;\;{b_{13}}{Y^2} + {b_{32}}{X^2}Y + {b_{23}}X{Y^2} + {b_{33}}{X^2}{Y^2} \end{array} \right. $$ (2) 式(1)与式(2)中,aij、bij代表多项式参数,直接求解至少分别需要10个和9个共同点。但是由于室内环境的特殊性,难以通过GPS等技术获取较多共同点的精确地理坐标,因此,多项式变换的方法对于室内地图统一数学基础转换的适用性较差。
2) 仿射变换与相似变换
仿射变换是一种建立两个空间平面之间坐标转换关系的常用方法。相似变换是通过对坐标轴的旋转、平移和缩放建立两个平面坐标系的转换关系。这两种变换方法已被广泛应用于地图数字化配准和影像几何纠正等方面[20-22]。
仿射变换关系式为:
$$ \left\{ \begin{array}{l} B = {a_1}X + {b_1}Y + {c_1}\\ L = {a_2}X + {b_2}Y + {c_2} \end{array} \right. $$ (3) 相似变换关系式为:
$$ \left\{ \begin{array}{l} B = k\left( {\cos \alpha \cdot X + \sin \alpha \cdot Y + \Delta X} \right)\\ L = k\left( { - \sin \alpha \cdot X + \cos \alpha \cdot Y + \Delta Y} \right) \end{array} \right. $$ (4) 式(3)中,ai、bi、ci代表仿射变换参数。式(4)中,ΔX、ΔY代表坐标轴平移量;α代表坐标轴旋转角度;k代表坐标轴缩放比例。直接求解式(3)和式(4)分别至少需要3个和2个共同点。但是,这两种方法的前提是假设建筑局部范围内的地理坐标系为平面坐标系,因此当建筑范围相对较大时,数学基础转换的精度难以得到有效保证。
2 基于过渡投影面的转换方法
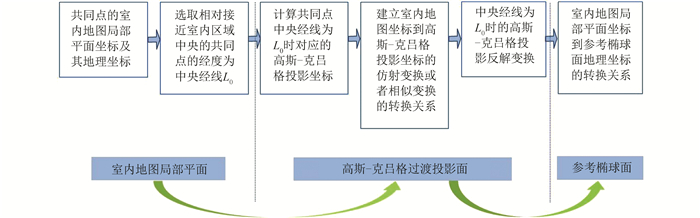
为了通过较少数目的共同点实现室内地图统一数学基础的高精度转换,本文提出了一种基于过渡投影面的转换方法。该方法首先采用某种地图投影将室内地图局部范围的地理坐标系投影为具有严格数学基础的平面坐标系,然后通过仿射变换或相似变换建立室内地图局部平面坐标系到过渡投影平面坐标系的转换关系,最后根据所采用地图投影的反解变换关系式建立局部平面坐标系到地理坐标系的转换关系。
由于地图投影变形的影响,基于过渡投影面的方法同样存在转换误差。高斯-克吕格投影具有等角性质且中央经线投影后无长度变形,广泛应用于大比例尺地形图编制和工程测量坐标系统等方面[19]。基于该投影过渡平面实现室内地图统一数学基础转换(图 1),需要根据共同点的数目选择采用仿射变换或相似变换作为基础变换。
1) 选取经线方向上相对接近制图区域中央的共同点,设为P1。根据高斯-克吕格投影的变形性质,相同纬度上距离中央经线越远投影变形越大,因此,中央经线要尽量位于制图区域中央。
2) 将P1的经度作为中央经线L0,共同点的地理坐标(B, L)到高斯-克吕格投影平面坐标(x,y)的正解关系式如下:
$$ \left\{ \begin{array}{l} x = S + \frac{1}{2}Nt{\cos ^2}B \cdot {l^2} + \frac{1}{{24}}Nt\left( {5 - {t^2} + 9{\eta ^2} + 4{\eta ^4}} \right){\cos ^4}B \cdot {l^4} + \\ \;\;\;\;\;\frac{1}{{720}}Nt\left( {61 - 58{t^2} + {t^4} + 270{\eta ^2} - 330{t^2}{\eta ^2}} \right){\cos ^6}B \cdot {l^6}\\ y = N\cos B \cdot l + \frac{1}{6}N\left( {1 - {t^2} + {\eta ^2}} \right){\cos ^3}B \cdot {l^3} + \frac{1}{{120}}N\left( {5 - 18{t^2} + {t^4} + 14{\eta ^2} - } \right.\\ \;\;\;\;\;\;\left. {58{t^2}{\eta ^2}} \right){\cos ^5}B \cdot {l^5} + \frac{1}{{5\;040}}N\left( {61 - 479{t^2} + 179{t^4} - {t^6}} \right){\cos ^7}B \cdot {l^7}\\ N = \frac{a}{{{{\left( {1 - {e^2}{{\sin }^2}B} \right)}^{1/2}}}},\eta = e'\cos B,t = {\rm{tg}}B\\ e = \frac{{\sqrt {{a^2} - {b^2}} }}{a},e' = \frac{{\sqrt {{a^2} - {b^2}} }}{b},l = L - {L_0} \end{array} \right. $$ (5) 式中,a、b分别代表参考椭球体的长半径和短半径;S代表由赤道至纬度B的经线弧长; l代表经差。
3) 如果存在不少于3个共同点,采用仿射变换建立室内地图坐标(X,Y)到高斯-克吕格投影平面坐标(x,y)的转换关系(式(6))。如果仅存在2个共同点,采用相似变换建立室内地图坐标(X,Y)到高斯-克吕格投影平面坐标(x,y)的转换关系(式(7))。
$$ \left\{ \begin{array}{l} x = {a_1}X + {b_1}Y + {c_1}\\ y = {a_2}X + {b_2}Y + {c_2} \end{array} \right. $$ (6) $$ \left\{ \begin{array}{l} x = k\left( {\cos \alpha \cdot X + \sin \alpha \cdot Y + \Delta X} \right)\\ y = k\left( { - \sin \alpha X + \cos \alpha \cdot Y + \Delta Y} \right) \end{array} \right. $$ (7) 式(6)中,ai、bi、ci代表仿射变换参数。式(7)中,ΔX、ΔY代表坐标轴平移量;α代表坐标轴旋转角度;k代表坐标轴缩放比例。
4) 中央经线L0的高斯-克吕格投影坐标(x,y)到地理坐标(B,L)的反解变换关系见式(8)。式中,Bf是底点纬度。根据式(6)或式(7)联立式(8), 即可建立室内地图坐标(X,Y)到地理坐标(B,L)的转换关系。
$$ \left\{ \begin{array}{l} B = {B_f} + \frac{1}{{2N_f^2}}{t_f}\left( { - 1 - \eta _f^2} \right){y^2} + \frac{1}{{24N_f^4}}{t_f}\left( {5 + 3t_f^2 + 6\eta _f^2 - 6t_f^2\eta _f^2 - 3\eta _f^4 - 9t_f^2\eta _f^4} \right){y^4} + \\ \;\;\;\;\;\;\frac{1}{{720N_f^6}}{t_f}\left( { - 61 - 90t_f^2 - 45t_f^4 - 107\eta _f^2 + 162t_f^2\eta _f^2 + 45t_f^4\eta _f^2} \right){y^6}\\ L = \frac{1}{{{N_f}\cos {B_f}}}y + \frac{1}{{6N_f^3\cos {B_f}}}\left( { - 1 - 2t_f^2 - \eta _f^2} \right){y^3} + \frac{1}{{120N_f^5\cos {B_f}}}\left( {5 + 28t_f^2 + 24t_f^4 + 6\eta _f^2 + 8t_f^2\eta _f^2} \right){y^5} + {L_0}\\ {N_f} = \frac{a}{{{{\left( {1 - {e^2}{{\sin }^2}{B_f}} \right)}^{1/2}}}},{\eta _f} = e'\cos {B_f},{t_f} = {\rm{tg}}{B_f} \end{array} \right. $$ (8) 3 试验与分析
3.1 试验数据
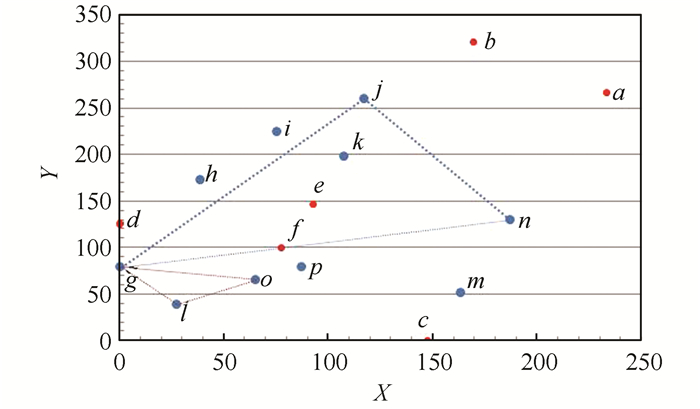
本文选取杭州市某住宅区多栋建筑室内地图共用的局部平面坐标系进行室内地图统一数学基础转换试验。实测点主要分布于建筑边缘或空旷地带,以提高地理坐标获取的精度,图 2所示为局部坐标系下实测点的分布情况,对应的坐标值见表 1,其中地理坐标采用WGS84参考椭球体。
表 1 某住宅区实测点坐标Table 1. Surveying Dots Coordinates of a Residential Area实测点 局部平面坐标 地理坐标 X/m Y/m B L a 233.533 266.207 30°19′26.148 12″N 120°06′59.980 11″E b 169.567 320.730 30°19′24.071 42″N 120°07′02.021 83″E c 147.452 0.000 30°19′23.349 93″N 120°06′50.016 14″E d 0.000 125.443 30°19′18.562 87″N 120°06′54.713 65″E E 92.869 146.129 30°19′21.578 94″N 120°06′55.486 86″E f 77.718 99.531 30°19′21.086 43″N 120°06′53.742 75″E g 0.438 78.361 30°19′18.576 60″N 120°06′52.951 24″E h 38.762 172.541 30°19′19.822 13″N 120°06′56.476 19″E i 75.698 224.644 30°19′21.022 13″N 120°06′58.426 12″E j 117.495 259.790 30°19′22.379 81″N 120°06′59.741 25″E k 107.976 198.073 30°19′22.070 06″N 120°06′57.431 10″E l 27.616 38.249 30°19′19.458 76″N 120°06′51.449 40″E m 163.830 51.185 30°19′23.882 34″N 120°06′51.931 96″E N 187.268 129.321 30°19′24.644 30″N 120°06′54.856 55″E o 65.315 65.536 30°19′20.683 30″N 120°06′52.470 37″E p 87.404 78.522 30°19′21.400 76″N 120°06′52.956 20″E 3.2 转换试验
实测点a至f作为数学基础转换的检查点,假设某检查点实测的地理坐标为(B1,L1),经过转换得到的地理坐标为(B2,L2),本文采用的距离误差ΔS的计算表达式如下:
$$ \left\{ \begin{array}{l} \Delta S = \sqrt {\Delta S_B^2 + \Delta S_L^2} \\ \Delta {S_B} = \Delta L \cdot R \cdot \cos {B_1},\Delta {S_L} = R \cdot \Delta B\\ \Delta B = \left| {{B_1} - {B_2}} \right|,\Delta L = \left| {{L_1} - {L_2}} \right|\\ R = \left( {a + b + a} \right)/3 \end{array} \right. $$ (9) 式中,a、b分别代表参考椭球长半径和短半径;R代表平均地球半径;ΔB、ΔL分别代表纬线和经线方向绝对弧度误差;ΔSB、ΔSL分别代表纬线和经线方向的距离误差。
1) 多项式变换
选取g至p的10个共同点进行二元三次多项式变换,选取g至o的9个共同点进行二元双二次多项式变换,检查点的经纬度绝对误差和距离误差见表 2。其中,二元三次多项式最大距离误差1.85 mm,平均距离误差0.72 mm;二元双二次多项式最大距离误差4.53 mm,平均距离误差1.27 mm。
表 2 多项式变换误差Table 2. Error of Polynomials Transformation检查点 二元三次多项式 二元双二次多项式 ΔB/(″) ΔL/(″) ΔS/mm ΔB/(″) ΔL/(″) ΔS/mm a 0.000 03″ 0.000 06″ 1.848 69 0.000 00″ 0.000 17″ 4.532 48 b 0.000 01″ 0.000 01″ 0.408 03 0.000 01″ 0.000 08″ 2.155 19 c 0.000 01″ 0.000 03″ 0.857 42 0.000 00″ 0.000 02″ 0.533 24 d 0.000 00″ 0.000 02″ 0.533 24 0.000 01″ 0.000 01″ 0.408 03 e 0.000 00″ 0.000 01″ 0.266 62 0.000 00″ 0.000 00″ 0.000 00 f 0.000 01″ 0.000 01″ 0.408 03 0.000 00″ 0.000 00″ 0.000 00 2) 仿射变换
分别选取g、j、n(方案1)和g、l、o(方案2)为共同点直接进行仿射变换,检查点的经纬度绝对误差和距离误差见表 3。其中,方案1的最大距离误差1.89 mm,平均距离误差1.00 mm;方案2的最大距离误差4.16 mm,平均距离误差1.66 mm。
表 3 直接进行仿射变换的误差Table 3. Error of Direct Affine Transformation检查点 共同点分布方案1 共同点分布方案2 ΔB/(″) ΔL/(″) ΔS/mm ΔB/(″) ΔL/(″) ΔS/mm a 0.000 01″ 0.000 07″ 1.891 70 0.000 08″ 0.000 10″ 3.635 15 b 0.000 02″ 0.000 05″ 1.469 27 0.000 11″ 0.000 09″ 4.159 54 c 0.000 04″ 0.000 02″ 1.345 66 0.000 02″ 0.000 02″ 0.816 06 d 0.000 01″ 0.000 02″ 0.616 24 0.000 01″ 0.000 01″ 0.408 03 e 0.000 01″ 0.000 01″ 0.408 03 0.000 02″ 0.000 00″ 0.617 75 f 0.000 00″ 0.000 01″ 0.266 62 0.000 01″ 0.000 00″ 0.308 88 对应于共同点分布方案1,选取实测点n的经度作为中央经线。对应于共同点分布方案2,选取实测点g的经度作为中央经线。基于过渡投影面分别进行仿射变换,两种共同点分布方案的检查点经纬度绝对误差和距离误差见表 4。其中,方案1的最大距离误差0.27 mm,平均距离误差0.04 mm;方案2的最大距离误差1.11 mm,平均距离误差0.64 mm。
表 4 基于过渡投影面进行仿射变换的误差Table 4. Error of Affine Transformation Based on Transition Projecting Plane检查点 共同点分布方案1 共同点分布方案2 ΔB/(″) ΔL/(″) ΔS/mm ΔB/(″) ΔL/(″) ΔS/mm a 0.000 00″ 0.000 00″ 0.000 00 0.000 01″ 0.000 04″ 1.110 29 b 0.000 00″ 0.000 00″ 0.000 00 0.000 01″ 0.000 04″ 1.110 30 c 0.000 00″ 0.000 00″ 0.000 00 0.000 01″ 0.000 01″ 0.408 03 d 0.000 00″ 0.000 00″ 0.000 00 0.000 00″ 0.000 01″ 0.266 62 e 0.000 00″ 0.000 01″ 0.266 62 0.000 00″ 0.000 02″ 0.533 24 f 0.000 00″ 0.000 00″ 0.000 00 0.000 01″ 0.000 01″ 0.408 03 3) 相似变换
分别选取g、n(方案3)和g、l(方案4)为共同点直接进行相似变换,检查点的经纬度绝对误差和距离误差见表 5。其中,方案3的最大距离误差30 567.11 mm,平均距离误差12 473.17 mm;方案4的最大距离误差43 827.73 mm,平均距离误差21 264.06 mm。
表 5 直接进行相似变换的误差Table 5. Error of Direct Similarity Transformation检查点 共同点分布方案3 共同点分布方案4 ΔB/(″) ΔL/(″) ΔS/mm ΔB/(″) ΔL/(″) ΔS/mm a 0.248 68″ 0.921 97″ 25 753.375 20 1.225 04″ 0.829 51″ 43 827.730 84 b 0.294 18″ 1.094 65″ 30 567.107 40 1.133 21″ 0.767 29″ 40 541.901 01 c 0.109 98″ 0.413 15″ 11 527.248 66 0.319 26″ 0.216 14″ 11 421.510 73 d 0.005 48″ 0.016 18″ 463.412 84 0.106 95″ 0.072 40″ 3 826.070 49 e 0.051 94″ 0.193 96″ 5 414.491 58 0.470 28″ 0.318 41″ 16 824.655 35 f 0.010 86″ 0.039 82″ 1 113.412 30 0.311 45″ 0.210 88″ 11 142.495 10 对应于共同点分布方案3,选取实测点n的经度作为中央经线。对应于共同点分布方案4,选取实测点g的经度作为中央经线。基于过渡投影面分别进行相似变换,两种共同点分布方案的检查点经纬度绝对误差和距离误差见表 6。其中,方案3的最大距离误差0.41 mm,平均距离误差0.12 mm;方案4的最大距离误差1.22 mm,平均距离误差0.64 mm。
表 6 基于过渡投影面进行相似变换的误差Table 6. Error of Similarity Transformation Based on Transition Projecting Plane检查点 共同点分布方案3 共同点分布方案4 ΔB/(″) ΔL/(″) ΔS/mm ΔB/(″) ΔL/(″) ΔS/mm a 0.000 01″ 0.000 00″ 0.308 88 0.000 03″ 0.000 03″ 1.224 09 b 0.000 01″ 0.000 01″ 0.408 03 0.000 02″ 0.000 03″ 1.010 63 c 0.000 00″ 0.000 00″ 0.000 00 0.000 01″ 0.000 01″ 0.408 03 d 0.000 00″ 0.000 00″ 0.000 00 0.000 00″ 0.000 01″ 0.266 62 e 0.000 00″ 0.000 00″ 0.000 00 0.000 01″ 0.000 02″ 0.616 24 f 0.000 00″ 0.000 00″ 0.000 00 0.000 01″ 0.000 00″ 0.308 88 3.3 试验分析
根据§3.2采用不同方法进行数学基础转换的检查点误差统计结果,本文从以下几个方面进行对比分析。
1) 传统方法对比分析。由表 2、表 3和表 5可以看出,多项式变换和直接进行仿射变换的误差相对较小;然而,直接进行相似变换的误差较大,难以满足室内地图的精度需求;二元三次多项式的转换精度相对优于二元双二次多项式。
2) 共同点分布的影响。由表 3~6可以看出,共同点的分布对直接或基于过渡投影面进行仿射变换和相似变换的精度存在一定影响,共同点均匀分布于转换区域的边缘时,数学基础转换的精度相对较高。
3) 本文方法的优势。由表 3和表 4的对比以及表 5和表 6的对比可以看出,相同共同点的情况下,本文方法可以显著提高直接进行仿射变换或相似变换的精度。从表 6与表 2的对比可以看出,本文方法的转换精度优于共同点数目较多的多项式转换,进一步验证了本文方法的有效性。
4 结语
室内外一体化是位置服务发展的必然趋势,室内外统一的地图数学基础是实现该目标的重要保证。本文在仿射变换和相似变换的基础上,提出了一种基于过渡投影面的转换方法,进行了某住宅区局部平面坐标系到地理坐标系的转换试验,并得出以下结论。
1) 相比直接进行仿射变换或相似变换,基于过渡投影面的转换方法可以显著提高数学基础的转换精度,具有较好的适用性和有效性。
2) 为了尽量提高室内地图统一数学基础的转换精度,共同点的选取应当均匀分布于转换区域的边缘,避免集中位于转换区域的局部范围。
3) 由于多项式变换与仿射变换、相似变换都属于数值变换,因此基于过渡投影面的多项式转换方法同样适用。考虑到仿射变换相比多项式变换的参数较少、易于实现,以及位置服务的实际应用需求,能够满足数学基础转换精度需求的情况下,基于过渡投影面的仿射变换方法具有相对更好的适用性。
由于本文的方法建立在传统数值变换的基础之上,因此其适用性与采用的数值变换密切相关。对于特大型建筑或地下工程,采用仿射变换或相似变换的精度也会随转换区域的增大而降低,若采用多项式变换同样会对共同点的数目需求较多。因此,本文下一步将开展基于过渡投影面的不同数值变换方法与不同室内区域范围数学基础转换精度需求的适用性分析。
-
表 1 某住宅区实测点坐标
Table 1 Surveying Dots Coordinates of a Residential Area
实测点 局部平面坐标 地理坐标 X/m Y/m B L a 233.533 266.207 30°19′26.148 12″N 120°06′59.980 11″E b 169.567 320.730 30°19′24.071 42″N 120°07′02.021 83″E c 147.452 0.000 30°19′23.349 93″N 120°06′50.016 14″E d 0.000 125.443 30°19′18.562 87″N 120°06′54.713 65″E E 92.869 146.129 30°19′21.578 94″N 120°06′55.486 86″E f 77.718 99.531 30°19′21.086 43″N 120°06′53.742 75″E g 0.438 78.361 30°19′18.576 60″N 120°06′52.951 24″E h 38.762 172.541 30°19′19.822 13″N 120°06′56.476 19″E i 75.698 224.644 30°19′21.022 13″N 120°06′58.426 12″E j 117.495 259.790 30°19′22.379 81″N 120°06′59.741 25″E k 107.976 198.073 30°19′22.070 06″N 120°06′57.431 10″E l 27.616 38.249 30°19′19.458 76″N 120°06′51.449 40″E m 163.830 51.185 30°19′23.882 34″N 120°06′51.931 96″E N 187.268 129.321 30°19′24.644 30″N 120°06′54.856 55″E o 65.315 65.536 30°19′20.683 30″N 120°06′52.470 37″E p 87.404 78.522 30°19′21.400 76″N 120°06′52.956 20″E 表 2 多项式变换误差
Table 2 Error of Polynomials Transformation
检查点 二元三次多项式 二元双二次多项式 ΔB/(″) ΔL/(″) ΔS/mm ΔB/(″) ΔL/(″) ΔS/mm a 0.000 03″ 0.000 06″ 1.848 69 0.000 00″ 0.000 17″ 4.532 48 b 0.000 01″ 0.000 01″ 0.408 03 0.000 01″ 0.000 08″ 2.155 19 c 0.000 01″ 0.000 03″ 0.857 42 0.000 00″ 0.000 02″ 0.533 24 d 0.000 00″ 0.000 02″ 0.533 24 0.000 01″ 0.000 01″ 0.408 03 e 0.000 00″ 0.000 01″ 0.266 62 0.000 00″ 0.000 00″ 0.000 00 f 0.000 01″ 0.000 01″ 0.408 03 0.000 00″ 0.000 00″ 0.000 00 表 3 直接进行仿射变换的误差
Table 3 Error of Direct Affine Transformation
检查点 共同点分布方案1 共同点分布方案2 ΔB/(″) ΔL/(″) ΔS/mm ΔB/(″) ΔL/(″) ΔS/mm a 0.000 01″ 0.000 07″ 1.891 70 0.000 08″ 0.000 10″ 3.635 15 b 0.000 02″ 0.000 05″ 1.469 27 0.000 11″ 0.000 09″ 4.159 54 c 0.000 04″ 0.000 02″ 1.345 66 0.000 02″ 0.000 02″ 0.816 06 d 0.000 01″ 0.000 02″ 0.616 24 0.000 01″ 0.000 01″ 0.408 03 e 0.000 01″ 0.000 01″ 0.408 03 0.000 02″ 0.000 00″ 0.617 75 f 0.000 00″ 0.000 01″ 0.266 62 0.000 01″ 0.000 00″ 0.308 88 表 4 基于过渡投影面进行仿射变换的误差
Table 4 Error of Affine Transformation Based on Transition Projecting Plane
检查点 共同点分布方案1 共同点分布方案2 ΔB/(″) ΔL/(″) ΔS/mm ΔB/(″) ΔL/(″) ΔS/mm a 0.000 00″ 0.000 00″ 0.000 00 0.000 01″ 0.000 04″ 1.110 29 b 0.000 00″ 0.000 00″ 0.000 00 0.000 01″ 0.000 04″ 1.110 30 c 0.000 00″ 0.000 00″ 0.000 00 0.000 01″ 0.000 01″ 0.408 03 d 0.000 00″ 0.000 00″ 0.000 00 0.000 00″ 0.000 01″ 0.266 62 e 0.000 00″ 0.000 01″ 0.266 62 0.000 00″ 0.000 02″ 0.533 24 f 0.000 00″ 0.000 00″ 0.000 00 0.000 01″ 0.000 01″ 0.408 03 表 5 直接进行相似变换的误差
Table 5 Error of Direct Similarity Transformation
检查点 共同点分布方案3 共同点分布方案4 ΔB/(″) ΔL/(″) ΔS/mm ΔB/(″) ΔL/(″) ΔS/mm a 0.248 68″ 0.921 97″ 25 753.375 20 1.225 04″ 0.829 51″ 43 827.730 84 b 0.294 18″ 1.094 65″ 30 567.107 40 1.133 21″ 0.767 29″ 40 541.901 01 c 0.109 98″ 0.413 15″ 11 527.248 66 0.319 26″ 0.216 14″ 11 421.510 73 d 0.005 48″ 0.016 18″ 463.412 84 0.106 95″ 0.072 40″ 3 826.070 49 e 0.051 94″ 0.193 96″ 5 414.491 58 0.470 28″ 0.318 41″ 16 824.655 35 f 0.010 86″ 0.039 82″ 1 113.412 30 0.311 45″ 0.210 88″ 11 142.495 10 表 6 基于过渡投影面进行相似变换的误差
Table 6 Error of Similarity Transformation Based on Transition Projecting Plane
检查点 共同点分布方案3 共同点分布方案4 ΔB/(″) ΔL/(″) ΔS/mm ΔB/(″) ΔL/(″) ΔS/mm a 0.000 01″ 0.000 00″ 0.308 88 0.000 03″ 0.000 03″ 1.224 09 b 0.000 01″ 0.000 01″ 0.408 03 0.000 02″ 0.000 03″ 1.010 63 c 0.000 00″ 0.000 00″ 0.000 00 0.000 01″ 0.000 01″ 0.408 03 d 0.000 00″ 0.000 00″ 0.000 00 0.000 00″ 0.000 01″ 0.266 62 e 0.000 00″ 0.000 00″ 0.000 00 0.000 01″ 0.000 02″ 0.616 24 f 0.000 00″ 0.000 00″ 0.000 00 0.000 01″ 0.000 00″ 0.308 88 -
[1] 张兰, 王光霞, 袁田, 等.室内地图研究初探[J].测绘与空间地理信息, 2013, 36(9):43-47 doi: 10.3969/j.issn.1672-5867.2013.09.012 Zhang Lan, Wang Guangxia, Yuan Tian, et al. Research on Indoor Map[J]. Geomatics & Spatial Information Technology, 2013, 36(9):43-47 doi: 10.3969/j.issn.1672-5867.2013.09.012
[2] 孙卫新, 王光霞, 张锦明, 等.源自建筑平面图的室内地图空间数据自动生成方法[J].测绘学报, 2016, 45(6):731-739 doi: 10.11947/j.AGCS.2016.20150658 Sun Weixin, Wang Guangxia, Zhang Jinming, et al.A Method of Generating Indoor Map Spatial Data Automatically from Architectural Plans[J]. Acta Geodaetica et Cartographica Sinica, 2016, 45(6):731-739 doi: 10.11947/j.AGCS.2016.20150658
[3] 周成虎, 朱欣焰, 王蒙, 等.全息位置地图研究[J].地理科学进展, 2011, 30(11):1331-1335 doi: 10.11820/dlkxjz.2011.11.001 Zhou Chenghu, Zhu Xinyan, Wang Meng, et al. Panoramic Location-Based Map[J]. Progress in Geography, 2011, 30(11):1331-1335 doi: 10.11820/dlkxjz.2011.11.001
[4] 朱庆, 熊庆, 赵君峤.室内位置信息模型与智能位置服务[J].测绘地理信息, 2014, 39(5):1-7 http://d.old.wanfangdata.com.cn/Periodical/chxxygc201405001 Zhu Qing, Xiong Qing, Zhao Junqiao. Indoor Location Information Model and Intelligent Location Service[J]. Journal of Geomatics, 2014, 39(5):1-7 http://d.old.wanfangdata.com.cn/Periodical/chxxygc201405001
[5] Nossum A S. Developing a Framework for Describing and Computing Indoor Maps[J]. The Cartographic Journal, 2013, 50(3):218-224 doi: 10.1179/1743277413Y.0000000055
[6] Nossum A S. Indoor Tubes a Novel Design for Indoor Maps[J]. Cartography and Geographic Information Science, 2011, 38(2):193-201 doi: 10.1559/15230406382192
[7] 游天, 周成虎, 陈曦.室内地图表示方法研究与实践[J].测绘科学技术学报, 2014, 31(6):635-640 http://www.irgrid.ac.cn/handle/1471x/995659 You Tian, Zhou Chenghu, Chen Xi. The Research and Practice of Indoor Map Representation[J]. Journal of Geomatics Science and Technology, 2014, 31(6):635-640 http://www.irgrid.ac.cn/handle/1471x/995659
[8] 许华燕, 李志辉, 王金月.室内地图应用现状及展望[J].测绘通报, 2014(9):119-121 http://www.docin.com/p-1526863159.html Xu Huayan, Li Zhihui, Wang Jinyue. Present Status and Prospect of Indoor Map Application[J]. Bulletin of Surveying and Mapping, 2014(9):119-121 http://www.docin.com/p-1526863159.html
[9] 李慧. 室内外融合导航系统的设计与实现[D]. 北京: 北京邮电大学, 2011 Li Hui. Design and Implementation of an Integrated Navigation System in Outdoor and Indoor[D]. Beijing: Beijing University of Posts and Telecommunications, 2011
[10] 宁津生.在统一的总体框架下建设"数字中国"[J].地理空间信息, 2004(2):1-2 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=dlkjxx200402001 Ning Jinsheng. The Construction of "Digital China" Under the Unified Overall Frame[J]. Spatial Information, 2004(2):1-2 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=dlkjxx200402001
[11] 毛锋. GIS中的空间基准问题[J].地理学与国土研究, 2002, 18(2):8-10 doi: 10.3969/j.issn.1672-0504.2002.02.003 Mao Feng. Some Problems on GIS Spatial Datum[J]. Geography and Territorial Research, 2002, 18(2):8-10 doi: 10.3969/j.issn.1672-0504.2002.02.003
[12] 王密, 龚健雅, 李德仁.大型遥感影像数据库的空间无缝数据组织[J].武汉大学学报·信息科学版, 2001, 26(5):419-424 http://ch.whu.edu.cn/CN/abstract/abstract5206.shtml Wang Mi, Gong Jianya, Li Deren. Spatial Seamless Data Organization of Large-scale Image Database[J]. Geomatics and Information Sicence of Wuhan University, 2001, 26(5):419-424 http://ch.whu.edu.cn/CN/abstract/abstract5206.shtml
[13] 刘宏林, 吕晓华, 江南, 等.影响地图投影多项式变换精度的若干因素分析[J].测绘科学技术学报, 2011, 28(2):108-112 http://kns.cnki.net/KCMS/detail/detail.aspx?filename=jfjc201102007&dbname=CJFD&dbcode=CJFQ Liu Honglin, Lv Xiaohua, Jiang Nan, et al. Analysis of Some Influencing Factors on Accuracy of Map Projective Polynomial Transformation[J]. Journal of Geomatics Science and Technology, 2011, 28(2):108-112 http://kns.cnki.net/KCMS/detail/detail.aspx?filename=jfjc201102007&dbname=CJFD&dbcode=CJFQ
[14] 杨启和.等面积投影的仿射变换及若干改良等面积投影的探求[J].测绘学报, 1992, 21(1):67-77 http://www.cnki.com.cn/Article/CJFDTOTAL-NMGD200003002.htm Yang Qihe. Affine Transformation of Equal Area Projections and some Discussion on Modified Equal Area Projection[J]. Acta Geodaetica et Cartographica Sinica, 1992, 21(1):67-77 http://www.cnki.com.cn/Article/CJFDTOTAL-NMGD200003002.htm
[15] 张剑清, 张祖勋.高分辨率遥感影像基于仿射变换的严格几何模型[J].武汉大学学报·信息科学版, 2002, 27(6):555-558 http://ch.whu.edu.cn/CN/abstract/abstract5009.shtml Zhang Jianqing, Zhang Zuxun. Strict Geometric Model Based on Affine Transformation for Remote Sensing Image with High Resolution[J]. Geomatics and Information Science of Wuhan University, 2002, 27(6):555-558 http://ch.whu.edu.cn/CN/abstract/abstract5009.shtml
[16] 郭金运, 朱明法, 徐泮林.地图数据几何纠正时仿射变换与相似变换的对比分析[J].测绘通报, 2001(4):23-24 http://d.wanfangdata.com.cn/Periodical_chtb200104010.aspx Guo Jinyun, Zhu Mingfa, Xu Panlin. Comparition Between the Affine Transformation and the Similarity Transformation and its Analysis[J]. Bulletin of Surveying and Mapping, 2001(4):23-24 http://d.wanfangdata.com.cn/Periodical_chtb200104010.aspx
[17] 李培华, 肖莉娟.基于Mean Shift的相似变换和仿射变换目标跟踪算法[J].中国图象图形学报, 2011, 16(2):258-266 http://kns.cnki.net/KCMS/detail/detail.aspx?filename=zgtb201102020&dbname=CJFD&dbcode=CJFQ Li Peihua, Xiao Lijuan. Mean Shift Based Object Tracking with Similarity and Affine Transformations[J]. Journal of Image and Graphics, 2011, 16(2):258-266 http://kns.cnki.net/KCMS/detail/detail.aspx?filename=zgtb201102020&dbname=CJFD&dbcode=CJFQ
[18] 吕晓华, 刘宏林.地图投影数值变换方法综合评述[J].测绘科学技术学报, 2002, 19(2):150-153 http://edu.wanfangdata.com.cn/Periodical/Detail/zgxxh201308039 Lv Xiaohua, Liu Honglin. A Comprehensive Appraisial of Numerical Transformation Method for Map Projection[J]. Journal of Geomatics Science and Technology, 2002, 19(2):150-153 http://edu.wanfangdata.com.cn/Periodical/Detail/zgxxh201308039
[19] 孙卫新, 马俊, 薛志伟, 等.高斯-克吕格投影反解的数值变换方法分析[J].测绘工程, 2013, 22(3):28-31 http://www.cqvip.com/QK/98521X/201303/46802277.html Sun Weixin, Ma Jun, Xue Zhiwei, et al. Analysis of Numerical Transformation of Gauss-Krüger Projection Reverse Transformation[J]. Engineering of Surveying and Mapping, 2013, 22(3):28-31 http://www.cqvip.com/QK/98521X/201303/46802277.html
[20] 胡安文, 张祖勋.对高分辨率遥感影像基于仿射变换的严格几何模型的讨论[J].武汉大学学报·信息科学版, 2006, 31(2):104-107 http://ch.whu.edu.cn/CN/abstract/abstract2378.shtml Hu Anwen, Zhang Zuxun. Discussion on "Strict Geometric Model Based on Affine Transformation for Remote Sensing Image with High Resolution"[J]. Geomatics and Information Science of Wuhan University, 2006, 31(2):104-107 http://ch.whu.edu.cn/CN/abstract/abstract2378.shtml
[21] 袁修孝, 付迎春, 张过, 等.多级空间信息网格间的平面坐标变换精度分析[J].武汉大学学报·信息科学版, 2005, 30(2):110-114 http://ch.whu.edu.cn/CN/abstract/abstract2130.shtml Yuan Xiuxiao, Fu Yingchun, Zhang Guo, et al. Accuracy Analysis of Planmetric Coordinate Transformation Between Spatial Information Multi-grids[J]. Geomatics and Information Science of Wuhan University, 2005, 30(2):110-114 http://ch.whu.edu.cn/CN/abstract/abstract2130.shtml
[22] 彭晓艳, 龙毅, 毋河海.基于仿射变换的MapInfo矢量图形坐标纠正的方法探讨[J].测绘信息与工程, 2002, 27(5):6-7 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=chxxygc200205003 Peng Xiaoyan, Long Yi, Wu Hehai. Coordinate Adjustment on Vector Graph in MapInfo Using Affine Transformation[J]. Journal of Geomatics, 2002, 27(5):6-7 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=chxxygc200205003
-
期刊类型引用(3)
1. 孙卫新,李鹤元,郑团结. 面向位置服务的室内外统一空间基准转换. 测绘工程. 2022(01): 35-39+51 .  百度学术
百度学术
2. 赵小凡,李情. 基于元数据管理的半结构化数据安全转换方法. 自动化仪表. 2022(03): 65-68+74 .  百度学术
百度学术
3. 谭金蓉,黄丽娜,肖屹,武光莹. 面向室内地图多尺度表达的POI符号设计与实现. 测绘地理信息. 2021(01): 83-87 .  百度学术
百度学术
其他类型引用(0)





 下载:
下载: