Influence of Horizontal Disturbing Gravity on Position Error in Inertial Navigation Systems
-
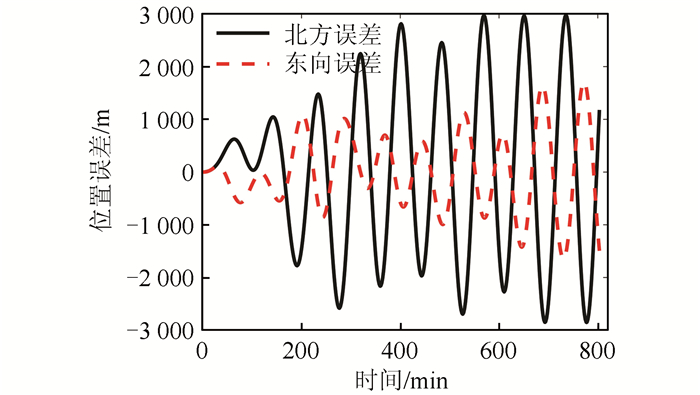
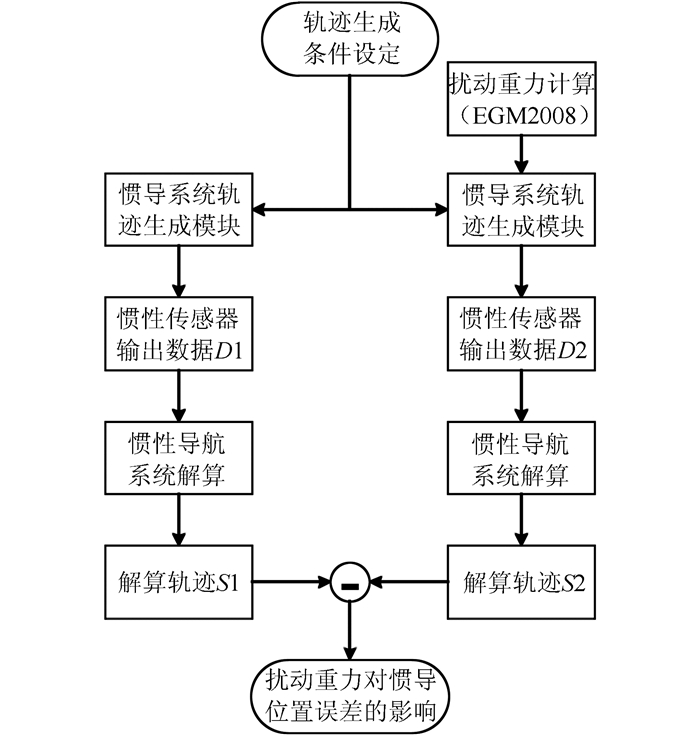
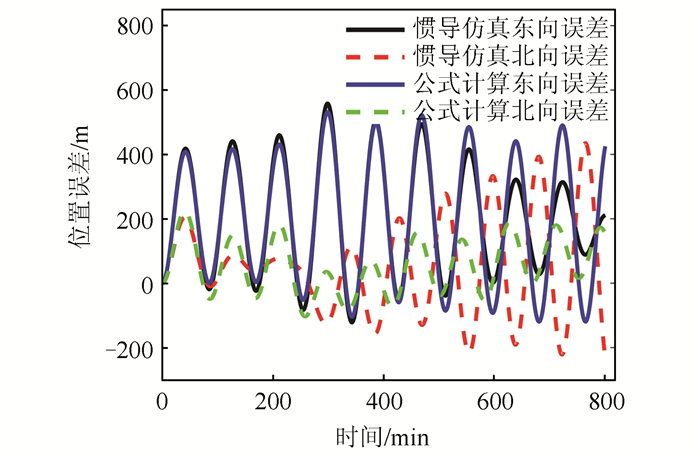
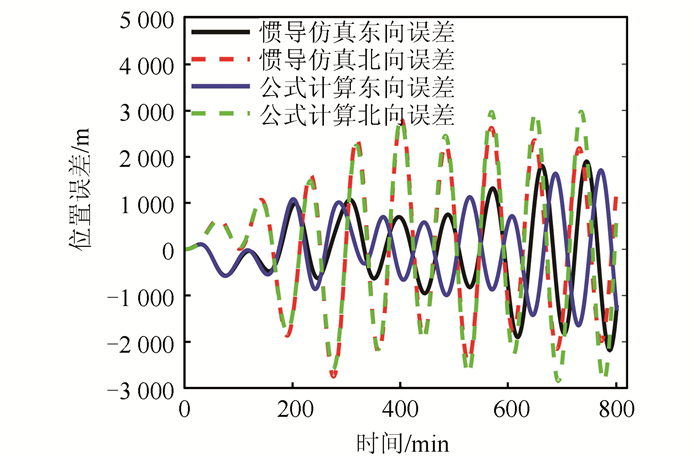
摘要: 研究了不同运动状态下扰动重力水平分量（HDG）对高精度惯导系统（inertial navigation system，INS）的位置误差影响。首先推导了HDG对INS误差影响的状态空间方程，进而推导出3种运动条件下INS位置误差与HDG之间的解析关系式，设计了基于惯导解算求解上述影响的方法。在匀速运动条件下，分别通过解析式与惯导解算两种方法计算了相同HDG引起的INS位置误差。解析式计算结果表明，±80 mGal（1 mGal=10-5 m/s2）范围内变化的HDG约可引起最大约3 000 m的INS位置误差；对两种方法计算结果的比较显示，所得INS位置误差的量级与变化情况基本一致，两组结果验证了各自方法的有效性。Abstract: The influence of horizontal disturbing gravity (HDG) on the positional error of high-precision Inertial Navigation Systems (INSs) was studied under different conditions of movement. A space-state equation expressing INS error with HDG was deduced and analytic expressions of INS position error with HDG were deduced under three conditions of movement. Additionally, a method of computing above influence based on inertial navigation calculation is designed. Under the condition of uniform motion, INS position error caused by the same HDG was calculated through analytic expressions and inertial navigation calculations. The results from analytic expressions show that disturbing gravity varied between ±80 mGal (1 mGal = 10-5 m/s2) causing about 3 000 m INS positional error at the maximum. A comparison of the results from two methods show that the level and change in INS positional error are basically consistent while the validity of each method was verified by these results.
-
Keywords:
- disturbing gravity /
- inertial navigation system /
- position error /
- acceleration /
- ultra-precise
-
-
![]()
图 5 通过惯导解算仿真HDG对INS的位置影响
Figure 5. Position Error of INS Caused by HDG Computed by Inertial Navigation Algorithm
![]()
图 6 海洋航线上两种方法计算的位置误差比较
Figure 6. Comparison of Position Error Computed by two Methods Along MarineRoute
![]()
图 7 陆地航线上两种方法计算的位置误差比较
Figure 7. Comparison of Position Error Computed by Two Methods Along Terrestrial Route
表 1 扰动重力对INS各误差量影响的传递函数
Table 1 Transfer Functions of Disturbing Gravity on Each Error Variation of INS
误差项 δgN(s) δgE(s) ψx(s) 0 $ - \frac{1}{{R\left( {{s^2} + \omega _s^2} \right)}} $ ψy(s) $ \frac{1}{{R\left( {{s^2} + \omega _s^2} \right)}} $ 0 ψz(s) 0 $ \frac{{\tan \varphi }}{{R\left( {{s^2} + \omega _s^2} \right)}} $ δvN(s) $ - \frac{s}{{\left( {{s^2} + \omega _s^2} \right)}} $ 0 δvE(s) 0 $ - \frac{s}{{\left( {{s^2} + \omega _s^2} \right)}} $ δφ(s) $ - \frac{1}{{R\left( {{s^2} + \omega _s^2} \right)}} $ 0 δλ(s) 0 $ - \frac{{\sec \varphi }}{{R\left( {{s^2} + \omega _s^2} \right)}} $ 
下载: 导出CSV
表 2 HDG及其引起位置误差的统计信息
Table 2 Statistics of HDG and Corresponding Position Error
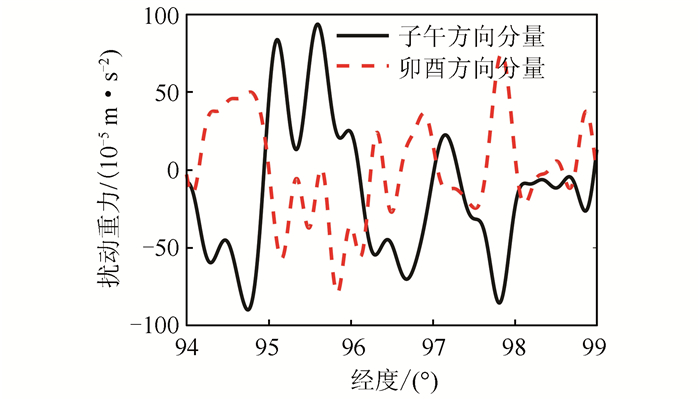
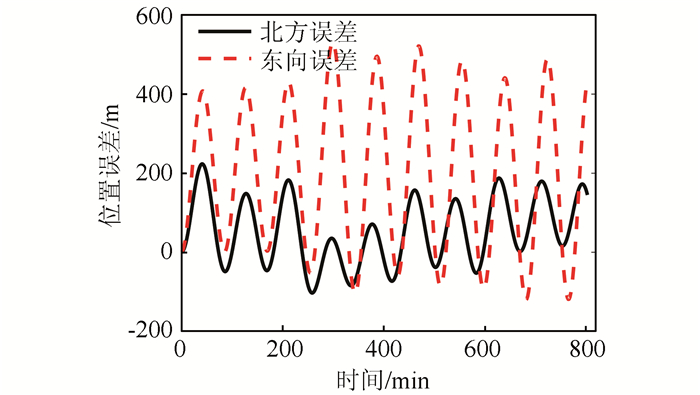
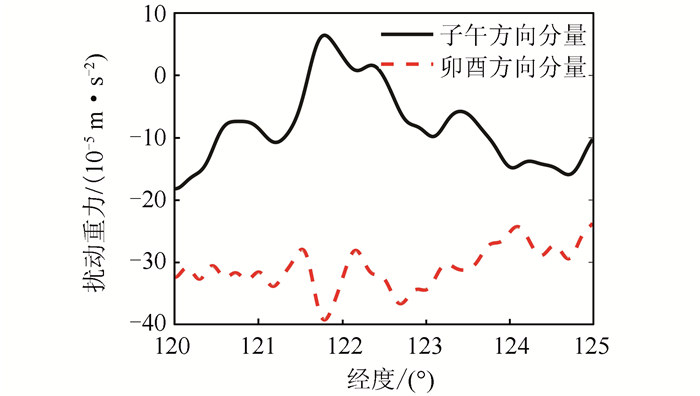
海洋航线 陆地航线 最大值 平均值 最大值 平均值 |δgN|/mGal 18.18 9.05 93.61 38.19 |δPN|/m 223.58 75.63 2 971.32 1 058.23 |δgE|/mGal 39.23 30.89 79.18 26.04 |δPE|/m 531.82 214.33 2583.78 787.55
下载: 导出CSV
表 3 惯性传感器仿真条件
Table 3 Simulation Condition of Inertial Instruments
条件1 条件2 条件3 陀螺零偏/((°)·h-1) 0.000 5 0.005 0.05 陀螺随机漂移/((°)·h-1, 1σ) 0.001 0.01 0.1 加速度计零偏/(m·s-2) 5×10-6 5×10-5 5×10-4 加计白噪声/(m·s-2, 1σ) 1×10-5 1×10-4 1×10-3
下载: 导出CSV
表 4 不同仿真条件下INS位置误差统计表
Table 4 Statistics of INS Position Error Under Different Simulation Conditions
|δPN|/m |δPE|/m 最大值 平均值 最大值 平均值 条件1 435.18 123.62 558.49 211.98 条件2 435.17 123.56 558.29 211.90 条件3 435.20 123.64 558.52 211.99
下载: 导出CSV
-
[1] Jekeli C, Lee J K, Kwon J H. Modeling Errors in Upward Continuation for INS Gravity Compensation[J]. Journal of Geodesy, 2007, (81): 297-309 doi: 10.1007/s00190-006-0108-y
[2] Jekeli C. Precision Free-inertial Navigation with Gravity Compensation by an Onboard Gradiometer[J]. Journal of Guidance, Control, and Dynamics, 2006, 29(3): 704-713 doi: 10.2514/1.15368
[3] Jordan S K. Effects of Geodetic Uncertainties on a Damped Inertial Navigation System[J]. IEEE Transactions on Aerospace and Electronic Systems, 1973, AES-9(5): 741-752 doi: 10.1109/TAES.1973.309774
[4] Vanderwerf K. Schuler Pumping of Inertial Velocity Errors due to Gravity Anomalies along a Popular North Pacific Airway[C]. The Position Location and Navigation Symposium, Atlanta, US, 1996
[5] Grejner-Brzezinska D A, Yi Yudan, Toth C, et al. On the Improved Gravity Compensation in Inertial Navigation[J]. Photogrammetric Engineering & Remote Sensing, 2004, 6(8): 663-664 http://stacks.iop.org/0957-0233/27/i=12/a=125007?key=crossref.0b2d510537feadd49c00d66ad3f73915
[6] Kwon J H, Jekeli C. Gravity Requirements for Compensation of Ultra-Precise Inertial Navigation[J]. The Journal of Navigation, 2005, 58(3): 479-492 doi: 10.1017/S0373463305003395
[7] 金际航, 边少锋.重力扰动对惯性导航系统的位置误差影响分析[J].武汉大学学报·信息科学版, 2010, 35(1): 30-32 http://ch.whu.edu.cn/CN/abstract/abstract819.shtml Jin Jihang, Bian Shaofeng. Analysis of Inertial Navigation System Positioning Error Caused by Gravity Disturbance[J]. Geomatics and Information Science of Wuhan University, 2010, 35(1): 30-32 http://ch.whu.edu.cn/CN/abstract/abstract819.shtml
[8] 李姗姗, 吴晓平, 王凯.扰动重力矢量对惯性导航系统的误差影响[J].大地测量与地球动力学, 2010, 30(3): 142-146 http://youxian.cnki.com.cn/yxdetail.aspx?filename=WHCH201710020&dbname=CJFDPREP Li Shanshan, Wu Xiaoping, Wang Kai. Error Effect of Disturbing Gravity Vector on Inertial Navigation System[J]. Journal of Geodesy and Geodynamics, 2010, 30(3): 142-146 http://youxian.cnki.com.cn/yxdetail.aspx?filename=WHCH201710020&dbname=CJFDPREP
[9] 卢鑫, 练军想, 吴美平.高精度舰载惯性导航系统的重力影响研究[J].导航与控制, 2010, 9(4): 15-21 http://dhykz.zghtqk.com/ch/reader/view_abstract.aspx?file_no=201004004&flag=1 Lu Xin, Lian Junxiang, Wu Meiping. Research of Gravity Error Compensation in Marine Inertial Navigation System[J]. Navigation and Control, 2010, 9(4): 15-21 http://dhykz.zghtqk.com/ch/reader/view_abstract.aspx?file_no=201004004&flag=1
[10] 尧颖婷, 沈晓蓉, 邹尧, 等.捷联惯性导航系统重力扰动影响分析[J].大地测量与地球动力学, 2011, 31(6): 159-163 http://www.cnki.com.cn/Article/CJFDTOTAL-DKXB201106037.htm Yao Yingting, Shen Xiaorong, Zou Yao, et al. Analysis of Gravity Disturbance Influence on Strapdown Inertial Navigation System[J]. Journal of Geodesy and Geodynamics, 2011, 31(6): 159-163 http://www.cnki.com.cn/Article/CJFDTOTAL-DKXB201106037.htm
[11] 李卓, 闫海蛟.中国海及邻域重力场异常的惯导系统误差分析[J].青岛大学学报(自然科学版), 2004, 17(1): 20-25 http://www.cnki.com.cn/Article/CJFDTOTAL-QDDD200401005.htm Li Zhuo, Yan Haijiao. INS Error Analysis of China Sea and Its Adjacent Regions Based on Gravitation Field Anomaly[J]. Journal of Qingdao University (Natural Science Edition), 2004, 17(1): 20-25 http://www.cnki.com.cn/Article/CJFDTOTAL-QDDD200401005.htm
[12] 陈永冰, 边少锋, 刘勇.重力异常对平台式惯性导航系统误差的影响分析[J].中国惯性技术学报, 2005, 13(6): 21-25 http://www.cnki.com.cn/Article/CJFDTOTAL-ZGXJ200506005.htm Chen Yongbing, Bian Shaofeng, Liu Yong. Analysis for Gravity Anomaly's Influence on Platform INS Error[J]. Journal of Chinese Inertial Technology, 2005, 13(6): 21-25 http://www.cnki.com.cn/Article/CJFDTOTAL-ZGXJ200506005.htm
[13] 郭恩志, 房建成, 俞文伯.一种重力异常对弹道导弹惯性导航精度影响的补偿方法[J].中国惯性技术学报, 2005, 13(3): 30-33. http://www.cnki.com.cn/Article/CJFDTOTAL-ZGXJ200503007.htm Guo Enzhi, Fang Jiancheng, Yu Wenbo. Compensation for Errors of Ballistic Missile Caused by Gravity Abnormality[J]. Journal of Chinese Inertial Technology, 2005, 13(3): 30-33 http://www.cnki.com.cn/Article/CJFDTOTAL-ZGXJ200503007.htm
[14] 李斐, 束蝉方, 陈武.高精度惯性导航系统对重力场模型的要求[J].武汉大学学报·信息科学版, 2006, 31(6): 508-511 http://ch.whu.edu.cn/CN/abstract/abstract2479.shtml Li Fei, Shu Chanfang, Chen Wu. The Requirement of Gravity Model for INS with High Precision[J]. Geomatics and Information Science of Wuhan University, 2006, 31(6): 508-511 http://ch.whu.edu.cn/CN/abstract/abstract2479.shtml
[15] 吴太旗, 边少锋, 蒋勃, 等.重力场对惯性导航定位误差影响研究与仿真[J].测绘科学技术学报, 2006, 23(5): 341-344 http://www.cnki.com.cn/Article/CJFDTOTAL-JFJC200605008.htm Wu Taiqi, Bian Shaofeng, Jiang Bo, et al, The Influence of Gravity Field on INS Positioning Error and its Simulation[J]. Journal of Zhengzhou Institute of Surveying and Mapping, 2006, 23(5): 341-344 http://www.cnki.com.cn/Article/CJFDTOTAL-JFJC200605008.htm
[16] 李胜全, 欧阳永忠, 常国宾, 等.惯性导航系统重力扰动矢量补偿技术[J].中国惯性技术学报, 2012, 20(4): 410-413 http://www.cnki.com.cn/Article/CJFDTOTAL-ZGXJ201204008.htm Li Shengquan, Ouyang Yongzhong, Chang Guobin, et al. Compensation Technology of Gravity Disturbance Vector in Inertial Navigation System[J]. Journal of Chinese Inertial Technology, 2012, 20(4): 410-413 http://www.cnki.com.cn/Article/CJFDTOTAL-ZGXJ201204008.htm
[17] Wang Hao, Xiao Xuan, Deng Zhihong, et al. The Influence of Gravity Disturbance on High-precision Long-time INS and Its Compensation Method[C]. Fourth International Conference on Instrumentation and Measurement, Computer, Communication and Control, Harbin, China, 2014
[18] Wang Jing, Yang Gongliu, Li Xiangyun, et al. Research on Time Interval of Gravity Compensation for Airborne INS[C]. The 34th Chinese Control Conference, Hangzhou, China, 2015
[19] Fang Jiancheng, Chen Linzhouting, Yao Jifeng. An Accurate Gravity Compensation Method for High-Precision Airborne POS[J]. IEEE Transactions on Geoscience and Remote Sensing, 2014, 52(8): 4 564-4 573 doi: 10.1109/TGRS.2013.2282423
[20] Titterton D H, Weston J L. Strapdown Inertial Navigantion Technology[M]. Lexington, Massachusetts, USA: American Institute of Aeronautics and Astronautics, Inc, 2004
[21] 赵忠, 王鹏.高精度惯性导航系统垂线偏差影响与补偿[J].中国惯性技术学报, 2013, 21(6): 701-705 http://www.cnki.com.cn/Article/CJFDTOTAL-ZGXJ201306001.htm Zhao Zhong, Wang Peng. Analysis and Compensation of Vertical Deflection Effect on High Accuracy Inertial Navigation System[J]. Journal of Chinese Inertial Technology, 2013, 21(6): 701-705 http://www.cnki.com.cn/Article/CJFDTOTAL-ZGXJ201306001.htm
[22] Pavlis N K, Holmes S A, Kenyon S C, et al. The Development and Evaluation of the Earth Gravitational Model 2008 (EGM2008)[J]. Journal of Geophysical Research: Solid Earth (1978-2012), 2012, 117(B4) http://adsabs.harvard.edu/abs/2012JGRB..117.4406P
[23] Jekeli C. Inertial Navigation Systems with Geodetic Applications[M]. Berlin, Germany: Walter de Gruyter, 2001:51-53
-
期刊类型引用(11)
1. 赵涛，叶世榕，罗歆琪，夏朋飞. GNSS-IR潮位反演中高仰角数据质量控制方法. 武汉大学学报(信息科学版). 2024(01): 68-76 . 
百度学术
2. 肖倩雨，周春霞，刘勇. 利用改进的亮温日较差法探测格陵兰冰盖表面融化. 武汉大学学报(信息科学版). 2024(10): 1931-1939 . 百度学术
3. 李荣兴，何美茜，葛绍仓，程远，安璐. 东南极历史冰流速过估改正. 武汉大学学报(信息科学版). 2023(10): 1661-1669 . 百度学术
4. 张冕，张春灌，赵敏，钟振华，袁炳强，周磊，韩梅. 地球磁异常EMAG2v3与全球重力数据库V29数据质量综合评估——以北极地区Aegir脊为例. 物探与化探. 2023(06): 1410-1416 . 百度学术
5. 张金辉，李姗姗，杨光，范雕，凌晴. 联合CTD、海底地形和ARGO数据构建北太平洋深海时变温度模型. 测绘通报. 2023(12): 94-101+126 . 百度学术
6. 徐天河，穆大鹏，闫昊明，郭金运，尹鹏. 近20年海平面变化成因研究进展及挑战. 测绘学报. 2022(07): 1294-1305 . 百度学术
7. 徐天河，杨元元，穆大鹏，尹鹏. 近海海平面变化成因分析. 武汉大学学报(信息科学版). 2022(10): 1750-1757 . 百度学术
8. 陈旭升，张云龙，张冠军. 优化局部均值分解在趋势信息提取中的应用. 测绘科学. 2022(11): 32-39 . 百度学术
9. 房婷婷，付广裕. 卫星重力与地球重力场的文献计量分析. 地球科学进展. 2021(05): 543-552 . 百度学术
10. 冯哲颖，岳林蔚，沈焕锋. 基于多源水文数据融合的GRACE水储量精度校正. 遥感技术与应用. 2021(03): 605-617 . 百度学术
11. 刘冰石，邹贤才. ENSO影响下的西太平洋地区海陆水储量变化分析. 武汉大学学报(信息科学版). 2019(09): 1296-1303 . 百度学术
其他类型引用(11)
计量
- 文章访问数: 1709
- HTML全文浏览量: 227
- PDF下载量: 259
- 被引次数: 22
