-
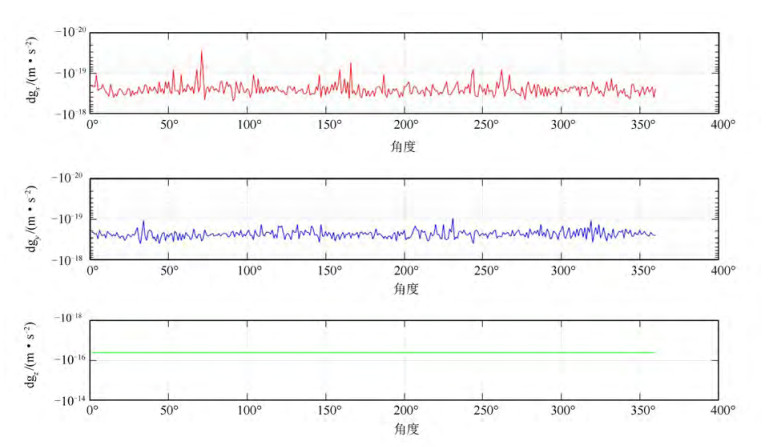
摘要: 基于$\frac{{{{\bar{P}}}_{nm}}\left( \cos \theta \right)}{\sin \theta }\left( m>0 \right)$的非奇异递推公式,给出了基于球坐标的引力矢量和垂线偏差非奇异计算公式;针对极点λ可任意取值引起的地方指北坐标系方向的不确定性问题,证明了引力矢量在转换到地心直角坐标系后不随λ的变化而变化,即与λ的取值无关。最终的数值计算结果表明,直角坐标系下的非奇异计算公式与本文提出的球坐标下的非奇异计算公式所得计算结果绝对值差异小于10-16m/s2,证明了该非奇异公式的正确性。最后总结了所有引力位球函数一阶导、二阶导非奇异性计算的一般思路。Abstract: When computing gravity vector and vertical deviation using spherical harmonic function, singular problem exists in the formulas expressed by spherical coordinates. This will cause some errors in gravity vector and vertical deflection data and influence their application. This paper aims at proposing an alternative method to solve this problem. Based on the non-singular expression of $ \frac{{{{\bar{P}}}_{nm}}\left( \cos \theta \right)}{\sin \theta } $(m > 0), the paper gives the non-singular formulas expressed by spherical coordinates for computing gravity vector and vertical deviation. At North and South Poles, the paper proves that even values of λ are arbitrary, the values of gravity vector are sole when the values are transferred to Earth fixed rectangular coordinate system. In order to show the validity of our method, the paper computes gravity vectors at points θ=0, λ=$ \frac{i}{360} $2π(i=0, 1...359) using the former 100 degrees and orders of EGM2008. The absolute differences between the computing results by our method and the non-singular formula expressed in Cartesian coordinates are smaller than 10-16 m/s2, which show the validity of our method. The non-singular expression based on spherical function derived by the paper can make full use of the high accurate algorithms of Legendre function, so the proposed method has better generality ability compared with the non-singular formula expressed by Cartesian coordinates. Finally the methods for non-singular computing of all the first or second derivations of gravity field potential are summarized. The method of this paper can also be directly applied to the non-singular calculation of the spherical harmonic model of magnetic field, and the basic idea is similar to that of this paper.
-
Keywords:
- gravity /
- vertical deviation /
- North and South Poles /
- non-singular /
- spherical coordinates
-
-
[1] Cunningham L. On the Computation of the Spherical Harmonic Terms Needed During the Numerical Integration of the Orbital Motion of an Artificial Satellite[J]. Celestial Mechanics, 1970, 2:207-216 doi: 10.1007/BF01229495
[2] 王正涛. 卫星跟踪卫星测量确定地球重力场的理论与方法[D]. 武汉: 武汉大学, 2005 http://cdmd.cnki.com.cn/Article/CDMD-10486-2006031327.htm Wang Zhengtao. Theory and Methodology of Earth Gravity Field Recovery by Satellite-to-Satellite Tracking Data[D]. Wuhan:Wuhan University, 2005 http://cdmd.cnki.com.cn/Article/CDMD-10486-2006031327.htm
[3] 邹贤才. 卫星轨道理论与地球重力场模型的确定[D]. 武汉: 武汉大学, 2007 Zou Xiancai. Theory of Satellite Orbit and Earth Gravity Field Determination[D]. Wuhan:Wuhan University, 2005
[4] 钟波. 基于GOCE卫星重力测量技术确定地球重力场的研究[D]. 武汉: 武汉大学, 2010 http://cdmd.cnki.com.cn/Article/CDMD-10486-2010167102.htm Zhong Bo. Study on the Determination of the Earth's Gravity Field from Satellite Gravimetry Mission GOCE[D]. Wuhan:Wuhan University, 2010 http://cdmd.cnki.com.cn/Article/CDMD-10486-2010167102.htm
[5] Yi Weiyong. An Alternative Computation of a Gravity Field Model from GOCE[J]. Advances in Space Research, 2012, 50(3):371-384 doi: 10.1016/j.asr.2012.04.018
[6] Holmes S, Featherstone W. A Unified Approach to the Clenshaw Summation and the Recursive Computation of very High Degree and Order Normalised Associated Legendre Functions[J]. Journal of Geodesy, 2002, 76(5):279-299 doi: 10.1007/s00190-002-0216-2
[7] Yu Jinhai, Wan Xiaoyun, Zeng Yanyan. The Integral Formulas for Legendre Functions and Associated Legendre Functions[J]. Journal of Geodesy, 2011, DOI: 10.1007/s00190-011-0529-0
[8] 吴星, 刘雁雨.多种超高阶次缔合勒让德函数计算方法的比较[J].测绘科学技术学报, 2006, 23(3):188-191 http://www.docin.com/p-885217372.html Wu Xing, Liu Yanyu. Comparison of Computing Methods of the Ultra-High Degree and Order[J]. Journal of Geomatics Science and Technology, 2006, 23(3):188-191 http://www.docin.com/p-885217372.html
[9] 魏子卿.完全正常化缔合勒让德函数及其导数与积分的递推关系[J].武汉大学学报·信息科学版, 2016, 41(1):27-36 http://ch.whu.edu.cn/CN/abstract/abstract3430.shtml Wei Ziqing. Recurrence Relations for Fully Normalized Associated Legendre Functions and Their Derivatives and Integrals[J]. Geomatics and Information Science of Wuhan University, 2016, 41(1):27-36 http://ch.whu.edu.cn/CN/abstract/abstract3430.shtml
[10] Casotto S, Fantino E. Evaluation of Methods for Spherical Harmonic Synthesis of the Gravitational Potential and its Gradients[J]. Advances in Space Research, 2007, 40(1):69-75 doi: 10.1016/j.asr.2007.01.021
[11] Fantino E, Casotto S. Methods of Harmonic Synthesis for Global Geopotential Models and Their First-, Second-and Third-Order Gradients[J]. Journal of Geodesy. 2009, 83(7):595-619 doi: 10.1007/s00190-008-0275-0
[12] Casotto S, Fantino E. Gravitational Gradients by Tensor Analysis with Application to Spherical Coordinates[J]. Journal of Geodesy, 2009, 83(7):621-634 doi: 10.1007/s00190-008-0276-z
[13] Tscherning C. Computation of Second-Order Deriv-atives of the Normal Potential Based on the Repre-sentation by a Legendre Series[J]. Manuscr Geod, 1976, 1:71-92 http://www.researchgate.net/publication/259055140_Computation_of_second-order_derivatives_of_the_normal_potential_based_on_a_representation_by_a_Legendre_series
[14] Balmino G, Barrriot J, Vales N. Non-singular Formulation of the Gravity Vector and Gravity Gradient Tensor in Spherical Harmonics[J]. Manuscr Geod, 1989, 15(1):11-16 http://www.researchgate.net/publication/241346652_Nonsingular_formulation_of_the_gravity_vector_and_gravity_gradient_tensor_in_spherical_harmonics
[15] Petrovskaya M, Vershkov A. Non-singular Expressions for the Gravity Gradients in the Local North-Oriented and Orbital Reference Frames[J]. Journal of Geodesy, 2006, 80(3):117-127 doi: 10.1007/s00190-006-0031-2
[16] 于锦海, 万晓云.计算Legendre函数导数的非奇异方法[J].测绘科学技术学报, 2010, 27(1):1-3 http://kns.cnki.net/KCMS/detail/detail.aspx?filename=jfjc201001004&dbname=CJFD&dbcode=CJFQ Yu Jinhai, Wan Xiaoyun. Non-singular Formulae for Computing Derivatives of Legendre Functions[J]. Journal of Geomatics Science and Technology, 2010, 27(1):1-3 http://kns.cnki.net/KCMS/detail/detail.aspx?filename=jfjc201001004&dbname=CJFD&dbcode=CJFQ
[17] 刘晓刚, 吴晓平, 赵东明, 等.扰动重力梯度的非奇异表示[J].测绘学报, 2010, 39(5):450-457 http://www.cnki.com.cn/Article/CJFDTotal-CHXB201005005.htm Liu Xiaogang, Wu Xiaoping, Zhao Dongming, et al. Non-singular Expression of the Disturbing Gravity Gradients[J]. Acta Geodaetica et Cartographica Sinica, 2010, 39(5):450-457 http://www.cnki.com.cn/Article/CJFDTotal-CHXB201005005.htm
[18] 刘晓刚. GOCE卫星测量恢复地球重力场模型的理论与方法[D]. 郑州: 信息工程大学, 2011 http://cdmd.cnki.com.cn/article/cdmd-90008-1012325190.htm Liu Xiaogang. Theory and Methods of the Earth's Gravity Field Model Recovery from GOCE Data[D]. Zhengzhou:Information Engineering University, 2011 http://cdmd.cnki.com.cn/article/cdmd-90008-1012325190.htm
[19] 万晓云.引力场梯度张量的非奇异公式推导[J].武汉大学学报·信息科学版, 2011, 36(12):1486-1489 http://ch.whu.edu.cn/CN/abstract/abstract732.shtml Wan Xiaoyun. New Derivation of Non-singular Expression for Gravitational Gradients Calculation[J]. Geomatics and Information Science of Wuhan University, 2011, 36(12):1486-1489 http://ch.whu.edu.cn/CN/abstract/abstract732.shtml
[20] 海斯卡涅, 莫里兹. 卢福康, 胡国理, 译. 物理大地测量学[M]北京; 测绘出版社, 1979 Heiskanen W A, Moritz H. Lu Fukang, Hu Guoli, Trans.Physical Geodesy[M]. Beijing:Surveying Press, 1979
[21] Oliver M, Eberhard G. Satellite Orbits:Models, Methods, and Applications[M]. Berlin:Springer, 2001
-
期刊类型引用(4)
1. 朱杰,郑加柱,陈红华,杨静,胡平昌,陆敏燕. 结合POI数据的南京市商业中心识别与集聚特征研究. 现代测绘. 2022(06): 34-39 .  百度学术
百度学术
2. 金澄,安晓亚,陈占龙,马啸川. 矢量居民地多边形多级图划分聚类方法. 武汉大学学报(信息科学版). 2021(01): 19-29 .  百度学术
百度学术
3. 张铭龙,何贞铭. 基于因子分析法的城市商业中心抽取研究. 地理空间信息. 2021(08): 58-60+64+5 .  百度学术
百度学术
4. 李卫东,张铭龙,段金龙. 基于POI数据的南京市空间格局定量研究. 世界地理研究. 2020(02): 317-326 .  百度学术
百度学术
其他类型引用(3)





 下载:
下载:


