Impact of Sample Rate of GPS Satellite Clock and Observation Data on LEO GPS-Based Precise Orbit Determination
-
摘要: 针对GPS卫星钟差及观测数据间隔对LEO卫星运动学和约化动力学定轨的影响问题进行了分析，并使用CODE（the Center for Orbit Determination in Europe）30 s、5 s间隔GPS卫星钟差分别进行了30 s和10 s间隔观测数据的LEO卫星定轨实验。结果表明，使用5 s间隔卫星钟差（10 s间隔观测数据）相比30 s间隔卫星钟差（30 s间隔观测数据）进行GRACE卫星精密定轨，约化动力学定轨精度提高了16%，运动学定轨精度提高了8.8%；使用30 s间隔卫星钟差和10 s间隔观测数据的定轨精度最低；对于30 s间隔观测数据，使用30 s或5 s间隔卫星钟差的定轨精度基本一致。Abstract: The influence of GPS precise clock and observation data sampling internal on LEO kinematic and reduced-dynamic precise orbit determination (POD) was analyzed. The several experiments of LEO POD were carried out respectively by using the 30 s or 5 s sampling interval GPS precise clock from CODE and the 30 s or 10 s GPS observation data. The results show that 5 s GPS clock and 10 s observation data comparing with 30 s clock and 30 s observation data, the accuracy of reduced-dynamic POD is improved by 16%, and the kinematic POD is 8.8%. The accuracy of LEO POD is the worst by using 10 s observation data and 30 s GPS clock. For 30 s observation data, the accuracy of LEO POD by using 30 s GPS clock is consistent with 5 s GPS clock products from CODE.
-
-
![]()
图 1 G05和G24卫星30 s钟差内插到5 s残差
Figure 1. Error of G05 and G24 Clock Interpolation from 30 s to 5 s Clock Products
![]()
图 2 2014年年积日305~334 d定轨结果与JPL参考轨道互差RMS
Figure 2. RMS of Residuals Between Precise Orbits and JPL Published Reference Orbits for DOY 305~334, 2014
![]()
图 3 2014年年积日305~334的运动学与约化动力学定轨结果的互差RMS图
Figure 3. RMS of Residuals Between Kinematic Orbits and Reduced-Dynamic Orbits for DOY 305~334, 2014
表 1 GRACE约化动力学定轨观测模型和力学模型
Table 1 Reduced-Dynamic POD Observation and Force Model for GRACE
类别 详细描述 观测量 无电离层组合L3 GPS卫星高度截止角 5° GPS卫星轨道及钟差 CODE最终轨道及5 s采样间隔钟差产品 载波相位模糊度 作为未知参数估计 接收机钟差 历元待估参数 GPS卫星和接收机天线相位中心改正 考虑 地球重力场模型 EGM2008 120×120 N体摄动力 太阳、月球以及其他行星摄动(JPL DE450) 潮汐摄动 固体潮、极潮及海洋潮汐 太阳光压和大气阻力 通过经验加速度参数吸收 卫星轨道状态参数 6个初始轨道根数+ 3个常数经验加速度参数 经验加速度 每6 min估计一组 
下载: 导出CSV
表 2 GRACE卫星精密定轨实验方案
Table 2 POD Experimental Program for GRACE
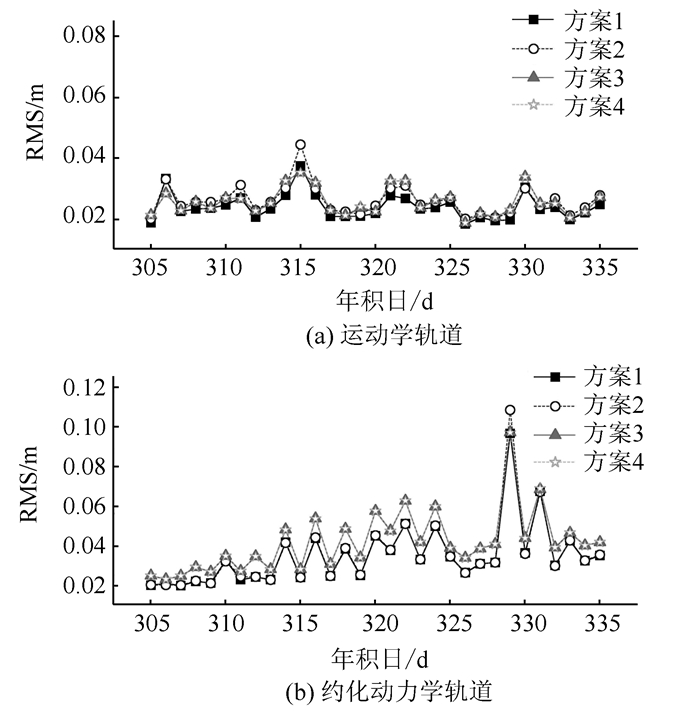
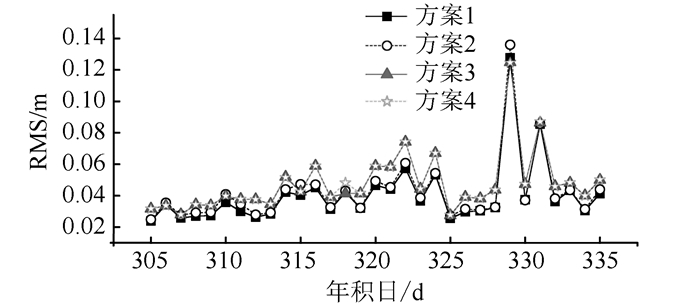
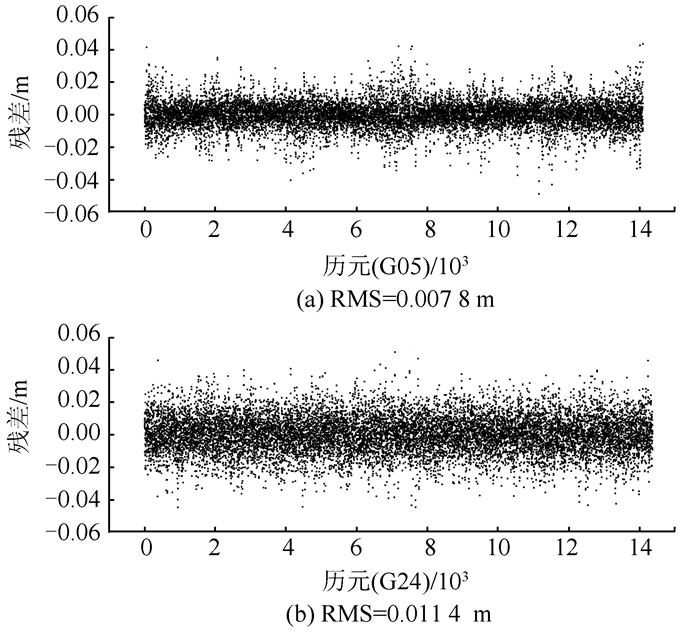
方案 GPS观测数据采样间隔/s GPS钟差采样间隔/s 1 10 5 2 10 30 3 30 5 4 30 30
下载: 导出CSV
表 3 定轨结果与JPL参考轨道差值RMS的均值/m
Table 3 RMS Mean Between Precise Orbits and JPL Published Reference Orbits for DOY 305~334, 2014/m
轨道 方案1 方案2 方案3 方案4 运动学轨道 0.024 7 0.027 7 0.027 1 0.027 1 约化动力学轨道 0.035 0 0.035 5 0.041 8 0.041 8
下载: 导出CSV
-
[1] Melbourne W G, Davis E S, Yunck T P, et al. The GPS Flight Experiment on TOPEX/POSEIDON[J]. Geophysical Research Letters, 1994, 21(19):2171-2174 doi: 10.1029/94GL02192
[2] Haines B, Bertiger W, Desai S, et al. Initial Orbit Determination Results for Jason-1:Towards a 1 cm Orbit[J].Navigation, 2003, 50(3):171-180 doi: 10.1002/navi.2003.50.issue-3
[3] Svehla D, Rothacher M. Kinematic and Reduced-Dynamic Precise Orbit Determination of Low Earth Orbiters[J]. Advances in Geosciences, 2003, 1:47-56 doi: 10.5194/adgeo-1-47-2003
[4] Kang Z, Tapley B, Bettadpur S, et al. Precise Orbit Determination for the GRACE Mission Usingonly GPS Data[J]. Journal of Geodesy, 2006, 80(6):322-331 doi: 10.1007/s00190-006-0073-5
[5] Bock H, Jaggi A, Svehla D, et al. Precise Orbit Determination for the GOCE Satellite Using GPS[J]. Advances in Space Research, 2007, 39(10):1638-1647 doi: 10.1016/j.asr.2007.02.053
[6] 赵齐乐, 刘经南, 葛茂荣, 等.用PANDA对GPS和CHAMP卫星精密定轨[J].大地测量与地球动力学, 2005, 25(2):113-116 doi: 10.3969/j.issn.1671-5942.2005.02.020 Zhao Qile, Liu Jingnan, Ge Maorong, et al.Precise Orbit Determination of GPS and CHAMP Satellites with PANDA Software[J].Journal of Geodesy and Geodynamics, 2005, 25(2):113-116 doi: 10.3969/j.issn.1671-5942.2005.02.020
[7] Dow J M, Neilan R E, Rizos C. The International GNSS Service in a Changing Landscape of Global Navigation Satellite Systems[J]. Journal of Geodesy, 2008, 83(3-4):191-198 doi: 10.1007/s00190-008-0300-3
[8] Montenbruck O, Gill E, Kroes R. Rapid Orbit Determination of LEO Satellites Using IGS Clock and Ephemeris Products[J]. GPS Solutions, 2005, 9(3):226-235 doi: 10.1007/s10291-005-0131-0
[9] Hugentobler U. CODE High Rate Clocks. IGS Mail No. 4913[OL]. igscb.jpl.nasa.gov/mail/igsmail/2004/msg00136.html, 2004
[10] Gendt G. Combined IGS Clocks with 30 Second Sampling Rate. IGS Mail No. 55253[OL]. igscb.jpl.nasa.gov/mail/igsmail/2007/msg00003.html, 2006
[11] Bock H, Dach R, Jaggi A, et al. High-Rate GPS Clock Corrections from CODE:Support of 1Hz Applications[J].Journal of Geodesy, 2009, 83(11):1083-1094 doi: 10.1007/s00190-009-0326-1
[12] Schaer S, Dach R. Model Changes Made at CODE. IGS Mail No.5771[OL]. http://igscb.jpl.nasa.gov, 2008
[13] Allan D W. Time and Frequency (Time-Domain) Characterization, Estimation, and Prediction of Precision Clocks and Oscillators[J].IEEE Transactions on Ultrasonics Ferroelectrics & Frequency Control, 1987, 34(6):647-654 https://www.researchgate.net/publication/5560127_Time_and...
[14] Case K, Kruizinga G L H, Wu S C. GRACE Level 1b Data Product User Handbook, JPL D-22027[OL]. http://podaac.jpl.nasa.gov/grace/documentation.html, 2004
[15] 田英国, 郝金明, 刘伟平, 等.利用KBR数据检核GRACE卫星精密轨道[J].测绘科学技术学报, 2014, 31(6):580-583 http://www.cqvip.com/QK/98086A/201406/663713483.html Tian Yingguo, Hao Jinming, Liu Weiping, et al.Checking the GRACE Satellite Orbit Precision Using the KBR Data[J]. Journal of Geomatics Science and Technology, 2015, 31(6):580-583 http://www.cqvip.com/QK/98086A/201406/663713483.html
[16] 秦显平, 焦文海, 程芦颖, 等.利用SLR检核CHAMP卫星轨道[J].武汉大学学报·信息科学版, 2005, 30(1):38-41 http://ch.whu.edu.cn/CN/abstract/abstract2075.shtml Qin Xianping, Jiao Wenhai, Cheng Luying, et al. Evaluation of CHAMP Satellite Orbit with SLR Measurements[J]. Geomantic and Information Science of Wuhan University, 2005, 30(1):38-41 http://ch.whu.edu.cn/CN/abstract/abstract2075.shtml
[17] Jaggi A. Pseudo-Stochastic Orbit Modeling Low Earth Satellites Using the Global Positioning System[D]. Schwitzerland:Astronomical Institute University of Berne, 2007 https://boris.unibe.ch/25278/
[18] 周晓青, 胡志刚, 张新远.低轨卫星星载GNSS精密定轨的精度检核方法[J].武汉大学学报·信息科学版, 2010, 35(11):1342-1345 http://ch.whu.edu.cn/CN/abstract/abstract1109.shtml Zhou Xiaoqing, Hu Zhigang, Zhang Xinyuan.Discussion on POD Accuracy Evaluations for Satellite-Bone LEO Satellites[J]. Geomantic and Information Science of Wuhan University, 2010, 35(11):1342-1345 http://ch.whu.edu.cn/CN/abstract/abstract1109.shtml
计量
- 文章访问数: 2295
- HTML全文浏览量: 161
- PDF下载量: 429
