Performance Assessment of Partial Ambiguity Resolution Based on BDS/GPS Combined Positioning
-
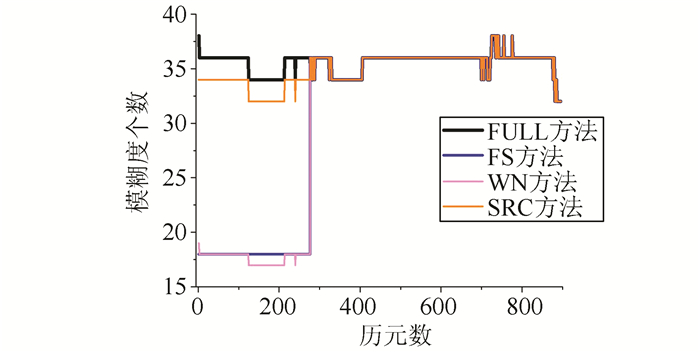
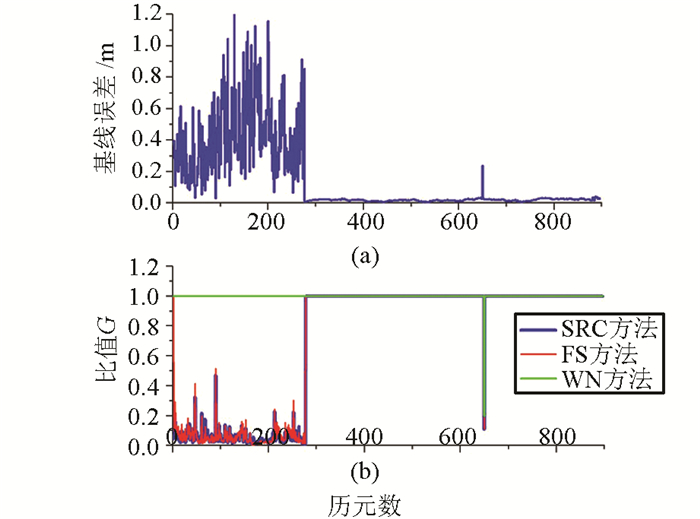
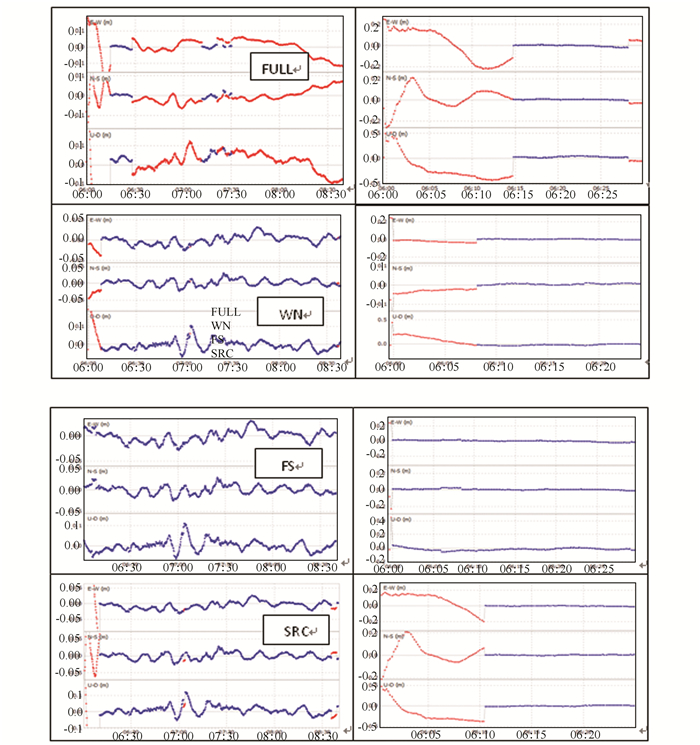
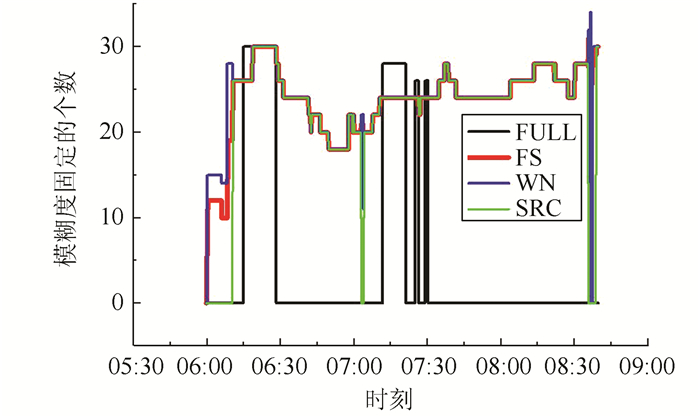
摘要: 多系统组合有利于提高卫星导航定位的精度及可靠性，然而对于载波差分定位由于模糊度维数的陡增、观测噪声、大气残余误差等原因用传统的Lambda方法很难得到所有模糊度的固定解，采用部分模糊度方法固定最优的模糊度子集则相对容易。总结了现有的部分模糊度固定方法，分析了不同方法的特点，并用实测数据分析了BDS/GPS组合动态定位时部分模糊度固定的效果。实验结果表明，部分模糊度方法可以显著提高模糊度固定时的成功率及Ratio值，并且可以缩短RTK定位时的初始化时间，加快坐标的收敛速度，提高组合系统动态定位结果的精度。Abstract: Multi-GNSS combination is helpful to improve the accuracy and relibality of satellite navigation and positioning. However, in case of BDS/GPS combined carrier phase positioning systems, it is difficult to fix all the ambiguities due to the increasing number of ambiguities, high measurement noises or residual atmosphere delays with the traditional Lambda method. But it is of greater probability to fix a subset of ambiguities. In this paper, we divided current partial ambiguity fixing methods into three categories and analyzed the characteristics of every method. Finally, the effect of the three partial resolution methods were tested with the measured BDS/GPS data. The results show that the success rate and ratio value is obviously improved when using partial ambiguity fixing, at the same time, the initialization time of RTK is shortened, the precision of kinematic positioning is also improved.
-
Keywords:
- partial ambiguity resolution /
- BDS/GPS /
- success rate /
- RTK /
- Lambda
-
-
![]()
图 3 3种部分模糊度与FULL方法基线解算精度比较
Figure 3. Contrast of Three Partial Ambiguities Resolution Methods with "FULL" Method about the Precision of Baseline Solution
![]()
图 4 4种模糊度固定方法解算的坐标误差结果
Figure 4. Performance of Four Ambiguity > Resolution Methods for "Baseline 2"
![]()
图 5 滤波过程中模糊度成功固定的个数
Figure 5. The Number of Successfully Fixed Ambiguities for "Baseline 2"
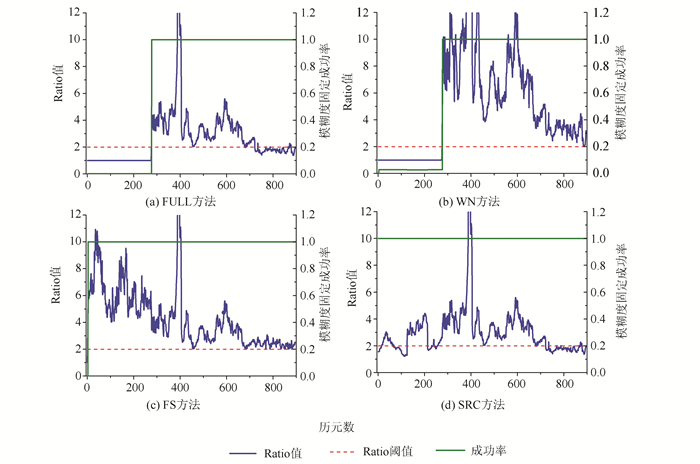
表 1 四种方法在不同的Ratio阈值下的固定率/%
Table 1 The Proportion of Successfully Fixed Epochs Under Different Ratio Threshold/%
Ratio阈值 FULL FS WN SRC 3.0 32.9 63.8 61.8 41.4 2.0 50.4 99.8 69.0 67.2 动态阈值 69.1 99.9 69.2 100 
下载: 导出CSV
表 2 4种解算方法的初始化时间及坐标误差结果
Table 2 The Initialization Time and Baseline Solution Error of Four Methods
模糊度固定方法 FULL FS WN SRC 初始化时间/s 910 20 500 640 N/m 0.040 0.012 0.014 0.030 E/m 0.063 0.016 0.016 0.036 U/m 0.111 0.030 0.042 0.082
下载: 导出CSV
-
[1] Teunissen P J G, et al. Geometry-free Ambiguity Success Rates in Case of Partial Fixing[C]. Proceedings of ION-NTM, San Diego, CA, 1999 http://www.researchgate.net/publication/266016106_Geometry-free_Ambiguity_Success_Rates_in_Case_of_Partial_Fixing
[2] 高星伟, 过静君, 程鹏飞, 等.基于时空系统统一的北斗与GPS融合定位[J].测绘学报, 2012, 41(5):743-748 http://kns.cnki.net/KCMS/detail/detail.aspx?filename=chxb201205021&dbname=CJFD&dbcode=CJFQ Gao Xingwei, Guo Jingjun, Cheng Pengfei, et al. Fusion Positioning of BeiDou/GPS Based on Spatio Temporal System Unification[J].Acta Geodaetica et Cartographica Sinica, 2012, 41(5):743-748 http://kns.cnki.net/KCMS/detail/detail.aspx?filename=chxb201205021&dbname=CJFD&dbcode=CJFQ
[3] Li Jinlong, Yang Yuanxi, Xu Junyi, et al. GNSS Multi-carrier Fast Partial Ambiguity Resolution Strategy Tested with Real BDS/GPS Dual-and Triple-frequency Observations[J]. GPS Solutions, 2015, 19(1):5-13 doi: 10.1007/s10291-013-0360-6
[4] Dai Liwen, Eslinger D, Sharpe T. Innovative Algorithms to Improve Long Range RTK Reliability and Availability[C]. Proceedings of the 2007 National Technical Meeting of the Institute of Navigation, San Diego, CA, 2007 http://www.researchgate.net/publication/242267642_Innovative_Algorithms_to_Improve_Long_Range_RTK_Reliability_and_Availability
[5] Wang Jun, Feng Yanming. Reliability of Partial Ambiguity Fixing with Multiple GNSS Constellations[J]. Journal of Geodesy, 2013, 87(1):1-14 doi: 10.1007/s00190-012-0573-4
[6] Li Pan, Zhang Xiaohong. Precise Point Positioning with Partiatial Ambiguity Fixing[J]. Sensors, 2015, 15(6):13627-13643 doi: 10.3390/s150613627
[7] Parkins A. Increasing GNSS RTK Availability with a New Single-epoch Batch Partial Ambiguity Resolution Algorithm[J]. GPS Solutions, 2011, 15(4):391-402 doi: 10.1007/s10291-010-0198-0
[8] Takasu T, Yasuda A. Kalman-filter-based Integer Ambiguity Resolution Strategy for Long-baseline RTK with Ionosphere and Troposphere Estimation[C]. Proceedings of the 23rd International Technical Meeting of the Satellite Division of the Institute of Navigation, Portland, OR, 2010 https://www.researchgate.net/publication/260387204_Kalman-Filter-Based_Integer_Ambiguity_Resolution_Strategy_for_Long-Baseline_RTK_with_Ionosphere_and_Troposphere_Estimation
[9] Cao Wei, O'Keefe K, Cannon M. Partial Ambiguity Fixing within Multiple Frequencies and Systems[C]. Proceedings of ION GNSS, Fort Worth, TX, 2007 http://www.researchgate.net/publication/250305744_Partial_Ambiguity_Fixing_within_Multiple_Frequencies_and_Systems
[10] Verhagen S, Teunissen P J G. The Ratio Test for Future GNSS Ambiguity Resolution[J]. GPS Solutions, 2013, 17(4):535-548 doi: 10.1007/s10291-012-0299-z
[11] Verhagen S, Teunissen P J G, van der Marel H, et al. GNSS Ambiguity Resolution:Which Subset to Fix[C]. Proceedings of IGNSS Symposium, Sydney, Australia, 2011 http://www.researchgate.net/publication/236213381_GNSS_ambiguity_resolution_which_subset_to_fix
[12] Teunissen P J G. GNSS Ambiguity Bootstrapping:Theory and Application[C]. Proceedings of International Symposium on Kinematic Systems in Geodesy, Geomatics and Navigation, Banff, Canada, 2001 http://www.researchgate.net/publication/228870630_GNSS_ambiguity_bootstrapping_theory_and_application
[13] Teunissen P J G. Success Probability of Integer GPS Ambiguity Rounding and Bootstrapping[J]. Journal of Geodesy, 1998, 72(10):606-612 doi: 10.1007/s001900050199
[14] Teunissen P J G. Instantaneous BeiDou+ GPS RTK Positioning with High Cut-off Elevation Angles[J]. Journal of Geodesy, 2014, 88(4):335-350 doi: 10.1007/s00190-013-0686-4
[15] Teunissen P J G. The Least-squares Ambiguity Decorrelation Adjustment:A Method for Fast GPS Integer Ambiguity Estimation[J]. Journal of Geodesy, 1995, 70(1/2):65-82 https://www.researchgate.net/publication/224969472_The_least-squares_ambiguity_decorrelation_adjustment_A_method_for_fast_GPS_integer_ambiguity_estimation
[16] Verhagen S. On the Approximation of the Integerleast-squares Success Rate:Which Lower or Upperbound to Use[J]. Journal of Global Positioning Systems, 2003, 2(2):117-124 doi: 10.5081/jgps
-
期刊类型引用(9)
1. 陈嘉琦，陈亮，林巍. GNSS钟差估计中的两种测站选取策略分析. 地理空间信息. 2022(11): 64-69 . 
百度学术
2. 刘赞，刘晓鹏，陈西宏，谢泽东，刘强. 对流层散射无源监视系统最优布局方法. 国防科技大学学报. 2021(01): 103-108 . 百度学术
3. 胡超，王中元，王潜心，饶鹏文. 一种改进的BDS-2/BDS-3联合精密定轨系统偏差处理模型. 武汉大学学报(信息科学版). 2021(03): 360-370 . 百度学术
4. 张磊. 基于超精密激光辅助的运动训练图像去噪处理模型研究. 激光杂志. 2021(04): 114-120 . 百度学术
5. 胡超，王潜心，毛亚. 一种基于DOP值的GNSS超快速观测轨道精化模型. 武汉大学学报(信息科学版). 2020(01): 28-37 . 百度学术
6. 杨鸿毅，王潜心，毛亚，胡超，何义磊. BDS-3观测数据质量分析及精密定轨. 导航与控制. 2019(06): 55-61 . 百度学术
7. 毛亚，王潜心，胡超，何义磊. 北斗在轨卫星广播星历精度分析. 宇航学报. 2018(09): 1013-1021 . 百度学术
8. 何颖，马戎，权绎弘，郭强. 标志点定向系统几何精度因子的计算方法. 测控技术. 2018(12): 66-69 . 百度学术
9. 毛亚，王潜心，于伟宣，胡超，张铭彬. 多卫星导航系统钟差解算效率分析. 金属矿山. 2017(10): 59-62 . 百度学术
其他类型引用(11)
计量
- 文章访问数:
- HTML全文浏览量:
- PDF下载量:
- 被引次数: 20
