时序InSAR的误差模型建立及模拟研究

何平; 许才军; 温扬茂; 丁开华; 王琪

doi:10.13203/j.whugis20140369

时序InSAR的误差模型建立及模拟研究

何平^{1, 2, 3,},
许才军^{2, 3},
温扬茂²,
丁开华^{1, 4},
王琪¹

1.
中国地质大学地球物理与空间信息学院, 地球内部多尺度成像湖北省重点实验室, 湖北武汉, 430074
2.
武汉大学测绘学院, 湖北武汉, 430079
3.
武汉大学地球空间环境与大地测量教育部重点实验室, 湖北武汉, 430079
4.
中国地质大学信息与工程学院, 湖北武汉, 430074

基金项目:

中国博士后科学基金 No. 2015M57228

湖北省地球内部多尺度成像重点实验室开放基金 No. SMIL-2015-01

中央高校基本科研业务费专项资金 No.CUGL150810

武汉大学地球空间环境与大地测量教育部重点实验室开放研究基金 Nos. 13-02-11, 14-01-01

地理空间信息工程国家测绘地理信息局重点试验室开放基金 No. 201421

详细信息

作者简介:
何平,博士,主要从事用于地表形变的InSAR和GPS时序分析的集成研究。phe@cug.edu.cn

中图分类号: P228
计量
- 文章访问数: 2243
- HTML全文浏览量: 145
- PDF下载量: 481
出版历程
- 收稿日期: 2015-09-07
- 发布日期: 2016-06-04

Analysis and Simulation for Time Series InSAR Error Model

HE Ping^{1, 2, 3,},
XU Caijun^{2, 3},
WEN Yangmao²,
DING Kaihua^{1, 4},
WANG Qi¹

1.
Hubei Subsurface Multi-scale Imaging Key Laboratory, Institute of Geophysics and Geomatics, China University of Geosciences, Wuhan 430074, China
2.
School of Geodesy and Geomatics, Wuhan University, Wuhan 430079, China
3.
Key Laboratory of Geospace Environment and Geodesy, Ministry of Education, Wuhan University, Wuhan 430079, China
4.
Faculty of Information Engineering, China University of Geosciences, Wuhan 430074, China

Funds:

The China Postdoctoral Science Foundation No. 2015M57228

the Basic Fund of Hubei Subsurface Multi-scale Imaging Key Laboratory, Institute of Geophysics and Geomatics, China University of Geosciences, Wuhan No. SMIL-2015-01

the Fundamental Research Funds for the Central Universities No.CUGL150810

the Basic Research Fund of Key Laboratory of Geospace Environment and Geodesy, Ministry of Education of China Nos. 13-02-11, 14-01-01

the Open Funds of Key Laboratory of Geo-Informatics of State Bureau of Surveying and Mapping No. 201421

More Information

Author Bio:
HE Ping, PhD, specializes in the integration of InSAR and GPS time series analysis for surface deformation. E-mail: phe@cug.edu.cn

摘要

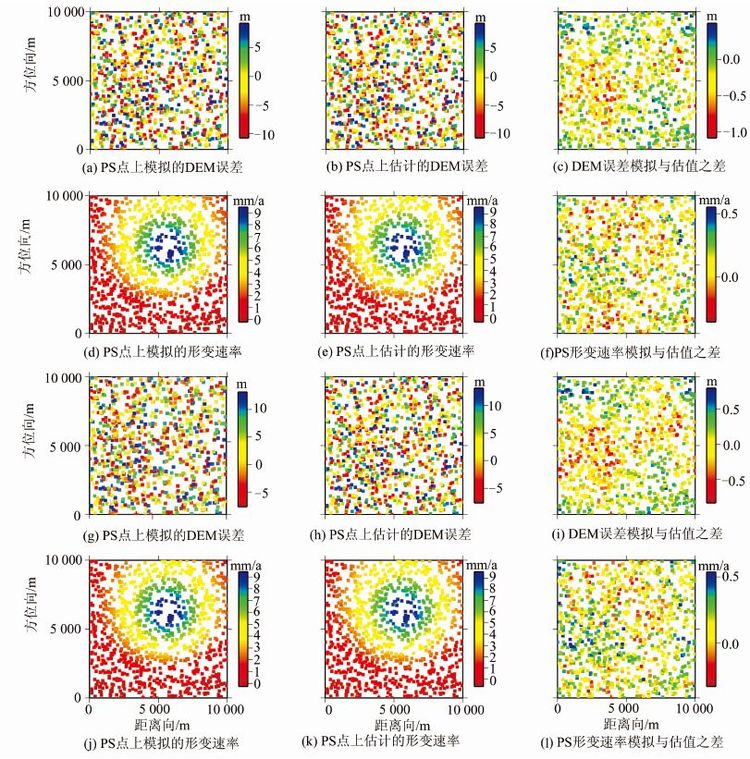
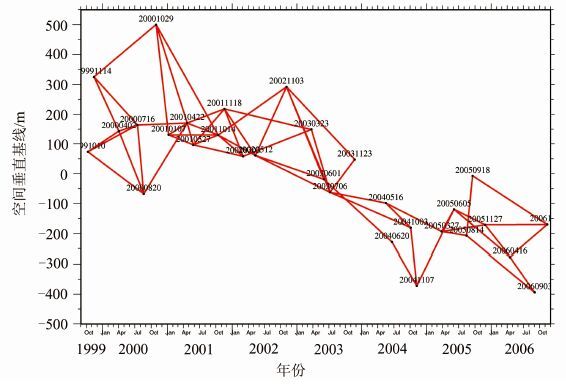
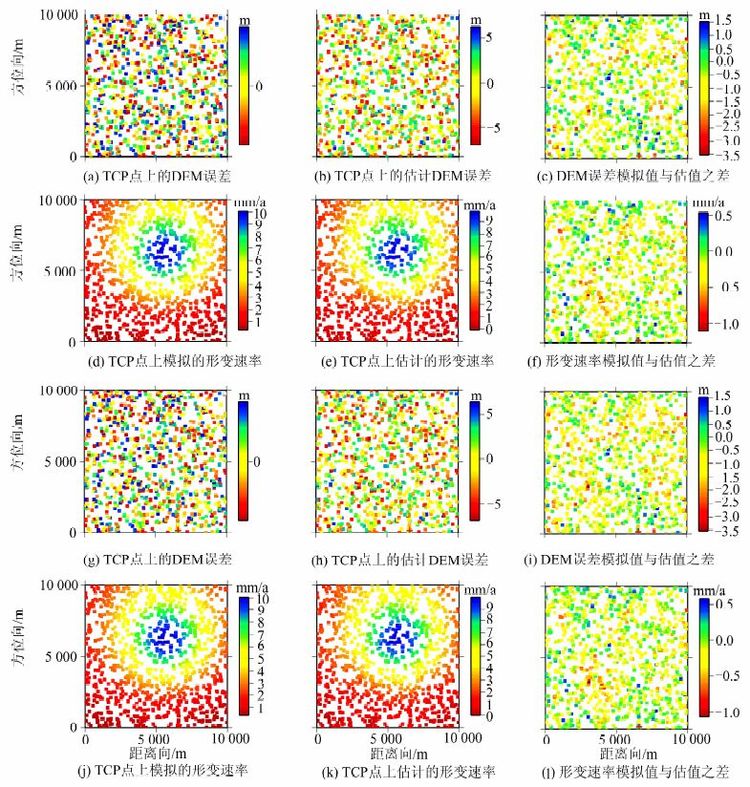
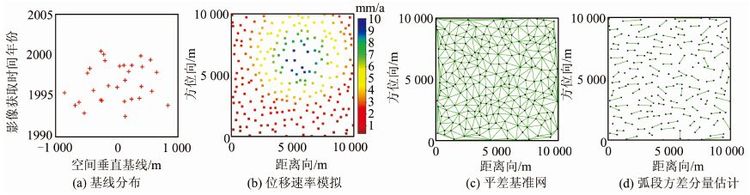
摘要: 基于时序InSAR函数模型,分别建立了单主影像和多主影像时序InSAR误差模型,在理论上完善了时序InSAR数学模型。利用构建的随机误差模型,模拟试验研究了随机误差模型对时序InSAR待估参数精度的影响。结果表明,与等权模型相比较,加权模型(随机误差模型)估计的参数精度有一定提高;但由于加权条件下的参数估计模型复杂、计算效率低,目前利用等权方法进行时序InSAR的参数估计更简便易行。
- 时序InSAR /
- 随机模型 /
- 参数估计
Abstract: Based on the function model of TS-InSAR (time series interferometric synthetic aperture radar) technique, this paper establishes stochastic function models (weighted/equal-weight) for single-master and multi-master image TS-InSAR method respectively to enrich the mathematical model of TS-InSAR. A simulation test is used to estimate the precision of model parameters for our equal-weighted function model (stochastic model). Compared to the equal-weight model, the precision of deformation parameters shows little improvement over the weighted model. However, complications in the weighted model means that much disk-space is consumed with low computational efficiency. Most computers therefore cannot undertake TS-InSAR analysis tasks with reasonable hardware configuration. At present, the equal-weight model is feasible for TS-InSAR.
- TS-InSAR /
- stochastic model /
- parameter estimation

HTML全文

致谢: 感谢Kampes博士提供的STUN软件包和张磊博士提供的部分Matlab代码。

图 1 单主影像的时序InSAR算法数据模拟

Figure 1. Simulation for Single-Master Image TS-InSAR

下载: 全尺寸图片幻灯片

图 2 单主影像时序InSAR等权(a~f)与随机模型加权(g~l)参数估计模拟

Figure 2. Parameters Estimated by Equal-Weight Model and Weighted Model for Single-Master Image TS-InSAR

下载: 全尺寸图片幻灯片

图 3 多主影像时序InSAR模拟干涉像对时空基线分布

Figure 3. Distribution of Temporal Spatial Baseline for Multi-master Image TS-InSAR

下载: 全尺寸图片幻灯片

图 4 多主影像时序InSAR等权(a)~(f)与随机模型加权(g)~(l)参数估计模拟

Figure 4. Parameters Estimated by Equal-Weight Model and Weighted Model for Multi-master Image TS-InSAR

下载: 全尺寸图片幻灯片

表 1 等权与加权模型的参数估计误差

Table 1 Parameters Estimation Error by Equal-Weight Model and Weighted Model

			最大值	最小值	平均值	均方差	计算耗时/s
单主影像	等权	DEM/m	-1.1	0.5	-0.2	0.3	213
	等权	形变速率/(mm\5a^-1)	0.55	-0.36	0.1	0.16	213
	加权	DEM/m	-0.83	0.80	0.02	0.29	731
	加权	形变速率/(mm\5a^-1)	0.54	-0.33	0.14	0.15	731
多主影像	等权	DEM/m	-3.5	1.5	-0.7	0.7	329
	等权	形变速率/(mm\5a^-1)	-1.1	0.54	-0.17	0.23	329
	加权	DEM/m	-3.5	1.8	-0.6	0.7	1 014
	加权	形变速率/(mm\5a^-1)	-1.07	0.58	-0.12	0.22	1 014

下载: 导出CSV

参考文献(17)

[1]	Sandwell D T, Sichoix L. Topographic Phase Recovery From Stacked ERS Interferometry and a Low-Resolution Digital Elevation Model[J]. Journal of Geophysical Research:Solid Earth (1978-2012), 2000, 105(B12):28211-28222
[2]	Ferretti A, Prati C, Rocca F. Nonlinear Subsidence Rate Estimation Using Permanent Scatterers in Differential SAR Interferometry[J]. IEEE Transactions on Geoscience and Remote Sensing, 2000, 38(5):2202-2212
[3]	Usai S. A New Approach for Long Term Monitoring of Deformations by Differential SAR Interferometry[D]. Netherlands:Delft University of Technology, 2001
[4]	Berardino P, Fornaro G, Fusco A, et al. A New Approach for Analyzing the Temporal Evolution of Earth Surface Deformations Based on the Combination of DIFSAR Interferograms[C]. IGARSS, Sydney, 2001
[5]	Blanco-Sanchez P, Mallorqui J J, Duque S, et al. The Coherent Pixels Technique (CPT):An Advanced Dinsar Technique for Nonlinear Deformation Monitoring[J]. Pure and Applied Geophysics, 2008, 165(6):1167-1193
[6]	Zhang L, Ding X, Lu Z. Ground Settlement Monitoring Based on Temporarily Coherent Points Between Two SAR Acquisitions[J]. Journal of Photogrammetry and Remote Sensing, 2011, 66(1):146-152
[7]	GonzaLez P J, FernaNdez J. Error Estimation in Multitemporal InSAR Deformation Time Series, with Application to Lanzarote, Canary Islands[J]. Journal of Geophysical Research:Solid Earth (1978-2012), 2011, 116, DOI: 10.1029/2011JB008412
[8]	何平,温扬茂,许才军,等.多时相InSAR技术的廊坊地区地下水体积变化研究[J].武汉大学学报·信息科学版,2012,37(10):1181-1185 He Ping, Wen Yangmao, Xu Caijun, et al. Volume Change of Groundwater in Langfang Region Derived from Multi-temporal InSAR[J]. Geomatics and Information Science of Wuhan University, 2012, 37(10):1181-1185
[9]	Hooper A, Zebker H, Segall P, et al. A New Method for Measuring Deformation on Volcanoes and Other Natural Terrains Using InSAR Persistent Scatterers[J]. Geophysical Research Letters, 2004, 31(23):275-295
[10]	Kwoun O I, Lu Z, Neal C, et al. Quiescent Deformation of the Aniakchak Caldera, Alaska, Mapped by InSAR[J]. Geology, 2006, 34(1):5-8
[11]	Anderssohn J, Motagh M, Walter T R, et al. Surface Deformation Time Series and Source Modeling for a Volcanic Complex System Based on Satellite Wide Swath and Image Mode Interferometry:the Lazufre System, Central Andes[J]. Remote Sensing of Environment, 2009, 113(10):2062-2075
[12]	Ketelaar V B H. Satellite Radar Interferometry:Subsidence Monitoring Techniques[M]. Netherlands:Springer, 2009
[13]	Kampes B M, Hanssen R F. Ambiguity Resolution for Permanent Scatterers Interferometry[J]. IEEE Transactions on Geoscience and Remote Sensing, 2004, 42(11):2446-2453
[14]	Hanssen R F. Radar Interferometry:Data Interpretation and Error Analysis[M]. New York:Springer, 2001
[15]	Kampes B M. Radar Interferometry:Persistent Scatterer Technique[M]. New York:Springer, 2006
[16]	Sudhaus H, Sigurjon J. Improved Source Modelling Through Combined Use of InSAR and GPS Under Consideration of Correlated Data Errors:Application to the June 2000 Kleifarvatn Earthquake, Iceland[J]. Geophysical Journal International, 2009, 176(2):389-404
[17]	许才军,何平,温扬茂.利用PSInSAR研究意大利Etna火山的地表形变[J].武汉大学学报·信息科学版,2011,36(9):1012-1016 Xu Caijun, He Ping, Wen Yangmao. Surface Deformation of Mt. Etna, Italy from PSInSAR[J]. Geomatics and Information Science of Wuhan University, 2011, 36(9):1012-1016

施引文献(18)

期刊类型引用(12)

1.	明小勇，田义超，张强，陶进，张亚丽，林俊良. 基于Sentinel-1A钦防地区地面沉降监测与分析. 自然资源遥感. 2024(01): 35-48 . 百度学术
2.	周茂桐，张永红，康永辉，吴宏安，魏钜杰. 一种基于结构函数的干涉图筛选方法. 测绘科学. 2024(02): 89-97 . 百度学术
3.	朱军桃，兰荣添，李海林，代程远，林知宇. 基于时序InSAR的厦门市地面沉降监测与分析. 海洋测绘. 2023(05): 56-60+66 . 百度学术
4.	郭伟，陈戈，郭帅，石中计. PS-InSAR技术在安徽省阜南县地面沉降监测中的应用. 安徽地质. 2023(04): 353-358 . 百度学术
5.	何希山，周平，陈刚. 一种联合GACOS与线性大气校正的StaMPS PSI时序分析方法. 测绘通报. 2022(10): 105-109+128 . 百度学术
6.	毕建涛，石振，祝彦敏. 北斗+InSAR技术在大坝变形及周边地质灾害监测中的应用. 卫星应用. 2021(11): 29-33 . 百度学术
7.	王硕，王晨阳，陈常松. 测绘业务与地质灾害监测预警业务之间的融合途径分析. 测绘通报. 2020(11): 151-154 . 百度学术
8.	高二涛，范冬林，付波霖，雍琦，兰艳萍. 基于PS-InSAR和SBAS技术监测南京市地面沉降. 大地测量与地球动力学. 2019(02): 158-163 . 百度学术
9.	肖儒雅，何秀凤. 时序InSAR水库大坝形变监测应用研究. 武汉大学学报(信息科学版). 2019(09): 1334-1341 . 百度学术
10.	余洁，王少华，焦帅，朱琳，常占强，陈蜜. 时空三维相位解缠算法改进研究. 武汉大学学报(信息科学版). 2019(09): 1355-1362 . 百度学术
11.	葛大庆，戴可人，郭兆成，李振洪. 重大地质灾害隐患早期识别中综合遥感应用的思考与建议. 武汉大学学报(信息科学版). 2019(07): 949-956 . 百度学术
12.	蓝秦隆，邹进贵. SBAS技术在地面沉降监测中的应用——以武汉市为例. 测绘通报. 2018(S1): 278-282 . 百度学术